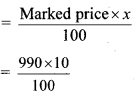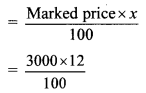Balbharti Maharashtra State Board Class 8 Maths Solutions covers the Practice Set 9.2 8th Std Maths Answers Solutions Chapter 9 Discount and Commission.
Discount and Commission Class 8 Maths Chapter 9 Practice Set 9.2 Solutions Maharashtra Board
Std 8 Maths Practice Set 9.2 Chapter 9 Solutions Answers
Question 1.
John sold books worth Rs 4500 for a publisher. For this he received 15% commission. Complete the following activity to find the total commission John obtained.
Solution:
Selling price of the books = Rs 4500
Rate of commission = 15%
Commission obtained = 15% of selling price
\(=\frac{[15]}{[100]} \times[4500]\)
= 15 × 45
∴ Commission obtained = 675 Rupees.
∴ The total commission obtained by John is Rs 675.
Question 2.
Rafique sold flowers worth Rs 15,000 by giving 4% commission to the agent. Find the commission he paid. Find the amount received by Rafique.
Solution:
Here, selling price of flowers = Rs 15,000,
Rate of commission = 4%
i. Commission = 4% of selling price
= \(\frac { 4 }{ 100 }\) × 15,000
= 4 x 150
∴ Commission = Rs 600
ii. Amount received by Rafique = selling price – commission
= 15,000 – 600
= Rs 14,400
∴ Rafique paid Rs 600 as commission and the amount received by him was Rs 14,400.
Question 3.
A farmer sold food grains for Rs 9200 through an agent. The rate of commission was 2%. How much amount did the agent get ?
Solution:
Here, selling price of food grains = Rs 9200,
Rate of commission = 2%
Commission = 2% of selling price
= \(\frac { 2 }{ 100 }\) × 9200
= 2 × 92
= Rs 184
∴ The agent got a commission of Rs 184.
Question 4.
Umatai purchased following items from a Khadi – Bhandar.
i. 3 sarees for Rs 560 each.
ii. 6 bottles of honey for Rs 90 each.
On the purchase, she received a rebate of 12%. How much total amount did Umatai pay?
Solution:
Here, number of sarees = 3,
Price of each saree = Rs 560
∴ Cost of 3 sarees = 560 × 3
= Rs 1680 …(i)
Also, number of honey bottles = 6,
Price of each bottle = Rs 90
∴ Cost of 6 honey bottles = 90 × 6
= Rs 540
Total amount of purchase
= cost of 3 sarees + cost of 6 honey bottles
= 1680 + 540 … [From (i) and (ii)]
= Rs 2220 …(iii)
Rate of rebate = 12%
Rebate = 12% of total amount of purchase
= \(\frac { 12 }{ 100 }\) × 2220
= 12 × 22.20
= Rs 266.40 ..(iv)
Amount paid by Umatai
= Total amount of purchase – Rebate
= 2,220 – 266.40 … [From (iii) and (iv)]
= Rs 1953.60
∴ The total amount paid by Umatai is Rs 1953.60.
Question 5.
Use the given information and fill in the boxes with suitable numbers.
Smt. Deepanjali purchased a house for Rs 7,50,000 from Smt. Leelaben through an agent. Agent has charged 2 % brokerage from both of them.
Solution:
i. Smt. Deepanjali paid 7,50,000 × \(\frac { 2 }{ 100 }\)
= 7,500 × 2 = Rs 15,000 brokerage for purchasing the house.
ii. Smt. Leelaben paid brokerage of Rs 15,000
iii. Total brokerage received by the agent is = 15,000 + 15,000 = Rs 30,000
iv. The cost of house Smt. Deepanjali paid is = 7,50,000 + 15,000 = Rs 7,65,000
v. The selling price of house for Smt.Leelaben is = 7,50,000 – 15,000
= Rs 7,35,000
Std 8 Maths Digest