Balbharti Maharashtra State Board Class 12 Economics Solutions Chapter 1 Introduction to Micro and Macro Economics Textbook Exercise Questions and Answers.
Std 12 Economics Chapter 1 Question Answer Introduction to Micro and Macro Economics Maharashtra Board
Class 12 Economics Chapter 1 Introduction to Micro and Macro Economics Question Answer Maharashtra Board
Economics Class 12 Chapter 1 Question Answer Maharashtra Board
1. Choose the correct option:
Question 1.
The branch of economics that deals with the allocation of resources.
a) Microeconomics
b) Macroeconomics
c) Econometrics
d) None of these
Options:
1) a, b and c
2) a and b
3) only a
4) None of these
Answer:
3) only a
![]()
Question 2.
Concepts studied under Micro economics.
a) National income
b) General price level
c) Factor pricing
d) Product pricing
Options :
1) b and c
2) b, c and d
3) a, b and c
4) c and d
Answer:
4) c and d
Question 3.
Method adopted in micro economic analysis.
a) Lumping method
b)Aggregative method
c) Slicing method
d) Inclusive method
Options :
1) a, c and d
2) a. b and d
3) only c
4) only a
Answer:
3) only c
Question 4.
Concepts studied under Macro economics.
a) Whole economy
b) Economic development
c) Aggregate supply
d) Product pricing
Options:
1) a, b and c
2) b, c and d
3) only d
4) a, b, c and d
Answer:
1) a, b and c
![]()
2. Complete the correlation:
1) Micro economics : Slicing method : : Macro economics: ……………. 1
2) Micro economics: Tree : : Macro economics: …………….. 2
3) Macro economic theory : Income and employment:: Micro economics : ……………. 4
4) Makros : Macro economics:: Mikros : ……………… 3
5) General equilibrium : Macro economics :: …………….. : Micro economics 5
Answers:
- Lumping method
- Forest
- Price theory
- Micro economics
- partial equilibrium
3. Identify and explain the concepts from the given illustrations:
Question 1.
Gauri collected the information about the income of a particular firm.
Answer:
Concept: Micro economics / Slicing method.
Explanation : Micro economics refers to the study of small unit from whole economy. Micro economics uses slicing method to split the whole economy into small individual units.
Gauri has used slicing method from micro economics to collect information about the income of a particular firm from various firms.
Question 2.
Ramesh decided to take all decisions related to production, such as what and how to produce?
Answer:
Concept: Free market economy.
Explanation : A free market economy is that economy where the economic decisions regarding production of goods are taken at individual level.
Eg. What to produce? How much to produce? How to produce? etc. decisions are taken by producers.
With the help of free market economy Ramesh has taken decision related to production such as What to produce? and How to produce?
Question 3.
Shabana paid wages to workers in her factory and interest on her bank loan.
Answer:
Concept : Factor Pricing.
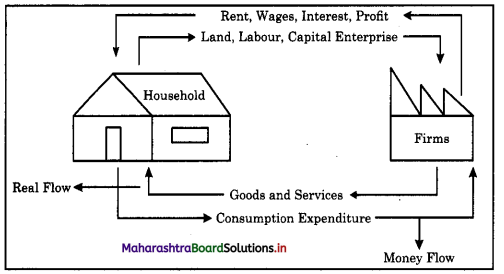
Explanation : Theory of factor pricing refers to determining the factor rewards for land, labour, capital and entrepreneur in the form of rent, wages, interest and profit respectively.
Shabana is an entrepreneur who has paid wages to its worker in a factory for production of goods and also paid interest on her bank loan in form of rewards to the factors of production.
![]()
4. Answer the following:
Question 1.
Explain the features of Micro economics.
OR
Explain the characteristics or nature of micro economics.
Answer:
Features of Micro Economics :
- Based on certain ssumption : Micro economics is based on ‘ceteris paribus’ assumption i.e., other things remaining constant like full employment, laissez faire policy, perfect competition, pure capitalism, etc.
- Study of Individual units : Micro economics deals with the study of behaviour of small individual units of the economy such as individual units of the economy such as individual consumer, individual firm, individual industries, individual prices, etc.
- Slicing Method : It divides or slices the economy into small units and studies each unit in detail e.g. study of a particular household demand in detail.
- Analysis of Market Structures : Micro economics analyses different market structures such as perfect competition, monopoly, monopolistic competition, oligopoly, etc.
- Use of Marginalism Principle : The term ‘marginal’ means change brought in total by an additional unit. Marginal analysis helps to study a variable through the changes by which producers and consumers take economic decisions using this principle.
- Price Theory : Micro economics is known as price theory because it determines the prices of goods and services as well as prices of factors of production.
- Limited Scope : The study of micro economics is limited to individual economic unit only. It does not deal with macro problems like unemployment, inflation, deflation, poverty, unemployment, population, etc.
- Partial I quilibrium : Micro economics analysis deals with partial equilibrium which analyses equilibrium position of an individual economic unit i.e. individual consumer, individual firm, etc.
Question 2.
Explain the importance of Macro economics.
Answer:
Importance of Macro Economics :
- Functioning of an Economy : It gives an idea of functioning of an economic system and help us to understand the behavioural pattern of aggregate variables.
- Economic fluctuations : It helps to analyse the causes of fluctuation in income, output and employment.
- National Income : It helps to study about National Income and makes possible to formulate correct economic policies.
- Economic Development : It helps us to understand the problems of the developing countries such as poverty, difference in the standards of living, etc., and suggest important steps to achieve economic development.
- Performance of an Economy : It helps us to analyse the performance of an economy where National Income estimates are used to measure the same.
- Study of Macro-economic Variables :
Study of macro economic variables are important to understand the working of the economy. - Level of Employment : Macro economics helps to analyse the general level of employment and output in an economy.
![]()
Question 3.
Explain the scope of Macro economics.
OR
“Scope of Macro Economics is wide.” Explain.
OR
Macro Economics is comprehensive in nature.
OR
Explain the subject matter of macro economics.
Answer:
Scope of Macro Economics:
The given chart helps us to understand the scope of macro economics.
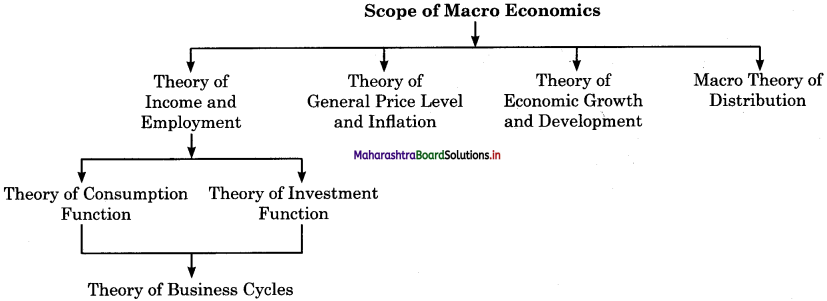
1. Theory of Income and Employment : It explains which factors determine the level of National Income and employment and what j causes fluctuations in the level of income, output and employment.
To understand how the level of employment is determined, we have to study the consumption function. It includes theory of business cycles.
2. Theory of General Price Level and Inflation: Macro economics analyses shows how the general price level is determined and the causes for fluctuations in it. This study is important for understanding the problems created by inflation and deflation.
3. Theory of Economic Growth and Development : Macro economics studies the causes of under development and poverty in poor countries and suggests strategies for accelerating growth and development in the country.
4. Macro theory of Distribution : Macro theory of distribution deals with the relative share of rent, wages, interest and profit in j the total national income of various classes.
5. State with reasons whether you agree or disagree with the following statements:
Question 1.
The scope of micro economics is unlimited.
Answer:
No, I do not agree with this statement.
- Micro economics deals with small or individual units.
- Micro economics is the study of particular firm, particular household, individual prices, wages, incomes, individual industries, particular commodities.
- Micro economics deals with small part of National economy. It does not deal with whole economy like National income, Aggregate demand, Aggregate supply, poverty, inflation, etc.
- Hence, the scope of micro economics is limited.
Question 2.
Macro economics deals with the study of individual behaviour.
OR
Macro economics studies small units.
Answer:
No, I do not agree with this statement.
OR
Macro Economics is the study of I aggregate.
OR
Macro economics is concerned with macro economic variables.
Yes, I agree with this statement.
- Macro Economics studies the behaviour ofthe economy as a whole and not individual behaviour.
- It studies about larger economic units or aggregate economic variables like aggregate demand, aggregate supply, total investment, total savings, total employment, etc.
- It studies the general price level and macro theory of distribution.
- Whereas Micro Economics deals with individual behaviour of the people in the economy. It studies about individual demand, market demand, individual income, price of particular commodity etc.
- According to Prof. Kenneth E. Boulding “Macro Economics deals not with individual; quantities as such, but with aggregates of these quantities, not with individual income but with National Income, not with individual prices but with general price level, not with individual output but with National Output.
![]()
Question 3.
Macro economics is different from micro economics.
OR
Macro economics is wider than Micro economics.
OR
There is difference between micro economics and macro economics.
Answer:
Yes, I agree with this statement.
- Micro economics is a study of a particular unit of an economy. On the other hand macro economics is the study of entire economy.
- Micro economics studies individual demand, individual supply, individual income, price determination of particular product, etc. On the other hand macro economics studies aggregate demand, aggregate supply, national income, etc.
- Micro economics follows partial equilibrium analysis and macro economics follows general equilibrium analysis.
- Micro economics uses slicing method for study of small unit and macro economics uses lumping method for study of large unit.
- Therefore, macro economics is different from micro economics.
Question 4.
Micro economics uses slicing method.
Answer:
Yes, I agree with this statement.
- Micro economics deals with small or individual units.
- Micro economics divides or slices the economy into small units and studies each unit in detail.
- It is concerned with microscopic study of these units.
- It is the study of particular firm, particular household, individual prices, wages, incomes, etc.
- Hence, micro economics uses slicing method.
Question 5.
Micro economics is known as Income theory.
Answer:
No, I do not agree with this statement.
OR Micro economics is also known as price theory.
Yes, I agree with this statement.
- Micro Economics is known as ‘Price Theory’.
- The scope of micro economics includes the study of product pricing and factor pricing.
- The theory of product pricing explains how the price of food grains, vegetables, clothes, etc., are determined.
- They are determined by the interaction of market demand and supply forces.
- The theory of factor pricing explains the distribution of factor income such as rent on land, wages to labourers, interest on capital and profit to entrepreneurs.
- The factor prices are also determined by the demand and supply forces.
- Therefore, Micro Economics is also known as ‘Price Theory’.
![]()
6. Answer in detail :
Question 1.
Explain the importance of Micro economics.
Answer:
Introduction : Micro economics is the Js branch of economics that studies the behaviour of individuals.
It includes individual prices, wages, income, individual industries, particular commodities, particular household, etc.
(1) Definition :
(a) According to Maurice Dobb – “Micro economics is in fact a microscopic study of l the economy.
(b) According to Prof. A. P. Lerner – “Micro economics consists of looking at the economy ? through a microscope as it were to see how the millions of cell in the body of economy – the individuals or households as consumers and individuals or firms as producers play their part in the working of the whole economics organism.
(2) Meaning:
Micro economics deals with small individual economics units such as an individual ( consumer, individual producer, the price of a particular commodity or factor etc.
(3) Importance :
(a) Price Determination : Micro economics j explains how the prices of different products < and various factprs of production are determined.
(b) Free Market Economy : A free market economy is that economy where the economic decisions are taken at individual levels without intervention by the government. Decisions are regarding production of goods such as What to produce? How much to produce? How to produce? etc.
(c) Foreign Trade : Micro economics also explains gains from foreign trade, effects of tarrifs, factors affecting exchange rate, etc.
(d) Economic Model Building : Micro
economics helps in understanding various complex economic situations with the help of economic models.
(e) Business Decision : Micro economics theories are helpful to businessman for taking important business decision related to determination of cost of production and prices of goods, maximization of output & profit, etc.
(f) Useful to Government : It is useful in formulating and evaluating economic policies including pricing and distribution policies that promote economic welfare. It is useful in determining tax policy, public, expenditure policy, etc.
(g) Basis of Welfare Economics : It explains how optimum use of resources can be made to increase the welfare of the society. It also studies how taxes affect social welfare.
Question 2.
Explain the concept of Macro economics and its features.
Answer:
Introduction : Macro economics is the branch of economics that studies the behaviour and performance of an economy as a whole. It includes inflation, unemployment, working of the monetary system, business cycles, economic policies, etc.
(1) Definition:
(a) J. L. Hansen : “Macro economics is that branch of economics which considers the relationship between large aggregates such as the volume of employment, total amount of savings, investment, national income, etc”.
(b) Prof. Carl Shapiro : “Macro economics deals with the functioning of the economy as a whole. ”
(2) Meaning:
Macro economics is the study of aggregates national income, total employment, total consumption, inflation, total saving, etc.
(3) Features:
(a) Study of Aggregate : Macro economics deals with the study of entire economy. It studies the overall condition in the economy, such as National Income, National Output, Total Employment, General Price levels, etc.
(b) General Price Level : Macro economic studies the determination and changes in general price level which is the average of all prices of goods and services currently being produced in the economy.
(c) policy Oriented : Macro economics is a policy oriented science which is useful in formulating economic policies to promote economic growth, to control inflation and depression, to generate employment, etc.
(d) Lumping Method : Lumping method is the study of the whole economy rather than in part. It considers aggregates like National Income, Total consumption, etc. instead of personal income, PCC, etc.
(e) General Equilibrium Analysis : Macro Economics analysis is based on general equilibrium which deals with the economic system as a whole and studies the inter relationships between the various macro variables in an economy. General equilibrium deals with the behaviour of demand, supply and prices in the whole economy.
(f) Income Theory : Macro economics studies the concept of National Income and its causes of fluctuations that lead to business cycles i.e. inflation and deflation.
(g) Growth Models : Macro economics studies various factors that contribute to economic growth and development. These growth models are used for studying economic development.
(h) Interdependence : There is an element of interdependence among the macro economic variables such as income, output, employment, investment, price level, etc.
Intext Questions
Try this (Textbook Page 6)
Visit the vegetable market in the nearest area and try to get information about income and expenditure items of a particular seller.
Answer:
[Note : Students should do this activity by themselves.]
12th Std Economics Questions And Answers:
- Introduction to Micro and Macro Economics Class 12 Economics Questions And Answers
- Utility Analysis Class 12 Economics Questions And Answers
- Demand Analysis Class 12 Economics Questions And Answers
- Elasticity of Demand Class 12 Economics Questions And Answers
- Supply Analysis Class 12 Economics Questions And Answers
- Forms of Market Class 12 Economics Questions And Answers
- Index Numbers Class 12 Economics Questions And Answers
- National Income Class 12 Economics Questions And Answers
- Public Finance in India Class 12 Economics Questions And Answers
- Money Market and Capital Market in India Class 12 Economics Questions And Answers
- Foreign Trade of India Class 12 Economics Questions And Answers