Prasanna

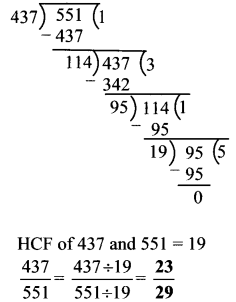
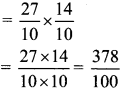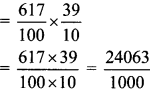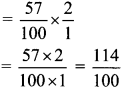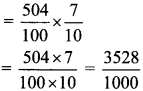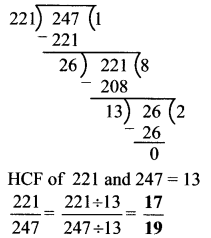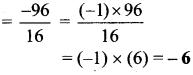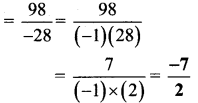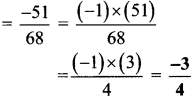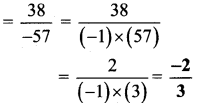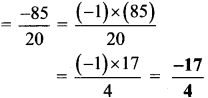Balbharti Maharashtra State Board Class 7 Maths Solutions covers the 7th Std Maths Practice Set 17 Answers Solutions Chapter 4 Angles and Pairs of Angles.

Angles and Pairs of Angles Class 7 Maths Chapter 4 Practice Set 17 Solutions Maharashtra Board

Std 7 Maths Practice Set 17 Solutions Answers

Question 1.
Write the measures of the supplements of the angles given below:
i. 15°
ii. 85°
iii. 120°
iv. 37°
v. 108°
vi. 0°
vii. a°
Solution:
i. Let the measure of the supplementary angle be x°.
∴ 15 + x = 180
∴ 15 + x – 15 = 180 – 15
….(Subtracting 15 from both sides)
∴ x = 165
∴ The measures of the supplement of an angle of 15° is 165°.

ii. Let the measure of the supplementary angle be x°.
∴ 85 + x = 180
∴ 85 + x – 85 = 180 – 85
….(Subtracting 85 from both sides)
∴ x = 95
∴ The measures of the supplement of an angle of 85° is 95°.

iii. Let the measure of the supplementary angle be x°.
∴ 120 + x = 180
∴ 120 + x – 120 = 180 – 120
….(Subtracting 120 from both sides)
∴ x = 60
∴ The measures of the supplement of an angle of 120° is 60°.

iv. Let the measure of the supplementary angle be x°.
∴ 37 + x = 180
∴ 37 + x – 37 = 180 – 37
….(Subtracting 37 from both sides)
∴ x = 143
∴ The measures of the supplement of an angle of 37° is 143°.

v. Let the measure of the supplementary angle be x°.
∴ 108 + x = 180
∴ 108 + x – 108 = 180 – 108
….(Subtracting 108 from both sides)
∴ x = 72
∴ The measures of the supplement of an angle of 108° is 72°.

vi. Let the measure of the supplementary angle be x°.
∴0 + x = 180
∴ x = 180
∴ The measures of the supplement of an angle of 0° is 180°.

vii. Let the measure of the supplementary angle be x°.
∴ a + x = 180
∴ a + x – a = 180 – a
….(Subtracting a from both sides) x = (180 – a)
∴ The measures of the supplement of an angle of a° is (180 – a)°.

Question 2.
The measures of some angles are given below. Use them to make pairs of complementary and supplementary angles.
m∠B = 60°
m∠N = 30°
m∠Y = 90°
m∠J = 150°
m∠D = 75°
m∠E = 0°
m∠F = 15°
m∠G = 120°
Solution:
i. m∠B + m∠N = 60° + 30°
= 90°
∴∠B and ∠N are a pair of complementary angles.

ii. m∠Y + m∠E = 90° + 0°
= 90°
∴∠Y and ∠E are a pair of complementary angles.

iii. m∠D + m∠F = 75° + 15°
= 90°
∴∠D and ∠F are a pair of complementary angles.

iv. m∠B + m∠G = 60° + 120°
= 180°
∴∠B and ∠G are a pair of supplementary angles.

v. m∠N + m∠J = 30° + 150°
= 180°
∴∠N and ∠J are a pair of supplementary angles.

Question 3.
In ΔXYZ, m∠Y = 90°. What kind of a pair do ∠X and ∠Z make?
Solution:
In ΔXYZ,
m∠X + m∠Y + m∠Z = 180° ….(Sum of the measure of the angles of a triangle is 180°)
∴m∠X + 90 + m∠Z = 180
∴m∠X + 90 + m∠Z – 90 = 180 – 90 ….(Subtracting 90 from both sides)
∴m∠X + m∠Z = 90°
∴∠X and ∠Z make a pair of complementary angles.

Question 4.
The difference between the measures of the two angles of a complementary pair is 40°. Find the measures of the two angles.
Solution:
Let the measure of one angle be x°.
∴Measure of other angle = (x + 40)°
x + (x + 40) = 90 …(Since, the two angles are complementary)
∴ 2x + 40 – 40 = 90 – 40 ….(Subtracting 40 from both sides)
∴2x = 50
∴x = \(\frac { 50 }{ 2 }\)
∴x = 25
∴x + 40 = 25 + 40
= 65
∴The measures of the two angles is 25° and 65°.

Question 5.
₹PTNM is a rectangle. Write the names of the pairs of supplementary angles.

Solution:
Since, each angle of the rectangle is 90°.
∴ Pairs of supplementary angles are:
i. ∠P and ∠M
ii. ∠P and ∠N
iii. ∠P and ∠T
iv. ∠M and ∠N
v. ∠M and ∠T
vi. ∠N and ∠T

Question 6.
If m∠A = 70°, what is the measure of the supplement of the complement of ∠A?
Solution:
Let the measure of the complement of ∠A be x° and the measure of its supplementary angle be y°.
m∠A + x = 90°
∴70 + x = 90
∴70 + x – 70 = 90 – 70 ….(Subtracting 70 from both sides)
∴x = 20
Since, x and y are supplementary angles.
∴x + y = 180
∴20 + y = 180
∴20 + y – 20 = 180 – 20 ….(Subtracting 20 from both sides)
∴y = 160
∴The measure of supplement of the complement of ∠A is 160°.

Question 7.
If ∠A and ∠B are supplementary angles and m∠B = (x + 20)°, then what would be m∠A?
Solution:
Since, ∠A and ∠B are supplementary angles.
∴m∠A + m∠B = 180
∴m∠A + x + 20 = 180
∴m∠A + x + 20 – 20 = 180 – 20 ….(Subtracting 20 from both sides)
∴m∠A + x = 160
∴m∠A + x – x = 160 – x ….(Subtracting x from both sides)
∴m∠A = (160 – x)°
∴The measure of ∠A is (160 – x)°.

Maharashtra Board Class 7 Maths Chapter 4 Angles and Pairs of Angles Practice Set 17 Intext Questions and Activities

Question 1.
Observe the figure and answer the following questions. (Textbook pg. no. 26)
T is a point on line AB.

1. What kind of angle is ∠ATB?
2. What is its measure?

Solution:

1. Straight angle
2. 180°

Std 7 Maths Digest

• Practice Set 15 Class 7 Answers
• Practice Set 16 Class 7 Answers
• Practice Set 17 Class 7 Answers
• Practice Set 18 Class 7 Answers
• Practice Set 19 Class 7 Answers
• Practice Set 20 Class 7 Answers
• Practice Set 21 Class 7 Answers

Balbharti Maharashtra State Board Class 6 Maths Solutions covers the Std 6 Maths Chapter 5 Decimal Fractions Class 6 Practice Set 16 Answers Solutions.

Decimal Fractions Class 6 Maths Chapter 5 Practice Set 16 Solutions Maharashtra Board

Std 6 Maths Practice Set 16 Solutions Answers

Question 1.
If, 317 × 45 = 14265, then 3.17 × 4.5 = ?
Solution:
3.17 × 4.5
= 14.265

Question 2.
If, 503 × 217 = 109151, then 5.03 × 2.17 = ?
Solution:
5.03 x 2.17
= 10.9151

Question 3.
i. 2.7 × 1.4
ii. 6.17 × 3.9
iii. 0.57 × 2
iv. 5.04 × 0.7
Solution:
i. 2.7 × 1.4

= 3.75

ii. 6.17 × 3.9

= 24.063

iii. 0.57 × 2

= 1.14

iv. 5.04 × 0.7

= 3.528

Question 4.
Virendra bought 18 bags of rice, each bag weighing 5.250 kg. How much rice did he buy altogether? If the rice costs Rs 42 per kg, how much did he pay for it?
Solution:
Weight of one bag of rice = 5.250 kg
Number of bags of rice = 18
∴ Total Weight = 18 × 5.250

Cost of 1 kg of rice = Rs 42
∴ Cost of 94.5 kg of rice = 42 × 94.5

∴ Total rice bought by Virendra is 94.5 kg, and the amount paid for it is Rs 3969.

Question 5.
Vedika has 23.5 metres of cloth. She used it to make 5 curtains of equal size. If each curtain required 4 metres 25 cm to make, how much cloth is left over?
Solution:
We know, that 1 m = 100 cm
Cloth required to make 1 curtain = 4 m 25 cm
= 4 m + \(\frac { 25 }{ 100 }\) m
= 4 m + 0.25 m

= 4.25 m
∴ Cloth required to make 5 curtains = 5 × 4.25

= 21.25 m
Cloth remaining with Vedika = Total cloth with Vedika – Cloth used
= 23.5 m – 21.25 m

= 2.25 m
∴ The length of cloth remaining with Vedika is 2.25 m.

Std 6 Maths Digest

• Practice Set 9 Class 6 Answers
• Practice Set 10 Class 6 Answers
• Practice Set 11 Class 6 Answers
• Practice Set 12 Class 6 Answers
• Practice Set 13 Class 6 Answers
• Practice Set 14 Class 6 Answers
• Practice Set 15 Class 6 Answers
• Practice Set 16 Class 6 Answers
• Practice Set 17 Class 6 Answers

Balbharti Maharashtra State Board Class 7 Maths Solutions covers the 7th Std Maths Practice Set 16 Answers Solutions Chapter 4 Angles and Pairs of Angles.

Angles and Pairs of Angles Class 7 Maths Chapter 4 Practice Set 16 Solutions Maharashtra Board

Std 7 Maths Practice Set 16 Solutions Answers

Question 1.
The measures of some angles are given below. Write the measures of their complementary angles.
i. 40°
ii. 63°
iii. 45°
iv. 55°
v. 20°
vi. 90°
vii. x°
Solution:
i. Let the measure of the complementary angle be x°.
∴ 40 + x = 90
∴ 40 + x – 40 = 90 – 40
….(Subtracting 40 from both sides)
∴ x = 50
∴ The measure of the complement of an angle of measure 40° is 50°.

ii. Let the measure of the complementary angle be x°.
∴ 63 + x = 90
∴ 63+x-63 = 90-63
….(Subtracting 63 from both sides)
∴ x = 27
∴ The measure of the complement of an angle of measure 63° is 27°.

iii. Let the measure of the complementary angle be x°.
∴ 45 + x = 90
∴ 45+x-45 = 90-45
….(Subtracting 45 from both sides)
∴ x = 45
∴ The measure of the complement of an angle of measure 45° is 45°.

iv. Let the measure of the complementary angle be x°.
∴ 55 + x = 90
∴ 55 + x-55 = 90-55
….(Subtracting 55 from both sides)
∴ x = 35
∴ The measure of the complement of an angle of measure 55° is 35°.

v. Let the measure of the complementary angle be x°.
∴ 20 + x = 90
∴ 20 + x – 20 = 90 – 20
….(Subtracting 20 from both sides)
∴ x = 70
∴ The measure of the complement of an angle of measure 20° is 70°.

vi. Let the measure of the complementary angle be x°.
∴ 90 + x = 90
∴ 90 + x – 90 = 90 – 90
….(Subtracting 90 from both sides)
∴ x = 0
∴ The measure of the complement of an angle of measure 90° is 0°.

vii. Let the measure of the complementary angle be a°.
∴ x + a = 90
∴ x + a – x = 90 – x
….(Subtracting x from both sides)
∴ a = (90 – x)
∴ The measure of the complement of an angle of measure x° is (90 – x)°.

Question 2.
(y – 20)° and (y + 30)° are the measures of complementary angles. Find the measure of each angle.
Solution:
(y – 20)° and (y + 30)° are the measures of complementary angles.
∴ (y – 20) + (y + 30) = 90
∴ y + y + 30 – 20 = 90
∴ 2y+10 = 90
∴ 2y = 90 – 10
∴ 2y = 80
∴ \(y=\frac { 80 }{ 2 }\)
= 40
Measure of first angle = (y – 20)° = (40 – 20)° = 20°
Measure of second angle = (y + 30)° = (40 + 30)° = 70°
∴ The measure of the two angles is 20° and 70°.

Maharashtra Board Class 7 Maths Chapter 4 Angles and Pairs of Angles Practice Set 16 Intext Questions and Activities

Question 1.
Observe the angles in the figure and enter the proper number in the empty place. (Textbook pg. no. 26)

1. m∠ABC = ___°.
2. m∠PQR = ___°.
3. m∠ABC + m∠PQR = ___°.

Solution:

1. 40
2. 50
3. 90

Note: Here, the sum of the measures of ∠ABC and ∠PQR is 90 °. Therefore, they are complementary angles.

Std 7 Maths Digest

• Practice Set 15 Class 7 Answers
• Practice Set 16 Class 7 Answers
• Practice Set 17 Class 7 Answers
• Practice Set 18 Class 7 Answers
• Practice Set 19 Class 7 Answers
• Practice Set 20 Class 7 Answers
• Practice Set 21 Class 7 Answers

Balbharti Maharashtra State Board Class 7 Maths Solutions covers the 7th Std Maths Practice Set 15 Answers Solutions Chapter 4 Angles and Pairs of Angles.

Angles and Pairs of Angles Class 7 Maths Chapter 4 Practice Set 15 Solutions Maharashtra Board

Std 7 Maths Practice Set 15 Solutions Answers

Question 1.
Observe the figure and complete the table for ∠AWB.

Points in the interior
Points in the exterior
Points on the arms of the angles

Solution:

Points in the interior	point C, point R, point N, point X
Points in the exterior	point T, point U, point Q, point V, point Y
Points on the arms of the angles	point A, point W, point G, point B

Question 2.
Name the pairs of adjacent angles in the figures below.

Solution:
i. ∠ANB and ∠ANC
∠BNA and ∠BNC
∠ANC and ∠BNC

ii. ∠PQR and ∠PQT

Question 3.
Are the following pairs adjacent angles? If not, state the reason.

1. ∠PMQ and ∠RMQ
2. ∠RMQ and ∠SMR
3. ∠RMS and ∠RMT
4. ∠SMT and ∠RMS

Solution:

1. ∠PMQ and ∠RMQ are adjacent angles.
2. ∠RMQ and ∠SMR not adjacent angles since they do not have separate interiors.
3. ∠RMS and ∠RMT not adjacent angles since they do not have separate interiors.
4. ∠SMT and ∠RMS are adjacent angles.

Maharashtra Board Class 7 Maths Chapter 4 Angles and Pairs of Angles Practice Set 15 Intext Questions and Activities

Question 1.
Observe the figure alongside and write the answers. (Textbook pg. no. 24)

1. Write the name of the angle shown alongside___.
2. Write the name of its vertex___.
3. Write the names of its arms___.
4. Write the names of the points marked on its arms___.

Solution:

1. ∠ABC
2. Point B
3. Ray BA, ray BC
4. Points A, B, C

Std 7 Maths Digest

• Practice Set 15 Class 7 Answers
• Practice Set 16 Class 7 Answers
• Practice Set 17 Class 7 Answers
• Practice Set 18 Class 7 Answers
• Practice Set 19 Class 7 Answers
• Practice Set 20 Class 7 Answers
• Practice Set 21 Class 7 Answers

Balbharti Maharashtra State Board Class 7 Maths Solutions covers the 7th Std Maths Practice Set 14 Answers Solutions Chapter 3 HCF and LCM.

HCF and LCM Class 7 Maths Chapter 3 Practice Set 14 Solutions Maharashtra Board

Std 7 Maths Practice Set 14 Solutions Answers

Question 1.
Choose the right option.
i. The HCF of 120 and 150 is __
(A) 30
(B) 45
(C) 20
(D) 120
Solution:
(A) 30

Hint:
120 = 2 x 2 x 2 x 3 x 5
150 = 2 x 3 x 5 x 5
∴ HCF of 120 and 150 = 2 x 3 x 5 = 30

ii. The HCF of this pair of numbers is not 1.
(A) 13,17
(B) 29,20
(C) 40, 20
(D) 14, 15
Solution:
(C) 40, 20

Hint:
40 = 2 x 2 x 2 x 5
20 = 2 x 2 x 5
∴ HCF of 40 and 20 = 2 x 5 = 10

Question 2.
Find the HCF and LCM.
i. 14,28
ii. 32,16
iii. 17,102,170
iv. 23,69
v. 21,49,84
Solution:
i. 14 = 2 x 7
28 = 2 x 14
= 2 x 2 x 7
∴ HCF of 14 and 28 = 2 x 7
= 14
LCM of 14 and 28 = 2 x 2 x 7
= 28

ii. 32 = 2 x 16
= 2 x 2 x 8
= 2 x 2 x 2 x 4
= 2 x 2 x 2 x 2 x 2
16 = 2 x 8
= 2 x 2 x 4
= 2 x 2 x 2 x 2
∴ HCF of 32 and 16 = 2 x 2 x 2 x 2
= 16
∴ LCM of 32 and 16 = 2 x 2 x 2 x 2 x 2
= 32

iii. 17 = 17 x 1
102 = 2 x 51
= 2 x 3 x 17
170 = 2 x 85
= 2 x 5 x 17
∴ HCF of 17, 102 and 170 = 17
∴ LCM of 17, 102 and 170 = 17 x 2 x 3 x 5
= 510

iv. 23 = 23 x 1
69 = 3 x 23
∴ HCF of 23 and 69 = 23
∴ LCM of 23 and 69 = 23 x 3
= 69

v. 21 = 3 x 7
49 = 7 x 7
84 = 2 x 42
= 2 x 2 x 21
= 2 x 2 x 3 x 7
∴ HCF of 21, 49 and 84 = 7
∴ LCM of 21, 49 and 84 = 7 x 3 x 7 x 2 x 2
= 588

Question 3.
Find the LCM.
i. 36, 42
ii. 15, 25, 30
iii. 18, 42, 48
iv. 4, 12, 20
v. 24, 40, 80, 120
Solution:
i. 36, 42

∴ LCM of 36 and 42 = 2 x 3 x 2 x 3 x 7
= 252

ii. 15, 25, 30

∴ LCM of 15, 25 and 30 = 5 x 3 x 5 x 2
= 150

iii. 18, 42, 48

∴ LCM of 18,42 and 48 = 2 x 3 x 2 x 2 x 3 x 7 x 2
= 1008

iv. 4, 12, 20

∴ LCM of 4, 12 and 20 = 2 x 2 x 3 x 5
= 60

v. 24, 40, 80, 120

∴ LCM of 24, 40, 80 and 120 = 2 x 2 x 2 x 5 x 3 x 2
= 240

Question 4.
Find the smallest number which when divided by 8,9,10,15,20 gives a remainder of 5 every time.
Solution:
Here, the smallest number for division is LCM of 8, 9, 10,15 and 20.
8 = 2 x 2 x 2
9 = 3 x 3
10 = 2 x 5
15 = 3 x 5
20 = 2 x 2 x 5
LCM of given numbers = 2 x 2 x 2 x 3 x 3 x 5 = 360
∴ Required, smallest number = LCM + Remainder
= 360 + 5
= 365
∴ The required smallest number is 365.

Question 5.
Reduce the fractions \(\frac{348}{319}, \frac{221}{247}, \frac{437}{551}\) to the lowest terms.
Solution:
i.

ii.

iii.

Question 6.
The LCM and HCF of two numbers are 432 and 72 respectively. If one of the numbers is 216, what is the other?
Solution:
Here, LCM = 432, HCF = 72, First number = 216
First number x Second number = LCM x HCF
∴ 216 x Second number = 432 x 72
∴ Second number = \(\frac{432 \times 72}{216}=432 \times \frac{72}{216}=432 \times \frac{1}{3}=144\)
∴ The other number is 144.

Question 7.
The product of two two-digit numbers is 765 and their HCF is 3. What is their LCM?
Solution:
Here, HCF = 3, Product of the given numbers = 765
Now, HCF x LCM = Product of the given numbers
∴ 3 x LCM = 765
∴ LCM = \(\frac { 765 }{ 3 }\) = 255
∴ The LCM of the two two-digit numbers is 255.

Question 8.
A trader has three bundles of string 392 m, 308 m and 490 m long. What is the greatest length of string that the bundles can be cut up into without any left over string?
Solution:
The required greatest length of the string is the highest common factor (HCF) of 392, 308 and 490.
∴ 392 = 2 x 2 x 2 x 7 x 7
308 = 2 x 2 x 7 x 11
490 = 2 x 7 x 7 x 5
∴ HCF of 392, 308 and 490 = 2 x 7
= 14
∴ The required greatest length of the string is 14 m.

Question 9.
Which two consecutive even numbers have an LCM of 180?
Solution:
LCM of two consecutive even numbers = 180
But, HCF of two consecutive even numbers = 2
Now, product of the given number = HCF x LCM
= 2 x 180
= 360
To find the two consecutive even numbers, we have to factorize 360.
360 = 2 x 2 x 2 x 3 x 3 x 5
360 = (2 x 3 x 3) x (2 x 2 x 5)
= 18 x 20
∴ The two consecutive even numbers whose LCM is 180 are 18 and 20.

Std 7 Maths Digest

• Practice Set 8 Class 7 Answers
• Practice Set 9 Class 7 Answers
• Practice Set 10 Class 7 Answers
• Practice Set 11 Class 7 Answers
• Practice Set 12 Class 7 Answers
• Practice Set 13 Class 7 Answers
• Practice Set 14 Class 7 Answers

Balbharti Maharashtra State Board Class 7 Maths Solutions covers the 7th Std Maths Practice Set 13 Answers Solutions Chapter 3 HCF and LCM.

HCF and LCM Class 7 Maths Chapter 3 Practice Set 13 Solutions Maharashtra Board

Std 7 Maths Practice Set 13 Solutions Answers

Question 1.
Find the LCM:
i. 12, 15
ii. 6, 8, 10
iii. 18, 32
iv. 10, 15, 20
v. 45, 86
vi. 15, 30, 90
vii. 105, 195
viii. 12,15,45
ix. 63,81
x. 18, 36, 27
Solution:
i. 12, 15

∴ LCM of 12 and 15 = 3 x 2 x 2 x 5
= 60

ii. 6, 8, 10

∴ LCM of 6, 8 and 10 = 2 x 2 x 3 x 2 x 5
= 120

iii. 18, 32

∴ LCM of 18 and 32 = 2 x 2 x 2 x 2 x 3 x 3 x 2
= 288

iv. 10, 15, 20

∴ LCM of 10, 15 and 20 = 5 x 2 x 3 x 2
= 60

v. 45, 86

∴ LCM of 45 and 86 = 2 x 3 x 3 x 5 x 43
= 3870

vi. 15, 30, 90

∴ LCM of 15,30 and 90 = 3 x 5 x 2 x 3
= 90

vii. 105, 195

∴ LCM of 105 and 195 = 5 x 3 x 7 x 13
= 1365

viii. 12, 15, 45

∴ LCM of 12, 15 and 45 = 3 x 3 x 2 x 5 x 2
= 180

ix. 63, 81

∴ LCM of 63 and 81 = 3 x 3 x 3 x 7 x 3
= 567

x. 18, 36, 27

∴ LCM of 18, 36 and 27 = 3 x 3 x 2 x 2 x 3
= 108

Question 2.
Find the HCF and LCM of the numbers given below. Verify that their product is equal to the product of the given numbers:
i. 32, 37
ii. 46, 51
iii. 15, 60
iv. 18, 63
v. 78, 104
Solution:
i. 32 = 2 x 16
= 2 x 2 x 8
= 2 x 2 x 2 x 4
= 2 x 2 x 2 x 2 x 2 x 1
37 = 37 x 1
∴ HCF of 32 and 37 =1
LCM of 32 and 37 = 2 x 2 x 2 x 2 x 2 x 37
= 1184
HCF x LCM = 1 x 1184
= 1184
Product of the given numbers = 32 x 37
= 1184
∴ HCF x LCM = Product of the given numbers.

ii. 46 = 2 x 23 x 1
51 = 3 x 17 x 1
∴ HCF of 46 and 51 = 1
LCM of 46 and 51 = 2 x 23 x 3 x 17
= 2346
HCF x LCM = 1 x 2346
= 2346
Product of the given numbers = 46 x 51
= 2346
∴ HCF x LCM = Product of the given numbers.

iii. 15 = 3 x 5
60 = 2 x 30
= 2 x 2 x 15
= 2 x 2 x 3 x 5
∴ HCF of 15 and 60 = 3 x 5
= 15
LCM of 15 and 60 = 3 x 5 x 2 x 2
= 60
HCF x LCM = 15 x 60
= 900
Product of the given numbers = 15 x 60
= 900
∴ HCF x LCM = Product of the given numbers.

iv. 18 = 2 x 9
= 2 x 3 x 3
63 = 3 x 21
= 3 x 3 x 7
∴ HCF of 18 and 63 = 3 x 3
= 9
LCM of 18 and 63 = 3 x 3 x 2 x 7
= 126
HCF x LCM = 9 x 126
= 1134
Product of the given numbers = 18 x 63
= 1134
∴ HCF x LCM = Product of the given numbers.

v. 78 = 2 x 39
= 2 x 3 x 13
104 = 2 x 52
= 2 x 2 x 26
= 2 x 2 x 2 x 13
∴ HCF of 78 and 104 = 2 x 13
= 26
LCM of 78 and 104 = 2 x 13 x 3 x 2 x 2
= 312
HCF x LCM = 26 x 312
= 8112
Product of the given numbers = 78 x 104
= 8112
∴ HCF x LCM = Product of the given numbers.

Maharashtra Board Class 7 Maths Chapter 3 HCF and LCM Practice Set 13 Intext Questions and Activities

Question 1.
Write the tables of the given numbers and find their LCM. (Textbook pg. no. 19)
i. 6, 7
ii. 8, 12
iii. 5, 6, 15
Solution:
i. Multiples of 6 : 6, 12, 18, 24, 30, 36, 42
Multiples of 7 : 7, 14, 21, 28, 35, 42, 49
∴ LCM of 6 and 7 = 42

ii. Multiples of 8 : 8, 16, 24, 32, 40
Multiples of 12 : 12, 24, 36, 48
∴ LCM of 8 and 12 = 24

iii. Multiples of 5 : 5, 10, 15, 20, 25, 30, 35
Multiples of 6 : 6, 12, 18, 24, 30, 36
Multiples of 15 : 15, 30, 45, 60
∴ LCM of 5,6 and 15 = 30

Std 7 Maths Digest

• Practice Set 8 Class 7 Answers
• Practice Set 9 Class 7 Answers
• Practice Set 10 Class 7 Answers
• Practice Set 11 Class 7 Answers
• Practice Set 12 Class 7 Answers
• Practice Set 13 Class 7 Answers
• Practice Set 14 Class 7 Answers

Balbharti Maharashtra State Board Class 7 Maths Solutions covers the 7th Std Maths Practice Set 11 Answers Solutions Chapter 3 HCF and LCM.

HCF and LCM Class 7 Maths Chapter 3 Practice Set 11 Solutions Maharashtra Board

Std 7 Maths Practice Set 11 Solutions Answers

Question 1.
Factorize the following numbers into primes:
i. 32
ii. 57
iii. 23
iv. 150
v. 216
vi. 208
vii. 765
viii. 342
ix. 377
x. 559
Solution:
i. 32

∴ 32 = 2 × 2 × 2 × 2 × 2

ii. 57

∴ 57 = 3 × 19

iii. 23

∴ 23 = 23 × 1

iv. 150

∴ 150 = 2 × 3 × 5 × 5

v. 216

∴ 216 = 2 × 2 × 2 × 3 × 3 × 3

vi. 208

∴ 208 = 2 × 2 × 2 × 2 × 13

vii. 765

∴ 765 = 3 × 3 × 5 × 17

viii. 342

∴ 342 = 2 × 3 × 3 × 19

ix. 377

∴ 377 = 13 × 29

x. 559

∴ 559 = 13 × 43

Std 7 Maths Digest

• Practice Set 8 Class 7 Answers
• Practice Set 9 Class 7 Answers
• Practice Set 10 Class 7 Answers
• Practice Set 11 Class 7 Answers
• Practice Set 12 Class 7 Answers
• Practice Set 13 Class 7 Answers
• Practice Set 14 Class 7 Answers

Balbharti Maharashtra State Board Class 7 Maths Solutions covers the 7th Std Maths Practice Set 10 Answers Solutions Chapter 3 HCF and LCM.

HCF and LCM Class 7 Maths Chapter 3 Practice Set 10 Solutions Maharashtra Board

Std 7 Maths Practice Set 10 Solutions Answers

Question 1.
Which number is neither a prime number nor a composite number?
Solution:
1

Question 2.
Which of the following are pairs of co-primes?
i. 8,14
ii. 4,5
iii. 17,19
iv. 27,15
Solution:
i. Factors of 8: 1, 2, 4, 8
Factors of 14: 1, 2, 7, 14
∴ Common factors of 8 and 14: 1,2
∴ 8 and 14 are not a pair of co-prime numbers.

ii. Factors of 4: 1, 4
Factors of 5: 1, 5
∴ Common factors of 4 and 5: 1
∴ 4 and 5 are a pair of co-prime numbers.

iii. Factors of 17: 1, 17
Factors of 19: 1, 19
∴ Common factors of 17 and 19: 1
∴ 17 and 19 are a pair of co-prime numbers.

iv. Factors of 27: 1, 3, 9, 27
Factors of 15: 1, 3, 5, 15 .
∴ Common factors of 27 and 15 : 1,3
∴ 27 and 15 are not a pair of co-prime numbers.

Question 3.
List the prime numbers from 25 to 100 and say how many they are.
Solution:
29, 31, 37, 41, 43, 47, 53, 59, 61, 67, 71, 73, 79, 83, 89, 97.
There are 16 prime numbers from 25 to 100.

Question 4.
Write all the twin prime numbers from 51 to 100.
Solution:

1. 59 and 61
2. 71 and 73

Question 5.
Write 5 pairs of twin prime numbers from 1 to 50.
Solution:

1. 3,5
2. 5,7
3. 11,13
4. 17,19
5. 29,31
6. 41,43

Question 6.
Which are the even prime numbers?
Solution:
2

Maharashtra Board Class 7 Maths Chapter 3 HCF and LCM Practice Set 10 Intext Questions and Activities

Question 1.
Answer the following questions. (Textbook pg. no. 15)
i. Which is the smallest prime number?
ii. List the prime numbers from 1 to 50. How many are they?
iii. Identify the prime numbers in the list below.
17, 15 ,4, 3, 1, 2, 12, 23, 27, 35, 41, 43, 58, 51, 72, 79, 91, 97.
Solution:
i. 2 is the smallest prime number.
ii. There are 15 prime numbers from 1 to 50.
They are: 2, 3, 5, 7, 11, 13, 17, 19, 23, 29, 31, 37, 41, 43, 47.
iii. [17], 15 ,4, [3], 1, [2], 12, [23], 27, 35, [41], [43], 58, 51, 72, [79], 91, [97].

Std 7 Maths Digest

• Practice Set 8 Class 7 Answers
• Practice Set 9 Class 7 Answers
• Practice Set 10 Class 7 Answers
• Practice Set 11 Class 7 Answers
• Practice Set 12 Class 7 Answers
• Practice Set 13 Class 7 Answers
• Practice Set 14 Class 7 Answers

Balbharti Maharashtra State Board Class 7 Maths Solutions covers the 7th Std Maths Practice Set 9 Answers Solutions Chapter 2 Multiplication and Division of Integers.

Multiplication and Division of Integers Class 7 Maths Chapter 2 Practice Set 9 Solutions Maharashtra Board

Std 7 Maths Practice Set 9 Solutions Answers

Question 1.
Solve
i. (-96) ÷ 16
ii. 98 ÷ (-28)
iii. (-51) ÷ 68
iv. 38 ÷ (-57)
v. (-85) ÷ 20
vi. (-150) ÷ (-25)
vii. 100 ÷ 60
viii. 9 ÷ (-54)
ix. 78 ÷ 65
x. (-5) ÷ (-315)
Solution:
i. (-96) ÷ 16

ii. 98 ÷ (-28)

iii. (-51) ÷ 68

iv. 38 ÷ (-57)

v. (-85) ÷ 20

vi. (-150) ÷ (-25)

vii. 100 ÷ 60

viii. 9 ÷ (-54)

ix. 78 ÷ 65

x. (-5) ÷ (-315)

Question 2.
Write three divisions of integers such that the fractional form of each will be \(\frac { 24 }{ 5 }\).
Solution:

1. \(\frac{24}{5}=\frac{24 \times 1}{5 \times 1}=\frac{24}{5}=24 \div 5\)
2. \(\frac{24}{5}=\frac{24 \times 2}{5 \times 2}=\frac{48}{10}=48 \div 10\)
3. \(\frac{24}{5}=\frac{24 \times(-10)}{5 \times(-10)}=\frac{-240}{-50}=(-240) \div(-50)\)

Question 3.
Write three divisions of integers such that the fractional form of each will be \(\frac { -5 }{ 7 }\).
Solution:

1. \(\frac{-5}{7}=\frac{-5 \times 2}{7 \times 2}=\frac{-10}{14}=(-10) \div 14\)
2. \(\frac{-5}{7}=\frac{-5 \times(-5)}{7 \times(-5)}=\frac{25}{-35}=25 \div(-35)\)
3. \(\frac{-5}{7}=\frac{-5 \times 7}{7 \times 7}=\frac{-35}{49}=(-35) \div 49\)

Question 4.
The fish in the pond below, carry some numbers. (Choose any 4 pairs and carry out four multiplications with those numbers. Now, choose four other pairs and carry out divisions with these numbers.
Examples:
i. (-13) × (-15) = 195
ii. (-24) ÷ 9 = \(\frac{-24}{9}=\frac{-8}{3}\)

Solution:

1. (-13) × 9 = -117
2. 12 × 13 = 156
3. 9 × (-37) = -333
4. (-15) × (-8) = 120
5. \((-28) \div 12=\frac{-28}{12}=\frac{(-1) \times(28)}{12}=\frac{-7}{3}\)
6. \(12 \div 9=\frac{12}{9}=\frac{4}{3}\)
7. \(9 \div(-24)=\frac{9}{-24}=\frac{9}{(-1) \times 24}=\frac{-3}{8}\)
8. \((-18) \div(-27)=\frac{-18}{-27}=\frac{(-1) \times 18}{(-1) \times 27}=\frac{2}{3}\)

Note: Problems 2, 3 and 4 have many answers. Students may write answers other than the ones given.

Std 7 Maths Digest

• Practice Set 8 Class 7 Answers
• Practice Set 9 Class 7 Answers
• Practice Set 10 Class 7 Answers
• Practice Set 11 Class 7 Answers
• Practice Set 12 Class 7 Answers
• Practice Set 13 Class 7 Answers
• Practice Set 14 Class 7 Answers

Balbharti Maharashtra State Board Class 7 Maths Solutions covers the 7th Std Maths Practice Set 8 Answers Solutions Chapter 2 Multiplication and Division of Integers.

Multiplication and Division of Integers Class 7 Maths Chapter 2 Practice Set 8 Solutions Maharashtra Board

Std 7 Maths Practice Set 8 Solutions Answers

Question 1.
Multiply:

1. (-5) × (-7)
2. (-9) × (6)
3. (9) × (-4)
4. (8) × (-7)
5. (-124) × (-1)
6. (-12) × (-7)
7. (-63) × (-7)
8. (-7) × (15)

Solution:

1. 35
2. -54
3. -36
4. -56
5. 124
6. 84
7. 441
8. -105

Maharashtra Board Class 7 Maths Chapter 2 Multiplication and Division of Integers Practice Set 8 Intext Questions and Activities

Question 1.
In the previous class, we have learnt to add and subtract integers. Using those methods, fill in the blanks below. (Textbook pg. no. 11)

1. 5 + 7 = __
2. 10 + (-5) = __
3. -4 + 3 = __
4. (-7) + (-2) = __
5. (+8) – (+ 3) = __
6. (+8) – (-3) = __

Solution:

1. 12
2. 5
3. -1
4. -9
5. 5
6. 11

Question 2.
Write a number in each bracket to obtain the answer ‘3’ in each operation. (Textbook pg. no. 11)

Solution:

Question 3.
Multiply the given integers and complete the table given below. (Textbook pg. no. 12)

Solution:

Std 7 Maths Digest

• Practice Set 8 Class 7 Answers
• Practice Set 9 Class 7 Answers
• Practice Set 10 Class 7 Answers
• Practice Set 11 Class 7 Answers
• Practice Set 12 Class 7 Answers
• Practice Set 13 Class 7 Answers
• Practice Set 14 Class 7 Answers