Class 12

Balbharti Maharashtra State Board 12th Chemistry Important Questions Chapter 6 Chemical Kinetics Important Questions and Answers.

Maharashtra State Board 12th Chemistry Important Questions Chapter 6 Chemical Kinetics

Question 1.
What is chemical kinetics?
Answer:
Chemical kinetics is a branch of physical chemistry which involves the study of the rates and mechanisms of chemical reactions and the influence of various factors like temperature, pressure, catalyst, etc., on the rates of reactions.

Question 2.
What is the importance of chemical kinetics?
Answer:

• It deals with the study of the rates and mechanism of reactions.
• The effect of temperature on the reaction rates can be studied.
• The influence of catalysts can be studied.
• The conditions for altering the rates and mechanisms of chemical reactions can be predicted.
• Thermodynamic parameters like energy, enthalpy changes, Δ5, ΔG of the reactions can be calculated.

Question 3.
How are reactions classified according to their rates? Give one example of each.
Answer:
According to the rates of the reactions, they can be classified as :
(1) Fast reactions,
(2) Very slow reactions,
(3) Moderately slow reactions.

(1) Fitst actions : In this, reactants react almost instantaneously, e.g., neutralisation reaction between H+ and OH-, forming water.
\(\mathrm{H}_{(\mathrm{xa})}^{+}+\mathrm{OH}_{(\mathrm{aq})}^{-} \rightarrow \mathrm{H}_{2} \mathrm{O}_{0 \mathrm{D}}\)

(2) Very slow reactions : In this, the reactants react extremely slow, so that there is no appreciable change in the concentrations of the reactants over a long period of time. E.g., reaction of silica with mineral acids, rusting of iron, etc.

(3) Moderately slow reactions : In this, the reactants react moderately slow with a measurable velocity, e.g., the hydrolysis of the esters.
\(\begin{aligned}
\mathrm{CH}_{3} \mathrm{COOC}_{2} \mathrm{H}_{5}+\mathrm{H}_{2} \mathrm{O} \stackrel{\mathrm{H}^{+}}{\longrightarrow} \mathrm{CH}_{3} \mathrm{COOH} \\
&+\mathrm{C}_{2} \mathrm{H}_{5} \mathrm{OH}
\end{aligned}\)

Question 4.
Define rate of a reaction.
Answer:
Definition : The rate of a chemical reaction is defined as the change in the concentration of the reactants or products per unit time.

It is often expressed in mol dm^-3s^-1.

Question 5.
Explain the following :
(A) Rate of the reaction in terms of the concentration of the reactants.
(B) Rate of reaction in terms of the concentration of the products.
Answer:
(A) Rate of the reaction in terms of the concentration of the reactants :
If c₁ and c₂ are the concentrations of the reactant A at time t₁ and t₂ respectively, then, the change in concentration, Δc = c₂ – c₁
Since c₂ < c₁, the term Δc is negative often written as – Δc.
The time interval is, Δt – t₂ – t₁
If Δ [A] is the change in concentration of A, then A[A] = C₂ – C₁
∴ Rate of the reaction = \(\mathrm{A}=\frac{-\Delta[\mathrm{A}]}{\Delta t}\)
∴ Rate of the reaction = \(\frac{-\Delta c}{\Delta t}\)

(B) Rate of the reaction in terms of the concentration of the products :
If x₁ and x₂ are the concentrations of the product B at time t₁ and t₂ respectively, then the change in concentration, Δx = x₂ – x₁.

∴ x₂ > x₁, the term Δx is positive.
The time interval is, Δt = t₂ – t₁

If Δ B is the change in concentration of product B, then Δ[B] = x₂ – x₁ = Δx
∴ Rate of formation of \(\mathrm{B}=+\frac{\Delta[\mathrm{B}]}{\Delta t}\)
∴ Rate of the reaction \(=\frac{\Delta x}{\Delta t}\)

Question 6.
What are the units of rate of a chemical reaction?
Answer:

∴ The unit of the rate of a chemical reaction : mol L^-1 3t^-1 or mol dm^-3s^-1 (According to IUPAC, the rate of a chemical reaction should be expressed in mol m^-3s^-1 [SI unit]).

Question 7.
Mention the factors that affect the rate of a chemical reaction.
Answer:
The rate of a chemical reaction depends on the following factors :

• Nature of the reactants.
• The concentration of the reactants. In case of a gaseous reaction the rate depends on the pressures of the reactants.
• Temperature of the reaction.
• The presence of a catalyst and its nature.

Question 8.
Explain the term Average rate of a reaction.
Answer:
In chemical kinetics the rate of a reaction is measured in terms of the changes in the concentrations of the reactants or the products per unit time. Average rate of a chemical reaction : It is expressed as a finite change in concentration (- Δc) of the reactant divided by the time interval (Δt) for the change in concentration.

Consider a reaction,
A → B
The rate of a reaction, \(R=\frac{-\Delta[\mathrm{A}]}{\Delta t}=\frac{-\Delta c}{\Delta t}=\frac{c_{2}-c_{1}}{t_{2}-t_{1}}\)


∴ Average rate \(=\frac{-\Delta[\mathrm{A}]}{\Delta t}\) (in mol dm^-3s^-1)

Δc is negative, since the concentrartion of the reactant decreases with the time.

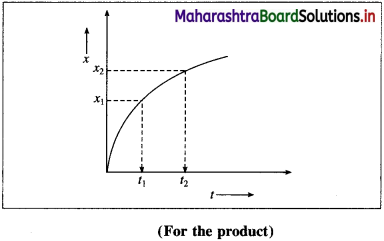
The rate of a reaction is also measured in terms of a finite change in the concentration (Δx) of the product divided by the time interval (Δt), for the change.

For the reaction,

Question 9.
Explain the term Instantaneous rate of a reaction.
Answer:
Instantaneous rate of a reaction : It is defined as a rate of a reaction at a specific instant during a course of the reaction.

If the average reaction rate is calculated over shorter and shorter intervals (making Δt very small) then instantaneous rate is obtained.

In case of reactant A, the instantaneous rate is represented as, \(R=\frac{-d[\mathrm{~A}]}{d t}\) and in case of product B, it is represented as \(R=\frac{+d[B]}{d t}\)

Question 10.
Define :
(a) Average rate of reaction.
(b) Instantaneous rate of reaction.
Answer:
(a) Average rate of a chemical reaction : It is expressed as a finite change in concentration (- Δc) of the reactant divided by the time interval (Δt) for the change in concentration.

∴ Average rate, \(R=\frac{-\Delta c}{\Delta t}\)

(b) Instantaneous rate of reaction : It is defined as a rate of a reaction at a specific instant during a course of the reaction.

Instantaneous rate \(=\frac{-d c}{d t}\)

Question 11.
Represent the average rates of the following reaction. N_2(g) + 3H_2(g) → 2NH_3(g).
Answer:
For the reation,

This is because the rate of consumption of H₂ is thrice the rate of consumption of N₂ while the rate of formation of NH₃ will be twice the rate of consumption of N₂.

Question 12.
Express the rate of a reaction in terms of change in concentration of each constituent in the following reaction : aA+bB → cC+ dD
Answer:
The rate of a reaction may be expressed in terms of decrease in the concentration of the reactants or in-crease in the concentration of the product per unit time,

∴ For the given reaction, aA T bB → cC +dD

Question 13.
For a hypothetical reaction, A + 2B → products, the concentration of A and B at different intervals of time are given in the following table. Find the rates of the reaction in terms of concentration changes in A and B.

The equilibrium concentration of A and B at different time intervals :

Time t/minute	[A]/mol L-1	[B]/ml L-1
0	1.000	2.000
10	0.534	1.068
20	0.342	0.360
30	0.180	0.360

Answer:
Rate of a reaction = \(\frac{-\Delta[\mathrm{A}]}{\Delta t}=-\frac{1}{2} \frac{\Delta[\mathrm{B}]}{\Delta t}\)
(1) Over time interval from O to 10 minutes

(Note that the rate of a reaction in terms of changes in concentration of any reactant or product at the given time remains the same.)

(2) Over the time interval from 10 to 20 minutes,

Question 14.
Show that the rate of reaction is the same whether expressed in terms of the rate of consumption of any reactant or of the formation of any product.
2N₂O_5(g) → 4NO_2(g) + O_2(g)
The concentrations of reactants and products at different time intervals are given in the following table :
Concentrations of various species at different times for the reaction N₂O_5(g) → 4NO_2(g) + O_2(g) :

Time/s	[N2O5]/M	[NO2]/M	[O2]/M
0	0.0300	0	0
200	0.0213	0.0174	0.00435
400	0.0152	0.0296	0.00740
600	0.0108	0.0384	0.00960

Answer:
The rate of the reaction can be expressed in terms of rate of consumption of reactants or rate of formation of products.

Consider concentrations at time t₁ = 200 seconds and t₂ = 400 seconds

The constant values of rate of reaction proves that the rate of the reaction may be measured in terms of concentration changes of reactants or products per unit time.

Question 15.
Define Rate law (or differential rate law).
Answer:
Rate law (or differential rate law) : It is defined as an experimentally determined mathematical equation which expresses the rate of a chemical reaction in terms of molar concentrations of the reactants which influence the rate of the reaction. For example, for a reaction, A + B → Products By rate law, Rate = R = k[A] x [B] where k is a rate constant and [Al and [B] are molar concentrations of the reactants A and B respectively.

Question 16.
Give examples of rate law with illustrations.
Answer:
Consider following examples :
(i) H_2(g) + I_2(g) → 2HI_(g)
R = k[H₂] [I₂]

(ii) 2H₂O_2(g) → 2H₂O_(I) + O_2(g)
Experimentally it is observed that the rate of the reaction is proportional to the concentration of H₂O₂.
∴ R = k [H₂O₂]

(iii) NO_2(g) + CO_(g) → NO_(g) + CO_2(g)
Experimentally it is observed that rate of the reaction does not depend on the concentration of CO but it is proportional to [NO₂]².
∴ R = k[NO₂]²

Question 17.
What are the applications of the rate law?
Answer:

• The rate of any reaction at the given concentration can be measured by knowing the rate law and the rate constant.
• The concentration of the reactants or the products at any instant during the progress of a reaction can be estimated with the help of rate law and the rate constant.
• The mechanisms of simple or complex chemical reactions can be predicted and studied.

Question 18.
Define the rate constant. What are the factors which influence the rate constant of a chemical reaction?
Answer:
(A) Rate constant : The rate constant of a chemical reaction is defined as the rate of the chemical reaction when the concentration (or active masses) of each reactant has unit value, i.e., 1 mol dm^-3 in the case of solution and the pressure is 1 atm in case of gases, e.g., for a reaction, A → products, Rate R = k[A].

If [A] = 1 mol dm^-3, then k = R.

(B) The rate constant of a reaction depends on the following factors:

• Nature of the reactants.
• Temperature of the reaction. As the temperature increases, the velocity constant (rate constant) increases.
• The conditions of the reactions like the presence of the catalyst, solvent, pH, etc.
• It does not depend on the concentration of the reactants. But if one or more substances are in excess concentration, then the order of the reaction is independent of them.

Question 19.
What are the characteristics of rate constant?
Answer:
The characteristics of rate constant are as follows :

• The rate constant depends upon the nature of the reaction.
• Higher the value of the rate constant, faster is the reaction.
• Lower the value of the rate constant, slower is the reaction.
• By increasing the temperature, the magnitude of the rate constant increases.
• For the given reaction, the rate constant has higher value in the presence of a catalyst than in the absence of the catalyst.
• The reactions having lower activation energy have higher values for rate constants.

Solved Examples 6.2 – 6.3.2

Question 20.
Solve the following :

(1) Write the rate expressions for the following reactions in terms of rate of consumption of the reactants and the rate of formation of the products.
(i) 2NO_(g) + O_2(g) → 2NO_2(g)
(ii) H_2(g) + I_2(g) → 2HI_(g)
Solution :
(i) Given : 2NO_(g) + O_2(g) → 2NO_2(g)
Rate of consumption of NO at time \(t=\frac{-d[\mathrm{NO}]}{d t}\)
Rate of consumption of O₂ at time \(t=\frac{-d\left[\mathrm{O}_{2}\right]}{d t}\)
Rate of formation of NO₂ at time \(t=\frac{d\left[\mathrm{NO}_{2}\right]}{d t}\)
Rate of the reaction \(=-\frac{1}{2} \frac{d[\mathrm{NO}]}{d t}=\frac{-d\left[\mathrm{O}_{2}\right]}{d t}\)
\(=\frac{1}{2} \frac{d\left[\mathrm{NO}_{2}\right]}{d t}\)

(ii) Given : H_2(g) + I_2(g) → 2HI_(g)
Rate of consumption of H₂ at time \(t=\frac{-d\left[\mathrm{H}_{2}\right]}{d t}\)
Rate of consumption of I₂ at time \(t=\frac{-d\left[\mathrm{I}_{2}\right]}{d t}\)
Rate of formation of HI at time \(t=\frac{d[\mathrm{HI}]}{d t}\)
∴ Rate of reaction at any time t \(=-\frac{d\left[\mathrm{H}_{2}\right]}{d t}=-\frac{d\left[\mathrm{I}_{2}\right]}{d t}=\frac{1}{2} \frac{d[\mathrm{HI}]}{d t}\)

(2) The gas-phase reaction between NO and Br₂ is represented by the equation. 2NO_(g) + Br_2(g) → 2NOBr_(g)
(a) Write the expressions for the rate of consumption of reactants and formation of products.
(b) Write the expression for the rate of overall reaction in terms of rates of consumption of reactants and formation of products.
Solution :
Given : 2NO_(g) + Br_2(g) → 2NOBr_(g)
(a) Rate of consumption of NO at time t \(=-\frac{d[\mathrm{NO}]}{d t}\)
Rate of consumption of Br₂ at time t \(=\frac{-d\left[\mathrm{Br}_{2}\right]}{d t}\)
Rate of formation of NOBr at time \(t=\frac{d[\mathrm{NOBr}]}{d t}\)
(b) Rate of reaction \(=-\frac{1}{2} \frac{d[\mathrm{NO}]}{d t}=\frac{-d\left[\mathrm{Br}_{2}\right]}{d t}\)
\(=\frac{1}{2} \frac{d[\mathrm{NOBr}]}{d t}\)

(3) The decomposition of N2Os is represented by the equation
2N₂O_5(g) → 4NO_2(g) + O_2(g)
(a) How is the rate of formation of NO₂ related to the rate of formation of O₂?
(b) How is the rate of formation of O₂ related to the rate of consumption of N₂O₅?
Solution :
Given : 2N₂O_5(g) → 4NO_2(g) + O_2(g)
(a) Rate of formation of NO₂ at time \(t=\frac{d\left[\mathrm{NO}_{2}\right]}{d t}\)
Rate of formation of O₂ at time \(t=\frac{d\left[\mathrm{O}_{2}\right]}{d t}\)

They are related to each other through rate of reaction.
∴ Rate of reaction \(=\frac{1}{4} \frac{d\left[\mathrm{NO}_{2}\right]}{d t}=\frac{d\left[\mathrm{O}_{2}\right]}{d t}\)

(b) Rate of consumption of N₂O₅ at time t \(=-\frac{d\left[\mathrm{~N}_{2} \mathrm{O}_{5}\right]}{d t}\)

Rate of reaction \(=-\frac{1}{2} \frac{d\left[\mathrm{~N}_{2} \mathrm{O}_{5}\right]}{d t}=\frac{d\left[\mathrm{O}_{2}\right]}{d t}\)

In general,
Rate of reaction \(=-\frac{1}{2} \frac{d\left[\mathrm{~N}_{2} \mathrm{O}_{5}\right]}{d t}=\frac{1}{4} \frac{d\left[\mathrm{NO}_{2}\right]}{d t}=\frac{d\left[\mathrm{O}_{2}\right]}{d t}\)

(4) Nitric oxide reacts with H₂ according to the reaction. 2NO_(g) + 2H_2(g) → N_2(g) + 2H₂O_(g)
What is the relationship among \(\frac{d[\mathrm{NO}]}{d t}=\frac{d\left[\mathrm{H}_{2}\right]}{d t}=\frac{d\left[\mathrm{~N}_{2}\right]}{d t} \text { and } \frac{d\left[\mathrm{H}_{2} \mathrm{O}\right]}{d t} ?\)
Solution :
Given : 2NO_(g) + 2H_2(g) → N_2(g) + 2H₂O_(g)
The relationship among the rate of consumption of the reactants and the rate of formation of products is as follows :

Rate of reaction :
\(R=-\frac{1}{2} \frac{d[\mathrm{NO}]}{d t}=-\frac{1}{2} \frac{d\left[\mathrm{H}_{2}\right]}{d t}=\frac{d\left[\mathrm{~N}_{2}\right]}{d t}=\frac{1}{2} \frac{d\left[\mathrm{H}_{2} \mathrm{O}\right]}{d t}\)

(5) The rate of decomposition of N2Os was studied in liquid bromine,
2N₂O_5(g) → 4NO_2(g) + O_2(g)
If at a certain time, the rate of disappearance of N₂O₅ is 0.015 Ms^-1 find the rates of formation of NO₂ and O₂. What is the rate of the reaction at this instant?
Solution :
Given : 2N₂O_5(g) → 4NO_2(g) + O_2(g)
Rate of disappearance of N₂O₅ = 0.015 M s^-1
Rate of formation of NO₂ =?
Rate of formation of O₂ =?
Rate of reaction = ?
Rate of disappearance of \(\mathrm{N}_{2} \mathrm{O}_{5}=\frac{-d\left[\mathrm{~N}_{2} \mathrm{O}_{5}\right]}{d t}\)
= 0.015 M s^-1

Since 4 moles of NO₂ are formed from 2 moles of N₂O₅ Rate of formation of NO₂
Answer:
Rate of formation of NO₂ = 0.03 Ms^-1
Rate of formation of O₂ = 0.0075 M s^-1
Rate of reaction = 0.0075 Ms^-1.

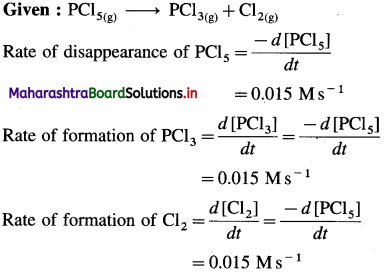
(6) In the reaction, PCl_5(g) → PCl_3(g) + CI_2(g), at a particular moment, the rate of disappearance of PCl₅ is 0.015 Ms^-1. What are the rates of formation of PCI₃ and Cl₂?
Solution :
Given : PCl_5(g) → PCl_3(g) + Cl_2(g)


Answer:
Rate of formation of PCl₃ = 0.015 Ms^-1
Rate of formation of Cl₂ = 0.015 Ms^-1

(7) In the reaction, 2N₃O_5(g) → 4NO_2(g) + O_2(g), at a certain time, the rate of formation of NO₂ is 0. 04 Ms^-1. Find the rate of consumption of N₂O₅, rate of formation of O₂ and the rate of the reaction.
Solution :
Given : 2N₂O_5(g) → 4NO_2(g) + O_2(g)
Rate of formation of NO₂ = \(\frac{d\left[\mathrm{NO}_{2}\right]}{d t}\) = 0.04 Ms^-1

From the reaction, rate of consumption of N₂O₅ is half the rate of formation of NO₂ since when 2 moles of N₂O₅ are consumed, 4 moles of NO₂ are formed.

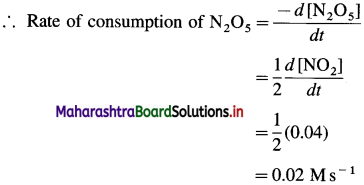
Rate of formation of O₂ is one-fourth rate of formation of NO₂.

Answer:
(i) Rate of consumption of N₂O₅
(ii) Rate of formation of O₂ = 0.01 Ms^-1
(iii) Rate of reaction = 0.01 Ms^-1

(8) Consider the reaction 2A + B → 2C. Suppose that at a particular moment during the reaction, rate of disappearance of A is 0.076 M/s,
(a) What is the rate of formation of C?
(b) What is the rate of consumption of B?
(c) What is the rate of the reaction?
Solution :
Given : 2A + B → 2C
Rate of disappearance of A = 0.076 Ms^-1
(a) Rate of formation of C =?
(b) Rate of consumption of B =?
(c) Rate of reaction = ?

Answer:
(a) Rate of formation of C = 0.076 Ms^-1
(b) Rate of consumption of B = 0.038 M s^-1
(c) Rate of reaction = 0.038 Ms^-1

(9) Consider the reation \(\mathbf{3 I}_{(\mathbf{a q})}^{-}+\mathbf{S}_{2} \mathbf{O}_{8(u q)}^{2-} \longrightarrow \mathbf{I}_{3(\mathrm{aq})}^{-}+2 \mathrm{SO}_{4}^{2-}\) At a particular time t, \(t, \frac{d\left[\mathrm{SO}_{4}^{2-}\right]}{d t}=2.2 \times 10^{-2} \mathrm{M} / \mathrm{s}\) What are the values of \(\text { (a) }-\frac{d\left[\mathrm{I}^{-}\right]}{d t}\) \(-\frac{d\left[\mathrm{~S}_{2} \mathrm{O}_{8}^{2-}\right]}{d t}\) \(\text { (c) } \frac{d\left[\mathbf{I}_{3}^{-}\right]}{d t}\) at the same time?
Solution :

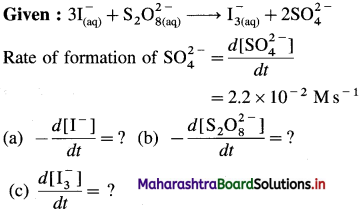
(a) Rate of consumption of \(\mathrm{I}^{-}=-\frac{d\left[\mathrm{I}^{-}\right]}{d t}\)
When 2 moIes of \(\mathrm{SO}_{4}^{2-}\) are formed, 3 moves of I^– are consumed in the same time.

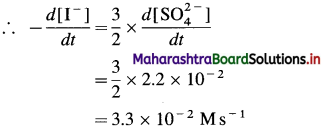
(b) In the formation of 2 moles of \(\mathrm{SO}_{4}^{2-}\), 1 mole of \(\mathrm{S}_{2} \mathrm{O}_{8}^{2-}\) is consumed in the same time.

Answer:

(10) Ammonia and oxygen react at high temperature as :
4NH_3(g) + 5O_2(g) → 4NO_(g) + 6H₂O_(g)
In an experiment, rate of formation of NO_(g) is 3.6 x 10^-3 mol L^-1s^-1.
Calculate-
(a) Rate of disappearance of ammonia
(b) Rate of formation of water.
Solution :

Answer:
(a) Rate of disappearance of NH₃
= 3.6 x 10^-3 mol L^-1s^-1
(b) Rate of formation of H₂O
= 5.4 x 10^-3 mol L^-1s^-1

(11) The rate law for the reaction
C₂H₄Br₂ + 3I^– → C₂H₄ + 2Br^– +I^–₃ is Rate = k [C₂H₄Br₂][I^–]. The rate of the reac-tion is found to be 1.1 x 10^-4 M/s when the concentrations of C₂H₄Br₂ and I^–– are 0.12M and 0.18 M respectively. Calculate the rate constant of the reaction.
Solution :
Given : C₂H₄Br₂ + 3I^– → C₂H₄ + 2Br^– +I^–₃
By rate law, Rate of reaction = R = k x [C₂H₄Br₂][I^–]
R = 1.1 x 10^-4 Ms^-1
[C₂H₄Br₂] = 0.12 M; [I^–] =0.18 M
Rate constant = k =?
R = k x [C₂H₄Br₂] x [I^–]

Answer:
Rate constant = k = 5.1 x 10^-3 M^-1s^-1

(12) For a reaction, 2A + B → C, the rate law is, rate =k x [A]² x [B]. If the rate constant of the reaction is 3.74 x 10^-2M^-2s^-1, calculate the rate of the reaction when the concentrations of A, B and C are 0.108 M, 0.132 M and 0.124 M respectively.
Solution :
Given : Rate constant of the reaction = k
= 3.74 x 10^-2M^-2s^-1
[A] =0.108 M, [B] = 0.132M, [C] = 0.124 M
Rate of the reaction = R = ?
By rate law,
R = k [A]² x [B] = (0.108)² x 0.132 = 1.54 x 10^-3 Ms^-1
(Concentration of C need not be considered since it is a product.)
Answer:
Rate of reaction = 1.54 x10^-3 Ms^-1

(13) For a reaction, A + B → C, if the concentration of A doubles, the rate of the reaction doubles. While if the concentration of B doubles the rate of the reaction increases by four fold. Write rate law. .
Solution :
Let x moles of A react with y moles of B. xA + yB → C
To write rate law, it is necessary to find x and y values.

(i) Initial rate \(=R_{1}=k[\mathrm{~A}]_{1}^{x}[\mathrm{~B}]_{1}^{y}\)
Final rate R₂ is doubled when the concentration of A is doubled, i.e., R₂ = 2R₁ when final concentration,

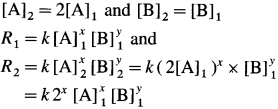
(It is assumed that the concentration of B remains same.)

(ii) Initial rate \(=R_{1}=k[\mathrm{~A}]_{1}^{x}[\mathrm{~B}]^{y}\)
If the concentration of B is doubled keeping of A constant, rate becomes four times, i.e.,

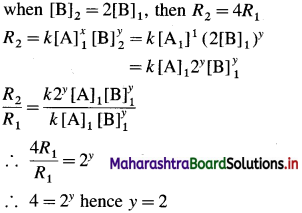
Hence the rate law is represented by an expression.
Rate = k[A] [B]²
Answer:
Rate law is. Rate = k [A] [B]²

(14) For the reaction, A2 + B + C → AC + AB, it is found that tripling the concentration of A₂ triples the rate, doubling the concentration of C doubles the rate and doubling the concentration of B has no effect,
(a) What is the rate law?
(b) Why the change in concentration of B has no effect?
Solution :
Given : A2 + B + C → AC + AB
(a) The rate law may be represented as,
Rate = k [A₂]^x [B]^y [C]^z
Let [A]₁, [B]₁ and [C]₁ represent initial concentration and [A]₂, [B]₂ and [C]₂ represent final concentrations, and let R₁ and R₂ be initial and final rates of the reaction when the concentrations are changed.

(i) If [A]₂ = 3[A]₁, R₂ = 3R₁

If the concentrations of B and C remain constant, then

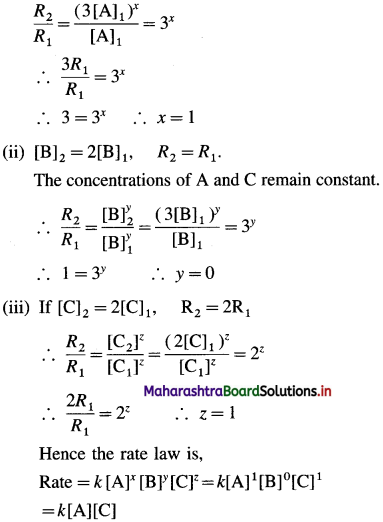
(b) In the rate determining step, B may not be involved as the reactant, hence rate is independent of changes in concentration of B. (OR B may be in large excess as compared to the concentrations of A and C.)
Answer:
(a) Rate law : Rate = k [A] [C]

Question 21.
Define and explain the term order of a chemical reaction.
Answer:
Order of a chemical reaction : The order of a chemical reaction is defined as the number of molecules (or atoms) whose concentrations influence the rate of the chemical reaction.
OR
The order of a chemical reaction is defined as the sum of the powers (or exponents) to which the concentration terms of the reactants are raised in the rate law expression for the given reaction.

Explanation :
Consider a reaction,
n₁A + n₂B → Products
where n₁ moles of A react with n₂ moles of B.

The rate of this reaction can be expressed by the rate law equation as,
R = k [A]^n₁ [B]^n₂
where k is the rate constant of the reaction, hence, the order of the reaction is n – n₁ + n₂, (observed, experimentally).

If n = 1, the reaction is called the first order reaction, if n = 2, it is called the second order reaction, etc.

If n = 0, it is called the zero order reaction, e.g., photochemical reaction of H_2(g) and Cl_2(g).

Question 22.
What are the features (or key points) of order of a reaction?
Answer:
The features of order of reaction are as follows :

• It represents the number of atoms, ions or molecules whose concentrations influence the rate of the reaction.
• It is not related to the stoichiometric equation of the reaction, hence it cannot be predicted from stoichiometric balanced equation.
• It is experimentally determined quantity.
• It is defined only in terms of the concentrations of the reactants and not of products.
• It may have values which are integers, fractional or zero.
• Higher values are rare. Reactions of first and second order are in large number. Third order reactions are very few like,
  2NO_(g) + Cl_2(g) → 2NOCl_(g).

Solved Examples 6.3.3

Question 23.
Solve the following :
(1) From the rate expressions for the following reactions, determine their order :
(a) 2N₂O_5(g) → 4NO_2(g) + O_2(g) : Rate = k [N₂O₅]
(b) CHCl_3(g) + Cl_2(g) → CCl_4(g) + HCl_(g) : Rate = k [CHL₃] [Cl₂]^1/2
(c) C₂H₅Cl_(g) → C₂H_4(g) + HCl_(g): Rate = k [C₂H₅Cl]
(d) 2NO_2(g) + F_2(g) → 2NO₂F_(g) → : Rate = k (NO₂] [F₂]
Solution :
(a) 2N₂O_5(g) → 4NO_2(g) + O_2(g)
The rate law expression given for the reaction is Rate = k x [N₂O₅]
Hence the reaction is of first order.

(b) CHCl_3(g) + Cl_2(g) → CCl_4(g) + HCl_(g)
The given rate law expression is, R = k [CHCl₃] x [Cl₂]^1/2 Here the order of a reaction is one with respect to CHCl_3(g) and half with respect to Cl_2(g). Therefore the overall order of the reaction is 1 + 1/2 = 1.5.

(c) C₂H₅Cl_(g) → C₂H_4(g) + HCl_(g)
The given rate law expression is, Rate = k [C₂H₅Cl]
Hence the reaction has order equal to one.

(d) 2NO_2(g) + F_2(g) → 2NO₂F_(g)
The given rate law expression for the reaction is Rate = k [NO₂] x [F₂]
Hence the reaction is first order with respect to NO2 and first order with respect to F₂. The overall order of the reaction is, n = n_NO2 + nF₁ = 1 + 1 = 2.

(2) Determine the order of following reactions from their rate expressions :
(a) 2H₂O₂ → 2H₂O + O₂ Rate = k [H₂O₂]
(b) NO₂ + CO → NO + CO₂ Rate = k [NO₂]²
(c) 2NO + O₂ → 2NO₂ Rate = k [NO]² x [O₂]
(d) CHCl_3(g) + Cl_2(g) → CCl_4(g) + HCl_(g)
Rate = k [CHCl₃] [Cl₂]
Solution :
(a) For the reaction,
2H₂O₂ → 2H₂O + O₂
Since the rate law expression given is,
Rate = k [H₂O₂]
Hence the reaction is of first order.

(b) For the reaction,
NO₂ + CO → NO + CO₂
Since the rate law given is Rate = k [NO₂]², the reaction is second order with respect to NO₂ and zero order with respect to CO. Hence the net order of the reaction is, n = nNO₂ + nco = 2 + 0 = 2

(c) For the reaction,
2NO + O₂ → 2NO₂
Since the rate law expression given is, Rate = k [NO]² x [O₂] the reaction is second order with respect to NO and first order with respect to O₂. Hence the overall order of reaction is n = nNO₂ + no₂ = 2 + 1 = 3.

(d) For the reaction, by rate law,
Rate = k [CHCl₃] x [Cl₂] reaction is first order with respect to CHCl₃ and first order with respect to Cl₂. Hence the overall order is, n = ncHcl₃ + ncl₂ = 1 + 1 = 2.

(3) Write the rate law expressions for the following reactions:
(1) 2N₂O_5(g) → 4NO₂ + O₂; order of the reaction is 1.
(2) CH₃CHO → CH₄ + CO; order of the reaction Is ³/₂.
Solution :
(1) For the given reaction, order is one hence the rate law expression is, Rate = k [N₂O₅].
(2) For the given reaction, order is ³/₂, hence the rate law expression is Rate = k x [CH₂CHO]^3/₂.

(4) The reaction \(\mathbf{H}_{2} \mathbf{O}_{2(\mathbf{a q})}+3 \mathbf{I}_{(\mathbf{a q})}^{-}+2 \mathbf{H}_{(\mathrm{aq})}^{+} \longrightarrow 2 \mathbf{H}_{2} \mathbf{O}_{(0)}+\mathbf{I}_{3(a q)}^{-}\) is first order in H₂O₂ and I^–, zero order in H⁺. Write the rate law.
Solution:
Given :
\(\mathrm{H}_{2} \mathrm{O}_{2(\mathrm{~g})}+3 \mathrm{I}_{(\mathrm{aq})}^{-}+2 \mathrm{H}^{+}{ }_{(\mathrm{aq})} \longrightarrow 2 \mathrm{H}_{2} \mathrm{O}_{(\mathrm{i})}+\mathrm{I}_{3(\mathrm{aq})}^{-}\)
Since the reaction is first order in H₂O₂ and F and zero order in H⁺, the expression for rate law will be,
Rate =k [H₂O₂]¹ [I^–]¹ [H⁺]⁰
∴ Rate = k [H₂O₂] [I^–]
Answer:
Rate = k [H₂O₂] [I^–]

(5) The rate law for the gas-phase reaction
2NO_(g) + O_2(g) → 2NO_2(g) is rate = k [NO₂]² [O₂]. What is the order of the reaction with respect to each of the reactants and what is the overall order of the reaction?
Solution :
Given : 2NO_(g) + O_2(g) → 2NO_2(g)
Rate = k [NO]²[O₂]
Order of the reaction with respect to NO = n_No = 2
Order with respect to O₂ = n_O2 = 1
Overall order of the reaction = n = n_NO + n_O2
= 2 + 1
= 3
Answer:
Order with respect to NO = 2
Order with respect to O₂ = 1
Overall order = 3

(6) What is the order for the following reactions?
(a) 2NO_2(g) + F_2(g) → 2NO₂F_(g), rate = k [NO₂][F₂]
(b) CHCl_3(g) + Cl_2(g) → CCl_4(g) + HCl_(g), rate = k[CHCl₃][Cl₂]^1/₂
Solution :
(a) Given : 2NO_2(g) + F_2(g) → 2NO₂F
Rate = k [NO₂][F₂]
Hence the reaction is first order with respect to NO₂ and first order with respect to F₂
∴ Order of reaction = n_NO2 + n_F2 = 1 + 1 = 2

(b) Given :
CHCl_3(g) + Cl_2(g) → CCl_4(g) + HCl_(g),
Rate = k [ CHCl₃] [Cl₂]^1/₂
Hence the reaction is first order in CHCl₃ and half order in Cl₂.
∴ Order of reaction
= n_CHCl3 + n_Cl2 = 1 + \(\frac{1}{2}\) = \(\frac{3}{2}\)
Answer:
(a) Order of the reaction = 2
(b) The order of the reaction = \(\frac{3}{2}\)

(7) Write the rate law for the following reactions :
(a) A reaction that is zero order in A and second order in B.
(b) A reaction that is second order in NO and first order in Br₂.
Solution :
(a) Given : A + B → Products
The reaction is zero order in A and second order in B. Hence the rate law is represented as, Rate = k [A]^O[B]²
Rate = k[B]²

(b) Given : 2NO_(g) + Br_2(g) → 2NOBr_(g)
The reaction is second order in NO and first in Br₂. Hence the rate law is,
∴ Rate = k [NO]²[Br₂]
Answer: (a) Rate law : Rate = k[B]²
(b) Rate law : Rate = k [NO]²[Br₂]

(8) The reaction A + B → Products, is first order in each of the reactants, (a) Write the rate law.
(b) How does the reaction rate change if the concentration of B is decreased by a factor 3?
(c) What is the change in the rate if the concentration of each reactant is tripled? (d) What is the change in the rate, if the concentration of A is doubled and that of B is halved?
Solution :
(a) The reaction is first order in A and B. Hence the equation for rate law is,
Rate = k [A] [B]
(b) Before changing the concentration of B, Initial rate = R₁ – k [A]₁ [B]₁
After change in concentration of B,

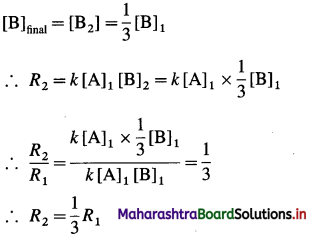
Hence the rate of the reaction will be decreased by a factor 3.

(c) When the concentration of each reactant is tripled, then the final concentrations will be, [A]₂ = 3[A]₁ and [B]₂ = 3[B₁]
∴ R₂ = k x 3[A]₁ x 3 [B]₁
∴ R₂ = k x 3[A]₁ x 3 [B]₁

Hence the rate of the reaction will be increased by 9 times.

(d) When the concentration A is doubled and that of B is halved then the final concentrations will be,

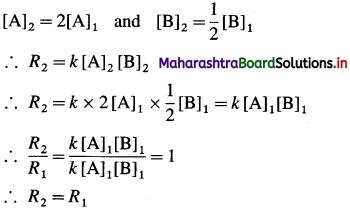
Rate of the reaction will remain unchanged.
Answer:
(a) Rate law is, Rate = k [A] [B],
(b) Rate is decreased by a factor 3,
(c) Rate is increased by 9 times,
(d) Rate remains unchanged.

(9) Consider the reaction A₂ + B → products. If the concentration of A₂ and B are halved, the rate of the reaction decreases by a factor of 8. If the concentration of A₂ is increased by a factor of 2.5, the rate increases by the factor of 2.5. What is the order of the reaction? Write the rate law.
Solution :
Given : A₂ + B → Products
(i) When concentration of A₂ and B are halved :
[A₂]_2(final) = 1/2 [A₂]_1(final) and [B]₂ = 1/2 [B]₁ then, R_2(final) = 1/8R_1(intial).

(ii) When concentration of A₂ is increased by the factor 2.5,
[A₂]₂ = 2.5 [A₂]₁ (concentration of B is same) then, R₂ = 2.5 R₁
Now let the reaction be, XA₂ + yB → Products

From data in (ii),

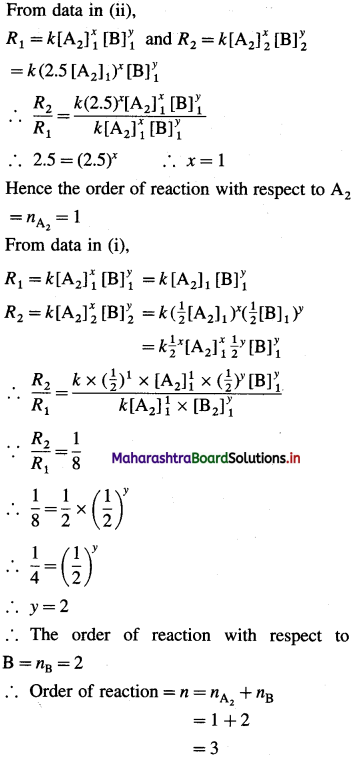
Hence the reaction is of third order. The rate law can be represented as,
Rate = k [A₂] [B]²
Answer:
(i) Order of the reaction = 3
(ii) Rate law : Rate = k [A₂] [B]³

(10) Consider the reaction C + D → Products. The rate of the reaction increases by a factor of 4 when the concentration of C is doubled. The rate of the reaction is tripled when concentration of D is tripled. What is the order of the reaction? Write the rate law.
Solution :
Given : C + D → Products OR xC + yD → Products
(i) When the concentration of C is doubled, the rate of the reaction increases by 4.

[C]_2(final) = 2[C]_1(initial) then R_2(final) = 4R_1(initial)
(In this, the concentration of D is assumed to be constant.)

Hence, the reaction is second order in C.
∴ n_C = 2
(ii) When the concentration of D is tripled, rate is tripled. The concentration of C is assumed to be constant.

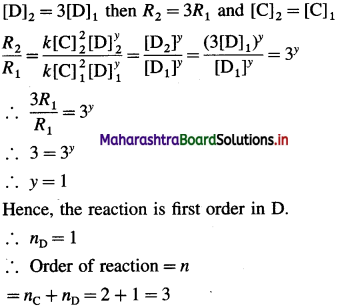
Rate law : Rate = A[C]²[D]
Answer:
(i) Order of the reaction = 3
(ii) Rate law : Rate = A[C]²[D]

(11) The reaction F_2(g) + 2ClO_2(g) → 2FClO_2(g) is first order in each of the reactants. The rate of the reaction is 4.88 x 10^-4 M/s when [F₂] = 0.015 M and [ClO₂]= 0.025 M. Calculate the rate constant of the reaction.
Solution :
Given :
F_2(g) + 2ClO_2(g) → 2FClO_2(g)
Order of reaction in F₂ = nF₂ = 1
Order of reaction in CIO₂ = n_ClO2 = 1
Rate = R = 4.88 x 10^-4 Ms^-1
[F₂] = 0.015 M; [ClO₂] = 0.025 M
Rate = k = ?
By rate law,

Answer:
Rate constant = 1 = 1.3 M^-2s^-1

(12) The reaction 2H_2(g) + 2NO_(g) → 2H₂O_(g) + N_2(g) is first order in H₂ and second order in NO. The rate constant of the reaction at a certain tem­perature is 0.42M^-2s^-1. Calculate the rate when [H₂] = 0.015 M and [NO] = 0.025 M.
Solution :
Given : 2H_2(g) + 2NO_(g) → 2H₂O_(g) + N_2(g)
Order of reaction in H₂ = n_H1 = 1
Order of reaction in NO = n_NO = 2
Rate constant = k = 0.42 M^-2s^-1
[H₂] = 0.015 M; [NO] = 0.025 M
Rate of reaction = R = ?
By rate law,
Rate = R = k [H₂] [NO]²
= 0.42 x 0.015 x (0.025)² M^-2s^-1 M M
= 3.94 x 10^-6 Ms^-1
Answer:
Rate of reaction = R = 3.94 x 10^-6 Ms^-1

(13) Find the order of following reactions whose rate laws are expressed as follows. C_A and C_B are the concentrations of reactants A and B respectively :

Solution :
Given :
(1) For, – \(\frac{d c}{d t}\) = k x \(\mathrm{C}_{A}^{0}\) the order of the reaction, n = 0. Hence it is a zero order reaction.

(2) For, – \(\frac{d c}{d t}\) = k x \(\mathrm{C}_{A}^{3 / 2}\), the overall order of the reaction is 3/2.

(3) For, –\(\frac{d c}{d t}\) = k x \(\mathrm{C}_{A}^{1 / 2} \mathrm{C}_{B}^{2}\), the reaction has order 1/2 with respect to A and 2 with respect to B.
∴ n = n_A + n_B = \(\frac{1}{2}\) + 2 = \(\frac{5}{2}\).
Hence the (overall) order of the reaction is \(\frac{5}{2}\).

(4) For, \(-\frac{d c}{d t}=k \mathrm{C}_{A}^{5 / 2} \times \mathrm{C}_{B}^{0}\)
The reaction has order \(\frac{5}{2}\) with respect to A and zero with respect to B.
∴ n = n_A + n_B = \(\frac{5}{2}\) + 0 = \(\frac{5}{2}\)
Hence the order of the reaction is \(\frac{5}{2}\).

(5) For, \(-\frac{d c}{d t}=k \times \mathrm{C}_{A}^{1 / 3} \times \mathrm{C}_{B}^{2 / 3}\). The reaction has order \(\frac{1}{3}\) with respect to A and \(\frac{2}{3}\) with respect to B.
∴ n = n_A + n_B = \(\frac{1}{3}\) + \(\frac{2}{3}\) = 1
Hence the order of the reaction is 1.

(14) The rate of a reaction, 2A + B → Products is 3.78 x 10^-4 M s^-1 when the concentrations of A and B are 0.3 M each. If the rate constant of the reaction is 4.2 x 10^-3s^-1 find the order of the reaction.
Solution :
Given : 2A + B → Products
Rate = R = 3.78 x 10^-4Ms^-1
[A] = [B] = 0.3 M
Rate constant = 1 = 4.2 x 10^-3 s^-1
Let the order of the reaction in A be x and in B be y.

Then, by rate law,
Rate = R = k [A]^x [B]^y 3.78 x 10^-4
= 4.2 x 10^-3(0.3)^x(0.3)^y
= 4.2 x 10^-3 (0.3)^x+y
∴ \(\frac{3.78 \times 10^{-4}}{4.2 \times 10^{-3}}\) = (0.3)^x+y
0.09 = (0.3)^x+y
(0.3)² = (0.3)^x+y                        .
∴ x + y = 2
Hence the order of overall reaction is 2.
Answer:
The order of the reaction is 2.

(15) The rate of the reaction, A → Products is 1.25 x 10^-2 M/s when concentration of A is 0. 45 M. Determine the rate constant if the reaction is
(a) first order in A
(b) second order in A.
Solution :
Given : A → Products
Rate = R = 1.25 x 10^-2 M/s
[A] = 0.45 M

(a) Rate constant, k = ? if order is one.
For first order, rate law is, R = k [A]
∴ \(k=\frac{R}{[\mathrm{~A}]}=\frac{1.25 \times 10^{-2}}{0.45}\)
= 2.78 x 10^-2s^-1

(b) Rate constant, k =? if order is two. For second order, rate law is, R = k [A]²

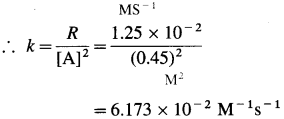
Answer:
(a) Rate constant, k = 2.78 x 10^-2
(b) Rate constant, k = 6.173 x 10^-2

Question 24.
Define and explain the term elementary reaction.
Answer:
Many reactions take place in a series of steps. Such reactions are called complex reactions. Each step taking place in a complex reaction is called an elementary reaction. This shows that a complex reaction is broken down in a series of elementary chemical reactions.

By adding all the elementary steps of a complex reaction we get the overall reaction.

The mechanism of a reaction is decided from the sequence of the elementary steps that are added to give overall reaction.

Elementary reaction : It is defined as the reac­tion which takes place in a single step and cannot be divided further into simpler chemical reactions.

The order and molecularity of the elementary reaction are same.

Some reactions take place in one step and cannot be broken down into simpler reactions. For example,

C₂H₅I_(g) → C₂H_4(g) + HI_(g)
O_3(g) → O_2(g) + O_(g)

Question 25.
Define and explain the term molecularity of a reaction. Give examples.
OR
Define the molecularity of a chemical reaction.
Answer:
Molecularity : The molecularity of an elementary reaction is defined as the number of molecules (or atoms or ions) which take part in a chemical reaction.

Explanation :

• The molecularity of a reaction is always integral.
• It cannot be determined experimentally.
• The minimum value of the molecularity is one.
• It cannot have fractional or zero values.
• The reactions are classified according to the mole­cularity as follows :

(a) Unimolecular reaction (OR First order reac­tion) : In this only one molecule takes part in the reaction, e.g., N₂O₅₍g) → 2NO_2(g) + \(\frac{1}{2}\)O_2(g)

The rate law expression for this reaction is, Rate = k [N₂O₅]. Hence it is unimolecular and first order.

Other unimolecular reactions are,
O_3(g) → O_2(g) + O_(g)
C₂H₅I_(g) → C₂H_2(g) + HI_(g)

(B) Bimolecular reaction In this two molecules take part in the reaction,
e.g., 2HI_(g) → H_2(g) + I_2(g)
O_3(g) + O_(g) → 2O_2(g)
2NO_2(g) → 2NO_(g) + O_2(g)

(c) Trimolecular reaction: In this three molecules take part in the reaction.
e.g., 2NO_(g) + O_2(g) → 2NO_2(g)

The higher molecularity is rare since the prob ability of simultaneous collisions between more molecules is very low.

Question 26.
Explain order and molecularity of elementary reactions.
Answer:
(1) The order and molecularity of elementary reaction are same.
(2) Consider second order bimolecular reaction,
2NO_2(g) → 2NO_(g) + O₂.
(3) The rate of the reaction is given by, Rate = k [NO₂]²
(4) Similarly consider unimolecular first order reaction,
C₂H₅I_(g) → C₂H_4(g) + HI_(g)
Rate = k [C₂H₅I]

Question 27.
Define and explain the term rate-determining step.
Answer:
(1) Many chemical reactions take place in a series of elementary steps. Among many steps of the reaction, one of the steps is the slowest step compared to other steps.

Rate determining step : The slowest step in the reaction mechanism which involves many steps is called the rate-determining step.

(2) Example :
Consider decomposition of gaseous NO₂Cl.
2NO₂Cl_(g) → 2NO_2(g) + Cl_2(g)
This reaction takes place in two steps :
Step I : \(\mathrm{NO}_{2} \mathrm{Cl}_{(g)} \stackrel{k_{1}}{\longrightarrow} \mathrm{NO}_{2(\mathrm{~g})}+\mathrm{Cl}_{(\mathrm{g})}\) (slow, unimolecular)

Step II: \(\mathrm{NO}_{2} \mathrm{Cl}_{(g)} \stackrel{k_{2}}{\longrightarrow} \mathrm{NO}_{2(\mathrm{~g})}+\mathrm{Cl}_{(\mathrm{g})}\) (fast, bimolecular)

2NO₂CI_(g) → 2NO_2(g) + CI_2(g) (overall reaction)

Among two steps, first step being slower represents rate-determining step. The rate law can be represented as, Rate = k₁ [NO₂CI]

Hence, the reaction is first order.

In this Cl_(g) is formed as a reaction intermediate.

Question 28.
What are the features of rate-determining step?
Answer:
Features of rate-determining step :

• The overall reaction can never occur faster than its rate-determining step.
• The rate-determining step can occur anywhere in the reaction mechanism and depends on nature of reactants, conditions of the reaction, etc.
• The rate law of a rate-determining step can directly be obtained from its stoichiometric equation.
• The rate law of a rate-determining step can directly be obtained from its stoichiometric equation.

Question 29.
What is reaction intermediate? Explain with an example.
Answer:
Reaction intermediate : The additional species other than the reactants or products formed in the mechanism during progress of the reaction is called reaction intermediate.

Features of reaction intermediate :

• The reaction intermediate appears in the reaction mechanism but does not appear in the overall reaction or in the products.
• It is always formed in one step and consumed in the subsequent step in the mechanism.
• Its concentration is very small and cannot be determined easily.
• Rate of the reaction is independent of concentration of this intermediate.
• The life period of the reaction intermediate is extremely small, hence cannot be isolated.
• The composition of the reaction intermediate, decides the mechanism of the reaction.
• Consider decomposition of gaseous NO₂Cl. 2NO₂Cl_(g) → 2NO_2(g) + Cl_2(g)

This reaction takes place in two steps :
Step I : \(\mathrm{NO}_{2} \mathrm{Cl}_{(\mathrm{g})} \stackrel{k_{1}}{\longrightarrow} \mathrm{NO}_{2(\mathrm{~g})}+\mathrm{Cl}_{(\mathrm{g})}\) (slow, unimolecular)

Step II : \(\mathrm{NO}_{2} \mathrm{Cl}_{(\mathrm{g})}+\mathrm{Cl}_{(\mathrm{g})} \stackrel{k_{2}}{\longrightarrow} \mathrm{NO}_{2(\mathrm{~g})}+\mathrm{Cl}_{2(\mathrm{~g})}\) (fast, bimolecular)
2NO₂Cl_(g) → 2NO_2(g) + Cl_2(g) (overall reaction)
Cl formed in Step I is removed in Step II, Hence Cl is a reaction intermediate.

Question 30.
Identify the molecularity and write the rate law for each of the following elementary reactions :
(a) NO_(g) + O_3(g) → NO_3(g) + O_(g)
(b) H₂I_(g) + I_(g) → 2HI_(g)
(c) CI_(g) + Cl_(g) + N_2(g) → N_2(g)
Answer:
NO_(g) + O_3(g) → NO_3(g) + O_(g) Molecularity is 2.
Rate law : Rate = k [NO] x [O₃]

(b) H₂I_(g) + I_(g) → 2HI_(g) Molecularity is 2.
Rate law : Rate = k [H₂I] x [I]

(c) Cl_(g) + Cl_(g) + N_2(g) →Cl_2(g) + N_2(g) Molecularity is 3.
Rate law : Rate = k [Cl]²

Question 31.
Write molecularity of the following reaction:
2NO_(g) + O_2(g) → 2NO_2(g).
Answer:
For the reaction, 2NO_(g) + O_2(g) → 2NO_2(g) Molecularity = 3.

Question 32.
How Is reaction intermediate predicted in the reaction?
Answer:
(1) When a reaction takes place in more than one steps, then a substance produced in one step is removed in the next step is called reaction intermediate.
(2) For example,
(I) NO_(g) + O_3(g) → NO_3(g) + O_(g)
(ii) NO_3(g) + O_(g) → NO_2(g) + O_(g)
In the reaction. NO₃ and O are reaction intermediates.

Question 33.
A certain reaction occurs in the following steps :
(i) Cl_(g) + O_3(g) → ClO_(g) + O_2(g)
(ii) ClO_(g) + O_(g) → Cl_(g) + O_2(g)
(a) Write the chemical equation for overall reaction.
(b) Identify the reaction intermediate.
(c) Identify the catalyst.
(d) What is the molecularity of each step?
Answer:
Step I : Cl_(g) + O_3(g) → ClO_(g) + O_2(g)
Step II : ClO_(g) + O_(g) → Cl_(g) + O_2(g)
(a) Overall reaction is obtained by adding both the reactions.
O_3(g) + O_(g) → 2O_2(g)
(b) Reaction intermediate is ClO_(g) which is formed in the first step and removed in the second step.
(c) Cl_(g) acts as a catalyst. It is an example of homo-geneous catalysis in which catalyst Cl_(g) forms an intermediate ClO_(g) and again is released in the second step.
(d) Since both the steps involve two reactants each, both the steps are bimolecular.

Question 34.
The rate law for the reaction 2H_2(g) + 2NO_(g) → N_2(g) + 2H₂O_(g) is given by rate = k [H₂] [NO]².
The reaction occurs in the following two steps :
(i) H_2(g) + 2NO_(g) → N₂O_(g) + H₂O_(g)
(ii) N₂O_(g) + H_2(g) → N_2(g) + H₂O_(g)
What is the role of N₂O in the mechanism? What is the molecularity of each of the elementary steps?
Answer:
N₂O is a reaction intermediate which is formed in the first step and removed in the second step. Molecularity of the elementary steps :
(a) First step – Termolecular.
(b) Second step-Bimolecular.

Question 35.
What is the rate law for the reaction,
NO_2(g) + CO_(g) → NO_(g) + CO_2(g)
The reaction occurs in the following steps :
NO₂ + NO₂ → NO₃ + NO (slow)
NO₃ + CO → NO₂ + CO₂ (fast)
What is the role of NO₃?
Answer:
Overall reaction :
NO_2(g) + CO_(g) → NO_(g) + CO_2(g)
Step-I NO₂ + NO₂ → NO₃ + NO (slow) (slow)
Step-II NO₃ + CO → NO₂ + CO₂ (fast)

(A) From first rate determining slow step, rate law is, Rate = k[NO₂]²
(B) Role of NO₃ : In the reaction, NO₃ is the reaction intermediate which is formed in first step and removed in the second step.

Question 36.
The rate law for the reaction 2NO_(g) + Cl_2(g) → 2NOCl_(g) is given by rate = k[NO][Cl₂]. The reaction occurs in the following steps :
(i) NO_(g) + Cl_2(g) → NOCl_2(g)
(ii) NOCl_2(g) + NO_(g) → 2NOCl_(g)
(a) Is NOCl₂ a catalyst or reaction intermedi-ate? Why?
(b) Identify the rate determining step.
Answer:
(a) NOCl₂ is a reaction intermediate since it is formed in the first step and removed in the second step. It is not a catalyst since it was not present in the first step or on reactant side nor in the second step on product side.
(b) Since rate law is, Rate = k[NO][Cl₂], and the sub-stances NO and Cl₂ are present in the first step as reactants, it is the slow and rate-determining step.

Question 37.
The rate law for the reaction 2H_2(g) + 2NO_(g) → N_2(g) + 2H₂O_(g) is given by rate = k[H₂][NO]². The reaction occurs in the following steps :
(i) H₂ + 2NO → N₂O + H₂O
(ii) N₂O + H₂ → N₂ + H₂O
What is the role of N₂O in the mechanism? Identify the slow step.
Answer:
(a) N₂O is the reaction intermediate since it is formed in the first step and removed in the second step.
(b) By rate law, Rate = k [H₂][NO]². Since the first step involves the substances H2 and NO, it is the slow and rate-determining step.

Question 38.
What are integrated rate laws?
Answer:
Integrated rate laws : The equations which are obtained by integrating the differential rate laws (expressions) and which provide direct relationship between the concentrations of the reactants and time are called integrated rate laws.

For example, integrated rate law for first order reaction is represented as,
\(k=\frac{2.303}{t} \log _{10} \frac{[\text { Reactant }]_{\text {final }}}{[\text { Reactant }]_{\text {initial }}}\)

Question 39.
Derive the expression for integrated rate law (equation) for the first-order reaction.
Answer:
Consider the following first-order reaction, A → B The rate of the chemical reaction is given by the rate law expression as, Rate, R = k [A] where [A] is the concentration of the reactant A and k is the velocity constant or specific rate of the reaction.
The instantaneous rate is given by,

If [A₀] is the initial concentration of the reactant and [A]_t at time t, then by integrating the above equation,

This is the integrated rate equation for the first order reaction. This is also called integrated rate law.

Question 40.
How is the integrated rate equation for the first order reaction represented by considering the concentration of the product?
Answer: The
integrated rate equation for the first order reaction can be represented as,
\(k=\frac{2.303}{t} \log _{10} \frac{[\mathrm{A}]_{0}}{[\mathrm{~A}]_{t}}\) where [A]₀ is the initial concentration of the reactant (at time, 1 = 0) and [A]_t is that at time t. Consider the reaction, A → B

If a is the initial concentration of the reactant A and x is the concentration of the product B after time t, then

Question 41.
Explain the exponential rate law expression for the first order reaction.
Answer:
The integrated rate equation for the first order reaction can be represented as,
\(k=\frac{1}{t} \log _{\mathrm{e}} \frac{[\mathrm{A}]_{0}}{[\mathrm{~A}]_{t}}\)
where k is a rate constant, [A]₀ and [A]_t are initial and final concentrations of the reactant after time t.
∴ k = \(-\frac{1}{t} \log _{\mathrm{e}} \frac{[\mathrm{A}]_{t}}{[\mathrm{~A}]_{0}}\)

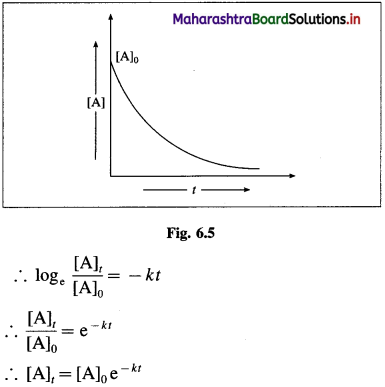
where [A]₀ and [A]_t are the concentrations of the reactant when t = 0 and t = t respectively.

Thus, the concentration of the reactant decreases exponentially with time and the time required to complete the first order reaction will be infinity.

Another feature of the exponential behaviour is the time required to complete a definite fraction of the reaction is always constant. Therefore, the first order reactions are also described in terms of the half-life of the reaction ™.

Question 42.
What are the units of rate constant of first order reaction?
Answer:
The units of rate constant (k) for the first order reaction is per time (or s^-1).

Question 43.
Give three examples of first order reaction.
Answer:
The examples of first order reaction are :
(1) Decomposition of H₂O₂ :
2H₂O_2(I) → 2H₂O₍₁₎ + O_2(g) Rate = k[H₂O₂]
(2) Decomposition of N₂O_s :
2N₂O_5(g) → 4NO_2(g) + O_2(g) Rate = k[N₂O₅]
(3) Isomerisation of cyclopropane to propene :

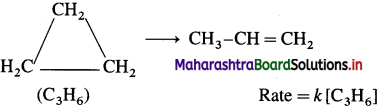
Question 44.
Write a note on a zero order reaction.
OR
What is a zero order reaction? Explain.
Answer:
(1) Definition : Zero order reaction : A reaction in which the rate of the reaction does not depend on the concentration of any reactant taking part in the reaction is called zero order reaction.
(2) Explanation : For example, consider photochemical reaction between H₂ and Cl₂ gases.

In this the rate of the reaction remains constant throughout the progress of the reaction, even if the concentrations of the reactants decrease with time, until the reactant has reacted entirely.

Hence, by the rate law,
R = k [H2]° [Cl2]° = k (constant).

Question 45.
Derive the expression for integrated rate law for zero-order reaction A → Products.
Answer:
Consider a zero order reaction, A → Products
The rate of the reaction is, Rate \(=\frac{-d[\mathrm{~A}]}{d t}\)

By rate law,
Rate = k x [A]⁰ = k
∴ – d[A] = k x dt

If [A]₀ is the initial concentration of the reactant A at t = 0 and [A]_t is the concentration of A present after time t, then by integrating above equation,

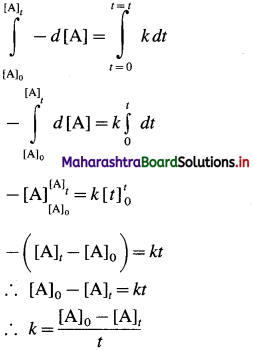
This is the integrated rate law expression for rate constant for zero order reaction.
∴ k x t = [A]₀ – [A]_t
∴ [A]_t = – kt + A₀

Question 46.
How would you obtain the unit of the velocity constant k for (i) the first order reaction (ii) the zero order reaction?
Answer:
(i) For a first order reaction :
Consider the reaction,
A → B
The rate (R) of the reaction will be, R = k [A] = kc, where [A] is concentration in mol dm^-3
Hence, the SI unit of velocity constant for the first order reaction is second^-1.

(ii) For a zero order reaction :
The rate of reaction is R = k [A]⁰ = k
Hence, the velocity constant k has the unit of the rate of the reaction, i.e., mol dm^-3 s^-1.

Question 47.
Obtain an expression for half-life period of zero order reaction.
Answer:
The rate law expression for zero order reaction is, [A]_t = – kt + [A]₀
where [A]₀ and [A]_t are the concentrations of the reactant at time, t = 0 and after time t respectively, Half-life period, t_1/2 is the time when the concentration reduces from [A]₀ to [A]₀/2. i.e., at t = t_1/2, [A]_t = [A]₀/2.

Hence for a zero-order reaction, the half-life period is directly proportional to the initial concentration of the reactant.

Question 48.
Give the examples of zero order reactions.
Answer:
Zero order reactions are not common. They take place under special conditions. They are hetero-geneous catalysed reactions generally involving metals as catalysts.

(1) Decomposition NH₃ on Pt surface :

(2) Decomposition of N₂O to N₂ and O₂ on Pt :

(3) Decomposition of PH₃ on hot tungsten catalyst at high pressure.

Question 49.
Decomposition of NH_3(g) on platinum surface at high temperature is a zero order reaction. Explain.
Answer:

• The decomposition of NH_3(g) on platinum surface is represented as,
  2NH_3(g) \(\frac{1130 \mathrm{~K}}{\mathrm{Pt}}\) N_2(g) + 3H_2(g)
• Since it is a heterogeneous catalysed reaction, NH₃ gaseous molecules at high pressure are adsorbed on the metal surface covering the surface area.
• The number of NH₃ molecules adsorbed is small compared to NH₃ molecules in the gaseous phase.
• Only the molecules adsorbed on the surface get decomposed. Hence rate of the decomposition becomes independent of the concentration (pressure) of NH₃. Therefore the decomposition reaction is zero order.

Question 50.
The catalysed decomposition of nitrous oxide (N₂O) to nitrogen and oxygen is a zero order reaction. Explain.
Answer:

• The decomposition of N₂O_(g) on platinum can be represented as, \(2 \mathrm{~N}_{2} \mathrm{O}_{(\mathrm{g})} \stackrel{\mathrm{Pt}}{\longrightarrow} 2 \mathrm{~N}_{2(\mathrm{~g})}+\mathrm{O}_{2(\mathrm{~g})}\)
• Since it is heterogeneously catalysed reaction, N₂O gaseous molecules are adsorbed on the metal surface covering the surface area.
• The number of N₂O molecules adsorbed is small compared to N₂O molecules in the gaseous phase.
• Only the molecules adsorbed on the metal surface get decomposed. Hence rate of decomposition becomes independent of the concentration (pressure) of N₂O. Therefore the decomposition of N₂O is a zero order reaction.

Question 51.
Inversion of cane sugar (sucrose) is a pseudo-first-order reaction. Explain.
OR
The reaction,

Can it be of pseudo-first-order type?
Answer:
The inversion of cane sugar (sucrose) is an acid catalysed hydrolysis reaction which can be represented as,

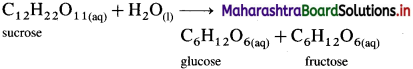
This is a bimolecular reaction. Hence, the true rate law for the reaction should be, Rate = k[C₁₂H₂₂O₁₁] [H₂O]. This shows that the reaction should be second order.

Since water (H₂O) is in large excess, its concentration remains constant and the rate depends only upon the concentration of cane sugar.

∴ Rate = k[C₁₂H₂₂O₁₁]

Therefore the second order true rate law becomes first order rate law. Hence the inversion of cane sugar is a pseudo first order reaction.

Solved Examples 6.4-6.5

Question 52.
Solve the following :

(1) For the reaction 2A + B → products, find the rate law from the following data :

[A]/M	[B]/M	rate/Ms-1
0.3	0.05	0.15
0.6	0.05	0.30
0.6	0.2	1.20

Solution:
In steps (i) and (ii), the concentration of A is doubled but the concentration of B remains constant. Since the rate is doubled the rate is proportional to the concentration of A or R α [A] and hence with respect to A order of the reaction is 1 or n_A = 1.

In steps (ii) and (iii), the concentration of A is kept constant but the concentration of B is increased 4 times and rate of the reaction is increased 4 times. Hence the rate of reaction is proportional to concentration of B, R α [B] and hence with respect of B, order is 1 or n_B = 1. Hence rate law will be, Rate = k [A] x [B].

(2) In a first order reaction A → product, 80 % of the given sample of compound decomposes in 40 min. What is the half life period of the reaction ?
Solution :

Answer:
Half life period = 17.22 min

(3) The reaction A + B → products is first order in each of the reactants.
(a) How does the rate of reaction change if the concentration of A is increased by factor 3?
(b) What is the change in the rate of reaction if the concentration of A is halved and concen­tration of B is doubled?
Solution :

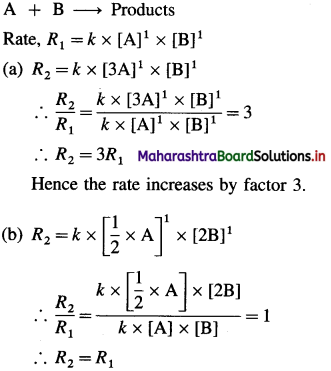
Hence the rate remains the same.
Answer:
(a) The rate increases by factor 3.
(b) The rate remains the same.

(4) Half-life period of a first order reaction is 41.09 min. Calculate rate constant in per second.
Solution :
Given : Half-life period = t_1/2
= 41.09 min = 41.09 x 60 s
= 2.465 x 10³s
Rate constant = k = ?
For a first order reaction,
\(\begin{aligned}
k &=\frac{0.693}{t_{1 / 2}} \\
&=\frac{0.693}{2.465 \times 10^{3}}
\end{aligned}\)
= 2.81 x 10^-4 s^-1
Answer:
Rate constant = k = 2.81 x 10^-4 s^-1

(5) A first order reaction takes 15 minutes to com­plete 25%. How much will it take to complete 65 %?
Solution:
(i) Given : For 25% completion, t₁ = 15 min.
For 35 % completion, t₂ = ?

Answer:
Time required to complete 65 % reaction = 547 min

(6) Gaseous A₂ dissociates as, A_2(g) → 2A_(g). Initial pressure of A₂ is 0.8 atm. After 20 minutes the pressure is 1.1 atm. Calculate rate constant and half-life period for the reaction.
Solution :
Given : [A]₀ = Initial pressure = P₀ = 0.8 atm
Final pressure = Total pressure = P_T = 1.1 atm
Rate constant = k = ?
Half life period = t_1/2 = ?
A_2(g) → 2A_(g)
P₀ – x 2x
Pressure of A₂ = P_t = P₀ – x
Total pressure of the mixture,
P_T = P₀ – x + 2x = P₀ + x
∴ x = P_T – P₀
∴ P_t = P₀ – X = P₀ – (P_T – P₀) – 2P₀ – P_T
\(k=\frac{2.303}{t} \log _{10} \frac{[\mathrm{A}]_{0}}{[\mathrm{~A}]_{t}}\)

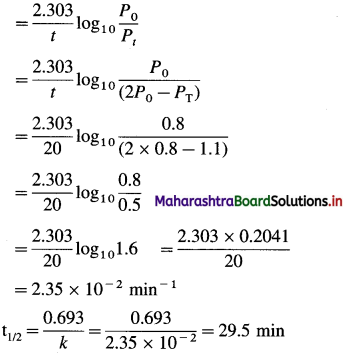
Answer:
Rate constant = k = 2.35 x 10^-2 min^-1
Half-life period = t_1/2 = 29.5 min

(7) The decomposition of N₂O_5(g) at 320 K according to the following equation follows first order reaction :
N₂O_5(g) → 2NO_2(g) + \(\frac{1}{2}\)O_2(g)
The initial concentration of N₂O_5(g) is 1-24 x 10^-2 mol. L^-1 and after 60 minutes,
0.20 x 10^-2 mol. L^-1. Calculate the rate con­stant of the reaction at 320 K.
Solution :
Given :
Initial concentration

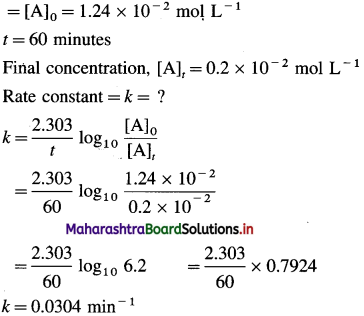
(8) From the following data for the liquid phase reaction A → B, determine the order of reaction and calculate its rate constant:

t/s	0	600	1200	1800
[A]/Mol L-1	0.624	0.446	0.318	0.226

Solution:

Answer:
Rate constant = k = 5.618 x 10^-4 s^-1

(9) The concentration of a reactant in a first-order reaction A → products, varies with time as follows :

t/min	0	10	20	30	40
[AJ/M	0.0800	0.0536	0.0359	0.0241	0.0161

Show that the reaction is first order.
Solution :
Given : A → Products
[A]₀ = 0.08 M

Since all the values of rate constant using first order rate law equation come constant, the reaction is of first order.
Answer:
Order of the reaction is one.

(10) In a first order reaction x → y, 40% of the given sample of compound remains unreacted in 45 minutes. Calculate rate constant of the reac­tion.
Solution :

Answer:
k = 0.02036 min^-1

(11) If the half-life period of a zero order reaction with initial concentration 0.1 M is 21.3 min, what will be the half-life when the concentration is 0.3 M?
Solution :
Given : Reaction is zero order. t_1/2 = 21.3, when
initial concentration = [A]₁ x = 0.1 M t_1/2 = 2 when
initial concentration = [A]₂ = 0.3 M
For zero order reaction, t_1/2 = \(\frac{[\mathrm{A}]_{0}}{2 k}\)

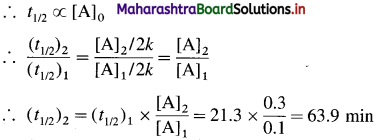
Answer:
Half life period = 63.9 min

(12) Consider the reaction 2A + 2B → 2C + D.
From the following data, calculate the order and rate constant of the reaction.

[A]0/M	[B]0/M	r0/Ms_1
0.488	0.160	0.24
0.244	0.160	0.06
0.244	0.320	0.12

Write the rate law of the reaction.
Solution :

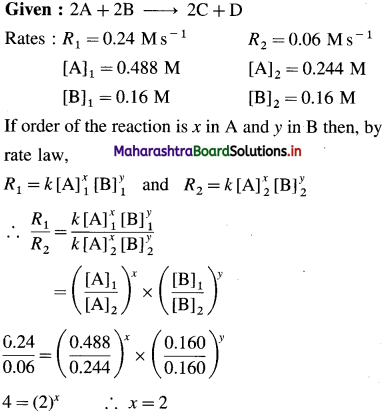
Hence the reaction is 2nd order in A.

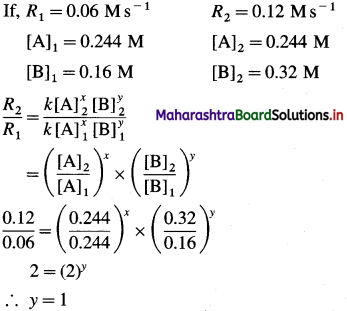
Hence the reaction is first order in B.
The order of overall reaction = n = n_A + n_B = 2 + 1 = 3
By rate law,
Rate = R = k[A]²[B]

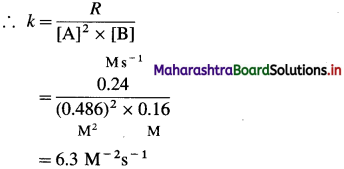
Answer:
(i) Order of reaction = 3
(ii) Rate constant = k = 63M^-2s^-1
(iii) Rate law : Rate = k [A]² [B]

(20) In acidic solution, sucrose is converted to a mixture of glucose and fructose in pseudo first order reaction. It has been found that the con-centration of sucrose decreased from 20 mmol L^-1 to 8 mmol L^-1 in 38 minutes. What is the half-life of the reaction?
Solution :
Given :
Initial concentration = [A]₀ = [sucrose]₀
= 20 mmol L^-1
= 20 x 10^-3 mol L^-1

Final concentration = [A]_t = [sucrose]_t
= 8 mmol L^-1
= 8 x 10^-3 mol L^-3
time = t = 38 min
Half-life period = t_1/2 =?
For first order reaction,

Answer:
Half-life period = t_1/2 = 28.74 min

(21) The half-life of a first order reaction is 1.7 hours. How long will it take for 20 % of the reactant to disappear?
Solution :
Given : Half-life period = t_1/2 = 1.7 hrs.


Answer:
Time required for 20% reaction = 32.86 min

(22) The gaseous reaction A₂ → 2A is first order in A₂. After 12.3 minutes, 65% of A₂ remains un­decomposed. How long will it take to decompose 90% of A₂? What is the half-life of the reaction?
Solution :
Given : A₂ → 2A
t₁ = 12.3 min
[A]₀ = 100, [A], = 65
t₂ = ? for 90 % decomposition Half-life period = t_1/2 = ?

Answer:
(i) Time required for 90% reaction = 65.8 min
(ii) Half-life periods = t_1/2 = 19.8 min

(23) Sucrose decomposes in acid solution to give glucose and fructose according to the first-order rate law. The half-life of the rection is 3 hours. Calculate the fraction of sucrose which will remain after 8 hours.
Solution :
Given : Half-life period = t_1/2 = 3 hrs
Time = t = 8 hrs

Answer:
Fraction of sucrose left = 0.1576

(24) The rate constant of a first order reaction is 6.8 x 10^-4 s^-1. If the initial concentration of the reactant is 0.04 M, what is its molarity after 20 minutes? How long will it take for 25% of the reactant to react?
Solution :
Given : Rate constant = k = 6.8 x 10^-4s^-1


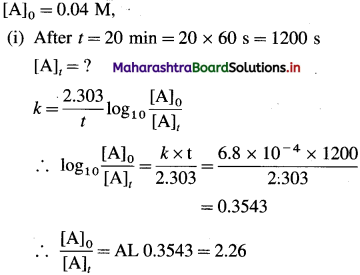
Answer:
(i) Molarity of reactant after 20 min = 0.0177 M
(ii) Time for 25 % of the reaction = 7.05 min

(25) The rate constant of a certain first-order reaction is 3.12 x 10^-3 min^-1,
(a) How many minutes does it take for the reactant concentra­tion to drop to 0.02 M if the initial concentration of the reactant is 0.045 M?
(b) What is the molarity of the reactant after 1.5 hr?
Solution :
Given : Rate constant = k = 3.12 x 10^-3 min^-1


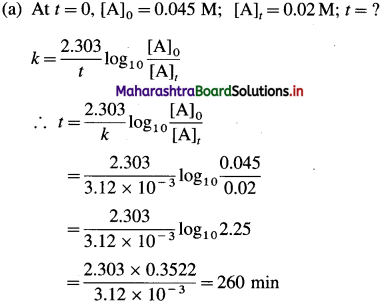
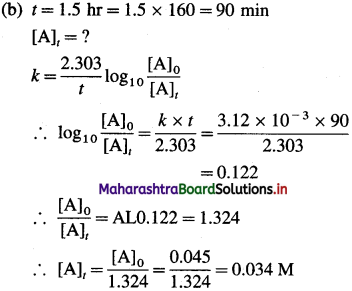
Answer:
(i) Time required to drop the concentration to 0.02 M = 260 min
(ii) Molarity after 1.5 hr = 0.034 M

(26) From the following data for the decomposition of azoisopropane,
(CH3₂)₂ CHN = NCH(CH₃)₂ → N₂ + C₆H₁₄ estimate the rate of the reaction when total pressure is 0.75 stm.

Time/s	Total pressure/atm
0	0.65
200	1.0

Solution :
Given :
(CH₃)₂CHN = NCH(CH₃)_2(g) → N_2(g) + C₆H_14(g)
At time t P₀ – x x x
At t = 0, [A]₀ = P₀ = 0.65 atm
At t = 200 s,
Total pressure = P_T = 0.75 atm, Rate =?
From the reaction,


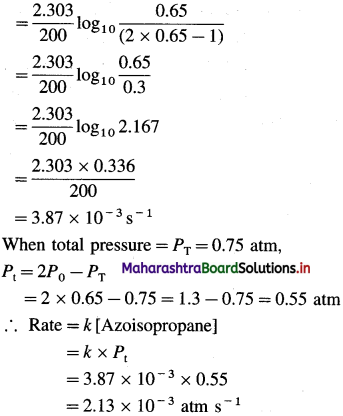
Answer:
Rate of the reaction = 2.13 x 10^-3 atm s^-1

(27) The rate constant for a zero order reaction is 0.04 Ms^-1. Calculate the half-life period of the reaction, when the initial concentration of the reactant is 0.01 M.
Solution :
Given : Order of the reaction = 0
Rate constant = k = 0.04 Ms^-1
Concentration = [A]₀ = 0.01 M
Half-life period = t_1/2 =?
For zero order reaction,
\(t_{1 / 2}=\frac{[\mathrm{A}]_{0}}{2 k}=\frac{0.01}{0.04}=0.25 \mathrm{~s}\)
Answer:
Half-life period = t_1/2 = 0.25 s

(28) A flask contains a mixture of A and B. Both the compounds decompose by first order kinetics. The half-lives are 60 min for A and 15 min for B. If the initial concentrations of A and B are equal, how long will it take for the concentration of A to be three times that of B?
Solution :
Given :
For A : tm = 60 min For B : t_1/2 = 15 min
Let initial concentrations of
[A]₀ = [B]₀ = M mol dm^-3
After time t, let the concentrations be, [B]_t = x, then [A]_t = 3x

Answer:
After 31.8 min, concentration of A will be three time that of B. ‘

Question 53.
Obtain Arrhenius equation from collision theory of bimolecular reactions.
Answer:
Consider a bimolecular reaction,
A – B + C → A + B – C
(i) Collisions of reactant molecules : The basic
requirement for a reaction to occur is reacting species A – B and C must come together and collide. The rate of reaction will depend on the rate and frequency of collisions between them. As the i concentration and temperature increase, rate of collisions increases, hence the rate of reaction increases. But the rate of reaction is low as com-pared to the rate of collisions.

(ii) Energy of activation : For fruitful collisions, the colliding molecules must possess a certain amount of energy called activation energy Ea. Due to collisions between A – B and C, there is a change in electron distribution about three nuclei namely A, B and C so that old A – B bond is weakened while new bond is partially formed between B and C, and results in the formation of an activated complex or a transition state.

Therefore transition state always has higher energy than reactants or products. Due to high energy, activated complex is unstable, short lived and decomposes into the products.

To form activated complex, the reactant mol-ecules have to climb the potential energy barrier i. e., activation energy level, hence molecular collision energy of colliding molecules must be high so that reactant molecules form activated complex and further decompose into products.

The fraction (f) of molecules at temperature T having activation energy E_a is given by f = e^-E_a/RT.

If P represents the probability of Z collisions with proper orientation then,
Reaction rate = P x Z x e^-E_a/RT,

Hence the rate constant k of the reaction may be represented as, k = A x e^-E_a/RT where A is called frequency factor or pre-exponential factor and ΔH is the enthalpy change of the reaction. This equation is called Arrhenius equation.

Question 54.
Define :
(i) Transition state or activated complex.
Answer:
Transition state or activated complex : The configuration of atoms formed from reactant molecules and which is at the peak of barrier in energy profile diagram having maximum potential energy compared to reactants and products is called transition state or activated complex.

Question 55.
If a gaseous reaction has activation energy 75k J mol^-1 at 298 K, find the fraction of successful collisions.
Answer:
Activation energy = E_a = 75 kJ mol^-1 = 75000 mol^-1; Temperature = T = 298 K The fraction (f) of successful collisions between the molecules with an energy equal to E_a is given by,


This shows that only 7 collisions out of 1014 collisions are sufficiently energetic to convert reactants into products.

Question 56.
Draw energy profile diagram and show
(i) Activated complex
(ii) Energy of activation for forward reaction
(iii) Energy of activation for backward reaction
(iv) Heat of reaction.
Answer:

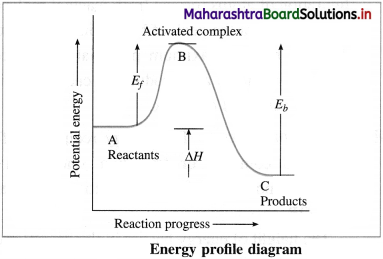
(i) B → Activated complex
(ii) E_f → Energy of activation for forward reaction
(iii) E_b → Energy of activation for backward reaction
(iv) ΔH → Heat of reaction.

Question 57.
Obtain Arrhenius equation, k = A x e^-E_a/RT
Answer:
(i) From experimental observations of variation in rate constants with temperature, Arrhenius developed a mathematical equation between reaction rate con­stant (k), activation energy (E_a) and temperature T.

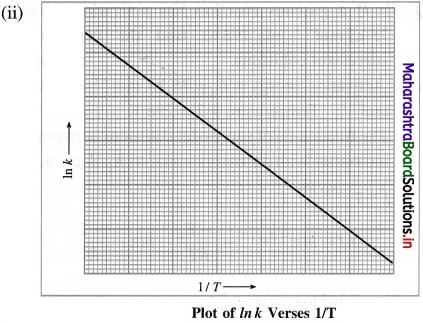
When a graph of Ink is plotted against reciprocal of temperature (1/T) a straight line with a negative slope is obtained. This is described by a mathematical equation as,

where k is a rate constant, R is the gas constant, E.a is activation energy, T is absolute temperature and the parameter A is called frequency factor or preexponential factor. This is Arrhenius equation.

Question 58.
What is a frequency factor or pre-exponential factor?
Answer:
In Arrhenius equation, k=A x e^-E_a/RT the factor A is called frequency factor and since it is a coefficient of exponential expression, e~^Ea/RT it is also called a pre-exponential factor.

In the above equation k is a rate constant at temperature T, E_a is the energy of activation and R is a gas constant.

A is related to frequency of collisions (Z) or rate of collisions. It is represented as, A = P x Z where P is the probability of collisions with proper orientations and Z is the frequency of collisions of reacting molecules.

The units of A are same as that of k.

Question 59.
Obtain a relation, \(\log _{10} \frac{k_{2}}{k_{1}}=\frac{E_{\mathrm{a}}\left(T_{2}-T_{1}\right)}{2.303 R \times T_{1} \times T_{2}}\),
OR
Obtain a relation showing variation in rate constant with temperature.
Answer:
By arrhenius equation, the rate constant k of the reaction at a temperature T is represented as, k = A x e^-E_a/RT where A is a frequency factor, R is a gas constant and Ed is the energy of activation.

By taking logarithm to the base e, we get,

If k_t and k₂ are the rate constants at temperatures T₁ and T₂ respectively, then

By measuring the rate constants k₁ and k₂ at two different temperatures T₁ and T₂, the energy of activation Ea of the reaction can be obtained.

Question 60.
How is the energy of activation determined from rate constants at two different temperatures?
Answer:
For the given reaction, rate constants k₁ and k₂ are measured at two different temperatures T₁ and T₂ respectively. Then \(\log _{10} \frac{k_{2}}{k_{1}}=\frac{E_{\mathrm{a}}\left(T_{2}-T_{1}\right)}{2.303 R \times T_{1} \times T_{2}}\) where E_a is the energy of activation.

Hence by substituting appropriate values, energy of activation E_a for the reaction is determined.

Question 61.
Obtain a relation, \(\frac{k_{2}}{k_{1}}=\frac{\left(t_{1 / 2}\right)_{2}}{\left(t_{1 / 2}\right)_{1}}\), where k₁ and k₂ are rate constants while (t_1/2)₁ and (t_1/2)₂ are halflife periods of the first order reaction at temperatures T₁ and T₂ respectively. Write the relation for activation energy.
Answer:
The rate constant k and half-life period t_1/2 are related as

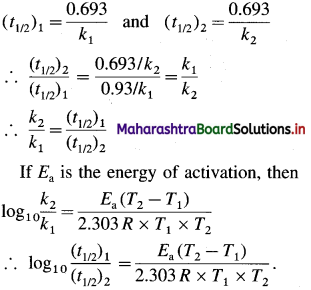
Question 62.
How does a catalyst differ from reaction intermediate?
Answer:

• A catalyst accelerates the rate of reaction, while reaction intermediate has no effect on the rate of the reaction.
• The catalyst is always present at the start of the reaction whereas reaction intermediate is produced during the mechanism of the reaction.
• A catalyst is consumed in one of the steps of mechanism and regenerated in a subsequent step while the reaction intermediate is formed in one step and consumed in subsequent step.
• The catalyst is stable but the reaction intermediate is unstable and short lived.

Question 63.
How is lowering of activation energy in the presence of a catalyst obtained?
Answer:

• In the presence of a catalyst, activation energy of a reaction is lowered, hence rate and rate constant increase.
• If ΔE_a is lowering of activation energy, while k₁ and k₂ are the rate constants of the reaction in the absence and presence of the catalyst respectively then,
  

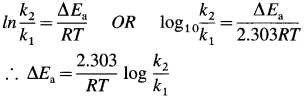
Question 64.
The rate constant of a reaction of 400 K is 1.35 x 10²s^-1. When a nickel catalyst is used, the rate constant of the reaction becomes 3.8 x 10²s^-1. Find activation energy. If the initial activation energy is 20 KJ, what will be activation energy in the presence of the catalyst?
Answer:
In the presence of a catalyst, the activation energy is lowered and rate constant is increased.

The decrease activation energy of the reaction in the presence of a catalyst will be E_a = 20 – 3.446 = 16.554 kJ.

Solved Examples 6.6-6.7

Question 65.
Solve the following :

(1) Calculate activation energy for a reaction of which rate constant becomes four times when temperature changes from 30 °C to 50 °C. (Given : R = 8.314 K^-1mol^-1)
Solution :
Given : k₂ = 4k₁
T₁ = 273 + 30 = 303 K
T₂ = 273 + 50 = 323 K
Activation energy = Ea =?

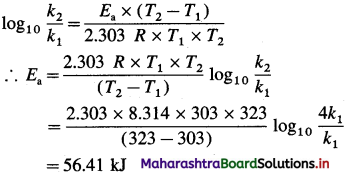
Answer:
Activation energy = E_a = 56.41 kJ

(2) The rate constant of a first order reaction are 0.58 s^-1 at 313 K and 0.045 s^-1 at 293 K. What is the energy of activation for the reaction?
Solution :

Answer:
Energy of activation = Ea = 97.46 kJ mol^-1

(3) The energy of activation for a first order reaction is 104 kJ mol^-1. The rate constant at 25°C is 3.7 x 10^-5s^-1. What is the rate constant at 30 °C?
Solution :
Given : Energy of activation = E_a = 104 kJ mol^-1 = 104 x 10³ mol^-1
Initial rate constant – k₁= 3.7 x 10^-5 s^-1
Initial temperature = T₁ = 273 + 25 = 298 K
Final temperature = T₂ = 273 + 30 = 303 K
Final rate constant = k₂ =?


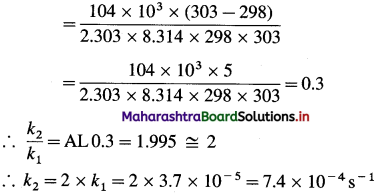
Answer:
Rate constant at 30 ⁰C = 7.4 x 10^-4 s^-1

(4) What is the activation energy for a reaction whose rate constant doubles when temperature changes from 30 °C to 40 °C?
Solution :
Given :
Initial rate constant = k₁
and final rate constant = k₂; \(\frac{k_{2}}{k_{1}}\) = 2
Initial temperature = T₁ = 273 + 30 = 303 K
Final temperature = T₂ = 273 + 40 = 313 K
Energy of activation = E_a = ?

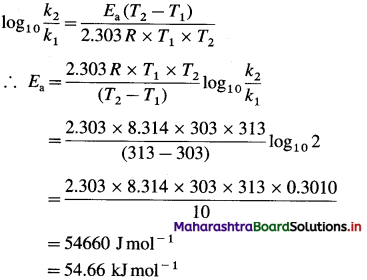
Answer:
Activation energy = E_a = 54.66 kj mol^-1

(5) The activation energy for a certain reaction is 334.4 kj mol^-1. How many times larger is the rate constant at 610 K than the rate constant at 600 K?
Solution :
Given :
Activating energy = E_a = 334.4 kJ mol^-1
= 334.4 x 10³ J mol^-1
Initial temperature = T₁ = 600 K
Final temperature = T₂ = 610 K


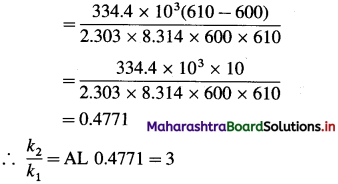
Answer:
Rate constant increase three time.

(6) The rate of a reaction at 600 K is 7.5 x 10⁵ times the rate of the same reaction at 400 K. Calculate the energy of activation for the reaction. (Hint: The ratio of rates is equal to the ratio of rate constants.)
Solution :
Given : \(\frac{R_{2}}{R_{1}}\) = 7.5 x 10⁵.
From the hint, \(\frac{R_{2}}{R_{1}}=\frac{k_{2}}{k_{1}}\) = 7.5 x 10^s
Initial temperature = T₁ = 400 K
Final temperature = T₂ = 600 K
Energy of activation = E_a = ?

Answer:
Activation energy = E_a = 135 kj mol^-1

(7) The rate constant of a first order reaction at 25 °C is 0.24 s’. If the energy of activation of the reaction is 88 kJmol^-1, at what temperature would this reaction have rate constant of 4 x 10^-2s^-1?
Solution :
Given : k₂ =0.24s^-1; k₂ =4 x 10^-2s^-1 T₁ = 273 + 25 = 298 K
Energy of activation = E_a
= 88 kJ mol^-1 = 88000 J mol^-1
T₂ = ?

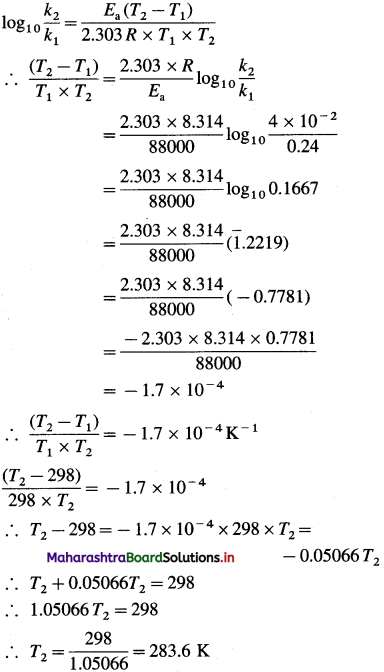
Answer:
Temperature = 283.6 K

(8) The half-life of a first order reaction is 900 min at 820 K. Estimate its half-life at 720 K if the energy of activation ot the reaction is 250 kJ mol^-1 (1.464 x 10⁵ mm).
Solution:
Given: Initial half-life period = (t_1/2)₁ = 900 min
Energy of activation = 250 kJ mol^-1
= 250 x 10³ kJ mol^-1
Initial temperature = T₁ = 820 K
Final temperature = T₂ = 720 K
Final half-life period = (t_1/2)₂ = ?


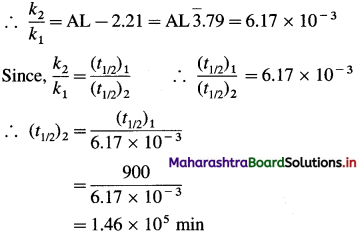
Answer:
Half-life period = 1.46 x 10⁵ min

(9) The rate of a gaseous reaction is 6.08 x 10^-2 Ms^-1 at 50°C. What will be its rate at 60°C? Energy of activation of the reaction is 18.26 kj mol^-1. (R = 8.314k^-1 mol^-1)
Solution :
Given : Initial rate = R₁ = 6.08 x 10″2Ms^-1
Energy of activation = E_a = 18.26 kJmol^-1 = 18260 mol^-1
Initial temperature = T₁ = 273 + 50 = 323 K
Final temperature = T₂ = 273 + 60 = 333 K
Final rate of the reaction = R₂ = ?

Answer:
Rate of reaction at 37°C = 7.46 x 10^-2 Ms^-1

(10) A first order gas-phase reaction has an energy of activation of 240 kj mol^-1. If the frequency factor of the reaction is 1.6 x 10¹³ s^-1, calculate its rate constant at 600 K.
Solution :
Given : Energy of activation = E_a = 240 kJ mol^-1 = 240 x 10³ mol^-1
Frequency factor = A = 1.6x 10¹³ s^-1
Temperature = T= 600 K
Rate constant = k = ?
By Arrhenius equation,

Answeer:
Rate constant = k = 2.01 x 10^-8 s^-1

(11) In the Arrhenius equation for a first order reaction, the values of ‘A’ and ‘E_a’ are 4 x 10¹³ sec^-1 and 98.6 kJ mol^-1 respectively. At what temperature will its half-life period be 10 minutes? [R = 8.314 JK^-1 mol^-2]
Solution :
Given

= 311.3 K
Answer:
Temperature = T = 311.3 K

(12) The frequency factor for a second-order reaction is 4.83 x 10¹²M^-1s^-1 at 27°C. If the rate con­stant of the reaction is 1.37 x 10^-3M^-1s^-1, find the energy of activation.
Solution :
Given : Frequency factor = A
= 4.83 x 10¹² M^-1s^-1
Rate constant = k= 1.37 x 10^-3 M^-1s^-1
Temperature = T = 273 + 27 = 300 K
Energy of activation = E_a = ?
By Arrhenius equation,

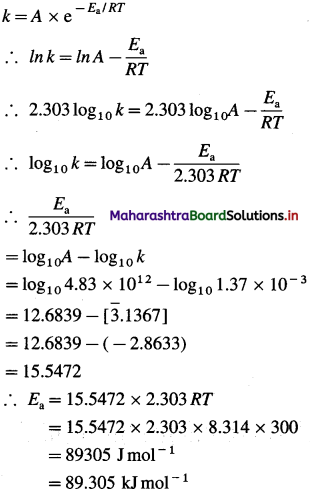
Answer:
Energy of activation = E_a = 89.305 kJ mol^–1

(13) Rate constants (k) for a reaction were measured at different temperatures. When log10ft was plotted against 1/T, the slope of the graph was 3.28 x 10³. Calculate the energy of activation.
Solution :
Given : Slope of a graph = 3.28 x 10³
Activation energy = Ea = ?
From Arrhenius equation, k = A x e^-E_a/RT
\(\log _{10} k=\frac{-E_{\mathrm{a}}}{2.303 R} \times \frac{1}{T}+\log _{10} A\)

The graph is a straight line with slope equal to E_a/2.303R
∴ \(\frac{E_{\mathrm{a}}}{2.303 R}\) = 3.28 x 10³
∴ E_a = 2.303/? x 3.28 x 10³
= 2.303 x 8.314 x 3.28 x 10³
= 62.8 x 10³ mol^-1
= 62.8 kJ mol^-1
Answer:
Activation energy = E_a = 62.8 kj mol^-1

Multiple Choice Questions

Question 66.
Select and write the most appropriate answer from the given alternatives for each subquestion :

1. The rate of a reaction is expressed in the units
(a) L mol^-1t^-1
(b) mol dm^-3 t^-1
(c) Ms
(d) M^-1s^-1
Answer:
(b) mol dm^-3 t^-1

2. For a gaseous reaction the unit of rate of reaction is
(a) L atm s^-1
(b) atm mol^-1s^-1
(c) atm s^-1
(d) mol s
Answer:
(c) atm s^-1

3. In the reaction A 4- 3B → 2C, the rate of formation of C is
(a) the same as rate of consumption of A
(b) the same as the rate of consumption of B
(c) twice the rate of consumption of A
(d) 3/2 times the rate of consumption of B
Answer:
(c) twice the rate of consumption of A

4. The units of rate of a reaction and rate constant are same for a reaction of order.
(a) zero
(b) one
(c) two
(d) fractional
Answer:
(a) zero

5. During the progress of a reaction, the rate constant of a reaction
(a) increases
(b) decreases
(c) remains unchanged
(d) first increases and then decreases
Answer:
(a) increases

6. For the reaction, 2A → 3C, the reaction rate is equal to

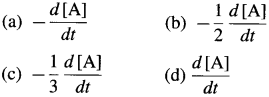
Answer:
(c)

7. For the reaction, 2X + 3Y → 4Z, reaction may be represented as

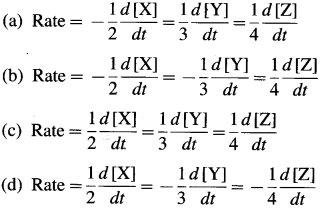
Answer:
(b)

8. For the reaction 2N₂O_5(g) → 4NO_2(g) + O_2(g) liquid bromine, which of the following rate equation is ‘incorrect’?

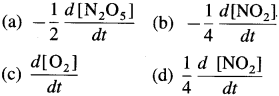
Answer:
(b)

9. The rate of reaction for certain reaction is expressed as :

The reaction is
(a) 3A → 2B + C
(b) 2B → 3A + C
(c) 2B+C → 3A
(d) 3A + 2B → C
Answer:
(c) 2B+C → 3A

10. Order of a reaction is
(a) number of molecules reacting in a reaction
(b) the number of molecules whose concentration changes during a reaction
(c) the number of molecules of reactants whose concentration determine the rate
(d) increase in number of molecules of products
Answer:
(c) the number of molecules of reactants whose concentration determine the rate

11. The unit of rate constant for zero order reaction is
(a) t^-1
(b) mol dm^-3 t^-1
(c) mol^-1 dm³ t^-1
(d) mol^-2 dm⁶ t^-1
Answer:
(b) mol dm^-3 t^-1

12. A → B is a first order reaction with rate 6.6 x 10^-5 ms^-1. When [A] is 0.6 m, rate constant of the reaction is-
(a) 1.1 x 10^-5 s^-1
(b) 1.1 x 10^-4 s^-1
(c) 9 x 10^-5 s^-1
(d) 9 x 10^-4 s^-1
Answer:
(b) 1.1 x 10^-4 s^-1

13. For a first order reaction, when the rate of a reaction is plotted against concentration of the reactant, then the graph obtained is
(a) a curve
(b) a straight line with negative slope
(c) a straight line with a positive slope
(d) a straight line with positive intercept
Answer:
(c) a straight line with a positive slope

14. For a chemical reaction, A → products, the rate of reaction doubles when the concentration of ‘A’ is increased by a factor of 4, the order of reaction is
(a) 2
(b) 0.5
(c) 4
(d) 1
Answer:
(b) 0.5

15. The order of reaction between equimolar mixture of H₂ and Cl₂ in the presence of sunlight is
(a) 0
(b) 1
(c) 2
(d) 3
Answer:
(a) 0

16. Molecularity of reaction can be
(a) zero
(b) integral
(c) fractional
(d) negative
Answer:
(b) integral

17. The reaction,
CH₃COOC₂H₅ + H₂O \(\stackrel{\mathrm{H}^{+}}{\longrightarrow}\) CH₃COOH + C₂H₅OH is of
(a) zero order
(b) first order
(c) second order
(d) pseudo first order reaction
Answer:
(d) pseudo first order reaction

18. A reaction is first order with respect to reactant A and second order with respect to reactant B. The rate law for the reaction is given by
(a) rate = k[A][B]²
(b) rate = [A][B]²
(c) rate = k [A]²[B]
(d) rate = k[A]⁰[B]²
Answer:
(a) rate = k[A][B]²

19. Molecularity of an elementary reaction
(a) may be zero
(b) is always integral
(c) may be semi-integral
(d) may be integral, fractional or zero.
Answer:
(b) is always integral

20. The unit of rate constant for first order reaction is
(a) min^-2
(b) s
(c) s^-1
(d) min
Answer:
(c) s^-1

21. The integrated rate equation for first order reaction A → products is given by

Answer:
(b)

22. Time required to complete 90% of the first order reaction is

Answer:
(a)

23. The rate constant of a first order reaction is given by

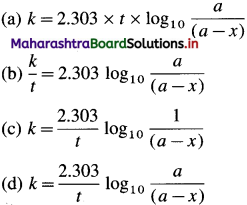
Answer:
(d)

24. The half-life of a first order reaction is 30 min and the initial concentration of the reactant is 0.1M. If the initial concentration of reactant is doubled, then the half-life of the reaction will be
(a) 1800s
(c) 15 min
(b) 60 min
(d) 900s
Answer:
(a) 1800s

25. The rate constant for a first order reaction is loos the time required for completion of 50% of reaction is-
(a) 0.0693 milliseconds
(b) 0.693 milliseconds
(c) 6.93 milliseconds
(d) 69.3 milliseconds
Answer:
(c) 6.93 milliseconds

26. The slope of the straight line obtained by plotting rate versus concentration of reactant for a first order reaction is
(a) – k
(b) – k/2.303
(c) k/2.303
(d) k
Answer:
(d) k

27. If C₀ and C are the concentrations of a reactant initially and after time t then, for a first order reaction
(a) C = C₀e^kr
(b) C₀ = 1/C e^-kr
(c) C = C₀e^-kr
(d) CO = C e^kr
Answer:
(b) C₀ = 1/C e^-kr

28. A graph corresponding to a first order reaction is

Answer:
(b)

29. For two first order reactions, A → products and B → products, k₁ and k₂ are the rate constants. The fIrst reaction (A) is slower than the second reaction (B). The graphical observation corresponding to this observation will be


Answer:
(b)

30. Half-life (t_1/2) of first order reaction is
(a) dependent of concentration
(b) independent of concentration
(c) dependent of time
(d) dependent of molecularity
Answer:
(b) independent of concentration

31. For a first order reaction, the half-life period is

Answer:
(c)

32. When half-life period of a zero order reaction is plotted against concentration of the reactant at constant temperature, the graph obtained is
(a) a curve
(b) a straight line with a positive slope
(c) a straight line with a negative slope
(d) an exponential graph
Answer:
(b) a straight line with a positive slope

33. The rate of a reaction between A and B is R = k [A]ⁿ x [B]^m On doubling the concentration of A and halving the concentration of B, the ratio of the new rate to the earlier rate of the reaction will be
(a) m + n
(b) n – m
(c) 2^(n-m)
(d) \(\frac{1}{{ }_{2} n+m}\)
Answer:
(c) 2^(n-m)

34. Consider the reaction

(a) 0,052 M/s
(b) 0.114 M/s
(c) 0.026 M/s
(d) -0.026 M/s
Answer:
(c)

35. The rate of the first order reaction A → products is 0.01 M/s, when reactant concentration is 0.2 M. The rate constant for the reaction will be
(a) 0.05 s^-1
(b) 0.05 min^-1
(c) 0.1 s^-1
(d) 0.01 s^-1
Answer:
(a) 0.05 s^-1

36. The rate constant of a reaction
(a) decreases with increasing E_a
(b) decreases with decreasing E_a
(c) is independent of E_a
(d) decreases with increasing temperature
Answer:
(a) decreases with increasing E_a

37. The slope of a graph In [A]_t versus t for a first order reaction is -2.5 x 10^-3s^-1. The rate constant for the reaction will be
(a) 5.76 x 10^-3s^-1
(b) 1.086 x 10^-3s^-1
(c) -2.5 x 10^-3s^-1
(d) 2.5 x 10^-3s^-1
Answer:
(d) 2.5 x 10^-3s^-1

38. For the reaction, Cl₂ + 2I^– → 2CI^– + I₂, the initial concentration of I^– was 0.2 mol L^– and the concentration after 20 minutes was 0.18 mol L^-1. Then the rate of formation of I₂ in mol L^– min^-1 will be
(a) 1 x 10^-3
(b) 5 x 10^-4
(c) 1 x 10^-4
(d) 2 x 10^-3
Answer:
(b) 5 x 10^-4

39. A catalyst increases the rate of the reaction by
(a) increasing E_a
(b) increasing T
(c) decreasing E_a
(d) decreasing T
Answer:
(c) decreasing E_a

40. The Arrhenius equation is
(a) A = ke^-E_a/RT
(b) A/k = e^-E_a/RT
(c) k = Ae^E_a/RT
(d) k = Aee^-RT/E_a
Answer:
(b) A/k = e^-E_a/RT

41. The Arrhenius equation is
(a) k = Ae^-RT/E_a
(b) A = ke^–E_a/RT
(c) k = Ae^-RT/E_a
(d) A = ke^E_a/RT
Answer:
(d) A = ke^E_a/RT

42. When the initial concentration of the reactant is doubled, the half-life period of the reaction is also doubled. Hence the order of the reaction is
(a) one
(b) two
(c) fraction
(d) zero
Answer:
(d) zero

43. If k₁ and k₂ are the rate constants of the given reaction in the presence and absence of the catalyst, then
(a) k₁ = k₂
(b) k₁ > k₂
(c) k₁ < k₂
(d) k₁ > k₂
Answer:
(b) k₁ > k₂

44. If the ratio of rate constants at two temperatures for the given reaction is 2.5, the ratio of corresponding half-life periods is
(a) 2.5
(b) 4
(c) 5
(d) 0.4
Answer:
(d) 0.4

45. For a zero order reaction, if Co is the initial concentration, then the half life period will be

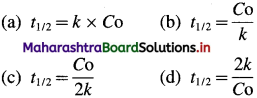
Answer:
(c)

46. The order of nuclear disintegration reaction is
(a) zero
(b) one
(c) two
(d) fraction
Answer:
(b) one

47. The unit of rate constant for zero order reaction is
(a) mol L^-2 s^-1
(b) mol^-1Ls^-1
(c) mol²L^-2s^-1
(d) mol L^-1 s^-1
Answer:
(d) mol L^-1 s^-1

48. When a graph of log₁₀k is plotted against 1 /T, the slope of the line is,

Answer:
(d)

49. The slope of a graph obtained by plotting half-life period and initial concentration of the reactant in zero order reaction is
\((a) \frac{2.303}{k}
(b) \frac{1}{k}
(c) \frac{1}{2 k}
(d) \frac{k}{2.303}\)
Answer:
(c)

50. When a graph of log, 0k against 1/T is plotted, for reaction, a graph with slope equal to 1 x 10³ is obtained. Hence the activation energy is
(a) 8.314 x 10³ Jmor^-1
(b) 3.61 kJ mol^-1
(c) 4.85 x 10³ Jmol^-1
(d) 19.1 kJ mol^-1
Answer:
(d) 19.1 kJ mol^-1

51. The correct expression for activation energy is,

Answer:
(c)

52. In the reaction, 2A_(g) → B_(g), the initial pressure of A is 2.5 atm. After 10 minutes the pressure becomes 2.2 atm. Hence the pressure of A is
(a) 1.2 atm
(b) 1.9 atm
(c) 2.3 atm
(d) 0.3 atm
Answer:
(b) 1.9 atm

53. The half-life period of zero order reaction A → product is given by –

Answer:
(c)

Balbharti Maharashtra State Board 12th Chemistry Important Questions Chapter 13 Amines Important Questions and Answers.

Maharashtra State Board 12th Chemistry Important Questions Chapter 13 Amines

Question 1.
What are amines?
Answer:
Amines : The alkyl or aryl derivatives of ammonia in which one, two or all the three hydrogen atoms attached to nitrogen are replaced by same or different alkyl or aryl groups are called amines. OR Amines are nitrogen-containing organic compounds having basic character.

Example : methyl amine : CH₃ – NH₂

Question 2.
Classify the following amines as primary, secondary and tertiary.
Answer:

Question 3.
Mention the functional group in :
(1) Primary amine
(2) Secondary amine
(3) Tertiary amine.
Answer:
(1) A primary amine has a functional group – NH₂ (amino group).
Example : ethylamine, C₂H₅ – NH₂
(2) A secondary amine has a functional group – NH – (imino group).
Example :
(3) A tertiary amine has a functional group (tertiary nitrogen atom)

Example :

Question 4.
Write common and IUPAC names of following compounds :
Answer:
(A) Primary amines :


(B) Secondary amine :

(C) Tertiary Aimines :
.

Question 5.
Give the structures of the following :
Answer:

Question 6.
Give the IUPAC names of the following amines :
Answer:

Question 7.
Write the IUPAC names of the following amines :
Answer:

Question 8.
Give the structures and IUPAC names of the following amines :
Answer:

Question 9.
Classify the following amines as primary, secondary and tertiary and write the IUPAC names.
Answer:

Question 10.
Write the structures and classify the following amines as primary, secondary, tertiary amines.
Answer:

Question 11.
Write the common and IUPAC name of a tertiary amine in which one methyl, one ethyl and one w-propyl group is attached to nitrogen.
Answer:

Question 12.
How will you prepare ethanamine from ethyl iodide?
Answer:
When ethyl iodide is heated with excess of alcoholic ammonia, under pressure at 373 K ethanamine is obtained as a major product.

Question 13.
How is a nitroalkane converted to a primary amine?
OR
What is the action of LiAlH4/ether on (i) 1-Nitropropane (ii) 2-MethyI-l-nitropropane?
Answer:
When a nitroalkane is refluxed with tin (or iron) and concentrated HCl it gives corresponding primary amine.

For example, (1) nitromethane on reduction by refluxing with Sn and concentrated HCl gives methylamine.

(2) 1-Nitropropane on reduction with Sn and concentrated HCl gives propan-1-amine.

(3) Niirobenzcnc on reducion with tin and concentrated HCI or by using H₂/Pd in ethanol gives anilinc.

(4) When nitropropane is reduced in the presence of LiAlH₄ in ether, n-propyl amine is obtained.

(5) When 2-methyl-1-nitropropane is reduced in the presence of LiAlH₄ in ether, 2-methyl propan-1-amine is obtained.

Question 14.
How will you prepare aniline from nitrobenzene?
OR
How is aniline prepared from nitro compounds?
Answer:
Nitrobenzene is reduced to aniline by passing hydrogen gas in the presence of finely divided nickel, palladium or platinum.

Question 15.
Identify the compounds A and B in the following reactions

Answer:

Question 16.
How will you obtain a primary amine from an alkyl cyanide (nitrile)?
OR
Write a short note on Mendius reduction.
Answer:
Alkyl cyanides (nitriles) on reduction by sodium and ethyl alcohol form corresponding primary amines. This reaction is called Mendius reduction.

For example; propionitrile on reduction by sodium and ethanol gives n-propyl amine (Propan-1-amine).

Methyl cyanide or acetonitrile on reduction by sodium and ethanol gives ethanaminc.

Question 17.
How will you prepare ethylamine from acetonitrile?
OR
How is ethanamine prepared from methyl cyanide?
OR
What is the action of a mixture of sodium and alcohol on acetonitrile?
Answer:
Methyl cyanide or acetonitrile on reduction by sodium and ethyl alcohol forms ethanamine. The reaction is called Mendius reduction.

Question 18.
How will convert phenyl acetonitrile to β-phenylethylamine?
Answer:
When phenyl acetonitrile is reduced in the presence of sodium and ethanol, β-phenyl ethylamine is obtained.

Question 19.
How will you obtain primary amine from an acid amide?
Answer:
Acid amides on reduction with lithium aluminium hydride or sodium, ethanol form corresponding primary amines.

For example : Acetamide on reduction with lithium aluminium hydride or sodium, ethanol gives ethylamines.

Question 20.
Explain Hoffmann degradation of amides.
Write a note on Hoffmann bromamide degradation.
Answer:
The conversion of amides into amines in the presence of bromine and alkali is known as Hoffmann degradation of amides. An important characteristic of this reaction is that an amine with one carbon less than those in the amide is formed. Thus, decreasing the length of carbon chain. This reaction is an example of molecular rearrangement and involves the migration of an alkyl or aryl group from the carbonyl carbon to the adjacent nitrogen atom. For example,

(1) When propanamide is treated with bromine and aqueous or alcoholic sodium hydroxide, ethanamine is obtained which has one carbon atom less.

(2) When benzamide is treated with bromine and aqueous or alcoholic sodium hydroxide, aniline is obtained.

Question 21.
How will you obtain methyl amine from acetamide?
Answer:
When acetamide is treated with bromine and aq or alcoholic solution of KOH, methyl amine is obtained, which has one cabon atom less.

Question 22.
How will you convert the following?

(1) Ethyl bromide to ethylamine.
Answer:

(2) Propionitrile to n-propyl amine.
Answer:

(3) Acetonitrile to ethylamine.
Answer:

(4) Phenyl acetonitrile to β-phenylethyl amine.
Answer:

(5) Acetamide to ethylamine.
Answer:

(6) Nitropropane to propan-l-amine.
Answer:

(7) Nitrobenzene to Aniline.
Answer:

(8) Benzamide to aniline.
Answer:

Question 23.
How will you prepare propan-l-amine from (1) butane nitrile (2) 1-nitropropane (3) propanamide (4) butanamide?
Answer:
(1) From butane nitrile :
When butane nitrile is reduced by sodium and ethanol, it gives propan-l-amine.

(2) From 1-nitropropane :
When 1-nitropropane is reduced in the presence of tin and cone, hydrochloric acid, propan-l-amine is obtained.

(3) From propanamide :
When propanamide is reduced in the presence of lithium aluminium hydride, propan-l-amine is obtained.

(4) From butanamide :
When butanamide is treated with bromine and aq. KOH, propan-l-amine is obtained.

Question 24.
Write a reaction to, convert acetic acid into methyl amine.
Answer:

Question 25.
Primary and secondary amines have boiling points higher than the tertiary amines. Explain why?
Answer:
(1) The N – H bond in amines is polar in nature because of electronegativities of nitrogen (3.0) and hydrogen (2.1) are different.
(2) Due to the polar nature of N – H bond, primary and secondary have strong intermolecular hydrogen bonding. Tertiary amines do not have intermolecular hydrogen bonding as there is no hydrogen atom on nitrogen of tertiary amine. Thus, intermolecular forces of attraction are strongest in primary and secondary amines and weakest in to tertiary amines. Hence, primary and secondary amines have boiling points higher than the tertiary amines.

Question 26.
Amines have boiling points higher than the hydrocarbon but lower than the alcohols of comparable masses. Explain, why?
Answer:
Amines are polar than alkanes but less polar than alcohols. Primary and secondary amines form intermolecular hydrogen bonds. This hydrogen bonding leads to an associated structure. The association is more in primary amines than that in secondary amines as there are two hydrogen atoms attached to the nitrogen atom. However, tertiary amines do not form intermolecular hydrogen bonds because they do not contain any hydrogen atoms attached to the nitrogen atom. Hence, amines have higher boiling points than the hydrocarbons but lower boiling points than the alcohols of comparable masses.

Compound	Molar mass	Boiling points (K)
nC2H5CH(CH3)2	72	300
nC4H9NH2	73	350.8
nC4H9OH	74	391

Question 27.
Arrange the following compounds in the decreasing order of their solubility in water.
(a) Ethyl amine, diethyl amine and triethyl amine.
Answer:
Diethyl amine > triethyl amine > ethyl amine
(The reason that ethyl group has greater +1 effect than methyl group)

(b) Ethyl amine, n-propyl amine and n-butyl amine.
Answer: n-butyl amine < n-propyl amine < ethyl amine

(c) n-Butane, n -butyl alcohol and n-butyl amine
Answer:
n-butyl alcohol < n-butyl amine < n-butane

Question 28.
Arrange the following compounds in the decreasing order of their boiling points.
(a) Ethane, ethyl amine and ethyl alcohol.
Answer:
Ethyl alcohol < ethyl amine < ethane

(b) Ethyl amine, n-propyl amine and n-butyl amine.
Answer:
n-butyl amine < n-propyl amine < ethyl amine

(c) n-propyl amine, ethyl methyl amine and trimethyl amine.
Answer:
n-propyl amine < ethyl methyl amine < trimethyl amine.

(d) Ethyl alcohol, dimethyl amine and ethyl amine.
Answer:
Ethyl alcohol < ethyl amine < dimethyl amine.

Question 29.
Explain the basic nature of amines with a suitable examples.
OR
Explain why amines are basic.

Question 38.
Tertiary amine (R₃N) or 3° amine is weaker base than secondary amine R₂NH or 2° amine. Explain.
Answer:


The increase in basic strength from 1° amine to 2° amine is explained on the basis of increased stabilization of conjugate acids by +1 effect of the increased number of the alkyl group. However, decreased basic strength of 3° implies that the conjugate acid of 3° amine is less stabilized and is weak base though the +1 effect of three alkyl groups in R₃NH is large.

R₂NH is best stabilized by solvation while the stabilization by solvation is very poor in R₃NH. Hence (R₃N) or tertiary amine or 3° amine is weaker base than secondary amine (R₂NH) or 2° amine.

Question 30.
Primary or aliphatic amine is a stronger base than ammonia. Explain.
Answer:
(1) The alkyl group in primary amines has +I effect i.e. (electron releasing).

The alkyl group tends to increase the electron density on the nitrogen atom. As a result, amines can donate the lone pair of electrons on nitrogen more easily than ammonia.

(2) The amine being a base, can donate a pair of electrons to an acid. The alkyl group with +I effect will disperse the positive charge on the cation more than ammonia.

Due to +I effect of alkyl group cation formed by primary amine is more stable compared to cation formed from ammonia. Also it is seen that observed increasing basic strength from ammonia to primary amine is explained on the basis of increased stabilization of conjugate acids by +I effect for the presence of alkyl (R) groups. Hence, primary or aliphatic amine is a stronger base than ammonia.

Question 31.
Aniline is less basic than ammonia. Explain.
Answer:
The less basic character of aniline can be explained on the basis of resonance shown by aniline.

Due to resonance, the nitrogen atom of amino group in aniline acquires a positive charge, hence, lone pair of electrons is less available for protonation as compared to that of ammonia. Aniline is resonance stabilized by five resonance structures. On the other hand, aniline in aqueous medium, accepts a proton does not have lone pair of electrons on nitrogen to produce a very low concentration of anilium ion and anilium ion shows only two resonance structures and therefore less stabilized than anline.

Thus, aniline is more stable than anilium ion. Hence aniline accepts proton less readily or less basic in nature than ammonia.

Question 32.
Explain the order of basicity in ammonia and aliphatic amines.
Answer:
Since nitrogen atom in ammonia molecule has a lone pair of electrons, it is a Lewis base.
Greater the availability of an electron pair, more is the basic character.

Since alkyl group (R -) is an electron releasing group with (+I) inductive effect, alkyl amines act as a stronger base than ammonia.

The decreasing order of basicity is –

The availability of a lone pair of electrons on a nitrogen atom in amines is influenced by steric factor due to crowding of alkyl groups which affects solvation along with inductive effect of alkyl groups.

Due to high energy of solvation of \(\mathrm{NH}_{4}^{+}\) ions, they acquire higher stability in aqueous solutions.

The presence of alkyl groups in secondary and tertiary amines, due to steric hindrance decrease the solvation energy.

This effect is more in tertiary amines making the tertiary ammonium ions (R₃NH⁺) unstable as compared to secondary ammonium ion (R₂N⁺H₂).
Hence the cumulative effect on the order of basicity of amines is, secondary amine > primary amine > tertiary amine > ammonia (NH₃).

Question 33.
Arrange the following amines in the decreasing order of their basic nature.
(a) Aniline, propan-l-amine and N-methylethanamine.
Answer:
N-methylethanamine < propan-l-amine < aniline

(b) Benzene-1, 4-diamine, ammonia and 4-aminobenzoic acid.
Answer:
Ammonia < benzene-1, 4-diamine < 4-aminobenzoic acid

(c) N-Methylaniline, phenylmethylamine and N-phenylaniline.
Answer:
N-Methylaniline < N-phenylaniline < phenylmethylamine

Question 34.
Arrange the following amines in the increasing order of their pKb values.
(a) Aniline, N-methylaniline and cyclohexalamine.
Answer:

(b) Phenylmethylamine, 2-aminotoluene and 2-fluoroaniline.
Answer:

(c) Aniline, 4-methoxyaniline and 4-nitroaniline.
Answer:

Question 35.
Arrange the following compounds in the decreasing order of their basic nature in the gaseous phase.
Ammonia, N-methylhexanamine, propan-1-amine and N, N-dimethylethanolamine.
Answer:
Propan-1-amine < N-methylethanamine < N,N-dimethylmethanamine < ammonia

Question 36.
Explain laboratory test for amines.
Answer:
(1) All amines are basic compounds. Aqueous solution of water soluble amines turns red litmus blue.

(2) When water insoluble amine is dissolved in aqueous HCl, forms water soluble substituted ammonium chloride, further a substituted ammonium chloride on reaction with excess aqueous NaOH regenerates the original insoluble amine.

(3) Diazotization reaction/ Orange dye test: In a sample of aromatic primary amine, 1-2 mL of cone. HCl is added. The aqueous solution of NaNO₂ is added with cooling. This solution is transfered to a test tube containing solution of β naphthol in NaOH. Formation of orange dye indicates presence of aromatic primary amino group. (It may be noted that temperature of all the solutions and reaction mixtures is maintained near 0 °C throughout the reaction).

Question 37.
Explain Hofmann’s exhaustive alkylation.
OR
Explain Hofmann’s exhaustive methylation of amines.
Answer:
Hofmann’s Exhaustive alkylation : When a primary amine is heated with excess of primary alkyl halide it gives a mixture of secondary amine, tertiary amine along with tetraalkylammonium halide

If excess of alkyl halide is used, tetraalkyl ammonium halide is obtained as major product. The reaction is known as exhaustive alkylation of amines.

Hofmann’s Exhaustive Methylation : The process of converting a primary, secondary or tertiary amine into quaternary ammonium halide by heating them with excess of methyl iodide, is called exhaustive methylation or Hoffmann’s exhaustive methylation.

Thus when methyl amine is heated with excess of methyl iodide it forms dimethylamine (secondary amine), then trimethylamine (a tertiary amine) and finally of quaternary ammonium iodide. The reaction is carried out in the presence of mild base NaHCO₃, to neutralize the large quantity of HI formed.

Question 38.
Predict the products of exhaustive methylation of following compounds.
(1) Ethylamine.
Answer:
A primary amine, ethylamine (CH₃ – CH₂ – NH₂) on exhaustive methylation, i.e., on heating with excess methyl iodide, forms secondary amine, tertiary amine and finally a quaternary ammonium salt, ethyl-trimethyl ammonium iodide.

(2) Benzylamine.
Answer:
Benzylamine C6H5CH2NH2 on exhaustive methylation i.e., on heating with excess methyl iodide forms benzylmethyl amine, benzyldimethyl ammonium chloride and finally benzyltrimethyl ammonium iodide.

Question 39.
Explain Hofmann elimination.
OR
Write a note on Hoffmann elimination.
Answer:
When tetra alkyl ammonium halide is heated with moist silver hydroxide, a quaternary ammonium hydroxide is obtained. Quaternary ammonium hydroxides are deliquescent crystalline solids and are basic in nature. Quaternary ammonium hydroxides on strong heating undergo ^-elimination to give tertiaryamine, alkenes and water, the reaction is called Hofmann elimination. The major product is least substituted alkene.

Question 40.
Write the bond line formula of the alkene which is obtained as major product from the following amines, on heating with excess of methyl iodide followed by strong heating with moist silver oxide.

Answer:

Answer:

Answer:

Question 41.
Compound X with a molecular formula C₅H₁₃N did not react with nitrous acid, but reacted with one mole of CH3I to form a salt. What is the structure of X?
Answer:
The structure of compound X is ethyl-N-methylethanamine since compound X is tertiary amine. It reacts with one mole of CH₃I to give a quaternary ammonium salt.

Question 42.
What is the action of acetyl chloride on :
(1) ethyl amine (ethanamine)
(2) diethyl amine (N-Ethylethanamine)
(3) triethyl amine?
OR
Write a short note on acylation of amines.
Answer:
The reaction of amines with acetyl chloride is called acetylation of amines.

(1) Acetyl chloride on reaction with ethylamine forms monoacetyl derivative, N-ethylacetamide (or N-acetyl ethylamine).

(2) Diethyl amine on reaction with acetyl chloride forms N-acetyl dimethylamine.

(3) Triethyl amine, being a tertiary amine does not have H atom attached to nitrogen of amine, hence it does not react with acetyl chloride.

Question 43.
What is the action of acetic anhydride on aniline?
Answer:
Aniline on reaction with acetic anhydride forms N-phenyl acetamide.

Question 44.
What is the action of benzoyl chloride on ethanamine?
Answer:
When benzoyl chloride is treated with ethanamine, N-ethyl benzamide is obtained.

Question 45.
What is the action of nitrous acid on ethylamine?
Answer:
Ethyl amine on reaction with nitrous acid in cold forms aliphatic diazonium salt, (unstable intermediate), which decomposes immediately by reaction with solvent water to produce ethyl alcohol and nitrogen gas.

Question 46.
What is the action of nitrous acid on aniline?
Answer:
Aniline reacts with nitrous acid in cold to form diazonium salt which has reasonable solubility at 273 K

Question 47.
How is benzenediazon|um chloride prepared?
Answer:
Benzenediazonium chloride is prepared by the action of nitrous acid on aniline at 273-278 K. Nitrous acid being unstable, is prepared in situ by the reaction between sodium nitrite and dilute hydrochloric acid.

Question 48.
Write resonance stabilized structures of aryl diazonium salt.
Answer:

Question 49.
Write a note on Sandmeyer’s reaction.
OR
How is aryl chloride or aryl bromide or aryl cyanide prepared from diazonium salt?
Answer:
[Replacement by Cl^–, Br^– and -CN : Sandmeyer reaction.] Freshly prepared aromatic diazonium salt on reaction with cuprous chloride gives aryl chloride, on reaction with cuprous bromide gives aryl bromide and on reaction with cuprous cyanide give aryl cyanide. The reaction in which copper (I) salts are used to replace nitrogen in diazonium salt is called Sandmeyer reaction.

Question 50.
How is aryl chloride or aryl bromide prepared by Gattermann reactions?
Answer:
The aryl chloride or bromides can also be prepared by Gattermann reactions in which diazonium salt reacts with

Question 51.
How is aryl iodide obtained from diazonium salt?
Answer:
When diazonium salt is warmed with potassium iodide, aryl iodide is obtained.

Question 52.
Explain the reduction of arene diazonium salt?
OR
How is arene obtained from arene diazonium salt?
OR
What is the action of benzene diazonium chloride on ethanol?
Answer:
Arene diazonium salt on treatment with mild reducing agents like phosphinic acid (hypophosphoric acid) or ethanol, arene is obtained.

Question 53.
How is phenol obtained from arene diazonium salt?
Answer:
When arene diazonium salt is slowly added to a large volume of boiling dilute sulphuric acid, phenol is obtained,

Question 54.
How is aryl fluoride obtained from diazonium salt?
Answer:
When fluoroboric acid is treated with the solution of diazonium salt, a precipitate of diazonium fluoroborate is obtained, which is filtered and dried. When dry diazonium fluoroborate is heated, it decomposes to give aryl fluoride. This reaction is called Balz-Schiemann reaction.

Question 55.
How is nitrobenzene obtained from benzene diazonium fluoroborate?
Answer:
When benzene diazonium fluoroborate is heated with aqueous solution of sodium nitrite in the presence of copper powder, nitrobenzene is obtained.

Benzene diazonium fluorobate can be obtained by reaction of benzene diazonium chloride with HBF₄.

Question 56.
What is meant by a coupling reaction? Explain with suitable examples.
OR
What is the action of benzene diazonium chloride on (a) phenol in alkaline medium (b) aniline?
OR
Write a note on the coupling reaction.
Answer:
Diazonium salts react with certain aromatic compounds having an electron-rich group (e.g.-OH, – NH₂, etc.) to form azo compounds. This reaction is an electrophilic substitution and is called coupling reaction. Azo compounds are brightly coloured and are used as dyes and indicators. Coupling reaction is an electrophilic substitution reaction. Benzene diazonium chloride reacts with alkaline solution of phenol to give p-hydroxy azo benzene (orange dye).

Benzene diazonium chloride reacts with aniline in mild alkaline medium to give p-aminobenzene (yellow dye).

Question 57.
What is the action of p-toluene sulphonyl chloride on ethyl amine and diethyl amine?
Answer:
(1) When ethyl amine is treated with p-toluene sulphonyl chloride, N-ethyl p-toluene sulphonamide is obtained.

(2) When diethyl amine is treated with p-toluene suiphonyl chloride. N.N-dicthyl p-toluene suiphonyl amide is formed.

Question 58.
How will you distinguish between :
(1) Ethylamine, diethyl amine and triethyl amine by using (i) nitrous acid (ii) Hinsberg’s reagent.
(2) Diethyl amine and triethyl amine by using acetic anhydride.
Answer:

Question 59.
Give a chemical test to distinguish between following pairs of compounds.
(i) Ethylamine and diethyl amine :
Answer:
Ethylamine (C₂H₅NH₂) is a primary amine while diethyl amine ( (C₂H₅)₂NH) is a secondary amine. So the two can be distinguished by the following test.

(ii) Ethyl amine and aniline :
Answer:
Ethylamine is an aliphatic amine, while aniline is an aromatic amine. So the two can be distinguished by the following test :

(iii) Aniline and benzyl amine :
Answer:

(iv) Aniline and N-ethylaniline :
Answer:

Question 60.
Compound ‘X’ with a molecular formula C₄H₁₁N did not react with Hinsberg’s reagent, but reacted with one mole of CH₃I to form a salt. What is the structure of ‘X’?
Answer:
The structure of compound ‘X’ is :

Since the compound ‘X’ does not react with NaN02 and HC1 i.e. nitrous acid (HO – N = O), it must be a tertiary amine.

The tertiary amine reacts with one mole of CH3I to give a quaternary ammonium salt.

Question 74.

p-(dimethylamino) azobenzene is yellow dye which was formerly used as a colouring agent in margarine. Write the structures of the reactants used in the preparation of this dye.
Answer:

Question 61.
Convert 3-Methyl aniline into 3-nitrotoluene.
Answer:

Question 62.
How will you bring about following conversions?
(1) N.Methyl aniline into N-methyl benzanilide.
Answer:

(2) 1.4-Dichlorobutane Into hexane-1,6-diamlne.
Answer:

(3) Benzene into 3-bromo aniline.
Answer:

(4) Chlorobenzene into 4-chioroanilinc.
Answer:

(5) 11enaniide into toluene.
Answer:

Question 63.
What is the action of aqueous bromine on aniline?
Answer:
Action of aqueous bromine on aniline : When aniline is treated with bromine water at room temperature, a white precipitate of 2, 4, 6-tri bromoaniline is obtained.

Question 64.
Explain the action of cone, nitric acid (nitrating mixture) on aniline.
Answer:
When aniline is warmed with a mixture of cone, nitric acid and cone, sulphuric acid (a nitrating mixture), a mixture of ortho, meta and para nitroaniline is obtained.

Question 65.
What is the action of acetic anhydride on aniline?
Answer:
When aniline is heated with acetic anhydride, an acetanilide is obtained.

Question 66.
How will you convert aniline to p-nltroanhline? (major product)
Answer:

Question 67.
What is the action of cone, sulphuric acid on aniline?
Answer:
Aniline on treatment with cold sulphuric acid forms anilium hydrogen sulphate which on heating with sulphuric acid at 453 K-475 K gives sulphanilic acid, (p-aminobenzene sulphonic acid) as major product.
Sulphanilic acid exists as a salt; called dipolar ion or zwitter ion. It is produced by the reaction between an acidic group and a basic group present in the same molecule.

Question 68.
How will you convert the following?
(1) Ethylamine to ethyl alcohol.
Answer:

(2) N-Methyl aniline to N-Nitroso-N-methyl aniline.
Answer:

(3) Diethylamine to N-nitrosodiethylamine
Answer:

(4) Triethylamine to triethyl ammonium nitrite.
Answer:

(5) Ethyl amine to N-ethylacetamide.
Answer:

(6) Diethyl amine to N-acetyl diethylamine.
Answer:

(7) Aniline to acetanilide.
Answer:

(8) Aniline to N-ethyl henzamide.
Answer:

(9) Ethylamine to ethyl isocyanide.
Answer:

(10) Aniline to phenyl isocyanide.
Answer:

(11) Aniline to 2,4,6-tribromoaniline.
Answer:

Question 69.
Give a plausible explanation for each of the following statements :
(1) Ethylamine is soluble in water whereas aniline is not.
Answer:
Ethylamine is soluble in water due to intermolecular hydrogen bonding resulting in the formation of C₂H₅NH₃ ion. Whereas in anline the hydrogen bonding with water is negligible due to the phenyl group (C₆H₅) is bulky and has -I effect. Therefore, aniline is nearly insoluble in water.

(2) Butan-1-ol is more soluble in water than butani-amine.
Answer:
Rutan- l-al is more soluble in watcr duc to intermoiccular hydrogen bonding. In alcohols, hydrogen bonding is through oxygen atoms. WIereas hutani-amine is less soluble in water due to the larger hydrocarbon part is hydrophobic in nature. Hence, butan-l-ol is more soluble in water than butani-amine.

(3) Butan-1-amlne has higher boiling point than N-ethylethanamine.
Answer:
Due to the presence of two H-atoms on N-atom in butait- I -amine, they undergo extensive intermolecular H-bonding while in N-cthylethanamine due to the presence of one-H atom on the N-atom, they undergo least intermolecular H-bonding. Hence, butan- l-amine has higher boiling point than-N-ethyl ethanamine.

(4) AnIline Is less basic than ethyl afine.
Answer:
Aniline (K_b4-2 x 10^-10) is less basic than ethyl amine (K_b5.1 x 10^-4). This is because -I effect of phenyl group in aniline as compared to + 1 effect of ethyl group in ethyl amine.

Due to resonance, the lone pair of electrons on the nitrogen atom gets delocalized over the benzene ring and thus less available for protonation. On the other hand, in ethyl anine, delocalization of the lone pair of electrons on the nitrogen atom by resonance is not possible. Further more, the electron density on the nitrogen atom is increased by +1 effect of the ethyl group. Hence, aniline is less basic than ethyl amine.

(5) pK_b value of diethyl amine is less than that of ethyl amine.
Answer:
The basic strength of amines is expressed in terms of pK_b values. Smaller is the value of pK_b more basic is the amine. The pK_b value of ethyl amine is 3.29 and that of diethyl amine is 3.00. Therefore, diethyl amine is more basic than ethyl amine.

(6) Aniline cannot be prepared by Gabriel phthalimide synthesis.
Answer:
In Gabriel-phthalimide synthesis of aniline, potassium phthalimide requires the treatment with chlorobenzene or bromobenzene. Since aryl halides do not undergo nucleophilic substitution reaction. Therefore, chlorobenzene or bromobenzene does not react with potassium phthalimide to give N-phenylphthalimide and hence aniline cannot be prepared by Gabriel phthalimide synthesis.

(7) Gabriel phthalimide synthesis is preferred for the preparation of aliphatic primary amines.
Answer:
In aromatic amines, the lone pair of electrons on the N-atom is delocalized over the benzene ring. As a result electron density on the nitrogen atom decreases. Whereas in aliphatic primary amines, due to +1 effect of alkyl group, electron density on nitrogen atom increases. As the pKh value of aliphatic amines is more than that of aromatic amines, aromatic amines are less basic than primary aliphatic amines. Hence, Gabriel phthalimide synthesis is preferred for the preparation of aliphatic amines.

(8) Arere diazonium salts are relatively more stable than alkyl diazonium salts.
Answer:
Arene diazonium salts are stable due to the dispersal of the positive charge over the benzene ring as shown below :

Alkane diazonium salts are unstable due to their tendency to eliminate a stable molecule of nitrogen to form carbocation. Aromatic diazonium salts have much lower tendency to remove nitrogen than aliphatic diazonium salts. Hence, arene diazonium salts are relatively more stable than alkyl diazonium salts.

(9) Tertiary amines cannot be acylated.
Answer:
Tertiary amines do not react with acetic anhydride or acetyl chloride i.e. they can be acylated because they do not contain a H-atom on the N-atom.

(10) Besides the ortho and para derivatives, considerable amount of meta derivatives is also formed during nitration of aniline.
OR
Although amino group is o- and p-directing in electrophilic substitution reactions, aniline on nitration gives substantial amount of m-nitroaniline.
Answer:
In aromatic amines, -NH₂ is an electron releasing or activating group. It activates the ortho and para positions in the benzene ring towards electrophilic substitution. When aniline is treated with nitrating mixture (cone. HNO₃+ cone. H₂SO₄), a mixture of ortho and para nitroaniline is obtained. However, a substantial amount of m-nitroaniline is also formed. Aniline being a base gets protonated in acidic medium to form anilium cation, which deactivates the ring and the substitution takes place at the meta position.

Question 70.
How will you convert :
(1) Aniline into benzyl alcohol.
Answer:

(2) Aniline into 4-bromoaniline.
Answer:

(3) Aniline into 1,3,5-tribromo benzene.
Answer:

(4) Aniline into 2,4,6-tribromo fluoro benzene.
Answer:

Question 71.
How will you convert :
(1) Propanoic acid into ethanoic acid.
Answer:

(2) Propanoic acid into ethanol
Answer:

(3) Ethanamine into propan-l-amine.
Answer:

(4) Propan-l-amine into ethanamine.
Answer:

(5) Propanoic acid into ethanamine.
Answer:

(6) Ethanamine into propanoic acid.
Answer:

(7) Benzene to aniline.
Answer:

(8) Aniline to Benzene.
Answer:

(9) Aniline into benzoic acid.
Answer:

(10) Benzoic acid into aniline.
Answer:

(11) Aniline into benzamide.
Answer:

(12) 3-Nitrotoluene into 3-methyl aniline.
Answer:

(13) 3-Methyl aniline into 3-Nitrotoluene.
Answer:

Question 72.
An organic compound ‘A’ having molecular formula C₂H₆O evolves hydrogen gas on treatment with sodium metal and on treatment with red phosphorous and iodine gives compound ‘B’. The compound ‘B’ on treatment with alcoholic KCN and on subsequent reduction gives compound ‘C’. The compound ‘C’ on treatment with nitrous acid evolves nitrogen gas. Write the balanced chemical equations for all the reactions involved and identify the compounds ‘A’, ‘B’ and ‘C;.
Answer:
A = C₂H₅OH ethanol
B = C₂H₅I ethyl iodide
C = C₂H₅CH₂NH₂ n-propyl amine
Compound C₂H₆O = C₂H₅OH

Question 73
Identify B, C and D write complete reactions :

Answer:

Question 74.
Identify the compounds B, C and D in the following series of reactions and rewrite the complete equations :

Answer:

Question 75.
Identify the compounds ‘A’ and ‘B’ in the following equation :

Answer:

Question 76.
Answer in one sentence :

(1) Arrange the following compounds in decreasing order of basic strength in their aqueous solutions. NH₃, C₂H₅NH₂, (CH₃)₂NH, (CH₃)₃N
Answer:
The decreasing order of basic strength is – (C₂H₅)₂NH > (C₂H₅)₃N > (C₂H₅)₂NH > NH₃
(The reason that ethyl group has greater +1 effect than methyl group).

(2) Arrange the following compounds in an increasing order of their solubility in water.

Answer:
The solubility increases in order in which molecular mass decreases.

(3) What is Hinsberg’s reagent?
Answer:
Benzenesulphonyl chloride (C₆H₅SO₂Cl) is known as Hinsberg’s reagent.

(4) Name the reaction in which a primary amine is formed from amide.
Answer:
Hoffmann bromamide degradation.

(5) NH₃ is a Lewis base.
Answer:
Since nitrogen in ammonia molecule has a lone pair of electrons, it is a Lewis base.

(6) How many primary amines are possible for the compound C₃H₉N?
Answer:
For the compound C₃H₉N, two primary amines are possible.

(7) State the hybridization of the nitrogen atom in amines.
Answer:
The hybridization of nitrogen atom in amines is sp³.

(8) Arrange the following compounds in an increasing order of basic strength. Aniline, p-nitroaniline, p-toluidine.
Answer:
p-nitroaniline < aniline < p-toluidine.

(9) Which of the two is more basic and why? CH₃NH₂ or NH₃
Answer:
Due to +1 effect of -CH₃ group, electron density on N-atom increases, hence methyl amine is a stronger base than ammonia.

(10) Which of the two is more basic and why? p-toluidine or aniline.
Answer:
p-toluidine is more basic due to the presence of -CH₃ group at para position. Due to +1 effect of -CH₃ group, electron density on nitrogen increases, hence the tendency to donate pair of electrons increases.

Multiple Choice Questions

Question 77.
Select and write the most appropriate answer from the given alternatives for each subquestion :

1. Which of the following is an amine?
(a) C₂H₅N(COCH₃)₂
(b) (C₂H₅)₂N – N = 0
(c) (C₂H₅)₃N
(d) All of these
Answer:
(d) All of these

2. N-methyl-N-ethyl-n-propyl amine is
(a) a primary amine
(b) a secondary amine
(c) a tertiary amine
(d) an alkyl nitrile
Answer:
(c) a tertiary amine

3. Which of the following is a tertiary amine?

Answer:
(d)

4. Tertiary butyl amine is a
(a) primary amine
(b) secondary amine
(c) tertiary amine
(d) quaternary ammonium salt
Answer:
(a) primary amine

5. The IUPAC name of

(a) ethyl propanamine
(b) ethyl butylamine
(c) 2-pentanamine
(d) 3-hexanamine
Answer:
(d) 3-hexanamine

6. The IUPAC name of ethyl dimethyl amine is ……………..
(a) 2-amino propane
(b) N,N-dimethyl ethanolamine
(c) ethyl methanamine
(d) propanamine
Answer:
(b) N,N-dimethyl ethanolamine

7. Isopropyl amine and trimethyl amine are ……………..
(a) acidic in nature
(b) electrophilic compounds
(c) structural isomers
(d) optically active compounds
Answer:
(c) structural isomers

8. N, N-dimethylethanolamine is ……………

Answer:
(b)

9. IUPAC name of diethylmethyl amine is ………………
(a) methyl amino propane
(b) N-Ethyl-N-methylhexanamine
(c) methyl diethanamine
(d) amino pentane
Answer:
(b) N-Ethyl-N-methylhexanamine

10. Ethyl bromide reacts with excess of alcoholic ammonia, the major product is …………..
(a) ethyl amine
(b) diethylamine
(c) triethylamine
(d) tetraethyl ammonium bromide
Answer:
(a) ethyl amine

11. Isopropylamine is obtained by the reduction of
(a) acetoxime
(b) acetaldoxime
(c) formaldoxime
(d) aldoxime
Answer:
(a) acetoxime

12. Which of the following compounds can be converted into amines in the presence of Na and alcohol?
(a) Alkyl nitriles
(b) Aldoxime
(c) Ketoxime
(d) All of these
Answer:
(d) All of these

13. Chloroethane when boiled with excess of aqueous-alcoholic ammonia gives hydrochloric acid and
(a) triethyl amine
(b) trimethyl amine
(c) diethyl amine
(d) ethyl amine
Answer:
(d) ethyl amine

14. How many hydrogen atoms are required for the reduction of 1-nitropropane to n-propyl amine?
(a) Four
(b) Three
(c) Six
(d) Two
Answer:
(c) Six

15. A secondary alkyl halide is heated with excess of ammonia, the major product obtained is
(a) primary amine
(b) secondary amine
(c) tertiary amine
(d) quaternary ammonium salt
Answer:
(a) primary amine

16. The true statement about ethylamine is
(a) it is weaker base than ammonia
(b) it is stronger base than diethyl amine
(c) it is stronger base than triethyl amine
(d) it is stronger base than alkali
Answer:
(c) it is stronger base than triethyl amine

17. The reaction which is given only by primary amines is
(a) acetylation
(b) alkylation
(c) reaction with HNO₂
(d) carbyl amine test
Answer:
(d) carbyl amine test

18. The amine which reacts with NaNO₂ and dil. HCl to give yellow oily compound is
(a) ethylamine
(b) isopropylamine
(c) sec-butylamine
(d) dimethylamine
Answer:
(d) dimethylamine

19. The name of the compound ‘C’ in the following series of reactions, is
(a) propan-l-ol
(b) propan-2-ol
(c) butan-l-ol
(d) butan-2-ol
Answer:
(b) propan-2-ol

20. Triethylamine when treated with nitrous acid gives
(a) an alcohol
(b) a nitrosamine
(c) a monoacetyl derivative
(d) a soluble nitrite salt
Answer:
(d) a soluble nitrite salt

21. Ammes are basic in nature because
(a) of the nitrogen atom contain or lone pair of electrons
(b) they give H+ ions in aqueous medium
(c) they form quaternary ammonium salts when heated with acids
(d) both (a) and (c)
Answer:
(a) of the nitrogen atom contain or lone pair of electrons

22. An aqueous solution of primary amine contains

Answer:
(d)

23. The basic nature of amines in an aqueous solution is in the order of
(a) tert. > sec. > pri.
(b) sec. > pri. > tert.
(b) pri. > sec. > tert.
(d) pri. > tert. > sec.
Answer:
(b) pri. > sec. > tert.

24. In trimethyl ammonium ion, the number of sigma bonds attached to nitrogen are
(a) 2
(b) 3
(c) 4
(d) 5
Answer:
(b) 3

25. The number of coordinate bond/bonds in a trialkyl ammonium ion is
(a) one
(b) two
(c) three
(d) four
Answer:
(a) one

26. The number of electrons in the valence shell of nitrogen in methyl amine is
(a) 5
(b) 3
(c) 8
(d) 7
Answer:
(c) 8

27. Ethanamine reacts with excess of acetyl chloride to form
(a) C₂H₅NHCOCH₃
(b) C₂H₅N(CH₃)₂
(c) C₂H₅N(COCH₃)₂
(d) C₂H₅N+H₃Cl
Answer:
(c) C₂H₅N(COCH₃)₂

28. The compound used for acylation of amine is
(a) (CH₃CO)₂O
(b) CH₃COOH
(c) CH₃COCl
(d) both (a) and (c)
Answer:
(d) both (a) and (c)

29. Dimethyl amine reacts with acetyl chloride to give
(a) N-acetyl methyl amine
(b) N-acetyl ethyl amine
(c) N-acetyl dimethyl amine
(d) N-acetyl diethyl amine
Answer:
(c) N-acetyl dimethyl amine

30. Identify ‘A’ in the following reaction :

Answer:
(c)

31. n-propyl alcohol is obtained when HNO₂ is treated with

Answer:
(c)

32. A mixture of CH₃NH₂, (CH₃)₂NH, (CH₃)₃N can be distinguished by using
(a) HCI
(b) HNO₂
(c) HNO₃
(d) H₂SO₄
Answer:
(b) HNO₂

33. In the acetylation reaction the H-atom of an amine is replaced by
(a) a carbonyl group
(b) an alkyl group
(c) an acetyl group
(d) an imino group
Answer:
(c) an acetyl group

34. Amines are basic in nature
(a) as they have a fishy odour
(b) as they form quaternary ammonium salts with alkyl halides
(c) due to the presence of an unshared pair of electrons on the nitrogen atom
(d) all of these
Answer:
(c) due to the presence of an unshared pair of electrons on the nitrogen atom

35. The correct order of increasing basic strength is
(a) NH₃ < CH₃NH₂ < (CH₃)₂NH
(b) CH₃NH₂ < (CH₃)₂NH < NH₃
(c) CH₃NH₂ < NH₃ < (CH₃)₂NH
(d) (CH₃)₂NH < NH₃ < CH₃NH₂
Answer:
(a) NH₃ < CH₃NH₂ < (CH₃)₂NH

36. Which of the following is the strongest base?

Answer:
(d)

37. Identify the weakest base amongst the following :
(a) p-methoxyaniline
(b) o-toluidine
(c) benzene-1, 4-diamine
(d) 4-aminobenzoic acid
Answer:
(d) 4-aminobenzoic acid

38. Amine that cannot be prepared by Gabriel phthalimide synthesis is
(a) aniline
(b) benzyl amine
(c) methyl amine
(d) iso-butyl amine
Answer:
(a) aniline

39. Which of the following exist as Zwitter ion?
(a) Salicylic acid
(b) Suphanilic acid
(c) p-Aminophenol
(d) p-Amino acetophenone
Answer:
(b) Suphanilic acid

40. Reduction of benzene diazonium chloride with Zn/HCl gives
(a) phenyl hydrazine
(b) hydrazine hydrate
(c) aniline
(d) ozo benzene
Answer:
(c) aniline

41. When primary amine reacts with CHCl₃ in alcoholic KOH, the product is
(a) aldehyde
(b) alcohol
(c) cyanide
(d) an isocyanide
Answer:
(d) an isocyanide

42. Which of the following amines cannot be prepared by Gabriel phthalimide synthesis?
(a) sec-Propylamine
(b) tert-Butylamine
(c) 2-Phenylethylamine
(d) N-Methyl benzyl amine
Answer:
(d) N-Methyl benzyl amine

43. Which of the following compounds has highest boiling point?
(a) Ethane
(b) Ethanoic acid
(c) Ethanol
(d) Ethanamine
Answer:
(b) Ethanoic acid

44. Identify the statement about the basic nature of amines.
(a) Alkylamines are weaker bases than ammonia.
(b) Arylamines are stronger bases than alkylamines.
(c) Secondary aliphatic amines are stronger bases than primary aliphatic amines.
(d) Tertiary aliphatic amines are weaker bases than arylamines.
Answer:
(c) Secondary aliphatic amines are stronger bases than primary aliphatic amines.

45. The compounds ‘A’, ‘B’ and ‘C’ react with methyl iodide to give finally quaternary ammonium iodides. Only ‘C’ gives carbylamines test while only ‘A’ forms yellow oily compound on reaction with nitrous acid. The compounds ‘A’, ‘B’ and ‘C’ are respectively.
(a) butan-1-amine, N-ethylethanamine and
N, N-dimethylethanamine.
(b) N-ethylethanamine, N, N-dimethylethanamine and butan-1 – amine.
(c) N, N-dimethylethanamine, N-ethylethanamine and butan-1-amine.
(d) N-ethylethanamine, butan-1-amine and N-ethylethanamine.
Answer:
(b) N-ethylethanamine, N, N-dimethylethanamine and butan-1 – amine.

46. Which of the following amines is most basic in nature?
(a) 2, 4-Dichloroaniline
(b) 2, 4-Dimethylaniline
(c) 2, 4-Dinitroaniline
(d) 2, 4-Dibromoaniline
Answer:
(b) 2, 4-Dimethylaniline

47. How many moles of methyl iodide are required to convert ethylamine, diethylamine and triethylamine into quaternary ammonium salt, respectively?
(a) 1, 2 and 3
(b) 2, 3 and 1
(c) 3, 2 and 1
(d) 3, 1 and 2
Answer:
(c) 3, 2 and 1

48. Which of the following amines does not undergo acetylation?
(a) t-Butylamine
(b) Ethylamine
(c) Diethylamine
(d) Triethylamine
Answer:
(d) Triethylamine

49. n-Propylamine can be prepared by catalytic reduction of
(a) n-propyl cyanide
(b) propionaldoxime
(c) acetoxime
(d) nitroethane
Answer:
(b) propionaldoxime

50. Identify the compound ‘B’ in the following series of reactions :

Answer:
(c)

51. Chloropicrin is used as
(a) antiseptic
(b) antibiotic
(c) insecticide
(d) anaesthetic
Answer:
(c) insecticide

52. Identify the compound B in the following series of reactions.
(a) n-propyl chloride
(b) propanamine
(c) n-propyl alcohol
(d) Isopropyl alcohol
Answer:
(c) n-propyl alcohol

53. Which of the following amines yields foul smelling product with haloform and alcoholic KOH?
(a) Ethyl amine
(b) Diethyl amine
(c) Triethyl amine
(d) Ethyl methyl amine
Answer:
(a) Ethyl amine

54. Which of the following compounds is NOT prepared by the action of alcoholic NH₃ on alkyl halide?
(a) CH₃NH₂
(b) CH₃-CH₂-NH₂
(c) CH₃ – CH₂ – CH₂ – NH₂
(d) (CH₃)₃CNH₂
Answer:
(d) (CH₃)₃CNH₂

Balbharti Maharashtra State Board 12th Chemistry Important Questions Chapter 8 Transition and Inner Transition Elements Important Questions and Answers.

Maharashtra State Board 12th Chemistry Important Questions Chapter 8 Transition and Inner Transition Elements

Question 1.
What are d-block elements? Give their general electronic configuration.
Answer:
Definition : d-block elements are defined as the elements in which the differentiating electron enters d-orbital of the penultimate shell i.e. (n – 1) d-orbital where ‘n is the last shell.

The general electronic configuration can be represented as, (n – n) d^{n – 10}, ns^{n – 2}

Question 2.
What is the position of the transition elements in the periodic table?
Answer:
The transition elements are placed in periods 4 to 7 and groups 3 to 12 of the periodic table.

Question 3.
In which block of the modern periodic table are the transition elements placed?
Answer:
Transition elements are placed in d-block of the modern periodic table.

Question 4.
Why are most of the d-block elements called transition elements?
Answer:

• d-block elements have electronic configuration,(n – n) d^{n – 10}, ns^{l – 2}. They are all metals.
• In the periodic table, they are placed between the ,s-block and p-block elements, i.e., in the groups between 2 and 13.
• They show characteristic properties which are intermediate between those of the elements of s-block and p-block.
• Hence, they show a transition in the properties from those of the most electropositive .v-block elements and less
• electropositive (or electronegative) p-block elements.
• Therefore, most of the d-block elements are called transition elements.

Question 5.
How many series of d-block elements are present in the long-form periodic table? Give their general electronic configuration.
Answer:
There are four series of d-block elements which are placed between 5 and p-block elements in the long-form periodic table as follows :

d-series	Period	Electronic configuration
(1) 3d-series	fourth	[Ar] 3d1 – 10, 4s1 – 2
(2) 4d-series	fifth	[Kr] 4d1 – 10, 5s1 – 2
(3) 5d-series	sixth	[Xe] 4f14 5d1 – 10 6s1 – 2
(4) 6d- series	seventh	[Rn] 5f14 6d1 – 10 7s2

Modern periodic table :

Question 6.
Represent the elements in the four series of transition elements.
Answer:

Question 7.
In which period of the periodic table, will an element, be found whose differentiating electron is a 4d-electron?
Answer:
An element whose differentiating electron is a 4d-electron will be present in fifth period of the periodic table.

Question 8.
Write the condensed electronic configuration of each series of transition elements.
Answer:
Condensed Electronic Configuration of Transition Elements

Question 9.
Write expected and observed electronic configuration of 3d-series block elements.
Answer:
Electronic configuration of 3d-series of d-block elements

Question 10.
Explain why transition elements with electronic configuration 3d44s2 and 3d94s2 do not exist.
Answer:
(1) d-orbitals are degenerate orbitals and they acquire extra stability when half-filled (3d⁵) or completely filled (3d¹⁰). Hence 3d⁴ and 3d⁹ electronic configurations are less stable.
(2) The energy difference between 3d and 4.s’ subshells is very low, hence there arises a transfer of one electron from 45 orbital to 3d orbital.
The electronic configuration changes as,
3d⁴, 4s² → 3d⁵ 4s¹
3d⁹, 4s² → 3d¹⁰ 45¹
Therefore transition elements, with electronic configurations 3d⁴, 4s² and 3d⁹, 4s² do not exist.

Question 11.
Write observed electronic configuration of elements from first transition series having half-filled d-orbitals.
Answer:
There are two elements namely Cr and Mn which have half-filled d-orbitals.
₂₄Crls²2s²2p⁶3s²3p⁶3d⁵4s¹
₂₅Mnls²2s²2p⁶3s²3p⁶3d⁵4s²

Question 12.
Explain the variable oxidation states of metals of first transition series.
Answer:

• The transition metals (or, elements) exhibit variable oxidation states due to their electronic configuration, (n – 1) d^{1 – 10} ns^{1 – 2} for the first row.
• They show only positive oxidation states due to loss of electrons from outer 45-orbital and the penultimate 3rf-orbital.
• Loss of one 45 electron forms M+ ion. Loss of two 45 electrons form M²⁺ ion.
• +2 is the common oxidation state of these elements.
• Higher oxidation states are due to loss of 3 d-electrons along with 45 electrons.
• As the number of unpaired electrons increases, the number of oxidation states shown by the element also increases.
• Sc has only one unpaired electron and it shows two oxidation states ( + 2 and + 3)
• Mn with 5 unpaired d electrons show six different oxidation states. They are +2, +3, +4, +5, +6 and + 7. Thus Mn has the highest oxidation state.
• From Fe onwards variable oxidation states decreases as the number of unpaired electron decreases.
• The last element in the series, Zn shows only one oxidation state ( + 2).

Question 13.
Show different oxidation states of 3d-series of transition elements.
Answer:
The following table shows, different oxidation states of 3d-series of transition elements.

Question 14.
Write oxidation states and outer electronic configuration of first transition series elements.
Answer:
Oxidation states of first transition series elements

Question 15.
Zinc shows only one oxidation slate. Explain.
Answer:

• The electronic conliguration of zinc is, ₃₀Zn Is² 2s² 2p⁶ 3s² 3p⁶ 3d¹⁰ 4s² or [Ar] 3d¹⁰ 4s².
• Due lo loss of two electrons from 4s suhshell Zn shows oxidation state +2. with elcctronic configuration. [Ar] ¹⁸3d¹⁰.
• Since Zn⁺² acquires an extra stability of completely fIlled 3d¹⁰ orbital. it shows only one oxidation state + 2.

Question 16.
Why is manganese more stable in the + 2 state than the + 3 state and the reverse is true for iron?
Answer:

• The electronic configuration of Mn is ₂₅Mn [Ar] 3d⁵ 4s²
• In + 2 and + 3 oxidation states, the electronic configuration of Mn is, Mn²⁺ [Ar] 3d⁵ and Mn³⁺ [Ar] 3d⁴
• Since half-filled d-orbital (3d⁵) has more stability and lower energy than 3d⁴, Mn²⁺ is more stable than Mn³⁺.
• The electronic configuration of Fe is ₂₆Fe [Ar] 3d⁶ 4s² In + 2 and + 3 oxidation states of Fe, the electronic configuration is, Fe²⁺ [Ar] 3d⁶ and Fe³⁺ [Ar] 3d⁵ Since half-filled orbital is more stable, + 3 state of Fe is more stable than + 2 state.

Question 17.
What are the electronic configurations of various ions of 3d-elements?
Answer:
Electronic configuration of various ions of 3d elements

Question 18.
Scandium shows only two oxidation states. Explain.
Answer:
Scandium has electronic configuration, ₂₁Sc : Is², 2s², 2p⁶, 3s², 3p⁶, 3d¹, 4s² Sc shows only two oxidation states namely + 2 and + 3.

• Due to the loss of two electrons from the 4s-orbital, Sc acquires + 2 oxidation state Sc^{2 +} : Is² 2s² 2p⁶ 3s² 3p⁶ 3d¹.
• Due to the loss of one more electron from the 3d-orbital, it acquires + 3 oxidation state with the extra stability of an inert element ₁₈Ar. Sc⁺³ : Is² 2s² 2p⁶ 3s² 3p⁶.
• Since Sc³⁺ acquires extra stability of inert element [Ar]¹⁸, it does not form higher oxidation state.

Question 19.
Write different oxidation states of iron.
OR
Write the electronic configurations of
(i) Fe
(ii) Fe²⁺ and
(iii) Fe³⁺.
Answer:
Oxidation states of iron are +2, +3, +4, +5, +6.
(i) ₂₆Fe : ls²2s²2p⁶3s²3p⁶3d⁶4s²
(ii) Fe²⁺ : Is² 2s² 2p⁶ 3s² 3p⁶ 3d⁶
(iii) Fe³⁺ : Is² 2s² 2p⁶ 3s² 3p⁶ 3d⁵.

Question 20.
Explain different oxidation states of chromium.
Answer:

• The observed electronic configuration of chromium is, ₂₄Cr [Ar] 3d⁵ 4s¹.
• Different possible oxidation states of Cr are 4-1 (3d⁵), + 2 (3d⁴), + 3 (3d³), + 4 (3d²), + 5 (3d¹) and + 6 (3d°).
• Although in + 1 state, Cr gets extra stability of half-filled 3d⁵-orbital, it does not exhibit + 1 state in common except with pyridine.
• Cr⁺² has few stable salts like CrCl₂, CrSO₄ while Cr⁺³ forms very stable salts like CrCl₃.
• Cr⁺⁴ and Cr ^{+ 5} are unstable oxidation states.
• Cr⁺⁶ is the most stable state due to inert gas [Ar] electronic configuration and form the salts like K₂Cr₂O₇.

Question 21.
Manganese shows variable oxidation states. Give reasons.
Answer:

• Manganese (₂₅Mn) has electronic configuration. ₂₅Mn [Ar]¹⁸ 3d⁵ 4s².
• Mn has stable half-filled d-subshell.
• Due to a small difference in energy between 3d and 4s-orbitals, Mn can lose or share electrons from both the orbitals, hence shows variable oxidation states.
• Mn shows oxidation states ranging from + 2 to + 7.

Question 22.
Write the different oxidation states of manganese. Why is + 2 oxidation state of manganese more stable than Mn³⁺?
Answer:

• The different oxidation states of Mn are +2, +3, +4, +5, + 6 and +7.
• The electronic configuration of Mn is Is² 2s² 2p⁶ 3s² 3p⁶ 3d⁵ 4s²
• + 2 oxidation state is very stable due to higher stability of half-filled 3d orbital.
• Mn³⁺ has electronic configuration, ls²2s² 2p⁶3s²3p⁶3d^A which is less stable.

Question 23.
Write the physical properties of first transition series.
Answer:
Physical properties of first transition series :

• All transition elements of the first series are metals.
• Except Zn, they are very hard and have low volatility.
• They show characteristic properties of metals. They are lustrous, malleable and ductile.
• They are good conductors of heat and electricity.
• They have high melting points and boiling points.
• Except Zn and Mn, they have one or more typical metallic structures at normal temperatures.

Question 24.
Which elements in the transition elements, 3d-series has
(i) the lowest density
(ii) the highest density?
Answer:
In 3d transition elements,
(i) Scandium (Sc) has lowest density and
(ii) Zinc (Zn) has the highest density.

Question 25.
Explain the variation in density of d-block elements.
Answer:
The densities of d-block elements are higher than 5-block elements due to higher nuclear charge which results in reduction in atomic size.

Question 26.
Explain the variation in melting points of the transition elements.
Answer:

1. All transition elements are metals and the strength of metallic bonding increases as the number of unpaired electrons increases.
2. In transition elements as atomic number increases, the number of unpaired electrons increases from (n – 1)d¹ to (n – 1 )d⁵.
   For example in 3d-series, melting points increase from ₂₁Sc to ₂₄Cr in 4d-series from ₃₉Y to ₄₂Mo, and in 5d-series from ₇₂Hf to ₇₄W.
3. After (n – l)d⁵ electronic configuration, the electrons start pairing, hence the number of unpaired electrons decrease, hence metallic character, melting points decrease from (n-1 )d6 to (n – 1)d¹⁰.
4. In all transition series the melting point increases steadily up to d⁵ configuration and after this melting point decreases regularly.
   

Question 27.
The first ionisation enthalpies of third transition series elements are much higher than those of the elements of first and second transition series. Explain.
Answer:

1. Third transition series elements have electronic configuration, 4f¹⁴ 5d^{1 – 10} 6s².
2. Thus, atoms of third series elements possess filled 4f-orbitals.
3. 4f-orbitals due to their diffused shape, exhibit poor shielding effect and give rise to lanthanide contraction. Hence the valence electrons experience greater nuclear attraction and greater amount of energy is required to ionise the elements of third transition series namely (H_f to Au).
4. Therefore the ionisation enthalpies of third transition series elements are much higher than those of the first and second transition series.

Question 28.
Explain the metalic character of transition metals.
Answer:

• All the transition elements are metals.
• They are hard, lustrous, malleable, ductile and they have high tensile strength.
• They have high melting points and boiling points.
• Their metallic character is due to vacant or partially filled (n – 1) d-orbitals, and they involve both metallic and covalent bonding.
• Since the strength of metallic bonds depends upon the number of unpaired electrons, it increases up to middle i.e., up to (n – 1 )d⁵, hence accordingly melting points and boiling points also increase.
• After (n – l)d⁵ configuration, the electrons start pairing, hence the metallic strength, melting points and boiling points decrease with the increase in atomic number.

Question 29.
How does metallic character vary in 3d transition elements?
Answer:

1. In 3d-series elements as atomic number increases from scandium (Sc [Ar]¹⁸ 3d¹ 4s²) the number of unpaired electrons increases up to 3d⁵ in chromium.
2. As the number of unpaired electrons increases, the metallic character increases, hence the melting points and boiling points increase from ₂₁Sc(3d¹) to ₂₄Cr (3d⁵).
3. After chromium the number of unpaired electrons goes on decreasing due to the pairing of electrons, hence metallic character, melting points and boiling points decrease from ₂₅Mn to ₂₉Cu.
4. Zinc has all electrons paired, hence it is soft, has a low melting and boiling points.

Question 30.
Which are the common arrangement of the atoms in the structure of transition metals?
Answer:
Most of the transition metals have simple hexagonal closed packed (hep), cubic closed packed (ccp) or body centred cubic (bcc) lattices.

Question 31.
Why do the compounds of transition metals exhibit magnetic properties?
Answer:
The compounds of transition metals exhibit magnetic properties due to the presence of unpaired electrons in their atoms or ions.

Question 32.
What is the cause of paramagnetism and ferromagnetism?
Answer:
Paramagnetism and ferromagnetism is due to the presence of unpaired electrons in species.

Question 33.
When does species become diamagnetic?
Answer:
When there is no unpaired electron, i.e. all electron spins are paired, the species become diamagnetic.

Question 34.
How do metals Fe, Co, Ni acquire permanent magnetic moment?
Answer:
The transition metals Fe, Co and Ni are ferromagnetic. When the magnetic field is applied, all the unpaired electrons in these metals (and their compounds) align in the direction of the applied magnetic field. Due to this the magnetic susceptibility is enhanced and these metals can be magnetised, that is, they acquire permanent magnetic properties.

Question 35.
In which oxidation state, is vanadium diamagnetic?
Answer:

• The electronic configuration of vanadium is, ₂₃V [Ar] 3d³ 4s².
• In +5 oxidation state, the electronic configuration is, V⁵⁺ [Ar].
• Since in V⁵⁺ state, vanadium has all electrons paired, it is diamagnetic.

Question 36.
How is a magnetic moment expressed?
Answer:
The magnetic moment is expressed in Bohr magneton (B.M.). It is denoted by μ.

Question 37.
What is Bohr magneton (B.M.)?
Answer:
Bohr magneton (B.M.) is a unit of magnetic moment :
\(1 \mathrm{~B} . \mathrm{M} .=\frac{e h}{4 \pi m_{\mathrm{e}} c}\)
where, h : Planck’s constant (h = 6.626 x 10^-34 Js)
e : electronic charge (1.60218 x 10^-19 C)
me : mass of an electron (9.109 x 10^-31 kg)
c : velocity of light. (2.998 x 10⁸ ms^-1)

Question 38.
Explain the magnetic properties of transition (or d-block) elements.
Answer:

• Most of the transition metal ions and their compounds are paramagnetic in nature due to the presence of one or more unpaired electrons in their (n – 1)d-orbitals. Hence they are attracted in the magnetic field.
• As the number of unpaired electrons increases from 1 to 5 in J-orbitals, the paramagnetic character and magnetic moment increase.
• The transition elements or their ions having all electrons paired are diamagnetic and they are repelled in the magnetic field.
• Metals like Fe, Co and Ni possess very high paramagnetism and acquire permanent magnetic moment hence they are ferromagnetic.

Question 39.
Explain the effective magnetic moment of the species.
Answer:

• The magnetic moment in the species arises due to the presence of unpaired electrons.
• The magnetic moment depends upon the sum of orbitals and spin contribution for each unpaired electron present in the species.
• In transition metal ions, the contribution of orbital magnetic moment is suppressed by the electrostatic field of other atoms, molecules or ions surrounding the metal ion in the compound.
• Hence the net or effective magnetic moment arises mainly due to spin of electrons. The effective magnetic moment μ_eff, of a paramagnetic substance is given by 1 spin only’ formula represented as, \(\mu=\sqrt{n(n+2)}\) B.M. where n is the number of unpaired electrons.

Question 40.
What is the importance of magnetic moment (μ)?
Answer:

• From the measurements of the magnetic moment (μ) of the species or metal complexes of the first row of transition elements, the number of unpaired electrons can be calculated with the spin-only formula.
• As magnetic moment is directly related to the number of unpaired electrons, value of μ will vary directly with the number of unpaired electrons.
• In 2nd and 3rd transition series, orbital angular moment is significant. Hence spin-only formula for the complexes of 2nd and 3rd transition series is not useful.

Question 41.
Calculate the magnetic moment of the following species :
(1) Cr³⁺
(2) Co
(3) Co³⁺
(4) Cu^{2 +}.
Answer:

Question 42.
Explain : A slight difference in the calculated and observed values of magnetic moments.
Answer:
Magnetic moments are determined experimentally in solution or in solid state where the central atom or ion is hydrated or bound to ligands. Hence a slight difference is observed in calculated and experimentally obtained values of magnetic moment (μ).

Question 43.
Calculate the magnetic moment of a divalent ion in an aqueous solution, if its atomic number is 24.
Answer:
(1) The electronic configuration of divalent inri M²⁺ having atomic number 24 is.

The ion has number of unpaired electrons. n = 4.
By spin only’ formula, the magnetic μ is given by, \(\mu=\sqrt{n(n+2)}=\sqrt{4(4+2)}=4.90 \mathrm{~B} . \mathrm{M}\)
(This M²⁺ ion is Cr²⁺ ion)

Question 44.
When does a substance appear coloured?
Answer:
A substance appears coloured when it absorbs a portion of visible light. The colour depends upon the wavelength of absorption in the visible region of electromagnetic radiation.

Question 45.
Why do the d-block elements form coloured compounds?
Answer:

• Compounds (or ions) of many d-block elements or transition metals are coloured.
• This is due to the presence of one or more unpaired electrons in (n – 1) d-orbital. The transition metals have incompletely filled (n – 1) cf-orbitals.
• The energy required to promote one or more electrons within the d-orbitals involving d-d transitions is very low.
• The energy changes for d-d transitions lie in visible region of electromagnetic radiation.
• Therefore transition metal ions absorb the radiation in the visible region and appear coloured.
• Colour of ions of d-block elements depends on the number of unpaired electrons in (n – 1) d-orbital. The ions having equal number of unpaired electrons have similar colour.
• The colour of metal ions is complementary to the colour of the radiation absorbed.

Question 46.
How is complementary colour of a compound identified?
Answer:

1. The transition metal ions absorb the radiation in the visible region and appeared coloured.
2. Metal ion absorbs radiation of certain wavelength from the visible region. Remaining light is transmitted and the observed colour corresponds to the complementary colour of the light observed.
3. The complementary colour can be identified (with the diagram given).

For example if red colour is absorbed then transmitted complementary colour is green.

Question 47.
Write outer electronic configuration (d-orbital) and colour of 3d-series of transition metal ions.
Answer:
Colour of 3d-transition metal ions

Question 48.
Mention the factors on which the colour of a transition metal ion depends.
Answer:
The factors on which the colour of transition metal ion depends are as follows :

• The presence of incompletely filled d-orbitals in metal ions. (The compounds with the configuration d° and d’0 are colourless.)
• The presence of unpaired electrons in d-orbitals.
• d → d transitions of electrons due to absorption of radiation in the visible region.
• Nature of groups (anions) (or ligands) linked to the metal ion in the compound or a complex.
• Type of hybridisation in metal ion in the complex.
• Geometry of the complex of the metal ion.

Question 49.
Give reasons : Zinc salts are colourless.
Answer:

• Colour of the ions of d-block elements depends on the number of unpaired electrons in (n – 1) d-orbitals.
• Zinc forms salts of Zn²⁺ ions.
• The electronic configuration of Zn⁺² is [Ar] 3d¹⁰.
• Since Zn⁺² does not have unpaired electrons in 3d-orbital, d→d transition cannot take place, hence, Zn⁺² ions form colourless salts.

Question 50.
Explain : The compounds of Cu(II) are coloured.
Answer:

• The electronic configuration of ₂₉Cu [Ar] 3d¹⁰ 4s¹ and Cu²⁺ [Ar] 3d⁹.
• In copper compounds Cu²⁺ ions have incompletely filled 3d-orbital (3d⁹).
• Due to the presence of one unpaired electron in 3 d-orbital, Cu²⁺ ions absorb red light from visible spectrum and emit blue radiation due to d → d transition. Therefore, copper compounds are coloured.

Question 51.
Explain why the solution of Ti³⁺ salt is purple in colour.
OR
Why is Ti³⁺ coloured? (atomic number Ti = 22)
Answer:

• Ti²⁺ ions in the aqueous solution exist in the hydrated complex form as [Ti(H₂O)₆]²⁺.
• The electronic configuration of Ti is, ₂₂Ti [Ar]¹⁸ 3d² 4s² and Ti³⁺ [Ar]¹⁸ 3d¹. Hence in complex, Ti³⁺ has one unpaired electron in 3d subshell.
• Initially, the 3d electron occupies lower energy d-orbital (in t_2g-orbitals).
• On the absorption of radiations of about 500 nm in yellow green region by a complex, 3d¹ electron is excited to the higher energy d-orbital (e_g-orbitals).
• When the electron returns back to the lower energy d-orbital (t_2g), it transmits radiation of complementary colour i.e. red blue or purple colour. Hence, the solution of hydrated Ti³⁺ is purple.

Question 52.
What will be the colour of Cd²⁺ salts? Explain.
Answer:

• The electronic configuration of, ₄₈Cd [Kr]³⁶ 3d¹⁰ 5s² and Cd²⁺ [Kr]³⁶ 3d¹⁰.
• Cd²⁺ ions have completely filled 3d subshell and there are no unpaired electrons in 3d-orbital.
• Hence d → d transition is not possible.
• Therefore, Cd²⁺ ions do not absorb radiations in the visible region and the salts of Cd²⁺ ions are colourless (or white).

Question 53.
Indicate which of the ions may be coloured- V³⁺, Sc³⁺, Cr³¹, Cu²⁺, Ti³⁺, Cu⁺
Answer:

• V³⁺ [Ar]¹⁸ 3d²-((green)
  Since there are two unpaired electrons available, for d → d transition, it will show a Green colour.
• Sc³⁺ [Ar]¹⁸ 3d° (colourless/white).
  Since there are no unpaired electrons in the 3d subshell, it will not show colour.
• Cr³⁺ [Ar]¹⁸ 3d³ – (violet)
  There are three unpaired electrons in the 3d subshell, hence due to d → d transition, it will show violet colour.
• Cu²⁺ [Ar]¹⁸ 3d⁹ (blue)
  It has one unpaired electron that can undergo a d → d transition, hence it will show the colour blue.
• Ti³⁺ [Ar]¹⁸ 3d¹ (purple)
  It has one unpaired electron that can undergo a d → d transition, hence it will show the colour purple.
• Cu¹⁺ [Ar]¹⁸ 3d¹⁰ (colourless)
  There are no unpaired electrons in the 3d subshell, hence it will not show colour.

Question 54.
Explain why is cobalt chloride pink in colour when dissolved in water but turns deep blue when treated with concentrated hydrochloric acid.
Answer:

• The electronic configuration of ₂₇Co : [Ar] 3d¹4s² and Co²⁺ [Ar] 3d¹.
• When dissolved in water cobalt chloride, Co²⁺ forms pink complex, [Co(H₂O)₆]²⁺.
• The complex has octahedral geometry.
• Due to absorption of radiation in the visible region and d – d transition, it forms pink coloured solution.
• When CoCl₂ solution is treated with concentrated HCl solution it turns deep blue.
• This change is due to the formation of another complex, [CoC1₄]²⁺ which has a tetrahedral geometry.
• Thus due to a change in geometry of the complex formed the colour of the solution changes from pink to deep blue.

Question 55.
Explain the catalytic properties of the rf-block or transition metals.
Answer:

• d-block elements or transition metals and their compounds or complexes influence the rate of a chemical reaction and hence act as catalysts.
• In homogeneous catalysis a catalyst forms an unstable intermediate compound which decomposes into products and regenerates the catalyst. But transition metals involve heterogeneous catalysis.
• The transition metals have incompletely filled d-subshells which adsorb reactants on the surface and provide a large surface area for the reactants to react.
• Since transition metals have variable oxidation states they are very good catalysts.
• Hence, compounds of Fe, Co, Ni, Pt, Pd, Cr etc are used as catalysts in many reactions.

Question 56.
Explain the use of different transition metals as catalysts.
Answer:
The transition metals are very good catalysts.

• MnO₂ is used as a catalyst in the decomposition of KClO₃.
  
• In the manufacture of ammonia by Haber’s process, Mo/Fe is used as a catalyst.
  
• In the synthesis of gasoline by Fischer Tropsch process, Co-Th alloy is used as a catalyst.
• Finely divided Ni (formed by reduction of heated oxide in hydrogen) is very efficient catalyst in hydrogenation of ethene to ethane at 140 °C.
  
• Commercially, hydrogenation with Ninkel as catalyst is used to convert inedible oils into solid fat for the production of margarine.
• In the contact process of industrial production of sulphuric acid, sulphur dioxide and oxygen (from air) react reversibly over a solid catalyst of platinised asbestos.
  
• Carbon dioxide and hydrogen are formed by the reaction of carbon monoxide and steam at 500 °C with Fe-Cr catalyst.
  

Question 57.
What are interstitial compounds of transition metals?
Answer:

• The interstitial compounds of the transition metals are those which are formed when small atoms like H, C or N are trapped inside the interstitial vacant spaces in the crystal lattices of the metals.
• Sometimes, sulphides and oxides are also trapped in the crystal lattices of transition elements.
• Presence of these elements in the crystal lattices of metals provide new properties to the metals.

Question 53.
Give one example of an interstitial compound.
Answer:
Steel and cast iron are examples of interstitial compounds of carbon and iron.

Question 54.
Give examples of interstitial compounds where the property of the transition metal is changed.
Answer:
Steel and cast iron are interstitial compounds of carbon and iron (carbides of iron). Due to the presence of carbon, the malleability and ductility of iron is reduced while its tenacity increases.

Question 55.
What are the properties of the interstitial compounds of transition metals?
Answer:

• The chemical properties of the interstitial compounds are the same as that of parent transition metals.
• They are hard and show the metallic properties like electrical and thermal conductivity, lustre, etc.
• Since metal-non-metal bonds in the interstitial compounds are stronger than metal-metal bonds in pure metals, the compounds have very high melting points, higher than the pure metals.
• They have lower densities than the parent metal.
• The interstitial compounds containing hydrogen (i.e., hydrides of metals) are powerful reducing agents.
• The compounds containing carbon, hence behaving as carbides, are chemically inert and extremely hard like diamond.
• In these compounds, malleability and ductility are changed. For example steel and cast iron.

Question 56.
What are interstitial compounds? Why do these compounds have higher melting points than corresponding pure metals?
Answer:

1. The interstitial compounds of the transition metals are those which are formed when small atoms like H, C or N are trapped inside the interstitial vacant spaces in the crystal lattice of the metals.
2. Since metal-nonmetal bonds in the interstitial compounds are stronger than metal-metal bonds in pure metals, the compounds have very high melting points, higher than the pure metals.

Question 57.
Explain the formation of alloys of transition metals.
Answer:

• The transition metals form a large number of alloys among themselves, which are hard with high melting points.
• During alloy formation atoms of one metal are distributed randomly in the lattice of another metal.
• The metals with similar atomic radii and similar properties readily form alloys.
• These alloys have industrial importance.
• The alloys can be ferrous alloys or nonferrous alloys.

Question 58.
How are the transition metal alloys classIfied?
Answer:
The transition metal alloys are classified into

• Ferrous alloys
• Nonferrous alloys.

Question 59.
Explain what are
(1) ferrous alloys and
(2) nonferrous alloys.
Answer:

1. Ferrous alloys: In ferrous alloys, atoms of other elemems are distributed randomly in atoms of iron in the mixture. As the percentage of iron is more in these alloys, they are termed as ferrous alloys. For expamle : nickel steel, chromium steel, stainless steel, (All steels have abot 2% carbon)
2. onferrous alloys : These are formed by mixing atoms of transition metal other than iron with a non transition elemeni. For example, brass is an alloy of Cu and Zn. Bronze is an alloy of Cu and Sn.

Question 60.
What are the uses of alloys?
Answer:

Name of alloy	Important use in industry
(1) Bronze (Cu + Sn)	In making statues, medals and trophies (as it is tough, strong and corrosion-resistant)
(2) Cupra-nickel (Cu + Ni)	In making machinery parts of marine ships, boats, marine conden­ser tubes.
(3) Stainless steel	In the construction of the outer fuselage of ultra-high-speed aircraft.
(4) Nichrome : (Ni+ Cr in the ration 80 : 20)	For gas turbine engines.
(5) Titanium alloys	For ultra-high-speed flight, fireproof bulkheads and exhaust shrouds (as they withstand high temperatures).

Question 61.
Write the preparation of potassium permanganate.
Answer:
Potassium permanganate (KMnO₄) is prepared in the following steps,

(1) Chemical Oxidation : When finely divided manganese dioxide (Mn0₂) is heated strongly with fused caustic potash (KOH) and an oxidising agent potassium chlorate (KCIO₃), dark green potassium manganate (K₂MnO₄) is obtained. (In neutral or acidic medium K₂MnO₄ disproportionates.)

The liquid is filtered through glass wool or sintered glass and evaporated. Potassium manganate crystallises as small, blackish crystals.

(2) Oxidation of K₂MnO₄ by
(i) Electrolytic oxidation : An alkaline solution of manganate ion is electrolysed between iron electrodes separated by a diaphragm. Manganate ion \(\left(\mathrm{MnO}_{4}^{2-}\right)\) undergoes oxidation at anode forming permanganate ion \(\left(\mathrm{MnO}_{4}^{-}\right)\). Oxygen evolved at anode converts \(\left(\mathrm{MnO}_{4}^{2-}\right)\) to \(\left(\mathrm{MnO}_{4}^{-}\right)\).

The overall reaction is as follows :
2K₂MnO₄ + H₂O + [O] → 2KMnO₄ + 2KOH

The electrolytic solution is filtered and evaporated to obtain deep purple black crystals of KMn0₄.

(ii) By passing CO₂ through the solution of K₂MnO₄ :
3K₂MnO₄ + 4CO₂ + 2H₂O → 2KMnO₄ + MnO₂ + 4 KHCO₃

Question 62.
What is meant by the disproportionation of an oxidation state? Explain giving example of manganese.
Answer:

1. Disproportionation reaction is a chemical reaction in which atom or an ion of an element forms two or more species having different oxidation states, one lower and one higher.
2. Manganese (Mn) shows different oxidation states + 2 to +7.
3. When one oxidation state, lower or higher oxidation state becomes unstable as compared to another oxidation state, it undergoes disproportionation reaction.
4. For example, + 6 oxidation state of Mn is less stable than + 7 and + 4.
   • Hence, in acidic medium \(\mathrm{Mn}^{6+} \text { in } \mathrm{MnO}_{4}^{2-}\) undergoes disproportionation reaction.
     
   • In neutral medium green K₂MnO₄ disproportionates to KMn04 and MnO₂.
     

Question 63.
Give examples of oxidising reactions of KMnO₄.
Answer:
(1) KMnO₄ in acidic medium :

(2) KMnO₄ in neutral or alkaline medium in neutral or weakly alkaline medium :
(i) Iodide is oxidised to iodate ion.

(ii) Thiosulphate ion is oxidised to sulphate ion.

(iii) Manganous salt is oxidised to MnO₂.

Question 64.
Balance the following equations :
KI + KMnO₄ + H₂SO₄ → K₂SO₄ + MnSO₄ + 8H₂O + I₂
H₂S + KMnO₄ + H₂SO₄ → K₂SO₄ + MnSO₄ + H₂O + S.
Answer:
10 KI + 2KMnO₄ + 8H₂SO_{4 →} 6K₂SO₄ + 2MnSO₄ + 8H₂O + 5I₂
5H₂S + 2KMnO₄ + 3H₂SO₄ → K₂SO₄ + 2MnSO₄ + 8H₂O + 5S.

Question 65.
Give the uses of potassium permanganate.
Answer:
Uses of potassium permanganate :

• as an antiseptic.
• as a powerful oxidising agent in laboratory and industry.
• in the detection of unsaturation in organic compounds in the laboratory. (Baeyer’s reagent, alkaline KMnO₄).
• for detecting halides in qualitative analysis.
• in volumetric analysis for the estimation of H₂O₂, FeSO₄ etc.)

Question 66.
Write the formula of chromite ore.
Answer:
FeOCr₂O₃.

Question 67.
How is potassium dichromate manufactured from chromite ore (FeOCr₂O₃)?
Answer:
Manufacture of potassium dichromate (K₂Cr₂O₂) from chrome iron ore (FeOCr₂O₃) involves following steps :
(1) Concentration of ore : The chromite ore (FeOCr₂O₃) is powdered and washed with current of water.
(2) Conversion of chromite ore into sodium chromate : The concentrated ore is mixed with anhydrous sodium carbonate (Na₂CO₃) and a flux of lime in excess air and heated in a reverberatory furnace.

Sodium chromate (Na₂CrO₄) formed in the reaction is then extracted with water so that Na₂CrO₄ dissolves into solution and insoluble substances separate out.
(3) Conversion of Na2CrO₄ into sodium dichromate (Na₂Cr₄O₇) : Na₂CrO₄ solution is acidified with concentrated H₂SO₂, so that sodium chromate is converted into sodium dichromate.

Less soluble sodium sulphate crystallises out as Na₂SO₄.10H₂O. which is filtered off.
(4) Conversion of Na₂Cr₂O₇ into K₂Cr₂O₇ : Concentrated solution of Na₂Cr₂O₇ is treated with KCl on by double decomposition, K₂Cr₂O₇ is obtained.
Na₂Cr₂O₇ + 2KCl → K₂Cr₂O₇ + 2NaCl
On concentrating and cooling the solution, less soluble orange coloured K₂Cr₂O₇ crystallises out which is filtered and purified by recrystallisation.

Question 68.
What happens when hydrogen sulphide gas (H₂S) is passed through acidified K₂Cr₂O₇ solution?
Answer:
When hydrogen sulphide (H₂S) gas is passed into solution of K₂Cr₂O₇, H₂S is oxidised to a pale yellow solid (precipitate) of sulphur. Orange coloured solution becomes green due to formation of chromic sulphate (green coloured).

In the reaction, H₂S is oxidised to S and K₂Cr₂O₇ is reduced to Cr₂(SO₄)₃.

Question 69.
What are the common physical properties of d-block elements?
Answer:
The common physical properties of d-block elements are :

• All d-block elements are lustrous and shining.
• They are hard and have high density
• They have high melting and boiling points.
• They are good electrical and thermal conductors.
• They have high tensile strength and malleability.
• They can form alloys with transition and nontransition elements
• Many metals and their compounds are paramagnetic.
• Most of the metals are efficient catalysts.

Question 70.
What are the common chemical properties of d-block elements?
Answer:
The common chemical properties of the d-block elements are :

• All d-block elements are electropositive metals.
• They exhibit variable oxidation states and form coloured salts and complexes.
• They are good reducing agents.
• They form insoluble oxides and hydroxides.
• Iron, cobalt, copper, molybdenum and zinc are biologically important metals.
• They catalyse biological reactions.

Question 71.
Give examples to show that elements of first row of d-block elements differ from second and third row with respect to the stabilisation of higher oxidation states.
Answer:

• Highest oxidation state for the first row element is + 7 as in Mn.
  For the second row, the highest oxidation state is + 8 as in Ru (RuO₄).
  For the third row, the highest oxidation state is + 8 as in Os (OsO₄).
• Compounds of Mo(V) of 2nd row and W(VI) of 3rd row of transitional elements are more stable than Cr(VI) and Mn (VIII) of first row elements.

Question 72.
How do metals occur in nature?
Answer:
In nature, few metals occur in earth’s crust in free state or native state while other metals occur in the combined form.
(1) Elements in free state or native state : The metals which are non-reactive with air, water, CO₂ and non-metals occur in free state or native state. For example, gold, platinum, palladium occur in free state. Metals like Cu, Ag and Hg occur partly in the free state.

(2) Combined form : The metals which are reactive occur in the combined state with other elements forming compounds like oxides, sulphides, sulphates, carbonates, silicates, etc.

Question 73.
What are minerals?
Answer:
Minerals : They are naturally occurring chemical substances in the earth’s crust containing metal in free state or in combined form and obtainable from mining are called minerals. For example, haematite Fe203, galena PbS, etc.

Question 74.
What are ores?
Answer:
Ores : The minerals containing a high percentage of metals from which metals can be profitably extracted are called ores.
[Note : Every ore is a mineral but every mineral is not an ore.]

Question 75.
Write names of minerals and ores of Iron, Copper and Zinc.
Answer:

Metals	Mineral	Ore
Iron	Haematite Fe2O3 Magnetite Fe3O4 Limonite 2Fe2O3, 3H2O Iron pyrites FeS2 Siderite FeCO3	Haematite
Copper	Chalcopyrite CuFeS2 Chalcocite Cuprite Cu2O	Chalcopyrite Chalcocite
Zinc	Zinc blende ZnS Zincite ZnO Calamine ZnCO3	Zinc blende

Question 76.
What is metallurgy?
Answer:
Metallurgy : The process of extraction of metal in a pure state from its ore is called metallurgy.

Question 77.
Define the following:
(1) Pyrometallurgy
(2) Hydrometallurgy
(3) Electrometallurgy.
Answer:

1. Pyrometallurgy : It is a process of extraction of metal from metal oxide from concentrated ore by reduction with a suitable reducing agent like carbon, hydrogen, aluminium, etc. at high temperature.
2. Hydrometallurgy : It is a process of extraction of metals by converting their ores into aqueous solutions of metal compounds and reducing them by suitable reducing agents.
3. Electrometallurgy : It is a process of extraction of highly electropositive metals like Na, K, Al, etc. by electrolysis of fused compounds of the metals where metal ions are reduced at cathode forming metals.

Question 78.
What is gangue?
Answer:
Gangue : The earthly and undesired impurities of various substances like sand (SiO₂), metal oxides, etc. present in the ore are called gangue or matrix.

Question 79.
Define concentration of an ore.
Answer:
Concentration : A process of removal of gangue or unwanted impurities from the ore is called concentration of an ore. It is also called benefaction or dressing of an ore.

Question 80.
What are common methods of concentration of an ore?
Answer:
The concentration of an ore involves different methods depending upon the differences in physical properties of compounds or the metal present and the nature of the gangue.

The common methods of concentration of ore are as follows :

1. Gravity separation or hydraulic washing :
   This can be carried out by two processes as follows :
   • Hydraulic washing by using Wilfley’s table method
   • Hydraulic classifier methods.
2. Magnetic separation
3. Froth floatation process.
4. Leaching.

The method depends upon the nature of ore.

Question 81.
What is leaching?
Answer:
Leaching : ft is a (chemical) process used in the concentration of an ore by extracting soluble material from an insoluble solid by dissolving in a suitable solvent. This method is used in the concentration process of ores of Al, Ag, Au, etc.

Question 82.
What is roasting of an ore?
Answer:
Roasting : It is a process of strongly heating a concentrated ore in the excess of air below melting point of metal, to convert it into oxide form. It is used for a sulphide ore. For example, ZnS ore on roasting forms ZnO.

Question 83.
Write an equation to show how zinc blende (ZnS) is converted to ZnO.
Answer:
When zinc blende is roasted, it is converted to ZnO.
\(\mathrm{ZnS}+\mathrm{O}_{2} \stackrel{\Delta}{\longrightarrow} \mathrm{ZnO}+\mathrm{SO}_{2}\)

Question 84.
Explain the term : Smelting
Answer:
Smelting : The process of extraction of a metal from its ore by heating and melting at high temperature is called smelting. Reduction of ore is carried out during smelting.

Question 85.
What is calcination?
Answer:
Calcination is a process in which the ore is heated to a high temperature below the melting point of the metal in the absence of air or limited supply of air in a reverberatory furnace.

It is generally used for carbonate and hydrated oxides to convert them into anhydrous oxides.

Question 86.
Define the terms :
(1) Flux
(2) Slag
Answer:
(1) Flux : A flux is a chemical substance which is added to the concentrated ore during smelting in order to remove the gangue or impurities by chemical reaction forming a fusible mass called slag.
(2) Slag : It is a waste product formed by combination of a flux and gangue (or impurities) during the extraction of metals by smelting process.

Iron is the fourth most abundant element in the earth’s crust.

Question 87.
What is the composition of haematite ore?
Answer:
Composition of Haematite ore is Fe2O₃ + SiO₂ + Al₂O₃ + phosphates

Question 88.
Which impurities (gangue) are present in haematite ore?
Answer:
SiO₂ and Al₂O₃ are the impurities present in the haematite ore.

Question 89.
Which reducing agents are used to reduce haematite ore into metallic iron?
Answer:
Haematite ore is reduced using coke and CO. Carbon in the coke is converted to carbon monoxide. Carbon and carbon monoxide together reduce Fe203 to metallic iron.

Fe₂O₃ + 3C → 2Fe + 3CO.
Fe₂O₃ + 3CO → 2Fe + 3CO₂.

Question 90.
Why is limestone used in the extraction of iron?
Answer:

• The ore of iron contains acidic gangue or impurity of silica, SiO₂.
• To remove silica gangue, basic flux like calcium oxide CaO, is required, which is obtained from the decomposition of limestone, CaCO₃. \(\mathrm{CaCO}_{3} \stackrel{\Delta}{\longrightarrow} \mathrm{CaO}+\mathrm{CO}_{2}\)
• Silica reacts with CaO and forms a fusible slag of CaSiO₃.
  \(\mathrm{SiO}_{2}+\mathrm{CaO} \stackrel{\Delta}{\longrightarrow} \mathrm{CaSiO}_{3}\)

Therefore in the extraction of iron, lime is used.

Question 91.
Name the furnace in which iron is extracted from Haematite ore.
Answer:
Extraction of iron is carried out in Blast furnace.

Question 92.
Explain the extraction of iron from haematite.
Answer:
Iron is mainly extracted from haematite, Fe₂O₃ by reduction process.
Haematite ore contains silica (SiO₂), alumina (Al₂O₃) and phosphates as impurity or gangue.

Coke is used for the reduction of ore.

To remove acidic gangue SiO₂, a basic flux CaO is used which is obtained from lime stone CaCO₃.

The extraction process involves following steps :
(1) Concentration of an ore : The powdered ore is concentrated by gravity separation process by washing it in a current of water. The lighter impurities (gangue) are carried away leaving behind the ore.
(2) Roasting : The concentrated ore is heated strongly in a limited current of air. During this, moisture is removed and the impurities like S, As and phosphorus are oxidised to gaseous oxides which escape.

After roasting, the ore is sintered to form small lumps.
(3) Reduction (or smelting) : The roasted or calcined ore is then reduced by heating in a blast furnace.

The blast furnace is a tall cylindrical steel tower about 25 m in height and has a diameter about 5-10m lined with fire bricks inside.

Blast furnace has three parts :

• the hearth,
• the bosh and
• the stack.

At the top, there is a cup and cone arrangement to introduce the ore and at the bottom, tapping hole for withdrawing molten iron and an outlet to remove a slag.

The roasted ore is mixed with coke and limestone in the approximate ratio of 12 : 5 : 3.

A blast of hot air at about 1000 K is blown from downwards to upwards by layers arrangement. The temperature range is from bottom 2000 K to 500 K at the top. The charge of ore from top and the air blast from bottom are sent simultaneously. There are three zones of temperature in which three main chemical reactions take place.

(i) Zone of combustion : The hot air oxidises coke to CO which is an exothermic reaction, due to which the temperature of furnace rises.
C + ^1/2 O₂ → CO ΔH= – 220kJ
Some part of CO dissociates to give finely divided carbon and O₂.
2CO → 2C + O₂
The hot gases with CO rise up in the furnace and heats the charge coming down. CO acts as a fuel as well as a reducing agent.

(ii) Zone of reduction : At about 900 °C, CO reduces Fe₂O₃ to spongy (or porous) iron.
Fe₂O₃ + 3CO → 2Fe + 3CO₂
Carbon also reduces partially Fe₂0₃ to Fe.
Fe₂O₃ + 3C → 2Fe + 3CO

(iii) Zone of slag formation : At 1200 K limestone, CaCO₃ in the charge, decomposes and forms a basic flux CaO which further reacts at 1500 K with gangue (SiO₂, Al₂O₃) and forms a slag of CaSiO₃ and Ca₃AlO₃.
CaCO₃ + CaO + CO₂.
CaO + SiO₂ → CaSiO₃
12CaO + 2Al₂O₃ → 4Ca₃AlO₃ + 3O₂

The slag is removed from the bottom of the furnace through an outlet.

(iv) Zone of fusion : The impurities in ore like MnO₂ and Ca₃(PO₄)₂ are reduced to Mn and P while SiO₂ is reduced in Si. The spongy iron moving down in the furnace melts in the fusion zone and dissolves the impurities like C, Si, Mn, phosphorus and sulphure. The molten iron collects at the bottom of furnace. The lighter slag floats on the molten iron and prevents its oxidation.

The molten iron is removed and cooled in moulds. It is called pig iron or cast iron. It contains about 4% carbon.

Question 93.
Write the reaction involved in the zone of reduction in blast furnace during extraction of iron.
Answer:
Zone of reduction : At about 900 °C, CO reduces Fe₂O₃ to spongy (or porous) iron.
Fe₂O₃ + 3CO → 2Fe + 3CO₂
Carbon also reduces Fe₂O₃ to Fe.
Fe₂O₃ + 3C → 2Fe + 3CO

Question 94
Write reactions involved at different temperatures in the blast furnace.
Answer:

Temperature K	Change taking place in the blast furnace	Reactions
1. 500 K	Haematite ore loses moisture	ore xH2O → ore
2. 900 K	Reduction of ore by CO	Fe2O3 + 3CO → 2Fe + 3CO
3. 1200K	Limestone decomposes	CaCO3 → CaO + CO2
4. 1500K	Reduction of ore by C	Fe2O3 + 3C → 2Fe + 3CO
5. 1600 K	(i) Reduction of FeO by C (ii) Fusion of iron and slag formation	FeO + C → Fe + CO CaO + SiO2 → CaSiO3
6. 2000 K	Combustion of coke	2C + O2 → CO

Question 95.
What is the action of carbon on Fe203 in blast furnace?
Answer:
Fe₂O₃ + 3C → 2Fe + 3CO

Question 96.
What is refining of metals?
Answer:
Refining of metals : The purification of impure or crude metals by removing metallic and nonmetallic impurities is known as refining of metals. H

Question 97.
How is pure iron obtained from crude iron?
Answer:
Pure iron can be obtained by electrolytic refining.

Question 98.
Name the methods of refining of metals.
Answer:
Methods of refining of metals :

• Electrorefining
• Liquefaction
• Distillation
• Oxidation m

Question 99.
What are the factors that govern the choice of extraction technique of metals?
Answer:
The choice of extraction technique is governed by the following factors.

• Nature of ore
• Availability and cost of reducing agent. (Generally, cheap coke is used).
• Availability of hydraulic power.
• Purity of metal required.
• Value of by-products. For example. SO₂ obtained during the roasting of sulphide ores is important for the manufacture of H₂SO₄.

Question 100.
Which are the commercial forms of iron?
Answer:
Commercial forms of iron are :

• Cast iron
• wrought iron
• steel. H

Question 101.
(A) What are f-block elements?
(B) What are inner transition elements?
Answer:
(A)

• Elements in which differentiating electron enters into the pre-penultimate shell the (n – 2) f-orbital are known as f.block elements.
• They include 28 elements with atomic numbers ranging from 58-71 and atomic numbers 90 to 103 collectively.
• There are two f-series or two f-block elements, namely 4f and 5f series.
• The f-block includes two inner transition series namely the lanthanoid series. Cerium (58) to LuteUum (71) or the 4 f-block elements and the actinoid series. Thorium (90) to I.awrencium (103) or the 5f block elements.

(B) f-block elements are called inner transition elements since f-orbital lies much inside the f-orbital in relation to the transition metals, These elements have 1 to 14 electrons in their f-orbital.

Question 102.
What are fIrst inner transition elements?
Answer:

1. 4f-hlock elements are called (first) inner transition elements and have partly filled inner orbitaIs or (4f) orbitais.
2. They have general outer electronic configuration \((n-2) f^{1-14},(n-1) d^{0-1}, n s^{2}\).
3. There are two f-series, namely 4f and 5f series, called lanthanoids and acùnoids respectively.
4. They shos intermediate properties as compared to electropositive s-block elements and electronegative p-block elements. Hence they are called (first) inner transition elements.

Question 103.
What are lanthanoids (or lanthanides)?
OR
What is the lanthanoid series?
Answer:

• Lanthanoids or Lanthanoid series or Lanthanones : The series of fourteen elements from ₅₈Ce to ₇₁Lu in which a differentiating electron enters 4f sub-shell and follows lanthanum is called lanthanoid series and the elements are called lanthanoids.
• They have general electronic configuration, [Xe] 4f^1-14 ,5d^0-1, 6s².
• They follow Lanthanum (Z = 57) in 3d-series.

Question 104.
What are rare earths?
Answer:

• Lanthanoids or 4f-block elements are called rare earths.
• Lanthanoids are never found in free state, and their minerals are not pure.
• They exhibit similar chemical properties hence cannot be extracted and separated by normal metallurgical processes.
• Lanthanoid metals are available on small scale. Therefore they are called rare earths.

Question 105.
Explain the position of lanthanoids in the periodic table.
OR
How is the position of lanthanoids justified?
Answer:

1. Position of Lanthanoids in the periodic table : Group – 3; Period – 6.
2. They interrupt the third transition series of t/-block elements (i.e. 5 d series) in the sixth period.
3. They are 14 elements from ₅₈Ce to ₇₁Lu and their position is in between La and Hf. Since they follow lanthanum, they are called lanthanoids.
4. They are called 4f-series elements and for the convenience, they are placed separately below the main periodic table.
5. The actual position of lanthanoids is in between Lanthanum (Z = 57) and Hafnium (Z = 72).
6. Their position is justified due to following reasons :
   • All these elements have the same electronic configuration in ultimate and penultimate shells, one electron in 5d-orbital and two electrons in 6s-orbital.
   • Group valence of all lanthanoids is 3.
   • All lanthanoids from ₅₈Ce to ₇₁Lu have similar physical and chemical properties.

Question 106.
Explain the meaning of inner-transition series.
Answer:
A series of f-block elements having electronic configuration (n – 2)f^1-14 (n – I) d^0-1 ns² placed separately in the periodic table represents inner transition series. The f-orbitals lie much inside the e/ orbitals.

Since the last electron enters pre-penultimate shell, these elements are inner transition elements.

There are two inner transition series as follows :
4f-series ₅₈Ce → ₇₁Lu
5f-series ₉₀Th → ₁₀₃Lr

Question 107.
Draw a skeletal diagram of the periodic table to show the position of d and/- block elements.
Answer:

Question 108.
What are the properties of lanthanoids?
Answer:

• Lanthanoids are soft metak with silvery white colour, Colour and brightness reduces on exposure to air.
• They are good conductors of heat and electricity.
• Except promethium (P_m), all are non-radioactive in nature.
• The atomic and ionic radii decrease from La to Lu. (Lanthanoid contraction).
• Coordination numbers arc greater than 6.
• They are paramagnetic.
• They become ferromagnetic at lower temperature.
• Their magnetic and optical properties are independent of environment.
• They are called rare earths as their exiractioli was difficult.
• They are abundant in earth’s crust
• All lanthanoids fonn hydroxides which are ionic and basic. l3asicity decreases with atomic number,
• They react with nitrogen to give nitrides and with halogen to give halides.
  
• When heated with carbon at very high temperature give carbides
  

Question 109.
Explain the variations in ionisation enthalpy of lanthanoids.
Answer:

• The first ionisation enthalpy of lanthanoids is nearly same. It is very high for Gd and Yb.
• The ionisation enthalpy increases from first (IE₁] to third (IE₃).

First, second and third ionization enthalpies of lanthanoids in kj/mol

Lanthanoid	IE1	IE2	IE3
La	538.1	1067	1850.3
Ce	528.0	1047	1949
Pr	523.0	1018	2086
Nd	530.0	1034	2130
Pm	536.0	1052	2150
Sm	543.0	1068	2260
Eu	547.0	1085	2400
Gd	592.0	1170	1990
Tb	564.0	1112	2110
Dy	572.0	1126	2200
Ho	581.0	1139	2200
Er	589.0	1151	2190
Tm	596.7	1163	2284
Yb	603.4	1175	2415
Lu	523.5	1340	2022

Question 110.
Give the general electronic configuration of 4f-series elements (OR lanthanoids).
Answer:

• The general electronic configuration of 4f-series elements is, Ln[Xe]⁵⁴ 4f^1-14 5d^0-1 6s² where Ln is a lanthanoid.
• Xenon has electronic configuration, [Xe] : Is² 2s² 2p⁶ 3s² 3p⁶ 3d¹⁰ 4s² 4p⁶ 4d¹⁰ 5s² 5p⁶.
• In lanthanoids, the differentiating electron enters prepenultimate shell, 4f m

Question 111.
What are the important features of the electronic configuration of lanthanoids?
Answer:

1. Lanthanoids show two types of electronic configurations
   (a) an expected or idealized
   (b) an observed electronic configuration.
   In the idealized electronic configuration, the filling of the 4/-orbitals is regular but in the observed configuration, there is the shift of a single electron from 5d to 4/ sub-shell.
2. Lanthanum (57) has an electronic configuration [Xe] 4f° 5d¹6s². It does not have any f-electron.
3. The next incoming electron does not enter the 5d sub-shell but goes to the 4f sub-shell.
4. 14 electrons are progressively filled in the 4f sub-shell as the atomic number increases by one unit from La to Lu.
5. La, Gd and Lu are the only elements which possess one electron in a 5d orbital, while in all other lanthanoids the 5d sub-shell is empty.
6. La-(4f°), Gd-(4f⁷) and Lu-(4f¹⁴) posses extra stability due to their empty, half-filled and completely filled 4f-orbitals respectively.
7. The 4f-electrons in the prepenultimate shell are shielded by the outermost higher orbitals, 5s², 5p⁶, 5d¹, 6s², i.e. by eleven electrons, hence they are less effective in chemical bonding.

Electronic configuration (Idealised and observed)

[Xe]⁵⁴ ls²2s²2p⁶3s²3p⁶3d¹⁰4s²4p⁶4d¹⁰5s²5p⁶

Question 112.
Write the expected electronic configuration of (a) Nd (Z = 60) (b) Tm (Z = 69).
Answer:
Expected electronic configuration :
(a) Nd = [Xe] 4f³ 5d¹ 6s²
(b) Tm= [Xe] 4f¹⁴5d¹6s²

Question 113.
Write electronic configurations of
(i) Gd
(ii) Yb.
Answer:
(i) ₆₄Gd [Xe] 4f⁷5d¹6s² (Observed)
(ii) ₇₀Yb [Xe] 4f¹⁴5d°6s² (Observed)

Question 114.
Write expected and observed electronic configurations of
(i) Ce
(ii) Tb.
Answer:

Element	Expected (Idealised)	Observed
(i) 58Ce	[Xe] 4f15d16s2	[Xe] 4f25d°6s2
(ii) 65Tb	[Xe] 4f85d16s2	[Xe] 4f95d°6s2

Question 115.
Why are the expected and observed ground state electronic configurations of gadolinium and lawrencium same?
Answer:

• The degenerate orbitals like 4f and 5f acquire extra stability when they are half filled (4f⁷) or completely filled (5f¹⁴).
• The expected and observed electronic configuration of gadolinium is, ₆₄Gd [Xe] 4f⁷ 5d¹ 6s².
• The expected and observed electronic configuration of lawrencium is ₁₀₃Lr [Rn] 5f¹⁴ 6d¹ 7s².

Question 116.
Explain oxidation states of lanthanoids.
Answer:

• The common oxidation state of the Lanthanoids is 3 + due to the loss of 2 electrons from outermost 6s orbital and one electron from the penultimate 5d sub-shell.
• Gd³⁺ and Lu³⁺ show extra stability due to their half-filled and completely filled f-orbitals, Gd³⁺ = [Xe]4f⁷, Lu³⁺ = [Xe]4f¹⁴
• Ce and Tb attain the 4f° and 4f⁷configurations in the 4 + oxidation states. Eu and Yb attain the 4f⁷ and 4f¹⁴ configurations in the 2 + oxidation states. Sm and Tm also show the 2+ oxidation state although their stability can be explained based on thermodynamic factors.
• Some lanthanoids show 2 + and 4 + oxidation states even though they do not have stable electronic configuration of 4f°, 4f⁷ or 4f¹⁴. E.g. Pr⁴⁺ (4f¹), Nd²⁺ (4f⁴), Sm²⁺ (4f⁶), Dy⁴⁺ (4f⁸) etc

Question 117.
Write the. electronic configuration of the following ions :
(1) La^{3 +} ;
(2) Gd³⁺;
(3) Eu³⁺;
(4) Ce³⁺.
Answer:
(1) La^{3 +} = [Xe]
(2) Gd³⁺ = [Xe] 4f⁷
(3) Eu³⁺ = [Xe] 4f⁶
(4) Ce³⁺ = |Xe] 4f¹

Question 118.
Write the electronic configuration of
(1) Nd²⁺
(2) Nd³⁺
(3) Nd⁴⁺.
Answer:
(1) Nd²⁺ [Xe] 4f⁴
(2) Nd³⁺ [Xe] 4f³
(3) Nd⁴⁺ [Xe] 4f²

Question 119.
Among the following lathanoids, which elements show only one oxidation state 3 +? Why? Dy, Gd, Yb, Lu.
Answer:
Gd and Lu show only one oxidation state 3 +, since they acquire electronic configurations with extra stability namely 4f⁷ and 4f¹⁴ respectively.

Question 120.
Write the expected electronic configurations of :
(1) europium (Z = 63),
(2) erbium (Z = 68).
Answer:
(1) Europium (₆₃Eu) [Xe]⁵⁴4f⁶ 5d¹ 6s²
(2) Erbium (₆₈Er) [Xe]⁵⁴4f¹¹ 5d¹ 6s²

Question 121.
Why does lanthanum form La³⁺ ion, while cerium forms Ce⁴⁺ ion? (Atomic number La = 57 and Ce = 58).
Answer:

1. Electronic configuration Lanthanum is La [Xe] 4f° 5d¹ 6s². By losing three electrons, La acquires stable electronic configuration of Xe and forms La³⁺.
2. Electronic configuration of Cerium is Ce [Xe] 4f¹ 5d¹ 6s². By losing four electrons, Ce acquires stable electronic configuration of Xe and forms Ce⁴⁺.

Question 122.
₆₃EU and ₇₀Yb show 2 + oxidation state. Explain.
Answer:
₆₃EU has electronic configuration, [Xe] 4f⁷ 5d°6s². By losing 2 electrons from 6s orbital, it acquires stable configuration and 4f-orbital is half-filled.
₇₀Yb has electronic configuration, [Xe] 4f¹⁴ 5d° 6s². By losing 2 electrons from 6 s orbital, it acquires stable configuration and 4/-orbital is completely filled.
Hence Eu and Yb show 2 + oxidation states.

Question 123.
Display electronic configuration, atomic and ionic radii of lanthanoids.
Answer:
Answers are given in bold.

Electronic configuration and atomic ionic radii of lanthanoids

Question 124.
Explain the trend in atomic and ionic sizes of lanthanoids.
Answer:

• From ₅₇La (187 pm) to first element of 4f-series ₅₈Ce (183 pm), the contraction in atomic radius is very large, 4 pm.
• But from Ce onwards as atomic number increases atomic radius decreases very steadily so that total decrease in atomic radius from Ce to Lu is only 10 pm.
• In case of tripositive ions due to large pull by nucleus, the decrease in ionic radii is slightly more, i.e. 18 pm. For example, Ce³⁺ (103 pm) to Lu³⁺ (85 pm ).
• Hence all lanthanoids have similar properties. Therefore they cannot be separated from each other easily by normal metallurgical methods but require special methods.
  

Question 125.
What is meant by lanthanoid contraction?
Answer:
Lanthanoid contraction : The gradual decrease in atomic and ionic radii of lanthanoids with the increase in atomic number is called lanthanoid contraction.

Question 153.
Explain the causes of the lanthanoid contraction.
Answer:
The causes of the lanthanoid contraction are as follows :

• As the atomic number of lanthanoids or 4f-block elements increases the positive nuclear charge increases and correspondingly electrons are added to the prepenultimate 4f sub-shell.
• The attraction of nucleus on 4 f-electrons increases with the increase in atomic number.
• The outer eleven electrons namely, 5s², 5p⁶, 5d³ and 6s² do not shield inner 4 f-electrons from the nucleus.
• There is imperfect shielding of each 4f-electron from other 4 f-electrons.
• As compared to d sub-shell, the extent of shielding for 4 f-electrons is less.
• Due to these cumulative effects, 4 f-electrons experience greater nuclear attraction and hence valence shell is pulled towards the nucleus to the greater extent decreasing atomic and ionic radii appreciably.
• From 57La to 58Ce, there is a sudden contraction in atomic radius from 187 pm to 183 pm but the further decrease up to the last 4f-element, ₇₁Lu is comparatively low (about 10 pm).

Question 126.
Explain lanthanoid contraction effect with respect to (1) decrease in basicity, (2) ionic radii of post-lanthanoids.
Answer:
The lanthanoid contraction has a definite effect on the properties of lanthanoids as well as on the properties of post-lanthanoid elements.
(1) Decrease in basicity :

• In lanthanoids due to lanthanoid contraction, as the atomic number increases, the size of the lanthanoid atoms and their try positive ions decreases, i.e. from La³⁺ to Lu³⁺.
• As size of the cation decreases, according to Fajan’s rule, the polarizability increases and thus the covalent character of the M-OH bond increases, and ionic character decreases.
• Therefore the basic nature of the hydroxides decreases.
• Basicity and ionic character decrease in the order La(OH)₃ > Ce(OH)₃ > … Lu(OH)₃.

(2) Ionic radii of post-lanthanoids :

• Elements following the lanthanoids in the 6th period (third transition series, i.e. 5d-series) are known as post-lanthanoids.
• Due to lanthanoid contraction the atomic radii (size) of elements which follow lanthanum in the 6th period (3rd transition series – Hf, Ta, W, Re)-are similar to the elements of the 5th period (4d-series Zr, Nb Mo, Tc).
• Due to similarity in their size, post-lanthanoid elements (5d-series) have closely similar properties to the elements of the 2nd transition series (4d-series) which lie immediately above them.
• Pairs of elements namely Zr-Hf(Gr-4), Nb-Ta (Gr-5), Mo-W(Gr-6), Tc-Re (Gr-7) are called chemical twins since they possess almost identical sizes and similar properties.

Question 127.
Why do lanthanoids form coloured compounds?
Answer:

• The colour in lanthanoid ions is due to the presence of unpaired electrons in partially filled 4f sub-shells.
• Due to the absorption of radiations in the visible region there arises the excitations of the unpaired electrons from f-orbital of lower energy to the f-orbital of higher energy-giving f → f transitions.
• The observed colour is complementary to the colour of the light absorbed.
• The colour of try positive ions (M³⁺) depends upon the number of unpaired electrons in f-orbitals. Hence the lanthanoid ions having equal number of unpaired electrons have similar colour.
• The colours of M³⁺ ions of the first seven lanthanoids, La³⁺ to Eu³⁺ are similar to those of seven elements Lu³⁺ to Tb³⁺ in the reverse order.

Question 128.
Explain, why Ce³⁺ ion is colourless.
Answer:

• The electronic configuration of Ce³⁺ is, [Xe] 4f⁷
• Even though there is one unpaired electron in 4f sub-shell, the f → f transition involves very low energy. Hence, Ce³⁺ ion does not absorb radiation in the visible region.

Therefore Ce³⁺ ion is colourless.

Question 129.
Explain why Gd³⁺ is colourless.
Answer:

• Gd³⁺ has electronic configuration, [Xe] 4f⁷
• Due to extra stability of half filled orbital, it does not allow f → f transition, and hence does not absorb radiations in the visible region.

Hence Gd³⁺ is colourless.

Question 130.
The salts of (1) La³⁺ and (2) Lu³⁺ are colourless. Explain.
Answer:
(1) (i) La³⁺ has electronic configuration, [Xe] 4f°
(ii) Since there are no unpaired electrons in 4 f-orbital, f → f transition is not possible. Hence La³⁺ ions do not absorb radiations in visible region, and they are colourless.

(2) (i) LU³⁺ has electronic configuration [Xe] 4 f¹⁴
(ii) Since there are no unpaired electrons in 4f-orbital, f → f transition is not possible. Hence Lu³⁺ ions do not absorb radiations in visible region and they are colourless.

Question 131.
Explain giving examples, the colour of nf electrons is about the same as those having (14-n) electrons.
Answer:
(1) Consider Pr³⁺ and Tm³⁺ ions.
Tm³⁺ (4f¹²) has nf electron 12 electrons.
Pr²⁺ (4f²) has (14 – n) = (14 – 2) = 12 electrons. Both, Tm³⁺ and Pr³⁺ are green.

(2) Consider Nd³⁺ and Er³⁺ ions. Er³⁺ (4f¹¹) has nf electrons 11.
Nd³⁺ (4f³) has (14 – n) is (14 – 3) = 11 electrons. These both ions Er³⁺, Na³⁺ are pink in colour.

Question 132.
Lu³⁺ has observed magnetic moment zero. How many unpaired electrons are present?
Answer:
Since magnetic moment is zero, it has no unpaired electrons.

Question 133.
What are the application of lanthanoids?
Answer:

1. Lanthanoid compounds are used inside the colour television tubes and computer monitor. For example mixed oxide (Eu, Y)₂ O₃ releases an intense red colour when bombarded with high energy electrons.
2. Lanthanoid ions are used as active ions in luminescent materials. (Optoelectronic application)
3. Nd : YAG laser is the most notable application. (Nd : YAG = neodymium doped ytterium aluminium garnet)
4. Erbium doped fibre amplifiers are used in optical fibre communication systems.
5. Lanthanoids are used in cars, superconductors and permanent magnets.

Question 134.
What are actinoids? Give their general electronic configuration.
Answer:

• Actinoids : The series of fourteen elements from ₉₀Th to ₁₀₃Lr which follow actinium (₈₉Ac) and in which differentiating electrons are progressively filled in 5f-orbitals in prepenultimate shell are called actinoids.
• Their general electronic configuration is, [Rn]⁸⁶ 5f^1-14 6d^0-1 7s².

Question 135.
Why are actinoids called inner transition elements?
Answer:

• Actinoids are 5f-series elements in which electrons progressively enter into 5f-orbitals, which are inner orbitals.
• They have electronic configuration [Rn]⁸⁶ 5f^1-14 6d^0-1 7s².
• They show intermediate properties as compared to electropositive 5-block elements and electronegative p-block elements. Hence they are called second inner transition elements.

Question 136.
Explain the position of actinoids in the periodic table.
OR
What is the position of actinoids in the periodic table?
Answer:

• Position of actinoids in the periodic table : Group-3; Period-7.
• They interrupt the fourth transition series (6d series) in the seventh period in the periodic table.
• After Actinium, 89Ac which has electronic configuration [Rn] 6d¹7s², the electrons enter progressively 5f orbital and they have general electronic configuration, [Rn] 5f^{1 – 14} 6d^{0 – 1} 7s².
• They are fourteen elements from ₉₀Th to ₁₀₃Lr and since they follow actinium, they are called actinoids.
• They are called 5f series or second inner transition series elements and for the convenience they are placed separately below the periodic table.

Question 137.
Write idealised and observed electronic configuration of actinoids.
Answer:

Question 138.
Explain the oxidation states of actinoids.
Answer:

• Due to availability of electrons in 5f, 6d and 7s sublevels, lanthanoids show varied oxidation states.
• The most common oxidation state is + 3 due to loss of one electron from 6d and two electrons from 6s-orbitals.
• Ac, Th and Am show + 2 oxidation state.
• Th, Pa, U, Np, Pu, Am and Cm show + 4 oxidation state.
• Np and Pu show the highest oxidation state + 7.
• U, Np, Bk, Cm and Am show stable oxidation state + 4.
• In + 6 oxidation state, due to high charge density the actinoid ions form oxygenated ions, e.g. \(\mathrm{UO}_{2}^{+}, \mathrm{NpO}_{2}^{+},\) etc.

Question 139.
Why do actinoids show variable oxidation states?
Answer:

• The large number of variable oxidation states of actinoids is due to very small energy difference between 5f, 6d and 7s subshells.
• The electronic configuration of actinoids is, [Rn] 5f^1-14 6d^0-1, 7s²
• Due to the loss of three electrons from 6d¹ and 7s², the common oxidation state is + 3, but due to further loss of electrons from 5f subshell, actinoids show higher oxidation states.
• The variable oxidation states are + 2 to + 7.

Electronic configuration of actinoids and their ionic radii in + 3 oxidation state

Question 140.
What is meant by actinoid contraction?
Answer:
Actinoid contraction: The gradual decrease in atomic and ionic radii of actinoids with the increase in atomic number is called actinoid contraction.

Question 141.
The extent of actinoid contraction is greater than lanthanoid contraction. Explain Why?
Answer:

• The electronic configurations of :
  Lanthanoids [Xe] 4f^{1 – 14} 5d^{0 – 1} 6s²
  Actinoids [Rn] 5f^{1 – 14}, 6d^{0 – 1} 7s²
• The mutual screening offered in case of 5f-electrons is less than that in the 4f-electrons.
• Hence, the outer orbitals are pulled to the greater extent by nuclei in actinoids (5f-series) than in lanthanoids (4f-series).
• Therefore, actinoid contraction is greater than lanthanoid contraction.

Question 142.
Describe the important properties of actinoids.
Answer:
Properties of actinoids :

• Actinoids are silvery white ( similar to lanthanoids).
• They are highly reactive radioactive elements.
• Most of these elements are not found in nature. They are radioactive and man made.
• They experience decrease in the atomic and ionic radii from Ac to Lw, known as actinoid contraction.
• The common oxidation state is +3. Elements of the first half of the series exhibit higher oxidation states.

Question 143.
What are the applications of actinoids?
Answer:

• Thorium oxide (ThO₂) with 1% CeO₂ is used as a major source of indoor lighting, as well as for outdoor camping.
• Uranium is used in the nuclear reactors.
• The isotopes of Thorium and Uranium have very long half-life, so that we get very negligible radiation from them: Hence they can be used safely.

Question 144.
What are transuranic elements?
Answer:

• The man-made elements heavier titan Uranium (Z = 92) in the Actinoid señes are called transuranic elements.
• These are synthetically or artificially prepared (man-made) elements starting from Neptunium (Z= 93).
• Transuranic elements arc generally considered to be from Neptunium (Z = 93) to Lawrencium (Z = 103).
• Recently elements from atomic number 104 (Rf) to atomic number 118 (Og) or (Uuo) in 6 d series have also been identified as transuranic elements.
• All transuranic elements are radioactive.

Question 145.
What are post actinoid elements?
Answer:

• Elements from atomic number 104 to 118 are called postactinoid elements.
• The post actinoid elements known so far are transition metals.
• They can be synthesised in the nuclear reactions.
• As they have very short half life period, it is difficult to study their chemistry.
• Ruiherfordium forms a chloride (RfCl₄) similar to zirconium and hafnium in + 4 oxidation state.
• Dubniurn resembles niobium and protactinium.

Question 146.
Name the transuranic elements.
Answer:
Names of transuranic elements

Name	Symbol	Atomic number
Neptunium	Np	93
Plutonium	Pu	94
Americium	Am	95
Curium	Cm	96
Berkelium	Bk	97
Californium	Cf	98
Einsteinium	Es	99
Ferminum	Fm	100
Mendelevium	Md	101
Nobelium	No	102
Lawrencium	Lr	103
Rutherfordium	Rf	104
Dubnium	Db	105
Seaborgium	Sg	106
Bohrium	Bh	107
Hassium	Hs	108
Meitnerium	Mt	109
Darmstadtium	Uun/Ds	110
Roentgenium	Uuu/Rg	111
Copernicium	Uub/Cn	112
Ununtrium	Uut	113
Ununquadium	Uuq	114
Ununpentium	Uup	115
Ununhexium	Uuh	116
Ununseptium	Uus	117
Ununoctium	Uuo	118

In the transuranic elements, elements from atomic number 93 to 103 are actinoids and from atomic number 104 to 118 are called postactinoid elements.

Question 147.
What are the similarities between lanthanides and actinides.
Answer:
Lanthanides and actinides show similarities as follows :

• Both, lanthanides and actinides show+ 3 oxidation state.
• In both the series, the f-orbitals are filled gradually.
• Ionic radius of the elements in both the series decreases with increase in atomic number.
• Electronegativity in both the series is low for all the elements.
• They all are highly reactive.
• The nitrates, perchlorates and sulphates of all elements are soluble while their hydroxides, theorides and carbonates
  are insoluble.

Question 148.
Differentiate between lanthanoids and actinoids.
Answer:

Lanthanoids	Actinoids
Electronic configuration [Xe] 4f1-14 5d0-1, 6s2	Electronic configuration [Rn] 5f1-14 6d0-1, 7s2
The differentiating electron enters the 4f subshell.	The differentiating electron enters the 5f subshell.
Except for Promethium all other elements occur in nature.	Except for Uranium and Thorium, all others are synthesized in the laboratory.
The binding energy of 4f electrons is higher.	5f-orbitals have lower binding energy.
Only Promethium is radioactive.	All elements are radioactive.
Besides 3 + oxidation state they show 2 + and 4 + oxidation states.	Besides 3 + oxidation state they show 2 + , 4 + , 5 + , 6 + , 7 + oxidation states.
They have a less tendency to form complexes.	They have greater tendency to form complexes.
Many lanthanoid ions are colourless. Their colour is not as deep and sharp as actinoids.	Actinoids are coloured ions. Their colour is deep, e.g. U3+ is red and U4+ is green.
Lanthanoids cannot form oxo-cations.	Actinoids form oxo-cations such as – UO2+, PuO2+, UO22+, PuO22+.
Lanthanoid hydroxides are less basic.	Actinoid hydroxides are more basic.
Lanthanoid contraction is relatively less.	Actinoid contraction from element to element is comparatively more.
Mutual shielding of 4f electrons is more.	Mutual shielding effect of 5f electrons is less.

Question 149.
Compare Pre-transition metals, Lanthanoid and transition metals.
Answer:

Multiple Choice Questions

Question 150.
Select and write the most appropriate answer from the given alternatives for each sub-question :

1. In transition elements, the different electron enters into
(a) ns subshell
(b) np subshell
(c) (n – 1) d subshell
(d) (n – 2)f subshell
Answer:
(c) (n – 1) d subshell

2. Chromium (Z = 24) has electronic configuration
(a) [Ar]4d^A 4s²
(b) [Ar] 4d⁵ 45¹
(c) [Ar] 3d⁵ 3s¹
(d) [Ar] 3d⁵ 4s¹
Answer:
(d) [Ar] 3d⁵ 4s¹

3. Manganese achieves the highest oxidation state in its compounds
(a) Mn₃O₄
(b) KMnO₄
(c) K₂MnO₄
(d) MnO₂
Answer:
(b) KMnO₄

4. The group which belongs to transition series is
(a) 2
(b) 7
(c) 13
(d) 15
Answer:
(b) 7

5. The last electron of transition element is called
(a) s-electron
(b) p-electron
(c) d-electron
(d) f-electron
Answer:
(c) d-electron

6. Which one of the following elements does NOT belong to first transition series?
(a) Fe
(b) V
(c) Ag
(d) Cu
Answer:
(c) Ag

7. The incomplete d-series is
(a) 3d
(b) 4d
(c) 5d
(d) 6d
Answer:
(d) 6d

8. The electronic configuration of Sc is
(a) [Ar] 3d² 4s²
(b) [Ar] 3d¹ 4s²
(c) [Kr] 3d¹ 4s²
(d) [Kr] 3d² 4s¹
Answer:
(b) [Ar] 3d¹ 4s²

9. The observed electronic configuration of copper is
(a) [Ar]¹⁸ 3d⁹ 4s²
(b) [Kr] 3d¹⁰ 45¹
(c) [Kr] 3d⁹ 4s²
(d) [Ar] 3d¹⁰ 45¹
Answer:
(d) [Ar] 3d¹⁰ 45¹

10. Fe belongs to the
(a) 3d-transition series elements
(b) 4d-transition series elements
(c) 5d-transition series elements
(d) 6d-transition series elements
Answer:
(a) 3d-transition series elements

11. Which one of the following elements does not exhibit variable oxidation states?
(a) Iron
(b) Copper
(c) Zinc
(d) Manganese
Answer:
(c) Zinc

12. In KMnO₄, oxidation number of Mn is
(a) 2+
(b) 4 +
(c) 6 +
(d) 7+
Answer:
(d) 7+

13. Which one of the following transition elements shows the highest oxidation state?
(a) Sc
(b) Ti
(c) Mn
(d) Zn
Answer:
(c) Mn

14. The colour of transition metal ions is due to
(a) s → s transition
(b) d → d transition
(c) p → p transition
(d) f → f transition
Answer:
(b) d → d transition

15. Which one of the following compounds is expected to be coloured?
(a) AgNO₃
(b) CuSO₄
(c) ZnCl₂
(d) CuCl
Answer:
(b) CuSO₄

16. The metal ion which is NOT coloured, is
(a) Fe³⁺
(b) V²⁺
(c) Zn²⁺
(d) Ti³⁺
Answer:
(c) Zn²⁺

17. A pair of coloured ion is
(a)Cu²⁺, Zn²⁺
(b)Cr³⁺ , Cu⁺
(c) Cd²⁺, Mn⁵⁺
(d) Fe²⁺, Fe³⁺
Answer:
(d) Fe²⁺, Fe³⁺

18. The highest oxidation state is shown by
(a) Fe
(b) Mn
(c) Os
(d) Cr
Answer:
(c) Os

19. Transition elements are good catalysts since
(a) they show variable oxidation states
(b) they have partially filled d-orbitals
(c) they have low I.P
(d) they have small atomic radii
Answer:
(a) they show variable oxidation states

20. Highest magnetic moment is shown by the ion
(a) V³⁺
(b) Co³⁺
(c) Fe³⁺
(d) Cr³⁺
Answer:
(c) Fe³⁺

21. The most common oxidation state of lanthanoids is
(a) +4
(b) +3
(c) +6
(d) +2
Answer:
(b) +3

22. Which one of the following elements belong to the actinoid series?
(a) Cerium
(b) Lutetium
(c) Thorium
(d) Lanthanum
Answer:
(c) Thorium

23. The total number of elements in each of f-series is
(a) 10
(b) 12
(c) 14
(d) 15
Answer:
(c) 14

24. The general electronic configuration of Lanthanoids is
(a) [Xe] 4f^{1 – 14} 5d^{0 – 1} 6s²
(b) [Xe] 4f^{2 – 14} 5d^{0 – 1} 6s²
(c) [Xe] 4f^{1 – 13} 5d^{0 – 1} 6s²
(d) [Xe] 4f^{0 – 14} 5d^{0 – 1} 6s¹
Answer:
(a) [Xe] 4f^{1 – 14} 5d^{0 – 1} 6s²

25. f-block elements are called ………………….
(a) transition elements
(b) representative elements
(c) inner transition elements
(d) alkalin earth metals
Answer:
(c) inner transition elements

26. Actinoids form coloured salts due to the transition of electrons in
(a) d – d
(b) f – f
(c) f – d
(d) s – f
Answer:
(b) f – f

27. In the periodic table, Gadolinium belongs to
(a) 4th Group 6th period
(b) 4th group 4th period
(c) 3rd group 5th period
(d) 3rd group 7th period.
Answer:
(d) 3rd group 7th period.

28. The transuranic elements are prepared by
(a) addition reaction
(b) substitution reactions
(c) decomposition reaction
(d) nuclear reactions
Answer:
(d) nuclear reactions

Balbharti Maharashtra State Board 12th Chemistry Important Questions Chapter 14 Biomolecules Important Questions and Answers.

Maharashtra State Board 12th Chemistry Important Questions Chapter 14 Biomolecules

Question 1.
What are biomolecules? Give examples of biomolecules.
Answer:
Biomolecules: The lifeless, complex organic molecules which combine in a specific manner to produce life or control biological reactions are called biomolecules.

Examples: Carbohydrates, lipids (fats and oils), nucleic acids, enzymes.

Question 2.
What is the importance of biomolecules?
Answer:
Biomolecules are organic molecules which combine in a particular fashion to give complex substances which help to sustain life and produce identical daughter cells and play an important role in the actions of an organism.

• Carbohydrates are the major constituents of food and source of energy.
• Proteins help in proper functioning of living beings. They are important constituents of skin, hair, muscles. Enzymes which catalyse chemical reactions that take place in cells are proteins.
• Lipids (fats and oils) function as the storehouses of energy.
• Nucleic acids, the ribonucleic acid (RNA), and deoxyribonucleic acid (DNA) are responsible for genetic characteristics and synthesis of proteins.

Question 3.
What are carbohydrates?
OR
Define the term : Carbohydrates.
Answer:
Carbohydrates : Carbohydrates are optically active polyhydroxy aldehydes or polyhydroxy ketones, or the compounds which on hydrolysis produce polyhydroxy aldehydes or polyhydroxy ketones.

Examples : Glucose, sucrose, fructose.

Question 4.
What is monosaccharide?
Answer:
The basic unit of all carbohydrates which is a simple carbohydrate and cannot be hydrolysed further is known as monosaccharide. The monosaccharide is crystalline and soluble in water. E.g. Glucose, fructose, ribose.

Question 5.
Mention the names of monosaccharides or simple carbohydrates.
Answer:
Monosaccharides are (1) glucose (2) fructose (3) ribose.

Question 6.
State the basic unit of all carbohydrates.
Answer:
The basic unit of all carbohydrates which is a simple carbohydrate and cannot be hydrolysed further is known as monosaccharide.

Question 7.
How are carbohydrates classified?
OR
Classification of carbohydrates with examples.
Answer:
Carbohydrates are classified as monosaccharides oligosaccharides and polysaccharides.
(1) Monosaccharides : These carbohydrates cannot be further hydrolysed into smaller units. They are basic units of all carbohydrates, and are called monosaccharides.

Examples : Glucose, fructose, ribose

(2) Oligosaccharides : An oligosaccharide is a carbohydrate (sugar) which on hydrolysis gives two to ten monosaccharide units.
Depending on the number of monosaccharides produced on hydrolysis, oligosaccharides are further classified as :

Oligosaccharide is homogeneous. In this, each molecule of oligosaccharide contains the same number of monosaccharide units joined together in the same order as every other molecule of the same oligosaccharide.

(3) Polysaccharides : These are carbohydrates which on hydrolysis give a large number of monosaccharides.

Polysaccharides are tasteless, amorphous, insoluble in water. They are long chain, naturally αcurring polymers of carbohydrates.

Example : Cellulose, starch, glycogen.

Question 8.
Classify the following carbohydrates into Monosaccharide, Disaccharide, Oligosaccharide, Polysaccharide.
(1) Glucose
(2) Starch
(3) Sucrose
(4) Maltose
(5) Galactose
(6) Lactose
(7) Ribose.
Answer:

Carbohydrates	Class
(1) Glucose	Monosaccharide
(2) Starch	Polysaccharide
(3) Sucrose	Disaccharide
(4) Maltose	Disaccharide
(5) Galactose	Monosaccharide
(6) Lactose	Disaccharide
(7) Ribose	Monosaccharide

Question 9.
Classify the following carbohydrates.
(1) Cellulose,
(2) Maltose,
(3) Raffinose,
(4) Fructose.
Answer:

Carbohydrates	Class
(1)     Cellulose (2)     Maltose (3)     Raffinose (4)     Fructose	Polysaccharide Disaccharide Trisaccharide Monosaccharide

Question 10.
Classify the following into monosaccharides, oligosaccharides and polysaccharides.
(1) Starch
(2) Glucose
(3) Stachyose
(4) Maltose
(5) Raffinose
(6) Cellulose
(7) Sucrose
(8) Lactose.
Answer:

Monosaccharides	Glucose
Oligosaccharides	Stachyose, maltose, raffinose, sucrose, lactose
Polysaccharides	Starch, cellulose

Question 11.
Classify the following into monosaccharides and disaccharides.
Ribose, maltose, galactose, fructose and lactose (~2 mark each)
Answer:

Monosaccharides	Ribose, galactose, fructose
Disaccharides	Maltose, lactose

Question 12.
Give the preparation of glucose from sucrose or cane sugar.
OR
Describe the laboratory method of preparation of glucose.
Answer:
Preparation of glucose from sucrose (cane sugar) : Laboratory method.

Glucose is prepared in the laboratory by hydrolysis of sucrose by boiling it with dilute hydrαhloric acid or dilute sulphuric acid for about two hours. On hydrolysis, sucrose gives one molecule of glucose and one molecule of fructose.

Alcohol is added during cooling to separate glucose and fructose since, glucose is almost insoluble in alcohol, hence it crystallizes out first. Fructose remains in the solution as it is more soluble than glucose.

Crystals of glucose are separated out by filtration and purified by recrystallization.

Question 13.
Give the preparation of glucose from starch.
OR
How is glucose prepared on commercial scale?
Answer:
Commercially, on a large scale, glucose is prepared by hydrolysis of starch with dilute sulphuric acid. Starchy material is mixed with water and dilute sulphuric acid and heated at 393 K under 2 to 3-atm pressure. Starch is hydrolysed to give glucose.

Question 14.
Explain the structure of glucose.
Answer:
Molecular formula of glucose is C₆H₁₂O₆.

Glucose has an aldohexose structure. In other words, glucose molecule contains one aldehydic, that is, formyl group and the remaining five carbons carry one hydroxyl group (-OH) each. The six carbons in glucose form one straight chain.

Question 15.
Describe the action of following reagents on glucose :
(1) HI
(2) Hydroxyl amine (NH₂OH)
(3) Hydrogen cyanide
(4) Bromine water
(5) dil. Nitric acid
(6) Acetic anhydride.
Answer:
(1) Action of HI : Glucose on prolonged heating with HI gives n-hexane, indicates that all the six carbon atoms are linked in straight chain.

(2) Action of hydroxyl amine : Glucose reacts with hydroxyl amine in an aqueous solution to form glucose oxime. This indicates the presence of CHO group in glucose.

(3) Action of hydrogen cyanide : Glucose reacts with hydrogen cyanide to form glucose cyanohydrin.

(4) Action of bromine water : Glucose on oxidation with mild oxidising agent like bromine water gives gluconic acid, which shows that the carbonyl group in glucose is aldehyde group.

(5) Action of dll. nitric acid : Glucose on oxidation with dilute nitric acid forms dicarboxylic acid, saccharic acid. This indicates the presence of a primary alcoholic group (-CH₂OH) in glucose.

(6) Action of acetic anhydride : When glucose is heated with acetic anhydride in the presence of catalyst pyridine, glucose penta acetate is formed. It indicates that glucose is a stable compound and contains five hydroxyl groups.

Question 16.
Write Fischer projection formulae for
(1) Glucose
(2) Gluconic acid
(3) Saccharic acid.
Answer:
Fischer projection formulae of glucose, gluconic acid and saccharic acid :

Question 17.
Explain D and L configuration in sugars.
Answer:
The simplest carbohydrates glyceraldehyde is chosen as the standard, to assign D and L configuration to monosaccharides. Glyceraldehyde contains one asymmetric carbon atom and exist in two enantiomeric forms

The dextro entantiomer is represented as (+) glyceraldehyde and it is referred as D-configuration i.e., D-glyceraldehyde. The laevo enantiomer of glyceraldehyde is represented as ( -) glyceraldehyde and it corelated as L-configuration i.e., L-glyceraldehyde.

In Fischer projection formula, a monosaccharide is assigned D-configuration if the (- OH) hydroxyl group at the last chiral carbon and lies towards right hand side. On the other hand it is assigned L-configuration if the – OH group on the last chiral carbon atom and lies on the left hand side. In monosaccharides, the most oxidised carbon (i.e., -CHO) is at the top.

Examples :

Question 18.
Write Fischer projection formulae for (a) L-( + )-erythrose (b) L-( +) ribulose.
Answer:

Question 19.
Is the following sugar, D-sugar or L-sugar?

Answer:
The compound is L-sugar.
The compound is L-sugar.

Question 20.
Assign D/L configuration to the following monosaccharides.


Answer:

Question 21.
Explain ring structure of glucose.
Answer:

Glucose has two cyclic structures (II and III) which are in equilibrium with each other through the open chain structure (I) in aqueous solution.

The ring structure of glucose is formed by reaction between the formyl ( – CHO) group and the alcoholic (- OH) group at C – 5. Thus, the ring structure is called a hemiacetal. The two hemiacetal structures (II and III) differ only in the configuration of C – I (Fig.), the additional chiral centre resulting from ring closure. The two ring structures are called α- and β- anomers of glucose and C-l is called the anomeric carbon. The ring of the cyclic structure of glucose contains five carbons and one oxygen. Thus, it is a six membered ring. It is called pyranose structure, in analogy with the six membered heterαyclic compound pyran (IV). Hence glucose is also called glucopyranose.

Question 22.
Write the structures of α-D-( + )-glucopyranose and β-D-( +) glucopyranose.
Answer:

Question 23.
Explain Haworth formula of glycopyranose.
Answer:

In the Haworth formula the pyranose ring is considered to be in a perpendicular plane with respect to the plane of paper. The carbons and oxygen in the ring are in the places as they appear in figure. The lower side of the ring is called α-side and the upper side is the β-side. The α-anomer has its anomeric hydroxyl (- OH) group (at C-l) on the α-side, whereas the β-anomer has its anomeric hydroxyl (- OH) group (at C-l) on the β-side. The groups which appear on right side in the Fischer projection formula appear on α-side in the Haworth formula, and the groups which appear on left side in the fischer projection formula appear on a β-side in the Haworth formula.

Question 24.
Explain the structure of fructose.
Answer:
Fructose has molecular formula C₆H₁₂O₆. It contains ketonic functional group at carbon number 2 and six carbon atoms in straight chain. It belongs to D-series and is a laevo rotatory compound. It is written as D-( – )-fructose. Being an α-hydroxy keto compound fructose is a reducing sugar.

Question 25.
Draw mirror images of glucose and fructose.
Answer:
(1) Glucose

(2) Fructose

Question 26.
Write the two cyclic structures of α-D-( – )-fructofuranose and β-D-( – )-fructofuranose exist in equilibrium with open chain structure.
Answer:

Question 27.
Write the Haworth projection formulae for α -D-( -) – Fructofuranose and β – D – ( -) – Fructo- furanose.
Answer:

Question 28.
Explain the structure of sucrose.
Answer:
Sucrose is a hexasaccharide and has molecular formula C₁₂H₂₂O₁₁. The structure of shcrose contains glycosidic linkage between C – 1 of α-glucose and C – 2 of β-fructose. Since aldehyde and ketone groups of both monosaccharide units are involved in the formation of glycosidic bond, sucrose is a nonreducing sugar.

Sucrose is dextrorotatory, on hydrolysis with dilute acid or an enzyme invertase gives equimolar mixture of dextrorotatory glucose and laevorotatory fructose.

The solution is laevorotatory because laevo rotation of fructose (- 92.4°) is more than dextrorotation of glucose ( + 52.50), hence the sign of rotation is changed from (+) to (-) after hydrolysis, the product is called invert sugar.

Question 29.
Explain the structure of maltose.
Answer:
Maltose is another disaccharide obtained by partial hydrolysis of starch or made of two units of D-glucose. In maltose, C-l of one α-D-glucose is linked to C-4 of another α-D-glucose molecule by glycosidic linkage. The glucose ring which uses its hydroxyl group at C-1 is α – 1 → 4 glycosidic linkage. It is a reducing sugar because a free aldehyde group can be produced at C₁ of second glucose molecule. Maltose on hydrolysis with dilute acids gives glucose.

Question 30.
Draw a neat diagram for Haworth formula of maltose.

Question 31.
Explain the structure of lactose.
Answer:

Lactose (C₁₂H₂₂O₁₁) is a disaccharide. It is found in milk, therefore, it is also known as milk sugar. It is formed from two monosaccharide units, namely D – galactose and D – glucose. The glycosidic linkage is formed between C-l of β-D-galactose and C -4 of glucose. Therefore the linkage in lactose is called β – 1,4 – glycosidic linkage. The hemiacetal group at C-l of the glucose unit is not involved in glycosidic linkage but is free. Hence lactose is a reducing sugar. The above figure shows Haworth formula of lactose.

Question 32.
What are the hydrolysis products of (1) lactose (2) sucrose?
Answer:
(1) Lactose on hydrolysis in presence of an acid or enzyme lactase gives one molecule each of glucose and galactose

(2) Sucrose on hydrolysis in the presence of dii. acid or the enzyme invertase gives one molecule each of glucose and fructose.

Question 33.
Explain the structure of starch.
Answer:
Starch is found in cereal grains, roots, tubers, potatoes, etc. It is a polymer of α-D-glucose and consists of two components, amylose and amylopectin.

Amylose is water soluble component forms blue coloured complex with iodine. It constitutes about 20 % of starch. Amylose contains 200 to 1000 α-D-glucose units linked together by glycosidic linkage between C-l of one unit and C-4 of another unit. i.e. α-1, 4 glycosidic linkages.

Amylopectin is insoluble in water and constitutes about 80 % starch which forms blue-violet coloured complex with iodine. It is a branched chain polymer. In amylopectin, α-D-glucose molecules are linked together by glycosidic linkage between C₁ – of one unit and C-4 of another unit to form long chain and branching αcurs by glycosidic linkage between C-l and C6 glycosidic linkage.

Question 34.
What are polysaccharides?
Answer:
A large number of same or different monosaccharides are joined together by glycosidic linkages are called polysaccharides. They have general formula (C₆H₁₀O₅)_n.

Question 35.
Explain the structure of cellulose.
Answer:

Cellulose mainly αcurs in plants. Cell wall of plant cells is made up of cellulose. It is a long chain polymer. In cellulose, β-D-glucose units are linked by glycosidic linkage between C₁-of one unit of glucose and C₄ of another glucose unit. Thus cellulose contains 1 → 4β glycosidic linkages like those in cellobiose.

Question 36.
Explain the structure of glycogen.
Answer:
The glucose is stored in animal body in the form of glycogen. It is also known as animal starch because its structure is similar to amylopectin. Glycogen is highly branched. Whenever the body is required glucose, enzymes breaks the glycogen to glucose.

Question 37.
How is glycogen different from starch?
Answer:
Starch is the main storage molecules of plants whereas glycogen is the main storage molecule of animals. Starch is found in cereals, roots, tubers, etc. Glycogen is present in liver, muscles and brain.

Question 38.
What do you understand by the term glycosidic linkage?
Answer:
The linkage between two monosaccharide units through oxygen atom is called glycosidic linkage.

Question 39.
What is the basic structural difference between starch and cellulose?
Answer:
Starch is a polymer of a-glucose and consists of two components-amylose and amylopectin. In amylose α-D-D-( + )-glucose units held by C,-C4 glycosidic linkage and in amylopectin, α-D-glucose units held by C1-C4 glycosidic linkage whereas branching αcurs by C₁-C₆ glycosidic linkage. [Refer Question 35 (i) (ii) Fig.] Cellulose is a straight chain polysaccharide composed only of β-D-glucose units held by C₁-C₄ glycosidic linkage. (Refer Question 37 Fig.)

Question 40.
Define the term : Protein OR What are proteins?
Answer:
Chemically proteins are polyamides which are high molecular weight polymers of the monomer units i.e. α-amino acids. OR It can also be defined as Proteins are the biopolymers of a large number of a-amino acids and they are naturally occurring polymeric nitrogenous organic compounds containing 16% nitrogen and peptide linkages (-CO-NH-).

Question 41.
Write the common sources of protein.
Answer:
Common sources of proteins are milk, pulses, peanuts, eggs, fishes, cheese, cereals, etc. They are also the principal materials of muscle, nerves, tendons, skin, blood, enzymes, many hormones and antibiotics.

Question 42.
What are the products of hydrolysis of proteins?
Answer:
On hydrolysis, proteins give a mixture of α-anlino acids.

The α-carbon in α-amino acids ohtained by hydrolysis of proteins has ‘L’ configuration.

Question 43.
What are the a-amino acids?
Answer:
α-Amino acids are carboxylic acids having an amino (- NH₂) group bonded to the α-carbon, i.e. the carbon next to the carboxyl (- COOH) group.

α-amino acids are derivatives of carboxylic acids, obtained by replacing – H atom by amino group. They are bifunctional compounds containing acidic and basic – NH₂ groups.
Example : (where R is an alkyl group or aryl group).

The amino acids are colourless, crystalline, water soluble, high melting solids. These acids in their aqueous solutions behave like salts due to presence of both acidic, and basic. (- NH₂) groups in the same molecule.

Such a doubly charged ion is known as zwitter ion. Example :

Question 44.
What are the final products of hydrolysis of proteins?
Answer:
Proteins on hydrolysis with dilute solution of acids, alkalies or enzymes give a mixture of large number of a-amino acids as final products.

For example :

Question 45.
Write the classification of amino acids, giving examples.
Answer:
The amino acids are of three types : acidic, basic and neutral. The symbol ‘R’ in the structure of a-amino acids represents side chain and may contain additional functional groups.

(1) Acidic amino acids : If ‘R’ contains a carboxyl (- COOH) group the amino acid is acidic amino acid, i.e. If carboxyl groups are more in number than amino groups, then amino acids are acidic in nature.

Examples : Glutamic acid HOOC-CH₂-CH₂-; Aspartic acid HOO-CH₂–

(2) Basic amino acids : If ‘R’ contains an amino (1°, 2°, or 3°) group, it is called basic amino acid i.e. If amino groups are more in number than carboxyl groups then amino acids are basic in nature.

Examples : Arginine

(3) Neutral amino acids : The other amino acids having neutral or no functional group in ‘R’ are called neutral amino acids, i.e. The amino acids having equal number of amino and carboxyl groups are neutral amino acids.

Examples : Alanine CH₃-; Valine (CH₃)₂-CH

Question 46.
What are essential and non-essential amino acids? Give two examples of each.
Answer:
The amino acids, which cannot be synthesised in the body and are supplied through diet are called essential amino acids. Examples : Lysine H₂N-(CH₂)₄-; Valine (CH₃)₂CH- The amino acids which are synthesized in the body are called non-essential amino acids.

Examples : Glutamic acid HOO-CH₂-CH₂-; Serine HO-CH₂–

Question 47.
What is meant by Zwitter ion?
Answer:
An a-amino acid molecule contains both acidic carboxyl ( – COOH) group as well as basic amino (- NH₂) group. Proton transfer from acidic group to basic group of amino acid forms a salt, which is a dipolar ion called a zwitterion.

Question 48.
Draw zwitter ion of alanine and other two forms.
Answer:

Question 49.
What is a peptide bond (peptide linkage)?
OR
Define peptide bond.
Answer:
Proteins are the polymers of a-amino acids and they are connected to each other. The bond that connects a-amino acids to each other is called peptide bond (peptide linkage, – CONH -).

Question 50.
How is peptide linkage (dipeptide linkage) formed in proteins? How is tripeptide formed?
Answer:
Peptide linkage is formed by condensation of acidic group of one molecule of a-amino acid and basic -NH₂ group of other molecule of α-amino acid with elimination of water.

When one more molecule of amino acid combines with dipeptide, it forms tripeptide.

Thus, it forms tetra, penta and finally a polypeptide chain i.e. proteins. Hence, proteins are basically polypeptides.

Question 51.
Write the structures of all possible dipeptides which can be obtained from glycine and alanine.
Answer:
(1) Dipeptide from glycine :
Carboxylic group of glycine reacting with amino group another molecule of glycine to form dipeptide

(2) Dipeptide from alanine :
Carboxylic goup of alanine reaction with amino goup of another molecule of alamine to form dipeptide

(3) Dipeptide from glycine and alanine :
Carboxylic group of glycine reacting with amino group another molecule of alanine to form dipeptide

Question 52.
How are proteins classified on the basis of molecular shapes?
Answer:
On the basis of their molecular shapes proteins are classified as :
(1) Fibrous proteins : The proteins in which the polypeptide chains lie parallel (side by side) to form fibre-like structure, are called fibrous proteins. The polypeptide chains held together by hydrogen bonds. These proteins are insoluble in water.

The fibrous proteins are tough and insoluble in water, and dilute acids or bases.

Example : myαin (in muscles), keratin (in hair, nails, skin), fibroin (in silk), collagen (in tendons), etc.

(2) Globular proteins : The proteins have spherical shape. This shape results from coiling around of the polypeptide chain of protein, and have intramolecular hydrogen bonding are called globular proteins.

They are soluble in water and dilute acids or bases.

Example : Haemoglobin (in blood), albumin (in eggs), insulin (in pancreas), etc.

Question 53.
Distinguish between globular and fibrous proteins.
Answer:

Globular proteins	Fibrous proteins
(1) The chains of polypeptides of protein coil around to give a spherical shape. (2) Globular proteins are soluble in water. (3) They are sensitive to small changes of temperature and pH. (4) They possess biological activity.	(1) The proteins in which the polypeptide chains lie parallel to form fibre like structure. (2) Fibrous proteins are insoluble in water. (3) They are stable to moderate changes of temperature and pH. (4) They do not possess biological activity.

Question 54.
Draw a neat labelled diagram for the secondary structure of protein.
Answer:
Secondary structure of proteins : The three-dimensional arrangement of lαalized regions of a long polypeptide chain is called the secondary structure of protein. Hydrogen bonding between N-H proton of one amide linkage and C = O oxygen of another gives rise to the secondary structure. There are two different types of secondary structures i.e. α-helix and β-pleated sheet.

α-Helix : In a-helix structure, a polypeptide chain gets coiled by twisting into a right handed or clαkwise spiral known as a-helixn. The characteristic features of α-helical structure of protein are :

(1) Each turn of the helix has 3.6 amino acids.
(2) A C = O group of one amino acid is hydrogen bonded to N – H group of the fourth amino acid along the chain.
(3) Hydrogen bonds are parallel to the axis of helix while R groups extend outward from the helix core.
Myosin in muscle and a-keratin in hair are proteins with almost entire a-helical secondary structure.

β-Pleated sheet : In secondary structure, when two or more polypeptide chains (strands) line up side-by-side is called β-pleated sheets. The β-picate sheet structure of protein consists of extended strands of polypeptide chains held together by intermolecular hydrogen bonding. The characteristics of β-pleated sheet structure are :

• The C = O and N – H bonds lie in the planes of the sheet.
• Hydrogen bonding occurs between the N – H and C = O groups of nearby amino acid residues in the neighbouring chains.
• The R groups are oriented above and below the plane of the sheet.

The β-pleated sheet arrangement is favoured by amino acids with small R groups.

Question 55.
What is denaturation of proteins? How is denaturation brought about?
OR
What is the effect of denaturation on the structure of proteins?
Answer:
The prαess by which the molecular shape of protein changes without breaking the amide / peptide bonds that form the primary structure is called denaturation. OR Proteins gets easily precipitated. It is an irreversible change and the prαess is called denaturation of proteins.

Denaturation uncoils the protein and destroys the shape and thus loses their characteristic biological activity. Denaturation is brought about by heating the protein with alcohol, concentrated inorganic acids or by salts of heavy metals. During denaturation secondary and tertiary and quternary structures are destroyed but primary structure remains intact.

Example : Boiling of egg to coagulate egg white, conversion of milk to curd.

Question 56.
Define : Enzymes
Answer:
All biological reactions are catalysed by bio-catalyst in living organisms called enzymes.

Question 57.
What are enzymes? Explain with suitable example.
Answer:
All biological or bio-catalysts which catalyse the reactions in living organisms are called enzymes. Chemically all enzymes are proteins. They are required in very small quantities as they are catalyst also they reduce the activation energy for a particular reaction.

Example : Enzyme maltase converts maltose to glucose.

Question 58.
Explain the catalytic action of enzymes.

Answer:
Mechanism of enzyme catalysis : Action of an enzyme on a substrate is known as lock-and-key mechanism.

Accordingly, the enzyme has active site on its surface. A substrate molecule can attach to this active site only if it has the right size and shape. Once in the active site, the substrate is held in the correct orientation, enzymes provide functional group which will attack the substrate and forms the products of reaction. The products leave the active site and the enzyme is ready to act as catalyst again.

Question 59.
Give examples of industrial application of enzyme catalysis.
Answer:

• Glucose Isomerase (enzyme) is used in conversion of glucose to sweet-tasting fructose.
• New antibiotics are manufactured using penicillin acylase (enzyme).
• Laundry detergentts are manufactured using proteases (enzyme).
• Esters used in cosmetics are manufactured using genetically engineered enzyme.

Question 60.
Draw a neat diagram for enzyme catalysis.
Answer:

Question 61.
State the main functions of enzymes.
Answer:
Enzymes are biological catalyst and they are highly specific in nature. The two main functions are as follows :
(1) They lower the requirement of activation energy.
(2) They speed up the rate of reaction.
E.g. Enzyme maltase catalyses maltose to glucose.
\(\mathrm{C}_{12} \mathrm{H}_{22} \mathrm{O}_{11}+\mathrm{H}_{2} \mathrm{O} \stackrel{\text { Maltase }}{\longrightarrow} 2 \mathrm{C}_{6} \mathrm{H}_{12} \mathrm{O}_{6}\)

Question 62.
What are nucleic acids?
Answer:
Nucleic acids are unbranched polymers of repeating monomers i.e. nucleotides. In other words, nucleic acids have a polynucleotide structure which in turn consists of a base, a pentose sugar and phosphate moiety.

Nucleic acids are biomolecules which are found in the nuclei of all living cells in the form of nucleoproteins or chromosomes.

(Nucleoproteins = Proteins + Nucleic acid)
(prosthetic group)

Question 63.
State the types of nucleic acids.
Answer:
The types of nucleic acids are : Ribonucleic acid (RNA) and deoxy ribonucleic acid (DNA). DNA molecules contain several million nucleotides while RNA molecules contain a few thousand nucleotides.

Question 64.
Explain chemical composition of nucleic acids.
Answer:
Nucleic acids have a polynucleotide structure. Nucleic acids (RNA and DNA) consists of three components :
(1) monosaccharide (sugar)
(2) nitrogen containing base and
(3) phosphate group.

(1) Monosaccharides : Nucleotides of both RNA consist of five membered monosaccharide ring (furanose), called as simply sugar component.

In RNA, the sugar component of nucleotide units is D-ribose and in DNA 2-deoxy-D-ribose.
2 – deoxy means no – OH group at C₂ position.

(2) Nitrogen containing base : Total five nitrogen – containing bases are present in nucleic acids. Three bases with one ring (cytosine, uracil and thymine) are derived from the parent compound pyrimidine. Two bases with two rings (adenine and guanine) are derived from the parent compound purine. Each base in designated by a one-letter symbol. Uracil (U) αcurs only in RNA while thymine (T) ocurs only in DNA.

(3) Phosphate group : The sugar units are joined to phosphate through C₃ and C₅ hydroxyl groups.

Question 65.
What is meant by nucleosides?
OR
Write the structure of nucleoside. Give examples.
Answer:

A nucleoside contains two basic components of nucleic acids i.e. a pentose sugar and a nitrogenous base.

A nucleoside is formed when 1 -position of a pyrimidine (cytosine, thymine or uracil) or 9-position of guanine or adenine base is attached to C- l of sugar by β-linkage.

Examples: Formation of nucleoside:

Question 66.
What is meant by nucleotide?
OR
Write the structure of nucleotide. Give example.
Answer:

A nucleotide contains all three basic components of nucleic acids i.e., a pentose sugar, a phosphoric acid and a nitrogenous base. These are obtained by esterification of \(\mathrm{C}_{5}^{1}-\mathrm{OH}\) group of the pentose sugar by phosphoric acid. Nucleotides are joined together through phosphate ester linkage. Thus, nucleotides are monophosphates of nucleosides. Abridged names of some nucleotides are AMP, dAMP, UMP, dTMP and so on. Here, the first capital letter is derived from the corresponding base. MP stands for monophosphate. Small letter ‘d’ in the beginning indicates deoxyribose in the nucleotide.

Example :

 

Question 67.
Write the structure of nucleic acids.

Answer:
Nucleic acids, both DNA and RNA, are polymers of nucleotides, formed by joining the 3′ – OH group of one nucleotide with 5′ – phosphate of another nucleotide. Two ends of polynucleotide chain are distinct from each other. One end having free phosphate group of 5′ position is called 5′ end. The other end is 3′ end and has free OH – group at 3′ position.

Question 68.
Draw a schematic representation of polynucleotide structure of nucleic acids.
Answer:

Question 69.
Explain double helix.
OR
State the salient features of the Watson and Crick mode of DNA.
Answer:

The Salient features are :

1. DNA is made of two polynucleotide strands that wind into a right-handed double helix.
2. The two strands run in opposite directions: one from the Y end to the 3’ end, while the other from the 3’ end to the Y end.
3. Pcrpcndicular to the axis of the helix, the sugar – phosphate backbone lies on the outside of the helix and the bases lic on the inside.
4. The hydrogen bonding between the hases of the two DNA strands stabilizes the double helix. This gives rise to a ladder-like structure of DNA double helix.
5. Adenine always forms two hydrogen bonds with thymine and guanine forms three hydrogen bonds with cytosinc. Thus A – T arid C – G arc complementary hase pairs and the Two strands of the double helix arc complementary to each other.

Question 70.
Give scientific reasons :
1. In the preparation of glucose from sucrose, ethyl alcohol is added at the time of cooling.
Answer:
Hydrolysis of sucrose with dilute hydrαhloric acid gives glucose along with fructose.

Ethyl alcohol is added at the time of cooling in the preparation of glucose, to separate glucose from fructose. Glucose being insoluble in alcohol, crystallizes out first, while fructose being more soluble in alcohol, remains in the solution.

Question 71.
Answer in one sentence :

(1) How is glucose stored in the animal body?
Answer:
Glucose is stored in the form of glycogen in the animal body.

(2) Write other term used for carbohydrates.
Answer:
Carbohydrates are often termed as saccharides or sugars.

(3) How many moles of acetic anhydride will be required to form glucose penta acetate from 1 mole of glucose?
Answer:
10 moles of acetic anhydride.

(4) What are reducing sugars?
Answer:
Reducing sugars : Carbohydrates which reduce Fehling solution to red ppt of Cu₂0 or Tollen’s reagent to shining metallic silver are called reducing sugars. All monosaccharides and oligosaccharides except sucrose are reducing sugars.

(5) What are non-reducing sugars?
Answer:
Non-reducing sugars : Carbohydrates which do not reduce Fehling solution and Tollen’s reagent are called non-reducing sugars. E.g. sucrose.

(6) Give an example each of reducing and non-reducing sugars.
Answer:
Reducing sugars : Maltose or lactose
Non-reducing sugars : Sucrose.

(7) Name the linkage which joins two monosaccharide units through oxygen atom.
Answer:
The linkage which joins two monosaccharide units through oxygen atom is called glycosidic linkage.

(8) Name the sugar present in DNA.
Answer:
The sugar present in DNA is deoxyribose.

(9) A nucleotide from DNA containing thymine is hydrolysed. What are the products formed?
Answer:
When nucleotide from DNA containing thymine is hydrolysed, 2-deoxy-D-ribose, thymine and phosphoric acid is obtained.

(10) How is zwitterion formed?
Answer:
In aqueous solution, the carboxyl group loses a proton while the amino group accepts it, as a result, a dipolar or zwitter ion is formed.

(11) Name the amino acids which are synthesized in the body.
Answer:
The amino acids which are synthesized in the body are called non-essential amino acids. Examples : Glutamic acid, serine.

(12) Name the four bases present in DNA which of these is not present in RNA.
Answer:
Purines-adenine (A) and guanine (G); Pyrimidines-thymine (T) and cytosine (C), these four bases are present in DNA. Out of these, thymine (T) is not present in RNA.

(13) What are different types of RNA which are found in the cell?
Answer:
There are three different types of RNA found in the cell. (1) The messenger RNA which carries the message to the ribosome (2) Ribosomal RNA where synthesis of protein takes place (3) The transport RNA.

(14) State the functions of RNA and DNA.
Answer:
RNA and DNA are responsible for generic characteristics : DNA preserves the information and uses it by producing duplicate identical DNA molecules. RNA carries messages and transports them.

Multiple Choice Questions

Question 72.
Select and write the most appropriate answer from the given alternatives for each subquestion :

1. Which of the following is not sugar?
(a) Sucrose
(b) Starch
(c) Fructose
(d) Glucose
Answer:
(b) Starch

2. Which of the following is the example of disaccharide?
(a) Glucose
(b) Raffinose
(c) Cellulose
(d) Sucrose
Answer:
(d) Sucrose

3. Fructose is
(a) aldopentose
(b) aldohexose
(c) ketopentose
(d) ketohexose
Answer:
(d) ketohexose

4. Oxidation product of glucose with bromine water is
(a) sorbitol
(b) gluconic acid
(c) glutamic acid
(d) saccharic acid
Answer:
(b) gluconic acid

5. The general formula of carbohydrates is
(a) C(H₂O)
(b) C_x(H₂O)_y
(c) C_x(H₂O)
(d) C_x(H₂O)_x
Answer:
(b) C_x(H₂O)_y

6. Monosaccharides containing aldehyde group are called
(a) aldoses
(b) ketoses
(c) polysaccharides
(d) disaccharides
Answer:
(a) aldoses

7. Which of the following sugars can be used to prepare glucose on a large scale?
(a) Cellulose
(b) Cane sugar
(c) Galactose
(d) Starch
Answer:
(d) Starch

8. Which of the following carbohydrates cannot undergo hydrolysis?
(a) Glucose
(b) Sucrose
(c) Cellulose
(d) Maltose
Answer:
(a) Glucose

9. Glucose differs from fructose in
(a) the functional group
(b) the number of chiral carbon atoms
(c) the number of carbon atoms
(d) both (a) and (b)
Answer:
(d) both (a) and (b)

10. The example of aldopentose is
(a) arabinose
(b) glucose
(c) fructose
(d) sucrose
Answer:
(a) arabinose

11. Dextrose, grape sugar and blood sugar αcurs in
(a) fructose
(b) glucose
(c) sucrose
(d) starch
Answer:
(b) glucose

12. The example of ketopentose is
(a) galactose
(b) ribose
(c) raffinose
(d) maltose
Answer:
(b) ribose

13. Cane sugar on hydrolysis gives
(a) glucose and maltose
(b) glucose and lactose
(c) glucose and fructose
(d) only glucose
Answer:
(c) glucose and fructose

14. On commerical scale, glucose is prepared from
(a) starch
(b) potato pulp
(c) sucrose
(d) both (a) and (b)
Answer:
(d) both (a) and (b)

15. The number of monosaccharide units formed on hydrolysis of glucose are
(a) zero
(b) one
(c) two
(d) three
Answer:
(a) zero

16. Which of the following is NOT TRUE about glucose?
(a) It is monosaccharide
(b) It is a polyhydroxy aldehyde
(c) It is polyhydroxy ketone
(d) It contains six carbon atoms
Answer:
(c) It is polyhydroxy ketone

17. Final hydrolysis product of simple protein is
(a) carboxylic acid
(b) α-amino acid
(c) mineral acid
(d) acetic acid
Answer:
(b) α-amino acid

18. Haemoglobin is the example of-
(a) simple protein
(b) derived protein
(c) fibrous protein
(d) conjugated protein
Answer:
(d) conjugated protein

19. Protein are also called
(a) polysaccharides
(b) polypeptides
(c) polyglycerides
(d) polyster
Answer:
(b) polypeptides

20. The simplest amino acid is
(a) glycine
(b) oxalic acid
(c) adipic acid
(d) caprolactam
Answer:
(a) glycine

21. Amino acids usually exist in the form of zwitter ion which consist of
(a) the basic group-NH₂ and the acidic group -COOH
(b) the acidic group -N⁺H₃ and the basic group COO^–
(c) the acidic group -COO⁺ and the acidic group NH^3-
(d) acidic or basic group
Answer:
(b) the acidic group -N⁺H₃ and the basic group COO-

22. The water insoluble protein is
(a) casein of milk
(b) albumin
(c) serum albumin
(d) keratin of hair
Answer:
(d) keratin of hair

23. The main structural feature of a protein molecule is the presence of
(a) an ester linkage
(b) an ether linkage
(c) a peptide linkage
(d) all of these
Answer:
(c) a peptide linkage

24. Milk sugar is
(a) sucrose
(b) lactose
(c) maltose
(d) glucose
Answer:
(b) lactose

25. The carbohydrates used for silvering of mirror is
(a) fructose
(b) starch
(c) glucose
(d) cellulose
Answer:
(c) glucose

26. Which one of the following is NOT produced by human body?
(a) DNA
(b) Hormones
(c) Enzymes
(d) Vitamins
Answer:
(c) Enzymes

27. A biological catalyst is essentially
(a) an amino acid
(b) an enzyme
(c) a nitrogen molecule
(d) a carbohydrate
Answer:
(d) a carbohydrate

28. Which one of the following is not a constituent of RNA?
(a) Ribose
(b) Uracil
(c) Thymine
(d) Phosphate
Answer:
(b) Uracil

29. DNA is a polymer of units of
(a) sugars
(b) ribose
(c) amino acids
(d) nucleotides
Answer:
(c) amino acids

30. Which one of the following molecules will form zwitter ion?
(a) CH₃COOH
(b) CH₃CH₂NH₂
(c) CCl₃NO₂
(d) NH₂CH₂COOH
Answer:
(d) NH₂CH₂COOH

31. In metabolic prαess the maximum energy is given by
(a) carbohydrates
(b) proteins
(c) vitamins
(d) fats
Answer:
(d) fats

32. DNA has a structure of helix was reported by
(a) Herman Fischer
(b) Fedrick Sauger
(c) Andreas Marggraf
(d) Watson and Crick
Answer:
(d) Watson and Crick

33. The secondary structure of a protein is determined by
(a) co-ordinate bond
(b) covalent bond
(c) ionic bond
(d) hydrogen bond
Answer:
(d) hydrogen bond

34. In maltose, glycosidic linkage is present between the two glucose units at positions
(a) 1, 2
(b) 1, 1
(c) 1, 3
(d) 1, 4
Answer:
(d) 1, 4

35. Which of the following amino acids is basic in nature?
(a) Valine
(b) Tyrosine
(c) Arginine
(d) Luecine
Answer:
(c) Arginine

36. Sucrose molecules consists of
(a) a glucofuranose and a fructopyranose
(b) a glucofuranose and a fructofuranose
(c) a glucopyranose and a fructopyranose
(d) a glucopyranose and a’ fructofuranose
Answer:
(d) a glucopyranose and a’ fructofuranose

37. Which one of the following statements is not correct about DNA molecule?
(a) It has double helix structure
(b) It serves as hereditary material
(c) The two DNA strands are exactly similar
(d) Its replication is called semi-conservative mode of replication
Answer:
(c) The two DNA strands are exactly similar

38. Glycine on heating forms


Answer:
(a)

39. Acidic amino acid is
(a) Glutamine
(b) Glutamic acid
(c) Tyrosine
(d) Lysine
Answer:
(b) Glutamic acid

40. Basic amino acid is
(a) Lysine
(b) Glycine
(c) Cystine
(d) Alanine
Answer:
(a) Lysine

41. Precipitation of protein is referred to as
(a) destruction of proteins
(b) separation of proteins
(c) denaturation of proteins
(d) fragmentation of proteins
Answer:
(c) denaturation of proteins

42. An amino acid containing sulphur is
(a) serine
(b) cysteine
(c) valine
(d) asparagine
Answer:
(b) cysteine

43. Rhamnose is a
(a) carbohydrate
(b) protein
(c) lipid
(d) vitamin
Answer:
(a) carbohydrate

44. Lactose on hydrolysis gives
(a) glucose + glucose
(b) glucose + fructose
(c) glucose + galactose
(d) fructose + galactose
Answer:
(c) glucose + galactose

45. Raffinose on hydrolysis gives
(a) glucose + glucose + galactose
(b) glucose + fructose + galactose
(c) glucose + galactose + galactose
(d) fructose + galactose + galactose
Answer:
(b) glucose + fructose + galactose

46. Naturally αcurring glucose is
(a) dextro rotatory
(b) laevo rotatory
(c) racemic mixture
(d) all of these
Answer:
(a) dextro rotatory

47. Amylopectin is
(a) soluble in water and constitutes about 80% of starch
(b) insoluble in water and constitutes about 80% of starch
(c) Soluble in alcohol and constitutes about 60% of starch
(d) in soluble in alcohol and constitutes about 60% of starch
Answer:
(b) insoluble in water and constitutes about 80% of starch

48. Insulin contains
(a) 51 amino acids
(b) 151 amino acids
(c) 15 amino acids
(d) 115 amino acids
Answer:
(a) 51 amino acids

49. Pyranose structure of glucose is
(a) an open chain structure of glucose
(b) a structure of reduction product of glucose
(c) a cyclic six-membered structure of glucose
(d) a four-membered cyclic form of glucose
Answer:
(c) a cyclic six-membered structure of glucose

50. The number of – OH groups present in ribulose is
(a) 3
(b) 4
(c) 6
(d) 5
Answer:
(b) 4

51. Peptide linkage is

Answer:
(d)

52. Stachyose is an example of
(a) monosaccharides
(b) disaccharides
(c) trisaccharides
(d) tetrasaccharides
Answer:
(d) tetrasaccharides

53. How many moles of (CH₃CO)₂O will be required to form glucose pentaacetate form 2 moles of glucose?
(a) 2
(b) 5
(c) 10
(d) 2.5
Answer:
(c) 10

54. Which of the following NOT present in DNA?
(a) Adenine
(b) Guanine
(c) Thymine
(d) Uracil
Answer:
(d) Uracil

55. Maltose is a
(a) polysaccharide
(b) disaccharide
(c) trisaccharide
(d) monosaccharide
Answer:
(b) disaccharide