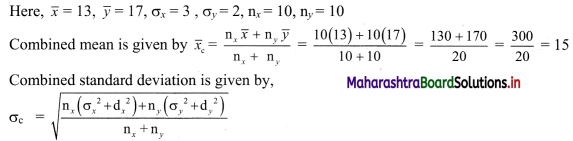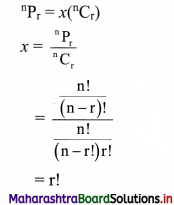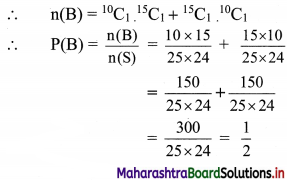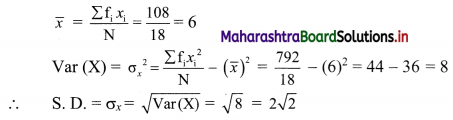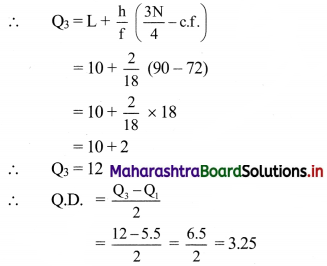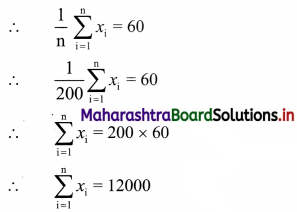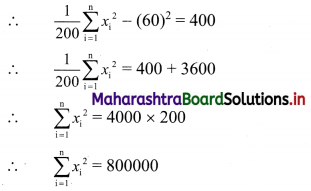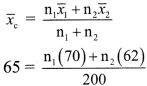Class 11

Permutations and Combinations Class 11 Commerce Maths 2 Chapter 6 Exercise 6.3 Answers Maharashtra Board

Balbharati Maharashtra State Board 11th Commerce Maths Solution Book Pdf Chapter 6 Permutations and Combinations Ex 6.3 Questions and Answers.

Std 11 Maths 2 Exercise 6.3 Solutions Commerce Maths

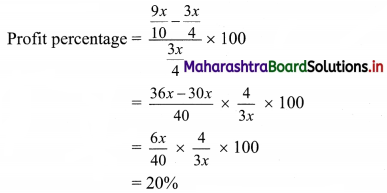
Question 1.
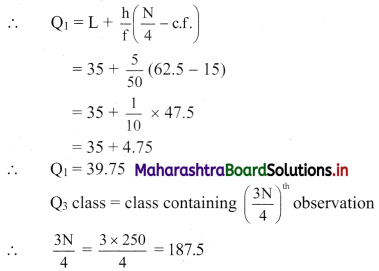
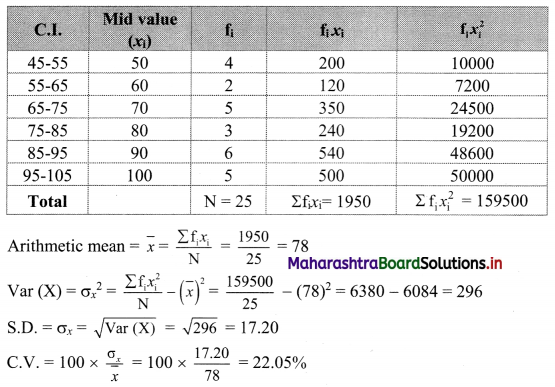
Find n if ⁿP₆ : ⁿP₃ = 120 : 1
Solution:
ⁿP₆ : ⁿP₃ = 120 : 1
∴ \(\frac{n !}{(n-6) !} \div \frac{n !}{(n-3) !}=\frac{120}{1}\)
∴ \(\frac{\mathrm{n} !}{(\mathrm{n}-6) !} \times \frac{(\mathrm{n}-3) !}{\mathrm{n} !}\) = 120
∴ \(\frac{n !}{(n-6) !} \times \frac{(n-3)(n-4)(n-5)(n-6) !}{n !}\) = 120
∴ (n – 3) (n – 4) (n – 5) = 120
∴ (n – 3) (n – 4) (n – 5) = 6 × 5 × 4
Comparing on both sides, we get
n – 3 = 6
∴ n = 9

Question 2.
Find m and n if ^(m+n)P₂ = 56 and ^(m-n)P₂ = 12
Solution:
^m+nP₂ = 56
∴ \(\frac{(\mathrm{m}+\mathrm{n}) !}{(\mathrm{m}+\mathrm{n}-2) !}\) = 56
∴ \(\frac{(m+n)(m+n-1)(m+n-2) !}{(m+n-2) !}\) = 56
∴ (m + n) (m + n – 1) = 8 × 7
Comparing on both sides, we get
m + n = 8 …..(i)
Also ^m-nP₂ = 12
∴ \(\frac{(m-n) !}{(m-n-2) !}\) = 12
∴ \(\frac{(m-n)(m-n-1)(m-n-2) !}{(m-n-2) !}\) = 12
∴ (m – n) (m – n – 1) = 4 × 3
Comparing on both sides, we get
m – n = 4 …..(ii)
Adding (i) and (ii), we get
2m = 12
∴ m = 6
Substituting m = 6 in (ii), we get
6 – n = 4
∴ n = 2

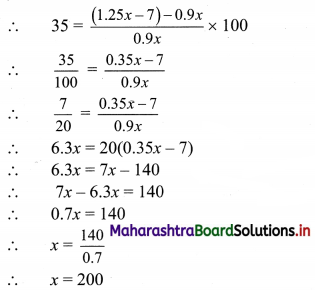
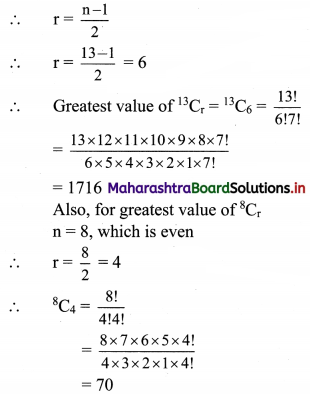
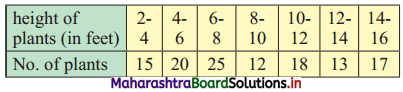
Question 3.
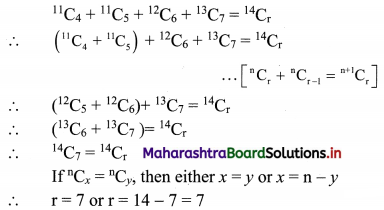
Find r if ¹²P_r-2 : ¹¹P_r-1 = 3 : 14
Solution:

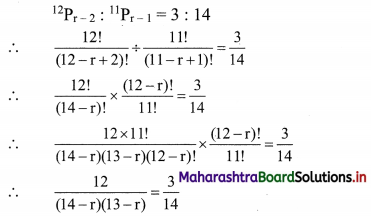
∴ (14 – r)(13 – r) = 8 × 7
Comparing on both sides, we get
14 – r = 8
∴ r = 6

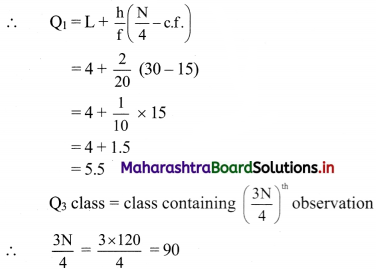
Question 4.
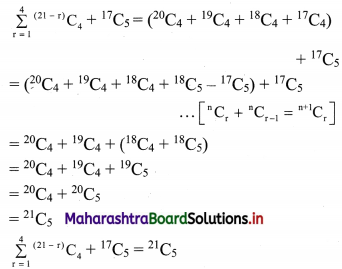
Show that (n + 1) ⁿP_r = (n – r + 1) ⁽ⁿ⁺¹⁾P_r.
Solution:

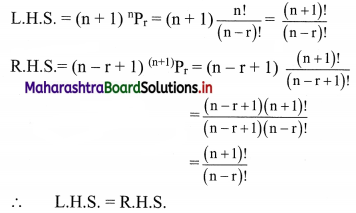
Question 5.
How many 4 letter words can be formed using letters in the word MADHURI if
(i) letters can be repeated?
(ii) letters cannot be repeated?
Solution:
There are 7 letters in the word MADHURI.
(i) A 4 letter word is to be formed from the letters of the word MADHURI and repetition of letters is allowed.
∴ 1st letter can be filled in 7 ways.
2nd letter can be filled in 7 ways.
3rd letter can be filled in 7 ways.
4th letter can be filled in 7 ways.
∴ Total no. of ways a 4-letter word can be formed = 7 × 7 × 7 × 7 = 2401
∴ 2401 four-lettered words can be formed when repetition of letters is allowed.

(ii) When repetition of letters is not allowed, the number of 4-letter words formed from the letters of the word MADHURI is
⁷P₄ = \(\frac{7 !}{(7-4) !}=\frac{7 \times 6 \times 5 \times 4 \times 3 !}{3 !}\) = 840
∴ 840 four-letter words can be formed when repetition of letters is not allowed.

Alternate method:
There are 7 letters in the word MADHURI.
(i) Since letters can be repeated
∴ In all places of a four-letter word, any one of seven letters M, A, D, H, U, R, I can appear.
∴ Using the Multiplication theorem, we get
Number of four-letter words with repetition of letters M, A, D, H, U, R, I = 7 × 7 × 7 × 7 = 2401

(ii) Since the letters cannot be repeated therefore 1st, 2nd, 3rd, 4th places can be filled in 7, 6, 5, 4 ways respectively
∴ Using the multiplication theorem, we get
Number of four-letter words, with no repetition of letters M, A, D, H, U, R, I = 7 × 6 × 5 × 4 = 840

Question 6.
Determine the number of arrangements of letters of the word ALGORITHM if
(i) vowels are always together.
(ii) no two vowels are together.
(iii) Consonants are at even positions
(iv) O is first and T is last.
Solution:
A word is to be formed using the letters of the word ALGORITHM.
There are 9 letters in the word ALGORITHM.
(i) When vowels are always together:
There are 3 vowels in the word ALGORITHM. (i.e, A, I, O)
Let us consider these 3 vowels as one unit.
This unit with 6 other letters is to be arranged.
∴ It becomes an arrangement of 7 things which can be done in ⁷P₇ i.e., 7! ways and 3 vowels can be arranged among themselves in ³P₃ i.e., 3! ways.
∴ the total number of ways in which the word can be formed = 7! × 3!
= 5040 × 6
= 30240
∴ 30240 words can be formed if vowels are always together.

(ii) When no two vowels are together:
There are 6 consonants in the word ALGORITHM.
They can be arranged among themselves in ⁶P₆ i.e., 6! ways.
Let consonants be denoted by C.
_C_C_ C_C_C_C_
6 consonants create 7 gaps in which 3 vowels are to arranged.
∴ 3 vowels can be filled in ⁷P₃
= \(\frac{7 !}{(7-3) !}\)
= \(\frac{7 \times 6 \times 5 \times 4 !}{4 !}\)
= 210 ways
∴ total number of ways in which the word can be formed = 6! × 210
= 720 × 210
= 151200
∴ 151200 words can be formed if no two vowels are together.

(iii) When consonants are at even positions:
There are 4 even places and 6 consonants in the word ALGORITHM.
1st, 2nd, 3rd, 4th even places are filled in 6, 5, 4, 3 way respectively.
∴ The number of ways to fill four even places by consonants = 6 × 5 × 4 × 3 = 360
The remaining 5 letters (3 vowels and 2 consonants) can be arranged among themselves in ⁵P₅ i.e., 5! ways.
∴ Total number of ways the words can be formed
In which even places are occupied by consonants = 360 × 5!
= 360 × 120
= 43200
∴ 43200 words can be formed if even positions are occupied by consonants.

(iv) When beginning with O and ends with T:
All the letters of the word ALGORITHM are to be arranged among themselves such that arrangement begins with O and ends with T.
7 letters other than O and T can be filled between O and T in ⁷P₇ i.e., 7! ways = 5040 ways.
∴ 5040 words beginning with O and ending with T can be formed.

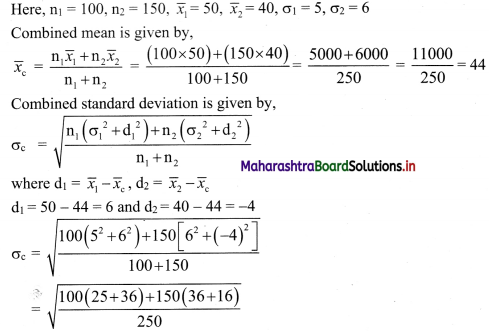
Question 7.
In a group photograph, 6 teachers and principals are in the first row and 18 students are in the second row. There are 12 boys and 6 girls among the students. If the middle position is reserved for the principal and if no two girls are together, find the number of arrangements.
Solution:
In 1st row middle seat is fixed for the principal.
Also 1st row, 6 teachers can be arranged among themselves in ⁶P₆ i.e., 6! ways.
In the 2nd row, 12 boys can be arranged among themselves in ¹²P₁₂ i.e., 12! ways.
13 gaps are created by 12 boys, in which 6 girls are to be arranged.
together which can be done in ¹³P₆ ways.
∴ total number of arrangements = 6! × 12! × ¹³P₆ …..[using Multiplications Principle]
= 6! × 12! × \(\frac{13 !}{(13-6) !}\)
= 6! × 12! × \(\frac{13 !}{7 !}\)
= \(\frac{6 ! \times 12 ! \times 13 !}{7 \times 6 !}\)
= \(\frac{12 ! 13 !}{7}\)

Question 8.
Find the number of ways letters of the word HISTORY can be arranged if
(i) Y and T are together
(ii) Y is next to T.
Solution:
There are 7 letters in the word HISTORY
(i) When ‘Y’ and ‘T’ are together.
Let us consider ‘ Y’ and ‘T’ as one unit
This unit with the other 5 letters is to be arranged.
∴ The number of arrangements of one unit and 5 letters = ⁶P₆ = 6!
Also, ‘Y’ and ‘T’ can be arranged among themselves in ²P₂ i.e., 2! ways.
∴ a total number of arrangements when Y and T are always together = 6! × 2!
= 720 × 2
= 1440
∴ 1440 words can be formed if Y and T are together.

(ii) When ‘Y’ is next to ‘T’
Let us take this (‘Y’ next to ‘T’) as one unit.
This unit with 5 other letters is to be arranged.
∴ The number of arrangements of 6 letters and one unit = ⁶P₆ = 6!
Also ‘Y’ has to be always next to ‘T’.
So they can be arranged in 1 way.
∴ total number of arrangements possible when Y is next to T = 6! × 1 = 720
∴ 720 words can be formed if Y is next to T.

Question 9.
Find the number of arrangements of the letters in the word BERMUDA so that consonants and vowels are in the same relative positions.
Solution:
There are 7 letters in the word “BERMUDA” out of which 3 are vowels and 4 are consonants.
If relative positions of consonants and vowels are not changed.
3 vowels can be arranged among themselves in ³P₃ i.e., 3! ways.
4 consonants can be arranged among themselves in ⁴P₄ i.e., 4! ways.
∴ total no. of arrangements possible if relative positions of vowels and consonants are not changed = 3! × 4!
= 6 × 24
= 144

Question 10.
Find the number of 4-digit numbers that can be formed using the digits 1, 2, 4, 5, 6, 8 if
(i) digits can be repeated
(ii) digits cannot be repeated
Solution:
(i) A 4 digit number is to be made from the digits 1, 2, 4, 5, 6, 8 such that digits can be repeated.
∴ The unit’s place digit can be filled in 6 ways.
10’s place digit can be filled in 6 ways.
100’s place digit can be filled in 6 ways.
1000’s place digit can be filled in 6 ways.
∴ total number of numbers = 6 × 6 × 6 × 6 = 6⁴ = 1296
∴ 1296 four-digit numbers can be formed if repetition of digits is allowed.

(ii) A 4 different digit number is to be made from the digits 1, 2, 4, 5, 6, 8 without repetition of digits.
∴ 4 different digits are to be arranged from 6 given digits which can be done in ⁶P₄
= \(\frac{6 !}{(6-4) !}\)
= \(\frac{6 \times 5 \times 4 \times 3 \times 2 !}{2 !}\)
= 360 ways
∴ 360 four-digit numbers can be formed, if repetition of digits is not allowed.

Question 11.
How many numbers can be formed using the digits 0, 1, 2, 3, 4, 5 without repetition so that the resulting numbers are between 100 and 1000?
Solution:
A number between 100 and 1000 is a 3 digit number and is to be formed from the digits 0, 1, 2, 3, 4, 5, without repetition of digits.
∴ 100’s place digit must be a non-zero number which can be filled in 5 ways.
10’s place digits can be filled in 5 ways.
Unit’s place digit can be filled in 4 ways.
∴ total number of ways the number can be formed = 5 × 5 × 4 = 100
∴ 100 numbers between 100 and 1000 can be formed.

Question 12.
Find the number of 6-digit numbers using the digits 3, 4, 5, 6, 7, 8 without repetition. How many of these numbers are
(i) divisible by 5?
(ii) not divisible by 5?
Solution:
A number of 6 different digits is to be formed from the digits 3, 4, 5, 6, 7, 8 which can be done in ⁶P₆ i.e., 6! = 720 ways
(i) If the number is divisible by 5, then
The unit’s place digit must be 5, and hence unit’s place can be filled in 1 way
Other 5 digits can be arranged among themselves in ⁵P₅ i.e., 5! ways
∴ Total number of ways in which numbers divisible by 5 can be formed = 1 × 5! = 120

(ii) If the number is not divisible by 5, then
Unit’s place can be any digit from 3, 4, 6, 7, 8 which can be selected in 5 ways.
Other 5 digits can be arranged in ⁵P₅ i.e., 5! ways
∴ The total number of ways in which numbers not divisible by 5 can be formed = 5 × 5!
= 5 × 120
= 600

Question 13.
A code word is formed by two distinct English letters followed by two non-zero distinct digits. Find the number of such code words. Also, find the number of such code words that end with an even digit.
Solution:
(i) There is a total of 26 alphabets.
A code word contains 2 English alphabets.
∴ 2 alphabets can be filled in ²⁶P₂
= \(\frac{26 !}{(26-2) !}\)
= \(\frac{26 \times 25 \times 24 !}{24 !}\)
= 650 ways
Also, alphabets to be followed by two distinct non-zero digits from 1 to 9 which can be filled in ⁹P₂
= \(\frac{9 !}{(9-2) !}\)
= \(\frac{9 \times 8 \times 7 !}{7 !}\)
= 72 ways
∴ Total number of a code words = 650 × 72 = 46800

(ii) There are total 26 alphabets.
A code word contains 2 English alphabets.
∴ 2 alphabets can be filled in ²⁶P₂
= \(\frac{26}{(26-2) !}\)
= \(\frac{26 \times 25 \times 24 !}{24 !}\)
= 650 ways
For a code word to end with an even integer, the digit in the unit’s place should be an even number between 1 to 9 which can be filled in 4 ways.
Also, 10’s place can be filled in 8 ways.
∴ Total number of codewords = 650 × 4 × 8 = 20800 ways
∴ 20800 codewords end with an even integer.

Question 14.
Find the number of ways in which 5 letters can be posted in 3 post boxes if any number of letters can be posted in a post box.
Solution:
There are 5 letters and 3 post boxes and any number of letters can be posted in all three post boxes.
∴ Each letter can be posted in 3 ways.
∴ Total number of ways in which 5 letters can be posted = 3 × 3 × 3 × 3 × 3 = 243

Question 15.
Find the number of arranging 11 distinct objects taken 4 at a time so that a specified object
(i) always occurs
(ii) never occurs
Solution:
There are 11 distinct objects and 4 are to be taken at a time.
(i) The number of permutations of n distinct objects, taken r at a time, when one specified object will always occur is r × ^(n-1)P_(r-1)
Here, r = 4, n = 11
∴ The number of permutations of 4 out of 11 objects when a specified object occurs.
= 4 × ^(11-1)P_(4-1)
= 4 × ¹⁰P₃
= 4 × \(\frac{10 !}{(10-3) !}\)
= 4 × \(\frac{10 !}{7 !}\)
= 4 × \(\frac{10 \times 9 \times 8 \times 7 !}{7 !}\)
= 2880
∴ There are 2880 permutations of 11 distinct objects, taken 4 at a time, in which one specified object always occurs.

(ii) When one specified object does not occur then 4 things are to be arranged from the remaining 10 things, which can be done in ¹⁰P₄ ways
= 10 × 9 × 8 × 7 ways
= 5040 ways
∴ There are 5040 permutations of 11 distinct objects, taken 4 at a time, in which one specified object never occurs.

11th Commerce Maths Digest Pdf

• 11th Commerce Maths Exercise 6.1 Solutions
• 11th Commerce Maths Exercise 6.2 Solutions
• 11th Commerce Maths Exercise 6.3 Solutions
• 11th Commerce Maths Exercise 6.4 Solutions
• 11th Commerce Maths Exercise 6.5 Solutions
• 11th Commerce Maths Exercise 6.6 Solutions
• 11th Commerce Maths Exercise 6.7 Solutions
• 11th Commerce Maths  Miscellaneous Exercise 6 Solutions

Linear Inequations Class 11 Commerce Maths 2 Chapter 8 Exercise 8.1 Answers Maharashtra Board

Balbharati Maharashtra State Board 11th Commerce Maths Solution Book Pdf Chapter 8 Linear Inequations Ex 8.1 Questions and Answers.

Std 11 Maths 2 Exercise 8.1 Solutions Commerce Maths

Question 1.
Write the inequations that represent the interval and state whether the interval is bounded or unbounded:
(i) [-4, \(\frac{7}{3}\)]
Solution:
[-4, \(\frac{7}{3}\)]
Here, x takes values between -4 and \(\frac{7}{3}\) including -4 and \(\frac{7}{3}\)
∴ the required inequation is -4 ≤ x ≤ \(\frac{7}{3}\)
∴ it is a bounded (closed) interval.

(ii) (0, 0.9]
Solution:
(0, 0.9]
Here, x takes values between 0 and 0.9, including 0.9 and excluding 0.
∴ the required inequation is 0 < x ≤ 0.9
∴ it is a bounded (semi-right closed) interval.

(iii) (-∞, ∞)
Solution:
(-∞, ∞)
Here, x takes values between -∞ and ∞
∴ the required inequation is -∞ < x < ∞
∴ it is an unbounded (open) interval.

(iv) [5, ∞)
Solution:
[5, ∞)
Here, x takes values between 5 and ∞ including 5.
∴ the required inequation is 5 ≤ x < ∞
∴ it is an unbounded (semi-left closed) interval.

(v) (-11, -2)
Solution:
(-11, -2)
Here, x takes values between -11 and -2
∴ the required inequation is -11 < x < -2
∴ it is a bounded (open) interval.

(vi) (-∞, 3)
Solution:
(-∞, 3)
Here, x takes values between -∞ and 3
∴ the required inequation is -∞ < x < 3
∴ it is an unbounded (open) interval.

Question 2.
Solve the following inequations
(i) 3x – 36 > 0
Solution:
3x – 36 > 0
Adding 36 both sides, we get
3x – 36 + 36 > 0 + 36
∴ 3x > 36
Dividing both sides by 3, we get
\(\frac{3 x}{3}>\frac{36}{3}\)
∴ x > 12
∴ x takes all real values more than 12
∴ Solution set = (12, ∞)

(ii) 7x – 25 ≤ -4
Solution:
7x – 25 ≤ -4
Adding 25 on both sides, we get
7x – 25 + 25 ≤ -4 + 25
∴ 7x ≤ 21
Dividing both sides by 7, we get
x ≤ 3
∴ x takes all real values less or equal to 3.
∴ Solution Set = (-∞, 3]

(iii) 0 < \(\frac{x-5}{4}\) < 3
Solution:
0 < \(\frac{x-5}{4}\) < 3
0 < x – 5 < 12
Adding 5 on both sides, we get
5 < x < 17
x takes all real values between 5 and 17.
∴ Solution set = (5, 17)

(iv) |7x – 4| < 10
Solution:
|7x – 4| < 10
-10 < 7x – 4 < 10 …….[|x| < k is same as -k < x < k]
Adding 4 on both sides, we get
-6 < 7x < 14
Dividing both sides by 7, we get
\(-\frac{6}{7}<x<\frac{14}{7}\)
∴ \(-\frac{6}{7}\) < x < 2
∴ x takes all real values between \(-\frac{6}{7}\) and 2.
∴ Solution set = (\(-\frac{6}{7}\), 2)

Question 3.
Sketch the graph which represents the solution set for the following inequations:
(i) x > 5
Solution:
x > 5
Here, x takes all real values that are greater than 5.
∴ Solution set represents the unbounded (open) interval (5, ∞)
∴ the required graph of the solution set is as follows:

(ii) x ≥ 5
Solution:
x ≥ 5
Here, x takes all real values that are greater than or equal to 5
∴ Solution set represents the unbounded (semi-left closed) interval [5, ∞)
∴ the required graph of the solution set is as follows:

(iii) x < 3
Solution:
x < 3
Here, x takes all real values that are less than 3.
∴ Solution set represents the unbounded (open) interval (-∞, 3)
∴ the required graph of the solution set is as follows:

(iv) x ≤ 3
Solution:
x ≤ 3
Here, x takes all real values less than and including 3
∴ Solution set represents the unbounded (semi-right closed) interval (-∞, 3]
∴ the required graph of the solution set is as follows:

(v) -4 < x < 3
Solution:
-4 < x < 3
Here, x takes all real values between -4 and 3.
∴ Solution set represents the bounded (open) interval (-4, 3)
∴ the required graph of the solution set is as follows:

(vi) -2 ≤ x < 2.5
Solution:
-2 ≤ x < 2.5
Here, x takes all values between -2 and 2.5 including -2.
∴ Solution set represents the bounded (semi-left closed) interval [-2, 2.5)
∴ the required graph of the solution set is as follows.

(vii) -3 ≤ x ≤ 1
Solution:
-3 ≤ x ≤ 1
Here, x takes all real values between -3 and 1 including -3 and 1
∴ Solution set represents the bounded (closed) interval [-3, 1]
∴ the required graph of the solution set is as follows:

(viii) |x| < 4
Solution:
|x| < 4 ⇒ -4 < x < 4
Here, x takes all real values between -4 and 4.
∴ Solution set represents bounded (open) interval (-4, 4)
∴ the required graph of the solution set is as follows:

(ix) |x| ≥ 3.5
Solution:
|x| ≥ 3.5 ⇒ x ≥ 3.5 or x ≤ -3.5
Here, x takes values greater than or equal to 3.5 or it takes values less than or equal to -3.5
∴ Solution set represents the unbounded (semi-left closed) interval [3.5, ∞) or the unbounded (semi-right closed) interval (-∞, -3.5]
∴ x ∈ (-∞, -3.5] ∪ [3.5, ∞)
∴ the required graph of the solution set is as follows:

Question 4.
Solve the inequations:
(i) 5x + 7 > 4 – 2x
Solution:
5x + 7 > 4 – 2x
Adding 2x on both sides, we get
7x + 7 > 4
Subtracting 7 from both sides, we get
7x > -3
Dividing by 7 on both sides, we get
∴ x > \(-\frac{3}{7}\)
i.e., x takes all real values greater than \(-\frac{3}{7}\)
∴ the solution set is (\(-\frac{3}{7}\), ∞)

(ii) 3x + 1 ≥ 6x – 4
Solution:
3x + 1 ≥ 6x – 4
Subtracting 3x from both sides, we get
1 ≥ 3x – 4
Adding 4 on both sides, we get
5 ≥ 3x
Dividing by 3 on both sides, we get
\(\frac{5}{3}\) ≥ x
i.e., x ≤ \(\frac{5}{3}\)
i.e., x takes all real values less than or equal to \(\frac{5}{3}\).
∴ the solution set is (-∞, \(\frac{5}{3}\)]

(iii) 4 – 2x < 3(3 – x)
Solution:
4 – 2x < 3(3 – x)
∴ 4 – 2x < 9 – 3x
Adding 3x on both sides, we get
4 + x < 9
Subtracting 4 from both sides, we get
x < 5
i.e., x takes all real values less than 5
∴ the solution set is (-∞, 5)

(iv) \(\frac{3}{4}\)x – 6 ≤ x – 7
Solution:
\(\frac{3}{4}\)x – 6 ≤ x – 7
Multiplying by 4 on both sides, we get
3x – 24 ≤ 4x – 28
Subtracting 3x from both sides, we get
-24 ≤ x – 28
Adding 28 on both the sides, we get
∴ 4 ≤ x i.e., x ≥ 4
i.e., x takes all real values greater or equal to 4.
∴ the solution set is [4, ∞)

(v) -8 ≤ -(3x – 5) < 13
Solution:
-8 < -(3x – 5) < 13 Multiplying by -1 throughout (so inequality sign changes) 8 ≥ 3x – 5 > -13
i.e., -13 < 3x – 5 ≤ 8
Adding 5 on both the sides, we get
-8 < 3x ≤ 13
Dividing, by 3 on both sides, we get
∴ \(-\frac{8}{3}\) < x ≤ \(\frac{13}{3}\)
i.e., x takes all real values between \(-\frac{8}{3}\) and \(\frac{13}{3}\) including \(\frac{13}{3}\).
∴ the solution set is \(\left(-\frac{8}{3}, \frac{13}{3}\right]\)

(vi) -1 < 3 – \(\frac{x}{5}\) ≤ 1
Solution:
-1 < 3 – \(\frac{x}{5}\) ≤ 1
Subtracting 3 from both sides, we get
-4 < –\(\frac{x}{5}\) < -2 Multiplying by -1 throughout (so inequality sign changes) ∴ 4 > \(\frac{x}{5}\) > 2
i.e., 2 < \(\frac{x}{5}\) < 4
Multiplying by 5 on both sides, we get
10 < x < 20
i.e., x takes all real values between 10 and 20.
∴ the solution set is (10, 20)

(vii) 2|4 – 5x| ≥ 9
Solution:
2|4 – 5x | ≥ 9
∴ |4 – 5x| ≥ \(\frac{9}{2}\)
∴ 4 – 5x ≥ \(\frac{9}{2}\) or 4 – 5x ≤ –\(\frac{9}{2}\) ……[|x| ≥ a implies x ≤ -a or x ≥ a]
Subtracting 4 from both sides, we get
-5x ≥ \(\frac{1}{2}\) or -5x ≤ \(\frac{-17}{2}\)
Divide by -5 (so inequality sign changes)
∴ x ≤ \(-\frac{1}{10}\) or x ≥ \(\frac{17}{10}\)
∴ x takes all real values less than or equal to \(-\frac{1}{10}\)
or it takes all real values greater or equal to \(\frac{17}{10}\).
∴ the solution set is (-∞, \(-\frac{1}{10}\)] or [\(\frac{17}{10}\), ∞)

(viii) |2x + 7| ≤ 25
Solution:
|2x + 7| < 25
∴ -25 ≤ 2x + 7 ≤ 25 …..[|x| ≤ a implies -a ≤ x ≤ a]
Subtracting 7 from both sides, we get
-32 ≤ 2x ≤ 18
Dividing by 2 on both sides, we get
-16 ≤ x ≤ 9
∴ x can take all real values between -16 and 9 including -16 and 9.
∴ the solution set is [-16, 9]

(ix) 2|x + 3| > 1
Solution:
2|x + 3| > 1
Dividing by 2 on both sides, we get
|x + 3| > \(\frac{1}{2}\)
∴ x + 3 < –\(\frac{1}{2}\) or x + 3 > \(\frac{1}{2}\) …..[|x| > a implies x < -a or x > a]
Subtracting 3 from both sides, we get
x < – 3 – \(\frac{1}{2}\) or x > -3 + \(\frac{1}{2}\)
∴ x < \(\frac{-7}{2}\) or x > \(\frac{-5}{2}\)
∴ x can take all real values less \(\frac{-7}{2}\) or it can take values greater than \(\frac{-5}{2}\).
∴ Solution set is (-∞, \(\frac{-7}{2}\)) ∪ (\(\frac{-7}{2}\), ∞)

(x) \(\frac{x+5}{x-3}\) < 0
Solution:
\(\frac{x+5}{x-3}\) < 0
Since \(\frac{a}{b}\) < 0, when a > 0 and b < 0 or a < 0 and b > 0
∴ either x + 5 > 0 and x – 3 < 0
or x + 5 < 0 and x – 3 > 0
Case I:
x + 5 > 0 and x – 3 < 0 ∴ x > -5 and x < 3
∴ -5 < x < 3
∴ solution set = (-5, 3)
Case II:
x + 5 < 0 and x – 3 > 0
∴ x < -5 and x > 3
which is not possible
∴ solution set = Φ
∴ solution set of the given inequation is (-5, 3)

(xi) \(\frac{x-2}{x+5}\) > 0
Solution:
\(\frac{x-2}{x+5}\) > 0
Since \(\frac{a}{b}\) > 0,
when a > 0 and b > 0 or a < 0 and b < 0 b
∴ either x – 2 > 0 and x + 5 > 0
or x – 2 < 0 and x + 5 < 0 Case I: x – 2 > 0 and x + 5 > 0
∴ x > 2 and x > -5
∴ x > 2
∴ solution set = (2, ∞)
Case II:
x – 2 < 0 and x + 5 < 0
∴ x < 2 and x < -5
∴ x < -5
∴ solution set = (-∞, -5)
∴ the solution set of the given inequation is (-∞, -5) ∪ (2, ∞)

Question 5.
Rajiv obtained 70 and 75 marks in the first two unit tests. Find the minimum marks he should get in the third test to have an average of at least 60 marks.
Solution:
Let x₁, x₂, x₃ denote the marks in 1st, 2nd and 3rd unit test respectively. Then
\(\frac{x_{1}+x_{2}+x_{3}}{3}\) ≥ 60
∴ \(\frac{70+75+x_{3}}{3}\) ≥ 60
∴ 145 + x₃ ≥ 3(60)
Subtracting 145 from both sides, we get
x₃ ≥ 180 – 145
∴ x₃ ≥ 35
Rajiv must obtain a minimum of 35 marks to maintain an average of at least 60 marks.

Question 6.
To receive Grade ‘A’ in a course, one must obtain an average of 90 marks or more in five examinations (each of 100 marks). If Sunita’s marks in the first four examinations are 87, 92, 94, and 95, find the minimum marks that Sunita must obtain in the fifth examination to get a grade ‘A’ in the course.
Solution:
Let x₁, x₂, x₃, x₄, x₅ denote the marks in five examinations. Then
\(\frac{x_{1}+x_{2}+x_{3}+x_{4}+x_{5}}{5}\) ≥ 90
∴ \(\frac{87+92+94+95+x_{5}}{5}\) ≥ 90
∴ 368 + x₅ ≥ 450
Subtracting 368 from both sides, we get
∴ x₅ ≥ 82
Sunita must obtain a minimum of 82 marks in the 5th examination to get a grade of A.

Question 7.
Find all pairs of consecutive odd positive integers, both of which are smaller than 10 such that their sum is more than 11.
Solution:
Let two consecutive positive integers be 2n – 1, 2n + 1 where n ≥ 1 ∈ Z,
Given that 2n – 1 < 10 and 2n + 1 < 10
∴ 2n < 11 and 2n < 9
∴ 2n < 9
∴ n < \(\frac{9}{2}\) …..(i) Also, (2n – 1) + (2n + 1) > 11
∴ 4n > 11
∴ n > \(\frac{11}{4}\) …….(ii)
From (i) and (ii)
\(\frac{11}{4}<n<\frac{9}{2}\) Since, n is an integer,
∴ n = 3, 4
n = 3 gives 2n – 1 = 5, 2n + 1 = 7
and n = 4 gives 2n – 1 = 7, 2n + 1 = 9
∴ The pairs of positive consecutive integers are (5, 7) and (7, 9).

Question 8.
Find all pairs of consecutive even positive integers, both of which are larger than 5 such that their sum is less than 23.
Solution:
Let 2n, 2n + 2 be two positive consecutive integers where n ≥ 1 ∈ Z.
Given that 2n > 5 and 2n + 2 > 5
∴ n > \(\frac{5}{2}\) and 2n > 3
∴ n > \(\frac{5}{2}\) and n > \(\frac{3}{2}\)
∴ n > \(\frac{5}{2}\) ……(i)
Also (2n) + (2n + 2) < 23
∴ 4n + 2 < 23
∴ 4n < 21
∴ n < \(\frac{21}{4}\) ……(ii)
From (i) and (ii)
\(\frac{5}{2}<n<\frac{21}{4}\) and n is an integer.
∴ n = 3, 4, 5
n = 3 gives 2n = 6, 2n + 2 = 8
n = 4 gives 2n = 8, 2n + 2 = 10
n = 5 gives 2n = 10, 2n + 2 = 12
∴ The pairs of positive even consecutive integers are (6, 8) (8, 10), (10, 12)

Question 9.
The longest side of a triangle is twice the shortest side and the third side is 2 cm longer than the shortest side. If the perimeter of the triangle is more than 166 cm then find the minimum integer length of the shortest side.
Solution:
Let the shortest side be x.
Then longest side length = 2x
and third side length = x + 2
Perimeter = x + 2x + x + 2 = 4x +2
Given, perimeter > 166
∴ 4x + 2 > 166
∴ 4x > 164
∴ x > 41
∴ Minimum integer length of shortest side is 42 cm.

11th Commerce Maths Digest Pdf

• 11th Commerce Maths Exercise 8.1 Solutions
• 11th Commerce Maths Exercise 8.2 Solutions
• 11th Commerce Maths Exercise 8.3 Solutions
• 11th Commerce Maths Miscellaneous Exercise 8 Solutions

Commercial Mathematics Class 11 Commerce Maths 2 Chapter 9 Exercise 9.2 Answers Maharashtra Board

Balbharati Maharashtra State Board 11th Commerce Maths Solution Book Pdf Chapter 9 Commercial Mathematics Ex 9.2 Questions and Answers.

Std 11 Maths 2 Exercise 9.2 Solutions Commerce Maths

Question 1.
Mr. Sarad purchased a laptop for ₹ 24,000 and sold it for ₹ 30,000. What was the profit percentage?
Solution:
Cost price (C.P.) = ₹ 24000
Selling price (S. P.) = ₹ 30,000
Profit = S.P. – C.P.
= 30,000 – 24,000
= 6,000

∴ Profit Percentage = 25%

Question 2.
Shraddha purchased a mobile phone and refrigerator for ₹ 18,000 and ₹ 15,000 respectively. She sold the refrigerator at a loss of 20% and the mobile at a profit of 20%. What is her overall profit or loss?
Solution:
C.P. of mobile phone = ₹ 18,000
Profit percentage on mobile phone = 20%
Selling price (S.P.) of mobile phone = 18,000 (1 + \(\frac{20}{100}\))
= 18,000 (1 + \(\frac{1}{5}\))
= 18,000 × \(\frac{6}{5}\)
= ₹ 21,600
C.P. of refrigerator = 15,000
Loss percentage on refrigerator = 20%
∴ Selling price (S.P.) = 15,000(1 – \(\frac{20}{100}\))
= 15,000(1 – \(\frac{1}{5}\))
= 15,000 × \(\frac{4}{5}\)
= ₹ 12,000
∴ Total gelling price for the transaction = 21,600 + 12,000 = ₹ 33,600
Total cost price (purchase price) for the transaction = 18,000 + 15,000 = ₹ 33,000
∴ Overall profit made by Shraddha = Total S.P. – Total C.P.
= 33,600 – 33,000
= ₹ 600
Thus, Shraddha made on overall profit of ₹ 600.

Question 3.
A vendor bought toffees at 6 for ₹ 10. How many for ₹ 10 must he sell to gain 20%?
Solution:
Vendor bought toffees at the rate of 6 for ₹ 10
∴ Cost price of one toffee = \(\frac{10}{6}\)
i.e. C.P. = \(\frac{10}{6}\) …….(i)
Let x be the number of toffees he must sell in ₹ 10 to gain 20%
i.e. S.P. = \(\frac{10}{x}\) …….(ii)
Profit percentage = \(\frac{\text { S.P. }-\text { C.P. }}{\text { C.P. }}\)
Using (i) and (ii) we have

∴ 30(6 – x) = 6x
∴ 180 – 30x = 6x
∴ 36x = 180
∴ x = 5
The vendor must sell 5 toffees for ₹ 10 in order to gain 20%.

Question 4.
The percentage profit earned by selling an article for ₹ 2,880 is equal to the percentage loss incurred by selling the same article for ₹ 1,920. At what price the article should be sold to earn a 25% profit?
Solution:
Let x be C.P. of the article
Let y % be both, the gain and loss made when article is sold at ₹ 2,880 and ₹ 1,920 respectively. Then
x + \(\frac{y}{100}\) x = 2880 ……(i)
x – \(\frac{y}{100}\) x = 1920 …..(ii)
Adding (i) and (ii), we get
2x = 4800
∴ x = 2400
i.e. C.P. of the article = ₹ 2400
Required profit percentage = 25%

∴ The article should be sold at ₹ 3000 to earn 25% profit.

Question 5.
A cloth merchant advertises for selling cloth at a 4% loss. By using a faulty meter scale, he is earning a profit of 20%. What is the actual length of the scale?
Solution:
Let the cost price of the cloth be ₹ ‘x’ per meter
He claims a loss of 4%
∴ Selling price of the cloth
S.P. = C.P.(1 – \(\frac{loss%}{100}\))
= x(1 – \(\frac{4}{100}\))
= 0.96x …..(i)
The actual cost price of the cloth is lower as the cloth is measured by a faulty meter scale.
Given that shopkeeper’s profit = 20%

∴ The actual cost price is 0.8 times the cost price as advertised.
In other words, the meter scale used for the fraud is 0.8 times the meter scale that should have been used.
∴ The length of the faulty meter scale used = 0.8 × 1 = 0.8 meter
∴ The actual length of the scale is 0.8 meters.

Question 6.
Sunil sells his bike worth ₹ 25,000 to Rohit at a profit of 20%. After 6 months Rohit sells the bike back to Sunil at a loss of 20%. Find the total profit percent of Sunil considering both the transactions.
Solution:
Sunil sells his bike to Rohit at 20% profit.
So S.P. of the bike for Sunil
= 25000 + \(\frac{20}{100}\) × 25000
= 25000 + 5000
= 30000
∴ Cost price of bike to Rohit = ₹ 30000
Rohit sells the bike back to Sunil at 20% loss
∴ S.P. of the bike for Rohit = 30000 – \(\frac{20}{100}\) × 30000
= 30000 – 6000
= 24000
∴ In second transaction Sunil pays 24000 to Rohit
In the first transaction, he had received 30000 from Rohit
∴ Sunil made a profit of ₹ (30000 – 24000) = ₹ 6000
Sunil earned this profit on the bike which costed him ₹ 25000
∴ Total profit % that Sunil makes = \(\frac{6000}{25000}\) × 100
= \(\frac{600}{25}\)
= 24
∴ Sunil makes 24% profit considering both the transactions.

Question 7.
By selling a book at ₹ 405 bookseller incurs a loss of 25%. Find the cost price of the book.
Solution:
S.P. = ₹ 405
Loss% = 25
S.P. when there is a loss is given by
S.P. = C.P. × \(\frac{\text { Loss } \%}{100}\)

∴ The cost price of the book is ₹ 540.

Question 8.
A cloth costs ₹ 675. If it is sold at a loss of 20%, what is its cost price as a percentage of its selling price?
Solution:
C.P. = ₹ 675
Loss% = 20%
∴ Loss made in selling = \(\frac{20}{100}\) × 675 = ₹ 135
S.P. = C.P. – Loss
= 675 – 135
= ₹ 540
Let C.P. be x % S.P.,
Then 675 = \(\frac{x}{100}\) × 540
∴ x = \(\frac{675 \times 100}{540}\) = 125
∴ Cost price is 125% of the selling price.

Question 9.
Ashwin buys an article for ₹ 500. He marks it for sale at 75% more than the cost price. He offers a 25% discount on the marked price to his customer. Calculate the actual percentage of profit made by Ashwin.
Solution:
C.P. = ₹ 500
Marked price = C.P. + \(\frac{75}{100}\) × C.P.
= \(\frac{75}{100}\) × 500
= 500 + 75 × 5
= 500 + 375
= 875
25% discount was given on marked price
∴ Discount = \(\frac{25}{100}\) × 875 = \(\frac{875}{4}\)
Selling price = marked price – discount

∴ Ashwin makes 31.25% profit.

Question 10.
The combined cost price of a refrigerator and a mixer is ₹ 12,400. If the refrigerator costs 600% more than the mixer, find the cost price of the mixer.
Solution:
Let ₹ x be the cost price of the mixer.
The cost price of the refrigerator = x + \(\frac{600}{100}\) x
= x + 6x
= 7x
Total cost price =12400 …..[Given]
i.e. x + 7x = 12400
i.e. 8x = 12400
∴ x = \(\frac{12400}{8}\) = 1550
∴ The cost price of mixer is ₹ 1550.

Question 11.
Find the single discount equivalent to the discount series of 5%, 7%, and 9%.
Solution:
Let the marked price be ₹ 100
After 1st discount the price = 100(1 – \(\frac{5}{100}\)) = 95
After 2nd discount the price = 95(1 – \(\frac{7}{100}\)) = \(\frac{95 \times 93}{100}\)
After 3rd discount the price = \(\frac{95 \times 93}{100}\left(1-\frac{9}{100}\right)\)
= \(\frac{95 \times 93 \times 91}{100 \times 100}\)
= \(\frac{803985}{10000}\)
= 80.3985 ~ 80.4
Selling price after 3 discounts is ₹ 80.4
Single equivalent discount = Marked price – Selling price
= 100 – 80.4
= ₹ 19.6
∴ Single equivalent discount is 19.6%.

Question 12.
The printed price of a shirt is ₹ 390. Lokesh pays ₹ 175.50 for it after getting two successive discounts. If the first discount is 10%, find the second discount.
Solution:
Marked price = ₹ 390
After the first discount of 10%, the price of the shirt

∴ x = 50
∴ Second discount is 50%

Question 13.
Amar, a manufacturer, gives a discount of 25% on the list price to his distributor Akbar, Akbar sells at a 10% discount on the list price to his customer Anthony. Anthony paid ₹ 540 for the article. What is the profit percentage of Akbar on his cost price?
Solution:
Let ₹ ‘x’ be the list price of the article.
Amar gives a discount of 25% on the list price.
∴ Selling price for Amar = \(x\left(1-\frac{25}{100}\right)\)
= \(x\left(1-\frac{1}{4}\right)\)
= ₹ \(\frac{3 x}{4}\)
Amar sells the article to Akbar
Cost price of article for Akbar = ₹ \(\frac{3 x}{4}\) ……(i)
Akbar sells the article to Anthony at 10% discount on list price
∴ Selling price for Akbar = \(x\left(1-\frac{10}{100}\right)\)
= \(x\left(1-\frac{1}{10}\right)\)
= ₹ \(\frac{9 x}{10}\) …..(ii)
Profit percentage = \(\frac{\text { S.P. }-\text { C.P. }}{\text { C.P. }} \times 100\)
Using (i) and (ii), we have the profit percentage for Akbar as,

∴ Akbar gets a profit of 20% on his cost price.

Question 14.
A man sells an article at a profit of 25%. If he had bought it at a 10% loss and sold it for ₹ 7 less, he would have gained 35%. Find the cost price of the article.
Solution:
Let ₹ ‘x’ be the C.P. of the article
∴ Article was sold at 25% profit
∴ S.P. of the article = \(x\left(1+\frac{25}{100}\right)\)
= \(x\left(1+\frac{1}{4}\right)\)
= 1.25x
If the article was bought at 10% loss
i.e., the new C.P. = \(x\left(1-\frac{10}{100}\right)\)
= \(x\left(\frac{9}{10}\right)\)
= 0.9x
and sold at ₹ 7 less
∴ New S.P. = 1.25x – 7
Then, the profit would have been 35%
Using profit percentage = \(\frac{\text { S.P.-C.P. }}{\text { C.P. }} \times 100\)

∴ Cost price of the article is ₹ 200

Question 15.
Mr. Mehta sold his two luxury cars at ₹ 39,10,000 each. On one he gains 15% but on the other, he loses 15%. How much does he gain or lose in the whole transaction?
Solution:
Let x, y be the C.P. of two cars.
S.P. of both the cars = 39,10,000 …..[Given]
∴ One car is sold at 15% loss
∴ S.P. of the first car = x – \(\frac{15}{100}\)x
∴ \(\frac{85}{100}\)x = 39,10,000
∴ x = \(\frac{39,10,000 \times 100}{85}\)
∴ x = 46,000 × 100
∴ x = 46,00,000
Other car is sold at 15% gain
∴ S.P. of second car = y + \(\frac{15}{100}\) y
∴ y + \(\frac{15}{100}\) y = 39,10,000
∴ \(\frac{115}{100}\)y = 39,10,000
∴ y = \(\frac{39,10,000 \times 100}{115}\)
∴ y = 34,000 × 100
∴ y = 34,00,000
x + y = Total C.P. of two cars
= 46,00,000 + 34,00,000
= 80,00,000
Total S.P. = 39,10,000 + 39,10,000 = 78,20,000
∴ S.P. < C.P.
∴ There is a loss of ₹ (80,00,000 – 78,20,000) = ₹ 1,80,000
∴ Loss % = \(\frac{1,80,000}{80,00,000} \times 100\)
= \(\frac{18}{8}\)
= 2.25
∴ Mr. Mehta bears a 2.25% loss in the whole transaction.

11th Commerce Maths Digest Pdf

• 11th Commerce Maths Exercise 9.1 Solutions
• 11th Commerce Maths Exercise 9.2 Solutions
• 11th Commerce Maths Exercise 9.3 Solutions
• 11th Commerce Maths Exercise 9.4 Solutions
• 11th Commerce Maths Exercise 9.5 Solutions
• 11th Commerce Maths Exercise 9.6 Solutions
• 11th Commerce Maths Exercise 9.7 Solutions
• 11th Commerce Maths Miscellaneous Exercise 9 Solutions

Measures of Dispersion Class 11 Commerce Maths 2 Chapter 2 Exercise 2.3 Answers Maharashtra Board

Balbharati Maharashtra State Board 11th Commerce Maths Solution Book Pdf Chapter 2 Measures of Dispersion Ex 2.3 Questions and Answers.

Std 11 Maths 2 Exercise 2.3 Solutions Commerce Maths

Question 1.
The mean and standard deviation of two distributions of 100 and 150 items are 50, 5, and 40, 6 respectively. Find the mean and standard deviation of all the 250 items taken together.
Solution:


∴ The mean and standard deviation of all 250 items taken together are 44 and √55.6 respectively.

Question 2.
For certain bivariate data, the following information is available.

Obtain the combined standard deviation.
Solution:

Question 3.
Calculate the coefficient of variation of marks secured by a student in the exam, where the marks are: 2, 4, 6, 8, 10. (Given: √8 = 2.8284)
Solution:

Question 4.
Find the coefficient of variation of a sample that has a mean equal to 25 and a standard deviation of 5.
Solution:
Given, \(\bar{x}\) = 25, σ = 5
C.V. = 100 × \(\frac{\sigma}{\bar{x}}\)
= 100 × \(\frac{5}{25}\)
= 20%

Question 5.
A group of 65 students of class XI has their average height as 150.4 cm with a coefficient of variation of 2.5%. What is the standard deviation of their height?
Solution:
Given, n = 65, \(\bar{x}\) = 150.4, C.V. = 2.5%
C.V. = 100 × \(\frac{\sigma}{\bar{x}}\)
∴ 2.5 = 100 × \(\frac{\sigma}{150.4}\)
∴ \(\frac{2.5 \times 150.4}{100}\) = σ
∴ σ = 3.76
∴ the standard deviation of students’ height is 3.76.

Question 6.
Two workers on the same job show1 the following results:

(i) Regarding the time required to complete the job, which worker is more consistent?
(ii) Which worker seems to be faster in completing the job?
Solution:

(i) Since, C.V. (P) < C.V.(Q)
∴ Worker P is more consistent regarding the time required to complete the job.

(ii) Since, \(\bar{p}\) > \(\bar{q}\)
i.e., the expected time for completing the job is less for worker Q.
∴ Worker Q seems to be faster in completing the job.

Question 7.
A company has two departments with 42 and 60 employees respectively. Their average weekly wages are ₹ 750 and ₹ 400. The standard deviations are 8 and 10 respectively.
(i) Which department has a larger bill?
(ii) Which department has larger variability in wages?
Solution:

(i) Since, \(\bar{x}_{1}>\bar{x}_{2}\)
i.e., average weekly wages are more for the first department.
∴ the first department has a larger bill.
(ii) Since, C.V. (1) < C.V. (2)
∴ the second department is less consistent.
∴ the second department has larger variability in wages.

Question 8.
The following table gives the weights of the students of class A. Calculate the coefficient of variation (Given √8 = 0.8944)

Solution:

Question 9.
Compute coefficient of variation for team A and team B. (Given: √2.5162 = 1.5863, √2.244 = 1.4980)

Which team is more consistent?
Solution:
Let f₁ denote no. of goals of team A and f₂ denote no. of goals of team B.


Since C.V. of team A > C.V. of team B.
∴ team B is more consistent.

Question 10.
Given below is the information about marks obtained in Mathematics and Statistics by 100 students in a class. Which subject shows the highest variability in marks?

Solution:

Since C.V. (S) > C.V. (M)
∴ The subject statistics show higher variability in marks.

11th Commerce Maths Digest Pdf

• 11th Commerce Maths Exercise 2.1 Solutions
• 11th Commerce Maths Exercise 2.2 Solutions
• 11th Commerce Maths Exercise 2.3 Solutions
• 11th Commerce Maths Miscellaneous Exercise 2 Solutions

Permutations and Combinations Class 11 Commerce Maths 2 Chapter 6 Exercise 6.7 Answers Maharashtra Board

Balbharati Maharashtra State Board 11th Commerce Maths Solution Book Pdf Chapter 6 Permutations and Combinations Ex 6.7 Questions and Answers.

Std 11 Maths 2 Exercise 6.7 Solutions Commerce Maths

Question 1.
Find n if ⁿC₈ = ⁿC₁₂
Solution:
ⁿC₈ = ⁿC₁₂
If ⁿC_x = ⁿC_y, then either x = y or x = n – y
∴ 8 = 12 or 8 = n – 12
But 8 = 12 is not possible
∴ 8 = n – 12
∴ n = 20

Question 2.
Find n if ²³C_3n = ²³C_2n+3
Solution:
²³C_3n = ²³C_2n+3
If ⁿC_x = ⁿC_y, then either x = y or x = n – y
∴ 3n = 2n + 3 or 3n = 23 – 2n – 3
∴ n = 3 or n = 4

Question 3.
Find n if ²¹C_6n = \({ }^{21} C_{n^{2}+5}\)
Solution:
²¹C_6n = \({ }^{21} C_{n^{2}+5}\)
If ⁿC_x = ⁿC_y, then either x = y or x = n – y
∴ 6n = n² + 5 or 6n = 21 – (n² + 5)
∴ n² – 6n + 5 = 0 or 6n = 21 – n² – 5
∴ n² – 6n + 5 = 0 or n² + 6n – 16 = 0
If n² – 6n + 5 = 0 then (n – 1)(n – 5) = 0
∴ n = 1 or n = 5
If n = 5 then n² + 5 = 30 > 21
∴ n ≠ 5
∴ n = 1
If n² + 6n – 16 = 0 then (n + 8)(n – 2) = 0
n = -8 or n = 2
n ≠ -8
∴ n = 2

Question 4.
Find n if ²ⁿC_r-1 = ²ⁿC_r+1
Solution:
²ⁿC_r-1 = ²ⁿC_r+1
If ⁿC_x = ⁿC_y, then either x = y or x = n – y
∴ r – 1 = r + 1 or r – 1 = 2n – (r + 1)
But r – 1 = r + 1 is not possible
∴ r – 1 = 2n – (r + 1)
∴ r + r = 2n
∴ r = n

Question 5.
Find n if ⁿC_n-2 = 15
Solution:
ⁿC_n-2 = 15
∴ ⁿC₂ = 15 …….[∵ ⁿC_r = ⁿC_n-r]
∴ \(\frac{n !}{(n-2) ! 2 !}\) = 15
∴ \(\frac{\mathrm{n}(\mathrm{n}-1)(\mathrm{n}-2) !}{(\mathrm{n}-2) ! 2 \times 1}\) = 15
∴ n(n – 1) = 30
∴ n(n – 1) = 6 × 5
Comparing both sides, we get
∴ n = 6

Question 6.
Find x if ⁿP_r = x ⁿC_r
Solution:

Question 7.
Find r if ¹¹C₄ + ¹¹C₅ + ¹²C₆ + ¹³C₇ = ¹⁴C_r
Solution:

Question 8.
Find the value of \(\sum_{r=1}^{4}{ }^{21-r} C_{4}+{ }^{17} C_{5}\)
Solution:

Question 9.
Find the differences between the largest values in the following:
(i) ¹⁴C_r – ¹²C_r
Solution:
Greatest value of ¹⁴C_r
Here n = 14, which is even
Greatest value of ⁿC_r occurs at r = \(\frac{n}{2}\) if n is even

∴ Difference between the greatest values of ¹⁴C_r and ¹²C_r = 3432 – 924 = 2508

(ii) ¹³C_r – ⁸C_r
Solution:
Greatest value of ¹³C_r
Here n = 13, which is odd
Greatest value of ⁿC_r occurs at r = \(\frac{\mathrm{n}-1}{2}\) if n is odd

∴ Difference between the greatest values of ¹³C_r and ⁸C_r = 1716 – 70 = 1646

(iii) ¹⁵C_r – ¹¹C_r
Solution:
Greatest value of ¹⁵C_r
Here n = 15, which is odd
Greatest value of ⁿC_r occurs at r = \(\frac{\mathrm{n}-1}{2}\) if n is odd

Difference between the greatest values of ¹⁵C_r and ¹¹C_r = 6435 – 462 = 5973

Question 10.
In how many ways can a boy invite his 5 friends to a party so that at least three join the party?
Solution:
Boy can invite = (3 or 4 or 5 friends)
Consider the following table:

∴ Number of ways a boy can invite his friends to a party so that three or more join the party = 10 + 5 + 1 = 16

Question 11.
A group consists of 9 men and 6 women. A team of 6 is to be selected. How many possible selections will have at least 3 women?
Solution:
There are 9 men and 6 women.
A team of 6 persons is to be formed such that it consists of at least 3 women.

∴ Number of ways this can be done = 1680 + 540 + 54 + 1 = 2275
∴ 2275 teams can be formed if team consists of at least 3 women.

Question 12.
A committee of 10 persons is to be formed from a group of 10 women and 8 men. How many possible committees will have at least 5 women? How many possible committees will have men in the majority?
Solution:
(i) A committee of 10 persons is to be formed from 10 women and 8 men such that the committee contains at least 5 women
Consider the following table:


∴ Number of committees = 14112 + 14700 + 6720 + 1260 + 81 = 36873
∴ At least 5 women are there in 36873 committees.

(ii) Number of committees with men in majority = Total number of committees – (Number of committees with women in majority + women and men equal in number)
= ¹⁸C₁₀ – 36873
= ¹⁸C₈ – 36873
= 43758 – 36873
= 6885

Question 13.
A question paper has two sections. Section I has 5 questions and section II has 6 questions. A student must answer at least two questions from each section among 6 questions he answers. How many different choices does the student have in choosing questions?
Solution:
There are 11 questions, out of which 5 questions are from section I and 6 questions are from section II.
The student has to select 6 questions taking at least 2 questions from each section.
Consider the following table:

∴ Number of choices = 150 + 200 + 75 = 425
∴ In 425 ways students can select 6 questions, taking at least 2 questions from each section.

Question 14.
There are 3 wicketkeepers and 5 bowlers among 22 cricket players. A team of 11 players is to be selected so that there is exactly one wicketkeeper and at least 4 bowlers in the team. How many different teams can be formed?
Solution:
There are 22 cricket players, of which 3 are wicketkeepers and 5 are bowlers.
A team of 11 players is to be chosen such that exactly one wicketkeeper and at least 4 bowlers are to be included in the team.
Consider the following table:

∴ Number of ways a team of 11 players can be selected = 45045 + 6006 = 51051

Question 15.
Five students are selected from 11. How many ways can these students be selected if
(i) two specified students are selected?
(ii) two specified students are not selected?
Solution:
5 students are to be selected from 11 students
(i) When 2 specified students are included
then remaining 3 students can be selected from (11 – 2) = 9 students.
∴ Number of ways of selecting 3 students from 9 students = ⁹C₃
= \(\frac{9 !}{3 ! \times 6 !}\)
= \(\frac{9 \times 8 \times 7 \times 6 !}{3 \times 2 \times 1 \times 6 !}\)
= 84
∴ Selection of students is done in 126 ways when 2 specified students are not selected.

(ii) When 2 specified students are not included then 5 students can be selected from the remaining (11 – 2) = 9 students
∴ Number of ways of selecting 5 students from 9 students = ⁹C₅
= \(\frac{9 !}{5 ! 4 !}\)
= \(\frac{9 \times 8 \times 7 \times 6 \times 5 !}{5 ! \times 4 \times 3 \times 2 \times 1}\)
= 126
∴ Selection of students is done in 126 ways when 2 specified students are not selected.

11th Commerce Maths Digest Pdf

• 11th Commerce Maths Exercise 6.1 Solutions
• 11th Commerce Maths Exercise 6.2 Solutions
• 11th Commerce Maths Exercise 6.3 Solutions
• 11th Commerce Maths Exercise 6.4 Solutions
• 11th Commerce Maths Exercise 6.5 Solutions
• 11th Commerce Maths Exercise 6.6 Solutions
• 11th Commerce Maths Exercise 6.7 Solutions
• 11th Commerce Maths  Miscellaneous Exercise 6 Solutions

Probability Class 11 Commerce Maths 2 Chapter 7 Exercise 7.4 Answers Maharashtra Board

Balbharati Maharashtra State Board 11th Commerce Maths Solution Book Pdf Chapter 7 Probability Ex 7.4 Questions and Answers.

Std 11 Maths 2 Exercise 7.4 Solutions Commerce Maths

Question 1.
Two dice are thrown simultaneously, if at least one of the dice shows a number 5, what is the probability that sum of the numbers on two dice is 9?
Solution:
When two dice are thrown simultaneously, the sample space is
S = {(1, 1), (1, 2), (1, 3), (1, 4), (1, 5), (1, 6), (2, 1), (2, 2), (2, 3), (2, 4), (2, 5), (2, 6), (3, 1), (3, 2), (3, 3), (3, 4), (3, 5), (3, 6), (4, 1), (4, 2), (4, 3), (4, 4), (4, 5), (4, 6), (5, 1), (5, 2), (5, 3), (5, 4), (5, 5), (5, 6), (6, 1), (6, 2), (6, 3), (6, 4), (6, 5), (6, 6)}
∴ n(S) = 36
Let A be the event that at least one die shows number 5.
∴ A = {(1, 5), (2, 5), (3, 5), (4, 5), (5, 1), (5, 2), (5, 3), (5, 4), (5, 5), (5, 6), (6, 5)}
∴ n(A) = 11
∴ P(A) = \(\frac{\mathrm{n}(\mathrm{A})}{\mathrm{n}(\mathrm{S})}=\frac{11}{36}\)
Let B be the event that sum of the numbers on two dice is 9.
∴ B = {(3, 6), (4, 5), (5, 4), (6, 3)}
Also, A ∩ B = {(4, 5), (5, 4)}
∴ n(A ∩ B) = 2
∴ P(A ∩ B) = \(\frac{n(A \cap B)}{n(S)}=\frac{2}{36}\)
∴ Probability of sum of numbers on two dice is 9, given that one dice shows number 5, is given by
P(B/A) = \(\frac{\mathrm{P}(\mathrm{A} \cap \mathrm{B})}{\mathrm{P}(\mathrm{A})}=\frac{\frac{2}{36}}{\frac{11}{36}}=\frac{2}{11}\)

Question 2.
A pair of dice is thrown. If sum of the numbers is an even number, what is the probability that it is a perfect square?
Solution:
When two dice are thrown simultaneously, the sample space is
S = {(1, 1), (1, 2), (1, 3), (1, 4), (1, 5), (1, 6), (2, 1), (2, 2), (2, 3), (2, 4), (2, 5), (2, 6), (3, 1), (3, 2), (3, 3), (3, 4), (3, 5), (3, 6), (4, 1), (4, 2), (4, 3), (4, 4), (4, 5), (4, 6), (5, 1), (5, 2), (5, 3), (5, 4), (5, 5), (5, 6), (6, 1), (6, 2), (6, 3), (6, 4), (6, 5), (6, 6)}
∴ n(S) = 36
Let A be the event that sum of the numbers is an even number.
∴ A = {(1, 1), (1, 3), (1, 5), (2, 2), (2, 4), (2, 6), (3, 1), (3, 3), (3, 5), (4, 2), (4, 4), (4, 6), (5, 1), (5, 3), (5, 5), (6, 2), (6, 4), (6, 6)}
∴ n(A) = 18
∴ P(A) = \(\frac{\mathrm{n}(\mathrm{A})}{\mathrm{n}(\mathrm{S})}=\frac{18}{36}\)
Let B be the event that sum of outcomes is a perfect square.
∴ B = {(1, 3), (2, 2), (3, 1), (3, 6), (4, 5), (5, 4), (6, 3)}
Also, A n B= {(1, 3), (2, 2), (3, 1)}
∴ n(A ∩ B) = 3
∴ P(A ∩ B) = \(\frac{\mathrm{n}(\mathrm{A} \cap \mathrm{B})}{\mathrm{n}(\mathrm{S})}=\frac{3}{36}\)
∴ Probability of sum of the numbers is a perfect square, given that sum of numbers is an even number, is given by
P(B/A) = \(\frac{\mathrm{P}(\mathrm{A} \cap \mathrm{B})}{\mathrm{P}(\mathrm{A})}=\frac{\frac{3}{36}}{\frac{18}{36}}=\frac{3}{18}=\frac{1}{6}\)

Question 3.
A box contains 11 tickets numbered from 1 to 11. Two tickets are drawn at random with replacement. If the sum is even, find the probability that both the numbers are odd.
Solution:
Two tickets can be drawn from 11 tickets with replacement in 11 × 11 = 121 ways.
∴ n(S) = 121
Let A be the event that the sum of two numbers is even.
The event A occurs, if either both the tickets with odd numbers or both the tickets with even numbers are drawn.
There are 6 odd numbers (1, 3, 5, 7, 9, 11) and 5 even numbers (2, 4, 6, 8, 10) from 1 to 11.
∴ n(A) = 6 × 6 + 5 × 5
= 36 + 25
= 61
∴ P(A) = \(\frac{\mathrm{n}(\mathrm{A})}{\mathrm{n}(\mathrm{S})}=\frac{61}{121}\)
Let B be the event that the numbers tickets drawn are odd
∴ n(B) = 6 × 6 = 36
∴ P(B) = \(\frac{\mathrm{n}(\mathrm{B})}{\mathrm{n}(\mathrm{S})}=\frac{36}{121}\)
Since 6 odd numbers are common between A and B.
∴ n(A ∩ B) = 6 × 6 = 36
∴ P(A ∩ B) = \(\frac{\mathrm{n}(\mathrm{A} \cap \mathrm{B})}{\mathrm{n}(\mathrm{S})}=\frac{36}{121}\)
∴ Probability of both the numbers are odd, given that sum is even, is given by
P(B/A) = \(\frac{\mathrm{P}(\mathrm{A} \cap \mathrm{B})}{\mathrm{P}(\mathrm{A})}=\frac{\frac{36}{121}}{\frac{61}{121}}=\frac{36}{61}\)

Question 4.
A card is drawn from a well-shuffled pack of 52 cards. Consider two events A and B as
A: a club card is drawn.
B: an ace card is drawn.
Determine whether events A and B are independent or not.
Solution:
One card can be drawn out of 52 cards in ⁵²C₁ ways.
∴ n(S) = ⁵²C₁
Let A be the event that a club card is drawn.
1 club card out of 13 club cards can be drawn in ¹³C₁ ways.
∴ n(A) = ¹³C₁
∴ P(A) = \(\frac{\mathrm{n}(\mathrm{A})}{\mathrm{n}(\mathrm{S})}=\frac{{ }^{13} \mathrm{C}_{1}}{{ }^{52} \mathrm{C}_{1}}\)
Let B be the event that an ace card is drawn.
An ace card out of 4 aces can be drawn in ⁴C₁ ways.
∴ n(B) = ⁴C₁
∴ P(B) = \(\frac{\mathrm{n}(\mathrm{B})}{\mathrm{n}(\mathrm{S})}=\frac{{ }^{4} \mathrm{C}_{1}}{{ }^{52} \mathrm{C}_{1}}\)
Since 1 card is common between A and B
∴ n(A ∩ B) = ¹C₁
∴ P(A ∩ B) = \(\frac{\mathrm{n}(\mathrm{A} \cap \mathrm{B})}{\mathrm{n}(\mathrm{S})}=\frac{{ }^{1} \mathrm{C}_{1}}{{ }^{52} \mathrm{C}_{1}}=\frac{1}{52}\) …….(i)
∴ P(A) × P(B) = \(\frac{{ }^{13} \mathrm{C}_{1}}{{ }^{52} \mathrm{C}_{1}} \times \frac{{ }^{4} \mathrm{C}_{1}}{{ }^{52} \mathrm{C}_{1}}=\frac{13 \times 4}{52 \times 52}=\frac{1}{52}\) …….(ii)
From (i) and (ii), we get
P(A ∩ B) = P(A) × P(B)
∴ A and B are independent events.

Question 5.
A problem in statistics is given to three students A, B, and C. Their chances of solving the problem are 1/3, 1/4, and 1/5 respectively. If all of them try independently, what is the probability that,
(i) problem is solved?
(ii) problem is not solved?
(iii) exactly two students solve the problem?
Solution:
Let A be the event that student A can solve the problem.
B be the event that student B can solve the problem.
C be the event that student C can solve problem.
∴ P(A) = \(\frac{1}{3}\), P(B) = \(\frac{1}{4}\), P(C) = \(\frac{1}{5}\)
∴ P(A’) = 1 – P(A) = 1 – \(\frac{1}{3}\) = \(\frac{2}{3}\)
P(B’) = 1 – P(B) = 1 – \(\frac{1}{4}\) = \(\frac{5}{4}\)
P(C’) = 1 – P(C) = 1 – \(\frac{1}{5}\) = \(\frac{4}{5}\)
Since A, B, C are independent events
∴ A’, B’, C’ are also independent events
(i) Let X be the event that problem is solved.
Problem can be solved if at least one of the three students solves the problem.
P(X) = P(at least one student solves the problem)
= 1 – P(no student solved problem)
= 1 – P(A’ ∩ B’ ∩ C’)
= 1 – P(A’) P(B’) P(C’)
= 1 – \(\frac{2}{3} \times \frac{3}{4} \times \frac{4}{5}\)
= 1 – \(\frac{2}{5}\)
= \(\frac{3}{5}\)

(ii) Let Y be the event that problem is not solved
∴ P(Y) = P(A’ ∩ B’ ∩ C’)
= P(A’) P(B’) P(C’)
= \(\frac{2}{3} \times \frac{3}{4} \times \frac{4}{5}\)
= \(\frac{2}{5}\)

(iii) Let Z be the event that exactly two students solve the problem.
∴ P(Z) = P(A ∩ B ∩ C’) ∪ P(A ∩ B’ ∩ C) ∪ P(A’ ∩ B ∩ C)
= P(A) . P(B) . P(C’) + P(A) . P(B’) . P(C) + P(A’) . P(B) . P(C)
= \(\left(\frac{1}{3} \times \frac{1}{4} \times \frac{4}{5}\right)+\left(\frac{1}{3} \times \frac{3}{4} \times \frac{1}{5}\right)+\left(\frac{2}{3} \times \frac{1}{4} \times \frac{1}{5}\right)\)
= \(\frac{4}{60}+\frac{3}{60}+\frac{2}{60}\)
= \(\frac{3}{20}\)

Question 6.
The probability that a 50-year old man will be alive till age 60 is 0.83 and the probability that a 45-year old woman will be alive till age 55 is 0.97. What is the probability that a man whose age is 50 and his wife whose age is 45 will both be alive for the next 10 years?
Solution:
Let A be the event that man will be alive at 60.
∴ P(A) = 0.83
Let B be the event that a woman will be alive at 55.
∴ P(B) = 0.97
A ∩ B = Event that both will be alive.
Also, A and B are independent events
∴ P(both man and his wife will be alive) = P(A ∩ B)
= P(A) . P(B)
= 0.83 × 0.97
= 0.8051

Question 7.
In an examination, 30% of the students have failed in subject I, 20% of the students have failed in subject II and 10% have failed in both subjects I and subject II. A student is selected at random, what is the probability that the student
(i) has failed in the subject I, if it is known that he is failed in subject II?
(ii) has failed in at least one subject?
(iii) has failed in exactly one subject?
Solution:
Let A be the event that the student failed in Subject I
B be the event that the student failed in Subject II
Then P(A) = 30% = \(\frac{30}{100}\)
P(B) = 20% = \(\frac{20}{100}\)
and P(A ∩ B) = 10% = \(\frac{10}{100}\)
(i) P (student failed in Subject I, given that he has failed in Subject II) = P(A/B)
\(\frac{\mathrm{P}(\mathrm{A} \cap \mathrm{B})}{\mathrm{P}(\mathrm{B})}=\frac{\left(\frac{10}{100}\right)}{\left(\frac{20}{100}\right)}=\frac{10}{20}=\frac{1}{2}\)

(ii) P(student failed in at least one subject) = P(A ∪ B)
= P(A) + P(B) – P(A ∩ B)
= \(\frac{30}{100}+\frac{20}{100}-\frac{10}{100}\)
= 0.40

(iii) P(student failed in exactly one subject) = P(A) + P(B) – 2P(A ∩ B)
= \(\frac{30}{100}+\frac{20}{100}-2\left(\frac{10}{100}\right)\)
= 0.30

Question 8.
One-shot is fired from each of the three guns. Let A, B, and C denote the events that the target is hit by the first, second and third gun respectively. Assuming that A, B, and C are independent events and that P(A) = 0.5, P(B) = 0.6, and P(C) = 0.8, then find the probability that at least one hit is registered.
Solution:
A be the event that first gun hits the target
B be the event that second gun hits the target
C be the event that third gun hits the target
P(A) = 0.5, P(B) = 0.6, P(C) = 0.8
∴ P(A’) = 1 – P(A) = 1 – 0.5 = 0.5
∴ P(B’) = 1 – P(B) = 1 – 0.6 = 0.4
∴ P(C’) = 1 – P(C) = 1 – 0.8 = 0.2
Now A, B, C are independent events
∴ A’, B’, C are also independent events.
∴ P (at least one hit is registered)
= 1 – P(no hit is registered)
= 1 – P(A’ ∩ B’ ∩ C’)
= 1 – P(A’) P(B’) P(C’)
= 1 – (0.5) (0.4) (0.2)
= 1 – 0.04
= 0.96

Question 9.
A bag contains 10 white balls and 15 black balls. Two balls are drawn in succession without replacement. What is the probability that
(i) first is white and second is black?
(ii) one is white and the other is black?
Solution:
Total number of balls = 10 + 15 = 25
Let S be an event that two balls are drawn at random without replacement in succession
∴ n(S) = ²⁵C₁ × ²⁴C₁ = 25 × 24
(i) Let A be the event that the first ball is white and the second is black.
First white ball can be drawn from 10 white balls in ¹⁰C₁ ways
and second black ball can be drawn from 15 black balls in ¹⁵C₁ ways.
∴ n(A) = ¹⁰C₁ × ¹⁵C₁
∴ P(A) = \(\frac{\mathrm{n}(\mathrm{A})}{\mathrm{n}(\mathrm{S})}=\frac{{ }^{10} \mathrm{C}_{1} \times{ }^{15} \mathrm{C}_{1}}{25 \times 24}=\frac{10 \times 15}{25 \times 24}=\frac{1}{4}\)

Question 10.
An urn contains 4 black, 5 white, and 6 red balls. Two balls are drawn one after the other without replacement, what is the probability that at least one ball is black?
Solution:
Total number of balls in the urn = 4 + 5 + 6 = 15
Two balls can be drawn without replacement in ¹⁵C₂ = \(\frac{15 \times 14}{1 \times 2}\) = 105 ways
∴ n(S) = 105
Let A be the event that at least one ball is black
i.e., 1 black and 1 non-black or 2 black and 0 non-black.
1 black ball can be drawn out of 4 black balls in ⁴C₁ = 4 ways
and 1 non-black ball can be drawn out of remaining 11 non-black balls in ¹¹C₁ = 11 ways
∴ 1 black and 1 non black ball can be drawn in 4 × 11 = 44 ways
Also, 2 black balls can be drawn from 4 black balls in ⁴C₂ = \(\frac{4 \times 3}{1 \times 2}\) = 6 ways
∴ n(A) = 44 + 6 = 50
∴ Required probability = P(A) = \(\frac{n(A)}{n(S)}=\frac{50}{105}\) = \(\frac{10}{21}\)

Alternate Solution:
Total number of balls = 15
Required probability = 1 – P(neither of two balls is black)
Balls are drawn without replacement
Probability of first non-black ball drawn = \(\frac{11}{15}\)
Probability of second non-black ball drawn = \(\frac{10}{14}\)
Probability of neither of two balls is black = \(\frac{11}{15} \times \frac{10}{14}=\frac{11}{21}\)
Required probability = 1 – \(\frac{11}{21}\) = \(\frac{10}{21}\)

Question 11.
Two balls are drawn from an urn containing 5 green, 3 blue, 7 yellow balls one by one without replacement. What is the probability that at least one ball is blue?
Solution:
Total number of balls in the urn = 5 + 3 + 7 = 15
Out of these 12 are non-blue balls.
Two balls can be drawn from 15 balls without replacement in ¹⁵C₂
= \(\frac{15 \times 14}{1 \times 2}\)
= 105 ways.
∴ n(S) = 105
Let A be the event that at least one ball is blue,
i.e., 1 blue and other non-blue or both are blue.
∴ n(A) = ³C₁ × ¹²C₁ + ³C₂
= 3 × 12 + 3
= 36 + 3
= 39
∴ P(A) = \(\frac{\mathrm{n}(\mathrm{A})}{\mathrm{n}(\mathrm{S})}=\frac{39}{105}=\frac{13}{35}\)

Alternate solution:
Total number of balls in the urn = 15
Required probability = 1 – P(neither of two balls is blue)
Balls are drawn one by one without replacement.
Probability of first non-blue ball drawn = \(\frac{12}{15}\)
Probability of second non-blue ball drawn = \(\frac{11}{14}\)
Probability of neither of two ball is blue = \(\frac{12}{15} \times \frac{11}{14}=\frac{22}{35}\)
∴ Required probability = 1 – \(\frac{22}{35}\) = \(\frac{13}{35}\)

Question 12.
A bag contains 4 blue and 5 green balls. Another bag contains 3 blue and 7 green balls. If one ball ¡s drawn from each bag, what is the Probability that two balls are of the same colour?
Solution:
Let A be the event that a blue ball is drawn from each bag.
Probability of drawing one blue ball out of 4 blue balls where there are a total of 9 balls in the first bag and that of drawing one blue ball out of 3 blue balls where there are a total of 10 balls in the second bag is
P(A) = \(\frac{4}{9} \times \frac{3}{10}\)
Let B be the event that a green ball is drawn from each bag.
Probability of drawing one green ball out of 5 green balls where there are a total of 9 balls in the first bag and that of drawing one green ball out of 7 green balls where there are a total of 10 balls in the second bag is
P(B) = \(\frac{5}{9} \times \frac{7}{10}\)
Since both, the events are mutually exclusive and exhaustive events
∴ P(that both the balls are of the same colour) = P(both are of blue colour) or P(both are of green colour)
= P(A) + P(B)
= \(\frac{4}{9} \times \frac{3}{10}+\frac{5}{9} \times \frac{7}{10}\)
= \(\frac{12}{90}+\frac{35}{90}\)
= \(\frac{47}{90}\)

Question 13.
Two cards are drawn one after the other from a pack of 52 cards with replacement. What is the probability that both the cards are drawn are face cards?
Solution:
Two cards are drawn from a pack of 52 cards with replacement.
∴ n(S) = 52 × 52
Let A be the event that two cards drawn are face cards.
First card from 12 face cards is drawn with replacement in ¹²C₁ = 12 ways
and second face card is drawn from 12 face card in ¹²C₁ = 12 ways after replacement.
∴ n(A) = 12 × 12
∴ P(that both the cards drawn are face cards) = P(A)
= \(\frac{n(A)}{n(S)}=\frac{12 \times 12}{52 \times 52}=\frac{9}{169}\)

11th Commerce Maths Digest Pdf

• 11th Commerce Maths Exercise 7.1 Solutions
• 11th Commerce Maths Exercise 7.2 Solutions
• 11th Commerce Maths Exercise 7.3 Solutions
• 11th Commerce Maths Exercise7.4 Solutions
• 11th Commerce Maths Miscellaneous Exercise 7 Solutions

Commercial Mathematics Class 11 Commerce Maths 2 Chapter 9 Exercise 9.7 Answers Maharashtra Board

Balbharati Maharashtra State Board 11th Commerce Maths Solution Book Pdf Chapter 9 Commercial Mathematics Ex 9.7 Questions and Answers.

Std 11 Maths 2 Exercise 9.7 Solutions Commerce Maths

Question 1.
Shantanu has a choice to invest in ₹ 10 shares of two firms at ₹ 13 or at ₹ 16. If the first firm pays a 5% dividend and the second firm pays a 6% dividend per annum, find:
(i) Which firm is paying better?
(ii) If Shantanu invests equally in both the firms and the difference between the return from them is ₹ 30. Find how much, in all, does he invest.
Solution:
(i) For firm 1:
Face value of the share (F.V.) = ₹ 10
Market value of the share (M.V.) = ₹ 13
Dividend = 5%
∴ Annual income from the share = \(\frac{5}{100}\) × 10 = ₹ 0.5

For firm 2:
Face value of the share (F.V.) = ₹ 10
Market value of the share (M.V.) = ₹ 16
Dividend = 5%
∴ Annual income from the share = \(\frac{6}{100}\) × 10 = ₹ 0.6

Since, the profit percentage from firm 1 > profit percentage from firm 2, the first firm is paying better.

(ii) Let ‘X’ be the amount Shantanu invests in each of the firms.
Given that difference between the return from them is ₹ 30, we have

In all, Shantanu invests = 2X
= 2 × 31,200
= ₹ 62,400/-

Question 2.
A dividend of 9% was declared on ₹ 100 shares selling at a certain price in the stock market. If the rate of return is 7.5% calculate
(i) The market price of each share, and
(ii) The amount to be invested to obtain an annual dividend of ₹ 630.
Solution:
(i) Given that,
Face value of the share (F.V) = ₹ 100
Dividend = 9%
Rate of return = 7.5%
Annual income from the share = \(\frac{9}{100}\) × 100 = ₹ 9

∴ The market price of the share is ₹ 120.

(ii) Let ‘X’ be the amount to be invested to obtain an annual dividend of ₹ 630.
∴ 7.5% of X is ₹ 630
∴ \(\frac{7.5}{100}\) × X = 630
∴ X = \(\frac{630 \times 100}{7.5}\)
∴ X = 8400
∴ ₹ 8400 need to be invested to obtain an annual dividend of ₹ 630.

Question 3.
Nilesh has the option of investing his money in 8% ₹ 10 shares at a premium of ₹ 3.50 or 7% ₹ 100 shares at a premium of 20%. Which of the two investments will be more profitable for him?
Solution:
For share 1:
Face value of the share (F.V.) = ₹ 10
Premium = ₹ 3.5
∴ Market value of the share (M.V.) = 10 + 3.5 = ₹ 13.5
Dividend = 8 %
∴ Annual income from the share = \(\frac{8}{100}\) × 10 = ₹ 0.8

= \(\frac{800}{135}\)
= 5.93%

For share 2:
Face value of the share (F.V.) = ₹ 100
Premium = 20%
∴ Market value of the share (M.V.) = 100 + (\(\frac{20}{100}\) × 100) = ₹ 120
Dividend = 7%
Annual income from the share = \(\frac{7}{100}\) × 100 = ₹ 7

Since, profit percentage from share 1 > profit percentage from share 2, investing in the first kind of shares will be more profitable for Nilesh.

Question 4.
Sudhakar invests ₹ 1344 in buying shares of face value ₹ 24 selling at a 12% premium. The dividend on the shares is 15% per annum. Calculate
(i) The number of shares Sudhakar buys, and
(ii) The dividend he receives annually.
Solution:
Given that,
Face value of the share (F.V.) = ₹ 24
Premium = 12%
∴ Market value of the share (M.V.) = 24 + (\(\frac{12}{100}\) × 24) = ₹ 26.88
(i) Sudhakar invests ₹ 1344 in the shares
∴ Number of shares purchased by Sudhakar = \(\frac{1344}{26.88}\) = 50
∴ Sudhakar buys 50 shares.
(ii) Dividend on the share = 15%
Annual income on one share = \(\frac{15}{100}\) × 24 = ₹ 3.6
∴ The total dividend he receives annually = 50 × 3.6 = ₹ 180
∴ Sudhakar receives ₹ 180 as his annual dividend.

Question 5.
Sameer invests ₹ 5625 in a company paying 7% per annum when the share of ₹ 10 stands for ₹ 12.50. Find Sameer’s income from this investment. If he sells 60% of these shares of ₹ 10 each, find his gain or loss in this transaction.
Solution:
Given:
Face value of the share (F.V.) = ₹ 10
Market value of the share (M.V.) = ₹ 12.5
Amount invested in shares = ₹ 5625
∴ Number of shares purchased by Sameer = \(\frac{5625}{12.5}\) = 450
Dividend = 7%
Annual income from one share = \(\frac{7}{100}\) × 10 = ₹ 0.7
∴ Sameer’s income from this investment = number of shares × annual income from one share
= 450 × 0.7
= ₹ 315
Sameer sells 60 % of these shares = \(\frac{60}{100}\) × 450 = 270 shares
Sameer purchased these shares at ₹ 12.5 per share.
∴ Purchase price for these shares = 270 × 12.5 = ₹ 3375
If he sells these shares at ₹ 10 per share, he would receive 270 × 10 = ₹ 2700
∴ In this transaction, Sameer would incur a loss of 3375 – 2700 = ₹ 675

Question 6.
Geeta buys ₹ 100 shares of a company that pays a 15% dividend. She buys the shares at a price from the market that gives her a 10% return on her investment. At what price did she buy each share?
Solution:
Given that,
Face value of the share (F.V.) = ₹ 100
Dividend = 15%
∴ Annual income from the share = \(\frac{15}{100}\) × 100 = ₹ 15
Rate of return on investment = 10%

∴ Geeta bought each share from the market at ₹ 150.

Question 7.
Tejas invests in 9% ₹ 100 shares at ₹ 145 but Shail invests in 7% ₹ 100 shares at ₹ 116. Whose investment is better?
Solution:
Investment of Tejas:
Given that, the Face value of the share (F.V.) = ₹ 100
The market value of the share (M.V.) = ₹ 145
Dividend = 9%
Annual income from the share = \(\frac{9}{100}\) × 100 = ₹ 9

Investment of Shail:
Face value of the share (F.V.) = ₹ 100
Market value of the share (M.V.) = ₹ 116
Dividend = 7%
Annual income from the share = \(\frac{7}{100}\) × 100 = ₹ 7

Since the rate of return for Tejas’s investment is greater than that for Shail’s, Tejas’s investment is better.

Question 8.
A 6% share yields 8%. Find the market value of a ₹ 100 share.
Solution:
Given that,
Face value of the share = ₹ 100
Dividend = 6%
Yield = 8%
Annual income on the share = \(\frac{6}{100}\) × 100 = ₹ 6

∴ The market value of the share = ₹ 75

Question 9.
Ashwini bought ₹ 40 shares at a premium of 40%. Find the income, if Ashwini invests ₹ 14,000 in these shares and receives a dividend at the rate of 8% on the nominal value of the shares.
Solution:
Given,
Face value of the shares (F.V.) = ₹ 40
Premium = 40%
Market value of the shares (M.V.) = 40 + (40 × \(\frac{40}{100}\))
= 40 + 16
= ₹ 56
Ashwini invests ₹ 14000 in these shares
∴ Number of shares bought by Ashwini = \(\frac{Amount Invested}{Market value of one share}\)
= \(\frac{14000}{56}\)
= 250
Dividend = 8%
∴ Annual income on one share = \(\frac{8}{100}\) × 40 = ₹ 3.2
∴ Income of Ashwini on 250 shares = 250 × 3.2 = ₹ 800
∴ Ashwini earns ₹ 800 on her investment.

Question 10.
Mr. Rutvik invests ₹ 30,000 in buying shares of a company that pays a 12% dividend annually on ₹ 100 shares selling at a premium of ₹ 50. Find
(i) The number of shares bought Mr. Rutvik and
(ii) His annual income from the shares.
Solution:
Given that,
Face value of a share (F.V.) = ₹ 100
Premium = ₹ 50
∴ Market value of a share (M.V.) = 100 + 50 = ₹ 150
Dividend =12%
Mr. Rutvik invests ₹ 30,000 in the shares.
(i) Number of shares bought by Mr. Rutvik = \(\frac{Amount invested}{Market value}\)
= \(\frac{30000}{150}\)
= 200

(ii) Dividend on the share = 12%
∴ Annual income from one share = \(\frac{12}{100}\) × 100 = ₹ 12
∴ His annual income from shares = number of shares × income from one share
= 200 × 12
= ₹ 2400

Question 11.
Rasika bought ₹ 40 shares at a discount of 40%. Find the income, if she invests ₹ 12,000 in these shares and receives a dividend at the rate of 11% on the nominal value of the shares.
Solution:
Given,
Face value of the shares (F.V.) = ₹ 40
Discount = 40%
∴ Market value of the shares (M.V.) = 40 – (40 × \(\frac{40}{100}\))
= 40 – 16
= ₹ 24
Rasika invests ₹ 12,000 in these shares.
∴ Number of shares bought by Rasika = \(\frac{Amount invested}{Market value of one share}\)
= \(\frac{12000}{24}\)
= 500
Dividend = 11%
∴ Annual income on one share = \(\frac{11}{100}\) × 40 = ₹ 4.4
∴ Rasika’s income on 200 such shares = 500 × 4.4 = ₹ 2200
∴ Rasika earns ₹ 2200 from her investment.

Question 12.
Nisha invests ₹ 15,840 in buying shares of nominal value ₹ 24 selling at a premium of 10%. The company pays a 15% dividend annually. Find
(i) The dividend she receives annually, and
(ii) The rate of return from her investment.
Solution:
Given that,
Face value of the share (F.V.) = ₹ 24
Premium = 10%
∴ Market value of the share (M.V.) = 24 + (24 × \(\frac{10}{100}\))
= 24 + 2.4
= ₹ 26.4
Dividend = 15%
∴ Annual income on the share = \(\frac{15}{100}\) × 24 = ₹ 3.6
Nisha invests ₹ 15,840 in these shares.
∴ Number of shares bought by Nisha

(i) Annual dividend received by Nisha = Number of shares × annual income from one share
= 600 × 3.6
= ₹ 2160

(ii) Rate of return from the investment

Question 13.
Ashutosh buys 80, ₹ 100 shares at a discount of 20% and receives a return of 12% on his money. Calculate
(i) The amount invested by Ashutosh.
(ii) The rate of dividend paid by the company.
Solution:
Given
Face value of the shares (F.V.) = ₹ 100
Discount = 20%
∴ Market value of the shares (M.V.) = 100 – (100 × \(\frac{20}{100}\)) = ₹ 80
(i) Amount invested by Ashutosh = number of shares × market value of the shares
= 80 × 80
= ₹ 6400

(ii) Ashutosh receives a return of 12% on his money.
∴ Ashutosh’s income from shares = \(\frac{12}{100}\) × 6400 = ₹ 768
∴ Ashutosh’s annual income from one share = \(\frac{768}{80}\) = ₹ 9.6
Annual income from one share = \(\frac{\text { Dividend }}{100} \times \text { Face value }\)
∴ 9 6 = \(\frac{\text { Dividend }}{100} \times 100\)
∴ Rate of dividend = 9.6%

Question 14.
Vaishnavi bought 1000, ₹ 100 shares from the stock market carrying 8% dividend quoted at ₹ 130. A few days later the market value of the shares went up by 10%. Vaishnavi sold all her shares. What was her total income from this transaction?
Solution:
Given that,
Face value of the shares (F.V.) = ₹ 100
The market value of the shares (M.V.) = ₹ 130
Dividend = 8%
Income from the each share = \(\frac{8}{100}\) × 100 = ₹ 8
Number of shares bought by Vaishnavi = 1000
∴ Vaishnavi’s income from dividend = 1000 × 8 = ₹ 8000
The price of the shares went up by 10%
New market value of the shares = 130 + (130 × \(\frac{10}{100}\)) = ₹ 143
Vaishnavi sold the shares at ₹ 143 which she bought at ₹ 130 each.
∴ Vaishnavi’s profit on one share =143 – 130 = ₹ 13
∴ Vaishnavi’s profit after selling all her shares =1000 × 13 = ₹ 13,000
Vaishnavi’s total income from this transaction = Income from dividend + income from sale of shares
= 8,000 + 13,000
= ₹ 21,000
∴ Vaishnavi’s total income from this transaction was ₹ 21,000.

Question 15.
Mr. Dinesh invests ₹ 20,800 in 6% ₹ 100 shares at ₹ 104, and ₹ 14,300 in 10.5% ₹ 100 shares at ₹ 143. What will be his annual income from the shares?
Solution:
For 1st kind of shares,
Face value of shares (F.V.) = ₹ 100
Dividend = 6%
∴ Annual income from one share = \(\frac{6}{100}\) × 100 = ₹ 6
Market value of the share (M.V.) = ₹ 104
Total amount invested = ₹ 20,800

∴ Total income from 1st kind of shares = 200 × 6 = ₹ 1200
For 2nd kind of shares,
Face value of shares (F.V.) = ₹ 100
Dividend = 10.5%
∴ Annual income from one share = \(\frac{10.5}{100}\) × 100 = ₹ 10.5
Market value of the share (M.V.) = ₹ 143
Total amount invested = ₹ 14300

∴ Total income from 2nd kind of shares = 100 × 10.5 = ₹ 1050
∴ Total annual income of Dinesh from both these shares = 1200 + 1050 = ₹ 2250

Question 16.
A company declares a semi-annual dividend of 5%. Daniel has 400 shares of the company. If Daniel’s annual income from the shares is ₹ 1,000, find the face value of each share.
Solution:
Given that,
Semi-annual dividend = 5%
∴ Annual dividend = 10%
Number of shares with Daniel = 400
Daniel’s annual income from the shares = ₹ 1000
∴ Annual income from one share = \(\frac{1000}{400}\) = ₹ 2.5
But annual income from one share = \(\frac{\text { Annualdividend }}{100} \times \text { Face value }\)
∴ 2.5 = \(\frac{10}{100}\) × Face value of the share
∴ Face value of the share = ₹ 25

Question 17.
Bhargav buys 400, ₹ 20 shares at a premium of ₹ 4 each and receives a dividend of 12%. Find
(i) The amount invested by Bhargav.
(ii) His total income from the shares.
(iii) Percentage return on his money.
Solution:
Given that,
Face value of the shares (F.V.) = ₹ 20
Premium = ₹ 4
∴ Market value of the shares (M.V.) = ₹ 24
Dividend = 12%
∴ Annual income from the share = \(\frac{12}{100}\) × 20 = ₹ 2.4
Bhargav buys 400 shares
(i) The amount invested by Bhargav = number of shares × market value
= 400 × 24
= ₹ 9600

(ii) Bhargav’s income from the shares = number of shares × annual income from one share
= 400 × 2.4
= ₹ 960

(iii) Percentage return on Bhargav’s money

∴ Bhargav gets 10% as the rate of return on his money.

Question 18.
Anil buys 350 ₹ 100 shares of a company at a premium of 20% from the market. The company pays 12% dividend annually. Find
(i) the investment made by Anil,
(ii) his annual income from the shares, and
(iii) the rate of return from the shares.
Solution:
Given that,
Face value of shares (F.V.) = ₹ 100
Premium = 20%
∴ Market value of shares (M.V.) = 100 + (\(\frac{20}{100}\) × 100) = ₹ 120
Dividend = 12%
∴ Annual income from one share = \(\frac{12}{100}\) × 100 = ₹ 12
Anil buys 350 shares.
(i) Amount invested by Anil = number of shares × market value
= 350 × 120
= ₹ 42,000

(ii) Anil’s annual income from the shares = number of shares × annual income from one share
= 350 × 12
= ₹ 4200

(iii) Rate of return from shares

∴ The rate of return from Anil’s shares is 10%.

11th Commerce Maths Digest Pdf

• 11th Commerce Maths Exercise 9.1 Solutions
• 11th Commerce Maths Exercise 9.2 Solutions
• 11th Commerce Maths Exercise 9.3 Solutions
• 11th Commerce Maths Exercise 9.4 Solutions
• 11th Commerce Maths Exercise 9.5 Solutions
• 11th Commerce Maths Exercise 9.6 Solutions
• 11th Commerce Maths Exercise 9.7 Solutions
• 11th Commerce Maths Miscellaneous Exercise 9 Solutions

Measures of Dispersion Class 11 Commerce Maths 2 Chapter 2 Exercise 2.2 Answers Maharashtra Board

Balbharati Maharashtra State Board 11th Commerce Maths Solution Book Pdf Chapter 2 Measures of Dispersion Ex 2.2 Questions and Answers.

Std 11 Maths 2 Exercise 2.2 Solutions Commerce Maths

Question 1.
Find the variance and S.D. for the following set of numbers.
7, 11, 2, 4, 9, 6, 3, 7, 11, 2, 5, 8, 3, 6, 8, 8, 2, 6
Solution:
Given data: 7, 11, 2, 4, 9, 6, 3, 7, 11, 2, 5, 8, 3, 6, 8, 8, 2, 6
The tabulated form of the above data is as given below:

We prepare the following table for the calculation of variance and S. D.

Question 2.
Find the variance and S.D. for the following set of numbers.
65, 77, 81, 98, 100, 80, 129
Solution:

Question 3.
Compute the variance and standard deviation for the following data:

Solution:
We prepare the following table for the calculation of variance and S.D.:

Question 4.
Compute the variance and S.D.

Solution:
We prepare the following table for the calculation of variance and S.D.:

Question 5.
The following data gives the age of 100 students in a school. Calculate variance and S.D.

Solution:
We prepare the following table for the calculation of variance and S.D:

Question 6.
The mean and variance of 5 observations are 3 and 2 respectively. If three of the five observations are 1, 3, and 5, find the values of the other two observations.
Solution:


∴ (x₄ – 4)(x₄ – 2) = 0
∴ x₄ = 4 or x₄ = 2
From (i), we get
x₅ = 2 or x₅ = 4
∴ The two numbers are 2 and 4.

Question 7.
Obtain standard deviation for the following data:

Solution:
We prepare the following table for the calculation of standard deviation.

Question 8.
The following distribution was obtained by change of origin and scale of variable X.

If it is given that mean and variance are 59.5 and 413 respectively, determine actual class intervals.
Solution:
Here, Mean = \(\bar{x}\) = 59.5, and
Var(X) = σ² = 413
Let x_i be a mid value of class and
d = \(\frac{x-a}{h}\), where a is assumed mean and h is class width.
We prepare the following table for calculation of mean and variance of d_i.


Now, Var(X) = h². Var(D)
∴ 413 = h² × 4.13
∴ h² = 100
∴ h = 10
Substituting h = 10 in (i), we get
-0.1 × 10 + a = 59.5
∴ -1 + a = 59.5
∴ a = 59.5 + 1
∴ a = 60.5
We prepare the following table to determine actual class intervals for corresponding values of d_i.

∴ The actual class intervals are 15.5 – 25.5, 25.5 – 35.5, …….., 95.5 – 105.5

11th Commerce Maths Digest Pdf

• 11th Commerce Maths Exercise 2.1 Solutions
• 11th Commerce Maths Exercise 2.2 Solutions
• 11th Commerce Maths Exercise 2.3 Solutions
• 11th Commerce Maths Miscellaneous Exercise 2 Solutions

Measures of Dispersion Class 11 Commerce Maths 2 Chapter 2 Miscellaneous Exercise 2 Answers Maharashtra Board

Balbharati Maharashtra State Board 11th Commerce Maths Solution Book Pdf Chapter 2 Measures of Dispersion Miscellaneous Exercise 2 Questions and Answers.

Std 11 Maths 2 Miscellaneous Exercise 2 Solutions Commerce Maths

Question 1.
Find the range for the following data.
116, 124, 164, 150, 149, 114, 195, 128, 138, 203, 144
Solution:
Here, largest value (L) = 203, smallest value (S) = 114
∴ Range = L – S
= 203 – 114
= 89

Question 2.
Given below the frequency distribution of weekly w ages of 400 workers. Find the range.

Solution:
Here, largest value (L) = 40, smallest value (S) = 10
∴ Range = L – S
= 40 – 10
= 30

Question 3.
Find the range of the following data.

Solution:
Here, upper limit of the highest class (L) = 175, lower limit of the lowest class (S) = 115
∴ Range = L – S
= 175 – 115
= 60

Question 4.
The city traffic police issued challans for not observing the traffic rules:

Find Q.D.
Solution:
The given data can be arranged in ascending order as follows:
24, 36, 40, 58, 62, 80
Here, n = 6
Q₁ = value of \(\left(\frac{n+1}{4}\right)^{\text {th }}\) observation
= value of \(\left(\frac{6+1}{4}\right)^{\text {th }}\) observation
= value of (1.75)th observation
= value of 1st observation + 0.75(value of 2nd observation – value of 1st observation)
= 24 + 0.75(36 – 24)
= 24 + 0.75(12)
= 24 + 9
∴ Q₁ = 33
Q₃ = value of 3\(\left(\frac{n+1}{4}\right)^{\text {th }}\) observation
= value of 3\(\left(\frac{6+1}{4}\right)^{\text {th }}\) observation
= value of (3 × 1.75)th observation
= value of (5.25)th observation
= value of 5th observation + 0.25(value of 6th observation – value of 5th observation)
= 62 + 0.25(80 – 62)
= 62 + 0.25(18)
= 62 + 4.5
= 66.5
∴ Q.D. = \(\frac{Q_{3}-Q_{1}}{2}=\frac{66.5-33}{2}=\frac{33.5}{2}\) = 16.75

Question 5.
Calculate Q.D. from the following data.

Solution:
We construct the less than cumulative frequency table as follows:

Here, N = 35
Q₁ class = class containing \(\left(\frac{N}{4}\right)^{\text {th }}\) observation
∴ \(\frac{\mathrm{N}}{4}=\frac{35}{4}\) = 8.75
Cumulative frequency which is just greater than (or equal to) 8.75 is 15.
∴ Q₁ lies in the class 20-30.
∴ L = 20, c.f. = 8, f = 7, h = 10

Question 6.
Calculate the appropriate measure of dispersion for the following data.

Solution:
Since open-ended classes are given, the appropriate measure of dispersion that we can compute is the quartile deviation.
We construct the less than cumulative frequency table as follows:

Here N = 250
Q₁ class class containing \(\left(\frac{N}{4}\right)^{t h}\) observation
∴ \(\frac{\mathrm{N}}{4}=\frac{250}{4}\) = 62.5
Cumulative frequency which is just greater than (or equal to) 62.5 is 65.
∴ Q₁ lies in the class 35-40.
∴ L = 35, c.f. = 15, f = 50, h = 5

The cumulative frequency which is just greater than (or equal to) 187.5 is 190.
∴ Q₃ lies in the class 45-50.
∴ L = 45, c.f. = 150, f = 40, h = 5

Question 7.
Calculate Q.D. of the following data.

Solution:
We construct the less than cumulative frequency table as follows:

Here, N = 120
Q₁ class = class containing \(\left(\frac{\mathrm{N}}{4}\right)^{\mathrm{th}}\) observation
∴ \(\frac{\mathrm{N}}{4}=\frac{120}{4}\) = 30
Cumulative frequency which is just greater than (or equal to) 30 is 35.
∴ Q₁ lies in the class 4-6.
∴ L = 4, c.f. = 15, f = 20, h = 2

Cumulative frequency which is just greater than (or equal to) 90 is 90.
∴ Q₃ lies in the class 10-12.
∴ L = 10, c.f. = 72, f = 18, h = 2

Question 8.
Find variance and S.D. for the following set of numbers.
25, 21, 23, 29, 27, 22, 28, 23, 27, 25 (Given √6.6 = 2.57)
Solution:
We prepare the following table for the calculation of variance and S.D.:

Question 9.
Following data gives no. of goals scored by a team in 90 matches.

Compute the variance and standard deviation for the above data.
Solution:
We prepare the following table for the calculation of variance and S.D:

Question 10.
Compute the arithmetic mean and S.D. and C.V. (Given √296 = 17.20)

Solution:
We prepare the following table for calculation of arithmetic mean and S.D.:

Question 11.
The mean and S.D. of 200 items are found to be 60 and 20 respectively. At the time of calculation, two items were wrongly taken as 3 and 67 instead of 13 and 17. Find the correct mean and variance.
Solution:
Here, n = 200, \(\bar{x}\) = Mean = 60, S.D. = 20
Wrongly taken items are 3 and 67.
Correct items are 13 and 17.
Now, \(\bar{x}\) = 60

Correct value of \(\sum_{i=1}^{n} x_{i}=\sum_{i=1}^{n} x_{i}\) (sum of wrongly taken items) + (sum of correct items)
= 12000 – (3 + 67) + (13 + 17)
= 12000 – 70 + 30
= 11960
Correct value of mean = \(\frac{1}{n}\) × correct value of \(\sum_{i=1}^{n} x_{i}\)
= \(\frac{1}{200}\) × 11960
= 59.8
Now, S.D. = 20
Variance = (S.D.)² = 20²
∴ Variance = 400
∴ \(\frac{1}{\mathrm{n}} \sum_{\mathrm{i}=1}^{\mathrm{n}} x_{\mathrm{i}}^{2}-(\bar{x})^{2}=400\)

Correct value of \(\sum_{i=1}^{n} x_{i}^{2}\)
= \(\sum_{i=1}^{n} x_{i}^{2}\) – (Sum of squares of wrongly taken items) + (Sum of squares of correct items)
= 800000 – (3² + 67²) + (13² + 17²)
= 800000 – (9 + 4489) + (169 + 289)
= 800000 – 4498 + 458
= 795960
∴ Correct value of Variance = (\(\frac{1}{n}\) × \(\sum_{i=1}^{n} x_{i}^{2}\)) – (correct value of \(\bar{x}\))²
= \(\frac{1}{200}\) × 795960 – (59.8)²
= 3979.8 – 3576.04
= 403.76
∴ The correct mean is 59.8 and correct variance is 403.76.

Question 12.
The mean and S.D. of a group of 48 observations are 40 and 8 respectively. If two more observations 60 and 65 are added to the set, find the mean and S.D. of 50 items.
Solution:

Question 13.
The mean height of 200 students is 65 inches. The mean heights of boys and girls are 70 inches and 62 inches respectively and the standard deviations are 8 and 10 respectively. Find the number of boys and combined S.D.
Solution:
Let n₁ and n₂ be the number of boys and girls respectively.
Let n = 200, \(\bar{x}_{\mathrm{c}}\) = 65, \(\bar{x}_{1}\) = 70, \(\bar{x}_{1}\) = 62, σ₁ = 8, σ₂ = 10
Here, n₁ + n₂ = n
∴ n₁ + n₂ = 200 …….(i)
Combined mean is given by

∴ 70n₁ + 62n₂ = 13000
∴ 35n₁ + 31n₂ = 6500 ……..(ii)
Solving (i) and (ii), we get
n₁ = 75, n₂ = 125
Combined standard deviation is given by,

Question 14.
From the following data available for 5 pairs of observations of two variables x and y, obtain the combined S.D. for all 10 observations,
where \(\sum_{i=1}^{n} x_{i}=30, \sum_{i=1}^{n} y_{i}=40, \sum_{i=1}^{n} x_{i}^{2}=225, \sum_{i=1}^{n} y_{i}^{2}=340\)
Solution:

Question 15.
The mean and standard deviations of two brands of watches are given below:

Calculate the coefficient of variation of the two brands and interpret the results.
Solution:

Since C.V. (I) > C.V. (II)
∴ the brand I is more variable.

Question 16.
Calculate the coefficient of variation for the data given below. [Given √3.3 = 1.8166]

Solution:

11th Commerce Maths Digest Pdf

• 11th Commerce Maths Exercise 2.1 Solutions
• 11th Commerce Maths Exercise 2.2 Solutions
• 11th Commerce Maths Exercise 2.3 Solutions
• 11th Commerce Maths Miscellaneous Exercise 2 Solutions

Partition Values Class 11 Commerce Maths 2 Chapter 1 Exercise 1.2 Answers Maharashtra Board

Balbharati Maharashtra State Board 11th Commerce Maths Solution Book Pdf Chapter 1 Partition Values Ex 1.2 Questions and Answers.

Std 11 Maths 2 Exercise 1.2 Solutions Commerce Maths

Question 1.
Calculate D₆ and P₈₅ for the following data:
79, 82, 36, 38, 51, 72, 68, 70, 64, 63
Solution:
The given data can be arranged in ascending order as follows:
36, 38, 51, 63, 64, 68, 70, 72, 79, 82
Here, n = 10
D₆ = value of 6\(\left(\frac{n+1}{10}\right)^{\text {th }}\) observation
= value of 6\(\left(\frac{10+1}{10}\right)^{\text {th }}\) observation
= value of (6 × 1.1)th observation
= value of (6.6)th observation
= value of 6th observation + 0.6(value of 7th observation – value of 6th observation)
= 68 + 0.6(70 – 68)
= 68 + 0.6(2)
= 68 + 1.2
∴ D₆ = 69.2
P₈₅ = value of \(\left(\frac{n+1}{100}\right)^{\text {th }}\) observation
= value of \(\left(\frac{10+1}{100}\right)^{\text {th }}\) observation
= value of (85 × 0. 11)th observation
= value of (9.35)th observation
= value of 9th observation + 0.35(value of 10th observation – value of 9th observation)
= 19 + 0.35(82 – 79)
= 79 + 0.35(3)
= 79 + 1.05
∴ P₈₅ = 80.05

Question 2.
The daily wages (in ₹) of 15 labourers are as follows:
230, 400, 350, 200, 250, 380, 210, 225, 375, 180, 375, 450, 300, 350, 250
Calculate D₈ and P₉₀.
Solution:
The given data can be arranged in ascending order as follows:
180, 200, 210, 225, 230, 250, 250, 300, 350, 350, 375, 375, 380, 400, 450
Here, n = 15
D₈ = value of 8\(\left(\frac{n+1}{10}\right)^{\text {th }}\) observation
= value of 8\(\left(\frac{15+1}{10}\right)^{\text {th }}\) observation
= value of (8 × 1.6)th observation
= value of (12.8)th observation
= value of 12th observation – 0.8(value of 13th observation – value of 12th observation)
= 375 + 0.8(380 – 375)
= 375 + 0.8(5)
= 375 + 4
∴ D₈ = 379
P₉₀ = value of 90\(\left(\frac{n+1}{10}\right)^{\text {th }}\) observation
= value of 90\(\left(\frac{15+1}{100}\right)^{\text {th }}\) observation
= value of (90 × 0.16)th observation
= value of (14.4)th observation
= value of 14th observation + 0.4 (value of 15th observation – value of 14th observation)
= 400 + 0.4(450 – 400)
= 400 + 0.4(50)
= 400 + 20
∴ P₉₀ = 420

Question 3.
Calculate 2nd decile and 65th percentile for the following:

Solution:
We construct the less than cumulative frequency table as given below:

Here, n = 200
D₂ = value of 2\(\left(\frac{n+1}{10}\right)^{\text {th }}\) observation
= value of 2\(\left(\frac{200+1}{10}\right)^{\text {th }}\) observation
= value of (2 × 20.1)th observation
= value of (40.2)th observation
Cumulative frequency which is just greater than (or equal to) 40.2 is 58.
∴ D₂ = 120
P₆₅ = value of 65\(\left(\frac{n+1}{100}\right)^{\text {th }}\) observation
= value of 65\(\left(\frac{200+1}{100}\right)^{\text {th }}\) observation
= value of (65 × 2.01)th observation
= value of (130.65)th observation
The cumulative frequency which is just greater than (or equal to) 130.65 is 150.
∴ P₆₅ = 280

Question 4.
From the following data calculate the rent of the 15th, 65th, and 92nd house.

Solution:
Arranging the given data in ascending order.

Here, n = 100
P₁₅ = value of 15
= value of 15\(\left(\frac{n+1}{100}\right)^{\text {th }}\) observation
= value of 15\(\left(\frac{100+1}{100}\right)^{\text {th }}\) observation
= value of (15 × 1.01 )th observation
= value of (15.15)th observation
Cumulative frequency which is just greater than (or equal to) 15.15 is 25.
∴ P₁₅ = 11000
P₆₅ = value of 65\(\left(\frac{n+1}{100}\right)^{\text {th }}\)observation
= value of 65\(\left(\frac{100+1}{100}\right)^{\text {th }}\) observation
= value of (65 × 1.01)th observation
= value of (65.65)th observation
Cumulative frequency which is just greater than (or equal to) 65.65 is 70.
∴ P₆₅ = 14000
P₉₂ = value of 92\(\left(\frac{n+1}{100}\right)^{\text {th }}\) observation
= value of 92\(\left(\frac{100+1}{100}\right)^{\text {th }}\) observation
= value of (92 × 1.01)th observation
= value of (92.92)th observation
Cumulative frequency which is just greater than (or equal to) 92.92 is 98.
∴ P₉₂ = 17000

Question 5.
The following frequency distribution shows the weight of students in a class.

(a) Find the percentage of students whose weight is more than 50 kg.
(b) If the weight column provided is of mid values then find the percentage of students whose weight is more than 50 kg.
Solution:
(a) Let the percentage of students weighing less than 50 kg be x.
∴ Px = 50

From the table, out of 20 students, 84 students have their weight less than 50 kg.
∴ Number of students weighing more than 50 kg = 120 – 84 = 36
∴ Percentage of students having there weight more than 50 kg = \(\frac{36}{120}\) × 100 = 30%

(b) The difference between any two consecutive mid values of weight is 5 kg.
The class intervals must of width 5, with 40, 45,….. as their mid values.
∴ The class intervals will be 37.5 – 42.5, 42.5 – 47.5, etc.
We construct the less than cumulative frequency table as given below:

Here, N = 120
Let P_x = 50
The value 50 lies in the class 47.5 – 52.5
∴ L = 47.5, h = 5, f = 29, c.f. = 55

∴ x = 58 (approximately)
∴ 58% of students are having weight below 50 kg.
∴ Percentage of students having weight above 50 kg is 100 – 58 = 42
∴ 42% of students are having weight above 50 kg.

Question 6.
Calculate D₄ and P₄₈ from the following data:

Solution:
The difference between any two consecutive mid values is 5, the width of class interval = 5
∴ Class interval with mid-value 2.5 is 0 – 5
Class interval with mid value 7.5 is 5 – 10, etc.
We construct the less than cumulative frequency table as given below:

Here, N = 100
D₄ class = class containing \(\left(\frac{4 \mathrm{N}}{10}\right)^{\text {th }}\) observation
∴ \(\frac{4 \mathrm{N}}{10}=\frac{4 \times 100}{10}\) = 40
Cumulative frequency which is just greater than (or equal to) 40 is 50.
∴ D₄ lies in the class 10 – 15.
∴ L = 10,h = 5, f = 25, c.f. = 25
∴ D₄ = \(\mathrm{L}+\frac{\mathrm{h}}{\mathrm{f}}\left(\frac{4 \mathrm{~N}}{10}-\text { c.f. }\right)\)
= 10 + \(\frac{5}{25}\) (40 – 25)
= 10 + \(\frac{1}{5}\) (15)
= 10 + 3
∴ D₄ = 13
P₄₈ class = class containing \(\left(\frac{48 \mathrm{~N}}{100}\right)^{\text {th }}\) observation
∴ \(\frac{48 \mathrm{~N}}{100}=\frac{48 \times 100}{100}\) = 48
Cumulative frequency which is just greater than (or equal to) 48 is 50.
∴ P₄₈ lies in the class 10 – 15.
∴ L = 10, h = 5, f = 25, c.f. = 25
∴ P₄₈ = \(\mathrm{L}+\frac{\mathrm{h}}{\mathrm{f}}\left(\frac{48 \mathrm{~N}}{100}-\text { c.f. }\right)\)
= 10 + \(\frac{5}{25}\) (48 – 25)
= 10 + \(\frac{1}{5}\) (23)
= 10 + 4.6
∴ P₄₈ = 14.6

Question 7.
Calculate D₉ and P₂₀ of the following distribution.

Solution:
We construct the less than cumulative frequency table as given below:

Here, N = 240
D₉ class = class containing \(\left(\frac{9 \mathrm{~N}}{10}\right)^{\mathrm{th}}\) observation
∴ \(\frac{9 \mathrm{~N}}{10}=\frac{9 \times 240}{10}\) = 216
Cumulative frequency which is just greater than (or equal to) 216 is 225.
∴ D₉ lies in the class 80 – 100.
∴ L = 80, h = 20, f = 90, c.f. = 135
∴ D₉ = \(L+\frac{h}{f}\left(\frac{9 N}{10}-c . f .\right)\)
= 80 + \(\frac{20}{90}\)(216 – 135)
= 80 + \(\frac{2}{9}\)(81)
= 80 + 18
∴ D₉ = 98
P₂₀ class = class containing \(\left(\frac{20 \mathrm{~N}}{100}\right)^{\text {th }}\) observation
∴ \(\frac{20 \mathrm{~N}}{100}=\frac{20 \times 240}{100}\) = 48
Cumulative frequency which is just greater than (or equal to) 48 is 50.
∴ P₂₀ lies in the class 40 – 60.
∴ L = 40, h = 20, f = 35, c.f. = 15

∴ P₂₀ = 58.86

Question 8.
Weekly wages for a group of 100 persons are given below:

D₃ for this group is ₹ 1100. Calculate the missing frequencies.
Solution:
Let a and b be the missing frequencies of class 500 – 1000 and class 2000 – 2500 respectively.
We construct the less than cumulative frequency table as given below:

Here, N = 62 + a + b
Since, N = 100
∴ 62 + a + b = 100
∴ a + b = 38 …..(i)
Given, D₃ = 1100
∴ D₃ lies in the class 1000 – 1500.
∴ L = 1000, h = 500, f = 25, c.f. = 7 + a
∴ \(\frac{3 \mathrm{~N}}{10}=\frac{3 \times 100}{10}=30\)
∴ D₃ = \(\mathrm{L}+\frac{\mathrm{h}}{\mathrm{f}}\left(\frac{3 \mathrm{~N}}{10}-\mathrm{c} . \mathrm{f} .\right)\)
∴ 1100 = 1000 + \(\frac{500}{25}\) [30 – (7 + a)]
∴ 1100 – 1000 = 20(30 – 7 – a)
∴ 100 = 20(23 – a)
∴ 100 = 460 – 20a
∴ 20a = 460 – 100
∴ 20a = 360
∴ a = 18
Substituting the value of a in equation (i), we get
18 + b = 38
∴ b = 38 – 18 = 20
∴ 18 and 20 are the missing frequencies of the class 500 – 1000 and class 2000 – 2500 respectively.

Question 9.
The weekly profit (in rupees) of 100 shops are distributed as follows:

Find the limits of the profit of middle 60% of the shops.
Solution:
To find the limits of the profit of the middle 60% of the shops, we have to find P₂₀ and P₈₀.
We construct the less than cumulative frequency table as given below:

Here, N = 100
P₂₀ class = class containing \(\left(\frac{20 \mathrm{N}}{100}\right)^{\text {th }}\) observation
∴ \(\frac{20 \mathrm{N}}{100}=\frac{20 \times 100}{100}=20\)
Cumulative frequency which is just greater than (or equal to) 20 is 26.
∴ P₂₀ lies in the class 1000 – 2000.
∴ L = 1000, h = 1000, f = 16, c.f. = 10
∴ P₂₀ = \(L+\frac{h}{f}\left(\frac{20 \mathrm{~N}}{100}-\text { c.f. }\right)\)
= 1000 + \(\frac{1000}{16}\) (20 – 10)
= 1000 + \(\frac{125}{2}\) (10)
= 1000 + 625
∴ P₂₀ = 1625
P₈₀ class = class containing \(\left(\frac{80 \mathrm{~N}}{100}\right)^{\text {th }}\) observation
∴ \(\frac{80 \mathrm{~N}}{100}=\frac{80 \times 100}{100}=80\)
Cumulative frequency which is just greater than (or equal to) 80 is 92.
∴ P₈₀ lies in the class 4000 – 5000.
∴ L = 4000, h = 1000, f = 20, c.f. = 72
∴ P₈₀ = \(L+\frac{h}{f}\left(\frac{80 \mathrm{~N}}{100}-\text { c.f. }\right)\)
= 4000 + \(\frac{1000}{20}\)(80 – 72)
= 4000 + 50(8)
= 4000 + 400
∴ P₈₀ = 4400
∴ the profit of middle 60% of the shops lie between the limits ₹ 1,625 to ₹ 4,400.

Question 10.
In a particular factory, workers produce various types of output units. The following distribution was obtained:

Find the percentage of workers who have produced less than 82 output units.
Solution:
Since the given data is not continuous, we have to convert it into a continuous form by subtracting 0.5 from the lower limit and adding 0.5 to the upper limit of every class interval.
∴ the class intervals will be 69.5 – 74.5, 74.5 – 79.5, etc.
We construct the less than cumulative frequency table as given below:

Here, N = 445
Let P_x = 82
The value 82 lies in the class 79.5 – 84.5
∴ L = 79.5, h = 5, f = 50, c.f. = 85

∴ 24.72% of workers produced less than 82 output units.

11th Commerce Maths Digest Pdf

• 11th Commerce Maths Exercise 1.1 Solutions
• 11th Commerce Maths Exercise 1.2 Solutions
• 11th Commerce Maths Exercise  1.3 Solutions
• 11th Commerce Maths Miscellaneous Exercise 1 Solutions