Bhagya

Permutations and Combinations Class 11 Commerce Maths 2 Chapter 6 Exercise 6.7 Answers Maharashtra Board

Balbharati Maharashtra State Board 11th Commerce Maths Solution Book Pdf Chapter 6 Permutations and Combinations Ex 6.7 Questions and Answers.

Std 11 Maths 2 Exercise 6.7 Solutions Commerce Maths

Question 1.
Find n if ⁿC₈ = ⁿC₁₂
Solution:
ⁿC₈ = ⁿC₁₂
If ⁿC_x = ⁿC_y, then either x = y or x = n – y
∴ 8 = 12 or 8 = n – 12
But 8 = 12 is not possible
∴ 8 = n – 12
∴ n = 20

Question 2.
Find n if ²³C_3n = ²³C_2n+3
Solution:
²³C_3n = ²³C_2n+3
If ⁿC_x = ⁿC_y, then either x = y or x = n – y
∴ 3n = 2n + 3 or 3n = 23 – 2n – 3
∴ n = 3 or n = 4

Question 3.
Find n if ²¹C_6n = \({ }^{21} C_{n^{2}+5}\)
Solution:
²¹C_6n = \({ }^{21} C_{n^{2}+5}\)
If ⁿC_x = ⁿC_y, then either x = y or x = n – y
∴ 6n = n² + 5 or 6n = 21 – (n² + 5)
∴ n² – 6n + 5 = 0 or 6n = 21 – n² – 5
∴ n² – 6n + 5 = 0 or n² + 6n – 16 = 0
If n² – 6n + 5 = 0 then (n – 1)(n – 5) = 0
∴ n = 1 or n = 5
If n = 5 then n² + 5 = 30 > 21
∴ n ≠ 5
∴ n = 1
If n² + 6n – 16 = 0 then (n + 8)(n – 2) = 0
n = -8 or n = 2
n ≠ -8
∴ n = 2

Question 4.
Find n if ²ⁿC_r-1 = ²ⁿC_r+1
Solution:
²ⁿC_r-1 = ²ⁿC_r+1
If ⁿC_x = ⁿC_y, then either x = y or x = n – y
∴ r – 1 = r + 1 or r – 1 = 2n – (r + 1)
But r – 1 = r + 1 is not possible
∴ r – 1 = 2n – (r + 1)
∴ r + r = 2n
∴ r = n

Question 5.
Find n if ⁿC_n-2 = 15
Solution:
ⁿC_n-2 = 15
∴ ⁿC₂ = 15 …….[∵ ⁿC_r = ⁿC_n-r]
∴ \(\frac{n !}{(n-2) ! 2 !}\) = 15
∴ \(\frac{\mathrm{n}(\mathrm{n}-1)(\mathrm{n}-2) !}{(\mathrm{n}-2) ! 2 \times 1}\) = 15
∴ n(n – 1) = 30
∴ n(n – 1) = 6 × 5
Comparing both sides, we get
∴ n = 6

Question 6.
Find x if ⁿP_r = x ⁿC_r
Solution:

Question 7.
Find r if ¹¹C₄ + ¹¹C₅ + ¹²C₆ + ¹³C₇ = ¹⁴C_r
Solution:

Question 8.
Find the value of \(\sum_{r=1}^{4}{ }^{21-r} C_{4}+{ }^{17} C_{5}\)
Solution:

Question 9.
Find the differences between the largest values in the following:
(i) ¹⁴C_r – ¹²C_r
Solution:
Greatest value of ¹⁴C_r
Here n = 14, which is even
Greatest value of ⁿC_r occurs at r = \(\frac{n}{2}\) if n is even

∴ Difference between the greatest values of ¹⁴C_r and ¹²C_r = 3432 – 924 = 2508

(ii) ¹³C_r – ⁸C_r
Solution:
Greatest value of ¹³C_r
Here n = 13, which is odd
Greatest value of ⁿC_r occurs at r = \(\frac{\mathrm{n}-1}{2}\) if n is odd

∴ Difference between the greatest values of ¹³C_r and ⁸C_r = 1716 – 70 = 1646

(iii) ¹⁵C_r – ¹¹C_r
Solution:
Greatest value of ¹⁵C_r
Here n = 15, which is odd
Greatest value of ⁿC_r occurs at r = \(\frac{\mathrm{n}-1}{2}\) if n is odd

Difference between the greatest values of ¹⁵C_r and ¹¹C_r = 6435 – 462 = 5973

Question 10.
In how many ways can a boy invite his 5 friends to a party so that at least three join the party?
Solution:
Boy can invite = (3 or 4 or 5 friends)
Consider the following table:

∴ Number of ways a boy can invite his friends to a party so that three or more join the party = 10 + 5 + 1 = 16

Question 11.
A group consists of 9 men and 6 women. A team of 6 is to be selected. How many possible selections will have at least 3 women?
Solution:
There are 9 men and 6 women.
A team of 6 persons is to be formed such that it consists of at least 3 women.

∴ Number of ways this can be done = 1680 + 540 + 54 + 1 = 2275
∴ 2275 teams can be formed if team consists of at least 3 women.

Question 12.
A committee of 10 persons is to be formed from a group of 10 women and 8 men. How many possible committees will have at least 5 women? How many possible committees will have men in the majority?
Solution:
(i) A committee of 10 persons is to be formed from 10 women and 8 men such that the committee contains at least 5 women
Consider the following table:


∴ Number of committees = 14112 + 14700 + 6720 + 1260 + 81 = 36873
∴ At least 5 women are there in 36873 committees.

(ii) Number of committees with men in majority = Total number of committees – (Number of committees with women in majority + women and men equal in number)
= ¹⁸C₁₀ – 36873
= ¹⁸C₈ – 36873
= 43758 – 36873
= 6885

Question 13.
A question paper has two sections. Section I has 5 questions and section II has 6 questions. A student must answer at least two questions from each section among 6 questions he answers. How many different choices does the student have in choosing questions?
Solution:
There are 11 questions, out of which 5 questions are from section I and 6 questions are from section II.
The student has to select 6 questions taking at least 2 questions from each section.
Consider the following table:

∴ Number of choices = 150 + 200 + 75 = 425
∴ In 425 ways students can select 6 questions, taking at least 2 questions from each section.

Question 14.
There are 3 wicketkeepers and 5 bowlers among 22 cricket players. A team of 11 players is to be selected so that there is exactly one wicketkeeper and at least 4 bowlers in the team. How many different teams can be formed?
Solution:
There are 22 cricket players, of which 3 are wicketkeepers and 5 are bowlers.
A team of 11 players is to be chosen such that exactly one wicketkeeper and at least 4 bowlers are to be included in the team.
Consider the following table:

∴ Number of ways a team of 11 players can be selected = 45045 + 6006 = 51051

Question 15.
Five students are selected from 11. How many ways can these students be selected if
(i) two specified students are selected?
(ii) two specified students are not selected?
Solution:
5 students are to be selected from 11 students
(i) When 2 specified students are included
then remaining 3 students can be selected from (11 – 2) = 9 students.
∴ Number of ways of selecting 3 students from 9 students = ⁹C₃
= \(\frac{9 !}{3 ! \times 6 !}\)
= \(\frac{9 \times 8 \times 7 \times 6 !}{3 \times 2 \times 1 \times 6 !}\)
= 84
∴ Selection of students is done in 126 ways when 2 specified students are not selected.

(ii) When 2 specified students are not included then 5 students can be selected from the remaining (11 – 2) = 9 students
∴ Number of ways of selecting 5 students from 9 students = ⁹C₅
= \(\frac{9 !}{5 ! 4 !}\)
= \(\frac{9 \times 8 \times 7 \times 6 \times 5 !}{5 ! \times 4 \times 3 \times 2 \times 1}\)
= 126
∴ Selection of students is done in 126 ways when 2 specified students are not selected.

11th Commerce Maths Digest Pdf

• 11th Commerce Maths Exercise 6.1 Solutions
• 11th Commerce Maths Exercise 6.2 Solutions
• 11th Commerce Maths Exercise 6.3 Solutions
• 11th Commerce Maths Exercise 6.4 Solutions
• 11th Commerce Maths Exercise 6.5 Solutions
• 11th Commerce Maths Exercise 6.6 Solutions
• 11th Commerce Maths Exercise 6.7 Solutions
• 11th Commerce Maths  Miscellaneous Exercise 6 Solutions

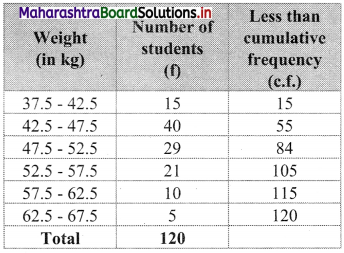
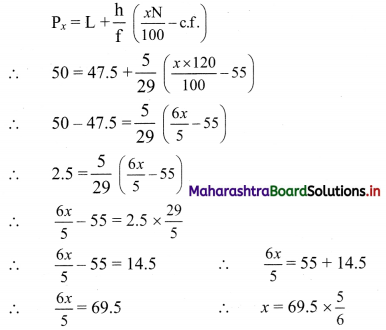
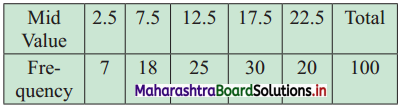
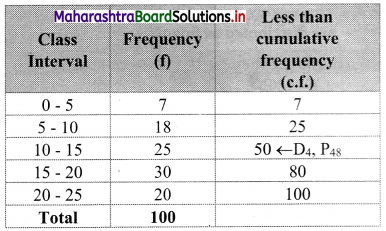
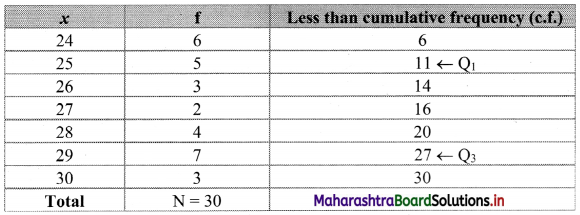
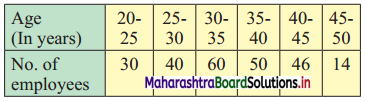
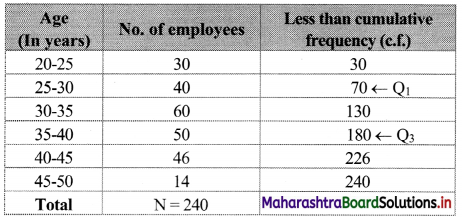
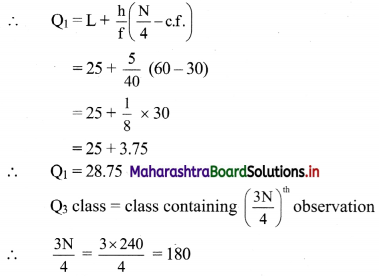
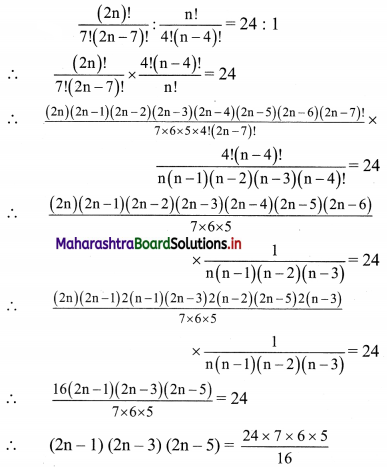
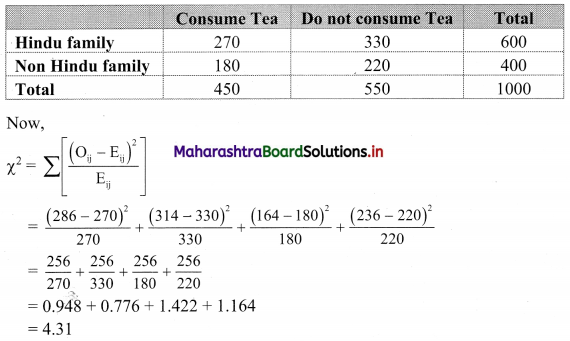
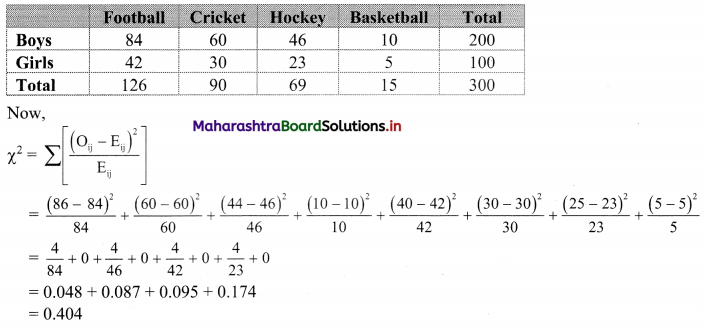
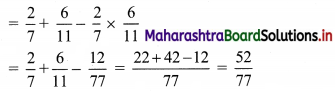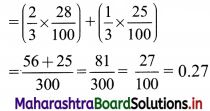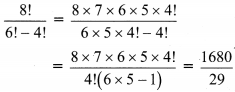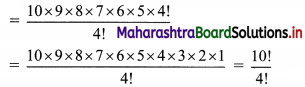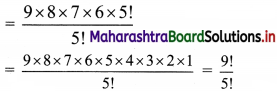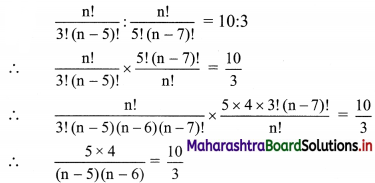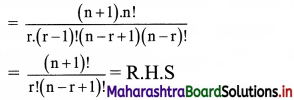Probability Class 11 Commerce Maths 2 Chapter 7 Exercise 7.4 Answers Maharashtra Board

Balbharati Maharashtra State Board 11th Commerce Maths Solution Book Pdf Chapter 7 Probability Ex 7.4 Questions and Answers.

Std 11 Maths 2 Exercise 7.4 Solutions Commerce Maths

Question 1.
Two dice are thrown simultaneously, if at least one of the dice shows a number 5, what is the probability that sum of the numbers on two dice is 9?
Solution:
When two dice are thrown simultaneously, the sample space is
S = {(1, 1), (1, 2), (1, 3), (1, 4), (1, 5), (1, 6), (2, 1), (2, 2), (2, 3), (2, 4), (2, 5), (2, 6), (3, 1), (3, 2), (3, 3), (3, 4), (3, 5), (3, 6), (4, 1), (4, 2), (4, 3), (4, 4), (4, 5), (4, 6), (5, 1), (5, 2), (5, 3), (5, 4), (5, 5), (5, 6), (6, 1), (6, 2), (6, 3), (6, 4), (6, 5), (6, 6)}
∴ n(S) = 36
Let A be the event that at least one die shows number 5.
∴ A = {(1, 5), (2, 5), (3, 5), (4, 5), (5, 1), (5, 2), (5, 3), (5, 4), (5, 5), (5, 6), (6, 5)}
∴ n(A) = 11
∴ P(A) = \(\frac{\mathrm{n}(\mathrm{A})}{\mathrm{n}(\mathrm{S})}=\frac{11}{36}\)
Let B be the event that sum of the numbers on two dice is 9.
∴ B = {(3, 6), (4, 5), (5, 4), (6, 3)}
Also, A ∩ B = {(4, 5), (5, 4)}
∴ n(A ∩ B) = 2
∴ P(A ∩ B) = \(\frac{n(A \cap B)}{n(S)}=\frac{2}{36}\)
∴ Probability of sum of numbers on two dice is 9, given that one dice shows number 5, is given by
P(B/A) = \(\frac{\mathrm{P}(\mathrm{A} \cap \mathrm{B})}{\mathrm{P}(\mathrm{A})}=\frac{\frac{2}{36}}{\frac{11}{36}}=\frac{2}{11}\)

Question 2.
A pair of dice is thrown. If sum of the numbers is an even number, what is the probability that it is a perfect square?
Solution:
When two dice are thrown simultaneously, the sample space is
S = {(1, 1), (1, 2), (1, 3), (1, 4), (1, 5), (1, 6), (2, 1), (2, 2), (2, 3), (2, 4), (2, 5), (2, 6), (3, 1), (3, 2), (3, 3), (3, 4), (3, 5), (3, 6), (4, 1), (4, 2), (4, 3), (4, 4), (4, 5), (4, 6), (5, 1), (5, 2), (5, 3), (5, 4), (5, 5), (5, 6), (6, 1), (6, 2), (6, 3), (6, 4), (6, 5), (6, 6)}
∴ n(S) = 36
Let A be the event that sum of the numbers is an even number.
∴ A = {(1, 1), (1, 3), (1, 5), (2, 2), (2, 4), (2, 6), (3, 1), (3, 3), (3, 5), (4, 2), (4, 4), (4, 6), (5, 1), (5, 3), (5, 5), (6, 2), (6, 4), (6, 6)}
∴ n(A) = 18
∴ P(A) = \(\frac{\mathrm{n}(\mathrm{A})}{\mathrm{n}(\mathrm{S})}=\frac{18}{36}\)
Let B be the event that sum of outcomes is a perfect square.
∴ B = {(1, 3), (2, 2), (3, 1), (3, 6), (4, 5), (5, 4), (6, 3)}
Also, A n B= {(1, 3), (2, 2), (3, 1)}
∴ n(A ∩ B) = 3
∴ P(A ∩ B) = \(\frac{\mathrm{n}(\mathrm{A} \cap \mathrm{B})}{\mathrm{n}(\mathrm{S})}=\frac{3}{36}\)
∴ Probability of sum of the numbers is a perfect square, given that sum of numbers is an even number, is given by
P(B/A) = \(\frac{\mathrm{P}(\mathrm{A} \cap \mathrm{B})}{\mathrm{P}(\mathrm{A})}=\frac{\frac{3}{36}}{\frac{18}{36}}=\frac{3}{18}=\frac{1}{6}\)

Question 3.
A box contains 11 tickets numbered from 1 to 11. Two tickets are drawn at random with replacement. If the sum is even, find the probability that both the numbers are odd.
Solution:
Two tickets can be drawn from 11 tickets with replacement in 11 × 11 = 121 ways.
∴ n(S) = 121
Let A be the event that the sum of two numbers is even.
The event A occurs, if either both the tickets with odd numbers or both the tickets with even numbers are drawn.
There are 6 odd numbers (1, 3, 5, 7, 9, 11) and 5 even numbers (2, 4, 6, 8, 10) from 1 to 11.
∴ n(A) = 6 × 6 + 5 × 5
= 36 + 25
= 61
∴ P(A) = \(\frac{\mathrm{n}(\mathrm{A})}{\mathrm{n}(\mathrm{S})}=\frac{61}{121}\)
Let B be the event that the numbers tickets drawn are odd
∴ n(B) = 6 × 6 = 36
∴ P(B) = \(\frac{\mathrm{n}(\mathrm{B})}{\mathrm{n}(\mathrm{S})}=\frac{36}{121}\)
Since 6 odd numbers are common between A and B.
∴ n(A ∩ B) = 6 × 6 = 36
∴ P(A ∩ B) = \(\frac{\mathrm{n}(\mathrm{A} \cap \mathrm{B})}{\mathrm{n}(\mathrm{S})}=\frac{36}{121}\)
∴ Probability of both the numbers are odd, given that sum is even, is given by
P(B/A) = \(\frac{\mathrm{P}(\mathrm{A} \cap \mathrm{B})}{\mathrm{P}(\mathrm{A})}=\frac{\frac{36}{121}}{\frac{61}{121}}=\frac{36}{61}\)

Question 4.
A card is drawn from a well-shuffled pack of 52 cards. Consider two events A and B as
A: a club card is drawn.
B: an ace card is drawn.
Determine whether events A and B are independent or not.
Solution:
One card can be drawn out of 52 cards in ⁵²C₁ ways.
∴ n(S) = ⁵²C₁
Let A be the event that a club card is drawn.
1 club card out of 13 club cards can be drawn in ¹³C₁ ways.
∴ n(A) = ¹³C₁
∴ P(A) = \(\frac{\mathrm{n}(\mathrm{A})}{\mathrm{n}(\mathrm{S})}=\frac{{ }^{13} \mathrm{C}_{1}}{{ }^{52} \mathrm{C}_{1}}\)
Let B be the event that an ace card is drawn.
An ace card out of 4 aces can be drawn in ⁴C₁ ways.
∴ n(B) = ⁴C₁
∴ P(B) = \(\frac{\mathrm{n}(\mathrm{B})}{\mathrm{n}(\mathrm{S})}=\frac{{ }^{4} \mathrm{C}_{1}}{{ }^{52} \mathrm{C}_{1}}\)
Since 1 card is common between A and B
∴ n(A ∩ B) = ¹C₁
∴ P(A ∩ B) = \(\frac{\mathrm{n}(\mathrm{A} \cap \mathrm{B})}{\mathrm{n}(\mathrm{S})}=\frac{{ }^{1} \mathrm{C}_{1}}{{ }^{52} \mathrm{C}_{1}}=\frac{1}{52}\) …….(i)
∴ P(A) × P(B) = \(\frac{{ }^{13} \mathrm{C}_{1}}{{ }^{52} \mathrm{C}_{1}} \times \frac{{ }^{4} \mathrm{C}_{1}}{{ }^{52} \mathrm{C}_{1}}=\frac{13 \times 4}{52 \times 52}=\frac{1}{52}\) …….(ii)
From (i) and (ii), we get
P(A ∩ B) = P(A) × P(B)
∴ A and B are independent events.

Question 5.
A problem in statistics is given to three students A, B, and C. Their chances of solving the problem are 1/3, 1/4, and 1/5 respectively. If all of them try independently, what is the probability that,
(i) problem is solved?
(ii) problem is not solved?
(iii) exactly two students solve the problem?
Solution:
Let A be the event that student A can solve the problem.
B be the event that student B can solve the problem.
C be the event that student C can solve problem.
∴ P(A) = \(\frac{1}{3}\), P(B) = \(\frac{1}{4}\), P(C) = \(\frac{1}{5}\)
∴ P(A’) = 1 – P(A) = 1 – \(\frac{1}{3}\) = \(\frac{2}{3}\)
P(B’) = 1 – P(B) = 1 – \(\frac{1}{4}\) = \(\frac{5}{4}\)
P(C’) = 1 – P(C) = 1 – \(\frac{1}{5}\) = \(\frac{4}{5}\)
Since A, B, C are independent events
∴ A’, B’, C’ are also independent events
(i) Let X be the event that problem is solved.
Problem can be solved if at least one of the three students solves the problem.
P(X) = P(at least one student solves the problem)
= 1 – P(no student solved problem)
= 1 – P(A’ ∩ B’ ∩ C’)
= 1 – P(A’) P(B’) P(C’)
= 1 – \(\frac{2}{3} \times \frac{3}{4} \times \frac{4}{5}\)
= 1 – \(\frac{2}{5}\)
= \(\frac{3}{5}\)

(ii) Let Y be the event that problem is not solved
∴ P(Y) = P(A’ ∩ B’ ∩ C’)
= P(A’) P(B’) P(C’)
= \(\frac{2}{3} \times \frac{3}{4} \times \frac{4}{5}\)
= \(\frac{2}{5}\)

(iii) Let Z be the event that exactly two students solve the problem.
∴ P(Z) = P(A ∩ B ∩ C’) ∪ P(A ∩ B’ ∩ C) ∪ P(A’ ∩ B ∩ C)
= P(A) . P(B) . P(C’) + P(A) . P(B’) . P(C) + P(A’) . P(B) . P(C)
= \(\left(\frac{1}{3} \times \frac{1}{4} \times \frac{4}{5}\right)+\left(\frac{1}{3} \times \frac{3}{4} \times \frac{1}{5}\right)+\left(\frac{2}{3} \times \frac{1}{4} \times \frac{1}{5}\right)\)
= \(\frac{4}{60}+\frac{3}{60}+\frac{2}{60}\)
= \(\frac{3}{20}\)

Question 6.
The probability that a 50-year old man will be alive till age 60 is 0.83 and the probability that a 45-year old woman will be alive till age 55 is 0.97. What is the probability that a man whose age is 50 and his wife whose age is 45 will both be alive for the next 10 years?
Solution:
Let A be the event that man will be alive at 60.
∴ P(A) = 0.83
Let B be the event that a woman will be alive at 55.
∴ P(B) = 0.97
A ∩ B = Event that both will be alive.
Also, A and B are independent events
∴ P(both man and his wife will be alive) = P(A ∩ B)
= P(A) . P(B)
= 0.83 × 0.97
= 0.8051

Question 7.
In an examination, 30% of the students have failed in subject I, 20% of the students have failed in subject II and 10% have failed in both subjects I and subject II. A student is selected at random, what is the probability that the student
(i) has failed in the subject I, if it is known that he is failed in subject II?
(ii) has failed in at least one subject?
(iii) has failed in exactly one subject?
Solution:
Let A be the event that the student failed in Subject I
B be the event that the student failed in Subject II
Then P(A) = 30% = \(\frac{30}{100}\)
P(B) = 20% = \(\frac{20}{100}\)
and P(A ∩ B) = 10% = \(\frac{10}{100}\)
(i) P (student failed in Subject I, given that he has failed in Subject II) = P(A/B)
\(\frac{\mathrm{P}(\mathrm{A} \cap \mathrm{B})}{\mathrm{P}(\mathrm{B})}=\frac{\left(\frac{10}{100}\right)}{\left(\frac{20}{100}\right)}=\frac{10}{20}=\frac{1}{2}\)

(ii) P(student failed in at least one subject) = P(A ∪ B)
= P(A) + P(B) – P(A ∩ B)
= \(\frac{30}{100}+\frac{20}{100}-\frac{10}{100}\)
= 0.40

(iii) P(student failed in exactly one subject) = P(A) + P(B) – 2P(A ∩ B)
= \(\frac{30}{100}+\frac{20}{100}-2\left(\frac{10}{100}\right)\)
= 0.30

Question 8.
One-shot is fired from each of the three guns. Let A, B, and C denote the events that the target is hit by the first, second and third gun respectively. Assuming that A, B, and C are independent events and that P(A) = 0.5, P(B) = 0.6, and P(C) = 0.8, then find the probability that at least one hit is registered.
Solution:
A be the event that first gun hits the target
B be the event that second gun hits the target
C be the event that third gun hits the target
P(A) = 0.5, P(B) = 0.6, P(C) = 0.8
∴ P(A’) = 1 – P(A) = 1 – 0.5 = 0.5
∴ P(B’) = 1 – P(B) = 1 – 0.6 = 0.4
∴ P(C’) = 1 – P(C) = 1 – 0.8 = 0.2
Now A, B, C are independent events
∴ A’, B’, C are also independent events.
∴ P (at least one hit is registered)
= 1 – P(no hit is registered)
= 1 – P(A’ ∩ B’ ∩ C’)
= 1 – P(A’) P(B’) P(C’)
= 1 – (0.5) (0.4) (0.2)
= 1 – 0.04
= 0.96

Question 9.
A bag contains 10 white balls and 15 black balls. Two balls are drawn in succession without replacement. What is the probability that
(i) first is white and second is black?
(ii) one is white and the other is black?
Solution:
Total number of balls = 10 + 15 = 25
Let S be an event that two balls are drawn at random without replacement in succession
∴ n(S) = ²⁵C₁ × ²⁴C₁ = 25 × 24
(i) Let A be the event that the first ball is white and the second is black.
First white ball can be drawn from 10 white balls in ¹⁰C₁ ways
and second black ball can be drawn from 15 black balls in ¹⁵C₁ ways.
∴ n(A) = ¹⁰C₁ × ¹⁵C₁
∴ P(A) = \(\frac{\mathrm{n}(\mathrm{A})}{\mathrm{n}(\mathrm{S})}=\frac{{ }^{10} \mathrm{C}_{1} \times{ }^{15} \mathrm{C}_{1}}{25 \times 24}=\frac{10 \times 15}{25 \times 24}=\frac{1}{4}\)

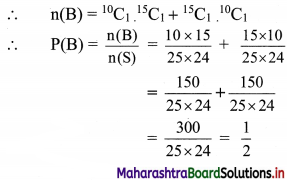
Question 10.
An urn contains 4 black, 5 white, and 6 red balls. Two balls are drawn one after the other without replacement, what is the probability that at least one ball is black?
Solution:
Total number of balls in the urn = 4 + 5 + 6 = 15
Two balls can be drawn without replacement in ¹⁵C₂ = \(\frac{15 \times 14}{1 \times 2}\) = 105 ways
∴ n(S) = 105
Let A be the event that at least one ball is black
i.e., 1 black and 1 non-black or 2 black and 0 non-black.
1 black ball can be drawn out of 4 black balls in ⁴C₁ = 4 ways
and 1 non-black ball can be drawn out of remaining 11 non-black balls in ¹¹C₁ = 11 ways
∴ 1 black and 1 non black ball can be drawn in 4 × 11 = 44 ways
Also, 2 black balls can be drawn from 4 black balls in ⁴C₂ = \(\frac{4 \times 3}{1 \times 2}\) = 6 ways
∴ n(A) = 44 + 6 = 50
∴ Required probability = P(A) = \(\frac{n(A)}{n(S)}=\frac{50}{105}\) = \(\frac{10}{21}\)

Alternate Solution:
Total number of balls = 15
Required probability = 1 – P(neither of two balls is black)
Balls are drawn without replacement
Probability of first non-black ball drawn = \(\frac{11}{15}\)
Probability of second non-black ball drawn = \(\frac{10}{14}\)
Probability of neither of two balls is black = \(\frac{11}{15} \times \frac{10}{14}=\frac{11}{21}\)
Required probability = 1 – \(\frac{11}{21}\) = \(\frac{10}{21}\)

Question 11.
Two balls are drawn from an urn containing 5 green, 3 blue, 7 yellow balls one by one without replacement. What is the probability that at least one ball is blue?
Solution:
Total number of balls in the urn = 5 + 3 + 7 = 15
Out of these 12 are non-blue balls.
Two balls can be drawn from 15 balls without replacement in ¹⁵C₂
= \(\frac{15 \times 14}{1 \times 2}\)
= 105 ways.
∴ n(S) = 105
Let A be the event that at least one ball is blue,
i.e., 1 blue and other non-blue or both are blue.
∴ n(A) = ³C₁ × ¹²C₁ + ³C₂
= 3 × 12 + 3
= 36 + 3
= 39
∴ P(A) = \(\frac{\mathrm{n}(\mathrm{A})}{\mathrm{n}(\mathrm{S})}=\frac{39}{105}=\frac{13}{35}\)

Alternate solution:
Total number of balls in the urn = 15
Required probability = 1 – P(neither of two balls is blue)
Balls are drawn one by one without replacement.
Probability of first non-blue ball drawn = \(\frac{12}{15}\)
Probability of second non-blue ball drawn = \(\frac{11}{14}\)
Probability of neither of two ball is blue = \(\frac{12}{15} \times \frac{11}{14}=\frac{22}{35}\)
∴ Required probability = 1 – \(\frac{22}{35}\) = \(\frac{13}{35}\)

Question 12.
A bag contains 4 blue and 5 green balls. Another bag contains 3 blue and 7 green balls. If one ball ¡s drawn from each bag, what is the Probability that two balls are of the same colour?
Solution:
Let A be the event that a blue ball is drawn from each bag.
Probability of drawing one blue ball out of 4 blue balls where there are a total of 9 balls in the first bag and that of drawing one blue ball out of 3 blue balls where there are a total of 10 balls in the second bag is
P(A) = \(\frac{4}{9} \times \frac{3}{10}\)
Let B be the event that a green ball is drawn from each bag.
Probability of drawing one green ball out of 5 green balls where there are a total of 9 balls in the first bag and that of drawing one green ball out of 7 green balls where there are a total of 10 balls in the second bag is
P(B) = \(\frac{5}{9} \times \frac{7}{10}\)
Since both, the events are mutually exclusive and exhaustive events
∴ P(that both the balls are of the same colour) = P(both are of blue colour) or P(both are of green colour)
= P(A) + P(B)
= \(\frac{4}{9} \times \frac{3}{10}+\frac{5}{9} \times \frac{7}{10}\)
= \(\frac{12}{90}+\frac{35}{90}\)
= \(\frac{47}{90}\)

Question 13.
Two cards are drawn one after the other from a pack of 52 cards with replacement. What is the probability that both the cards are drawn are face cards?
Solution:
Two cards are drawn from a pack of 52 cards with replacement.
∴ n(S) = 52 × 52
Let A be the event that two cards drawn are face cards.
First card from 12 face cards is drawn with replacement in ¹²C₁ = 12 ways
and second face card is drawn from 12 face card in ¹²C₁ = 12 ways after replacement.
∴ n(A) = 12 × 12
∴ P(that both the cards drawn are face cards) = P(A)
= \(\frac{n(A)}{n(S)}=\frac{12 \times 12}{52 \times 52}=\frac{9}{169}\)

11th Commerce Maths Digest Pdf

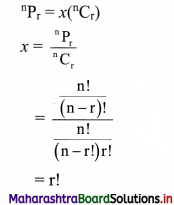
• 11th Commerce Maths Exercise 7.1 Solutions
• 11th Commerce Maths Exercise 7.2 Solutions
• 11th Commerce Maths Exercise 7.3 Solutions
• 11th Commerce Maths Exercise7.4 Solutions
• 11th Commerce Maths Miscellaneous Exercise 7 Solutions

Commercial Mathematics Class 11 Commerce Maths 2 Chapter 9 Exercise 9.7 Answers Maharashtra Board

Balbharati Maharashtra State Board 11th Commerce Maths Solution Book Pdf Chapter 9 Commercial Mathematics Ex 9.7 Questions and Answers.

Std 11 Maths 2 Exercise 9.7 Solutions Commerce Maths

Question 1.
Shantanu has a choice to invest in ₹ 10 shares of two firms at ₹ 13 or at ₹ 16. If the first firm pays a 5% dividend and the second firm pays a 6% dividend per annum, find:
(i) Which firm is paying better?
(ii) If Shantanu invests equally in both the firms and the difference between the return from them is ₹ 30. Find how much, in all, does he invest.
Solution:
(i) For firm 1:
Face value of the share (F.V.) = ₹ 10
Market value of the share (M.V.) = ₹ 13
Dividend = 5%
∴ Annual income from the share = \(\frac{5}{100}\) × 10 = ₹ 0.5

For firm 2:
Face value of the share (F.V.) = ₹ 10
Market value of the share (M.V.) = ₹ 16
Dividend = 5%
∴ Annual income from the share = \(\frac{6}{100}\) × 10 = ₹ 0.6

Since, the profit percentage from firm 1 > profit percentage from firm 2, the first firm is paying better.

(ii) Let ‘X’ be the amount Shantanu invests in each of the firms.
Given that difference between the return from them is ₹ 30, we have

In all, Shantanu invests = 2X
= 2 × 31,200
= ₹ 62,400/-

Question 2.
A dividend of 9% was declared on ₹ 100 shares selling at a certain price in the stock market. If the rate of return is 7.5% calculate
(i) The market price of each share, and
(ii) The amount to be invested to obtain an annual dividend of ₹ 630.
Solution:
(i) Given that,
Face value of the share (F.V) = ₹ 100
Dividend = 9%
Rate of return = 7.5%
Annual income from the share = \(\frac{9}{100}\) × 100 = ₹ 9

∴ The market price of the share is ₹ 120.

(ii) Let ‘X’ be the amount to be invested to obtain an annual dividend of ₹ 630.
∴ 7.5% of X is ₹ 630
∴ \(\frac{7.5}{100}\) × X = 630
∴ X = \(\frac{630 \times 100}{7.5}\)
∴ X = 8400
∴ ₹ 8400 need to be invested to obtain an annual dividend of ₹ 630.

Question 3.
Nilesh has the option of investing his money in 8% ₹ 10 shares at a premium of ₹ 3.50 or 7% ₹ 100 shares at a premium of 20%. Which of the two investments will be more profitable for him?
Solution:
For share 1:
Face value of the share (F.V.) = ₹ 10
Premium = ₹ 3.5
∴ Market value of the share (M.V.) = 10 + 3.5 = ₹ 13.5
Dividend = 8 %
∴ Annual income from the share = \(\frac{8}{100}\) × 10 = ₹ 0.8

= \(\frac{800}{135}\)
= 5.93%

For share 2:
Face value of the share (F.V.) = ₹ 100
Premium = 20%
∴ Market value of the share (M.V.) = 100 + (\(\frac{20}{100}\) × 100) = ₹ 120
Dividend = 7%
Annual income from the share = \(\frac{7}{100}\) × 100 = ₹ 7

Since, profit percentage from share 1 > profit percentage from share 2, investing in the first kind of shares will be more profitable for Nilesh.

Question 4.
Sudhakar invests ₹ 1344 in buying shares of face value ₹ 24 selling at a 12% premium. The dividend on the shares is 15% per annum. Calculate
(i) The number of shares Sudhakar buys, and
(ii) The dividend he receives annually.
Solution:
Given that,
Face value of the share (F.V.) = ₹ 24
Premium = 12%
∴ Market value of the share (M.V.) = 24 + (\(\frac{12}{100}\) × 24) = ₹ 26.88
(i) Sudhakar invests ₹ 1344 in the shares
∴ Number of shares purchased by Sudhakar = \(\frac{1344}{26.88}\) = 50
∴ Sudhakar buys 50 shares.
(ii) Dividend on the share = 15%
Annual income on one share = \(\frac{15}{100}\) × 24 = ₹ 3.6
∴ The total dividend he receives annually = 50 × 3.6 = ₹ 180
∴ Sudhakar receives ₹ 180 as his annual dividend.

Question 5.
Sameer invests ₹ 5625 in a company paying 7% per annum when the share of ₹ 10 stands for ₹ 12.50. Find Sameer’s income from this investment. If he sells 60% of these shares of ₹ 10 each, find his gain or loss in this transaction.
Solution:
Given:
Face value of the share (F.V.) = ₹ 10
Market value of the share (M.V.) = ₹ 12.5
Amount invested in shares = ₹ 5625
∴ Number of shares purchased by Sameer = \(\frac{5625}{12.5}\) = 450
Dividend = 7%
Annual income from one share = \(\frac{7}{100}\) × 10 = ₹ 0.7
∴ Sameer’s income from this investment = number of shares × annual income from one share
= 450 × 0.7
= ₹ 315
Sameer sells 60 % of these shares = \(\frac{60}{100}\) × 450 = 270 shares
Sameer purchased these shares at ₹ 12.5 per share.
∴ Purchase price for these shares = 270 × 12.5 = ₹ 3375
If he sells these shares at ₹ 10 per share, he would receive 270 × 10 = ₹ 2700
∴ In this transaction, Sameer would incur a loss of 3375 – 2700 = ₹ 675

Question 6.
Geeta buys ₹ 100 shares of a company that pays a 15% dividend. She buys the shares at a price from the market that gives her a 10% return on her investment. At what price did she buy each share?
Solution:
Given that,
Face value of the share (F.V.) = ₹ 100
Dividend = 15%
∴ Annual income from the share = \(\frac{15}{100}\) × 100 = ₹ 15
Rate of return on investment = 10%

∴ Geeta bought each share from the market at ₹ 150.

Question 7.
Tejas invests in 9% ₹ 100 shares at ₹ 145 but Shail invests in 7% ₹ 100 shares at ₹ 116. Whose investment is better?
Solution:
Investment of Tejas:
Given that, the Face value of the share (F.V.) = ₹ 100
The market value of the share (M.V.) = ₹ 145
Dividend = 9%
Annual income from the share = \(\frac{9}{100}\) × 100 = ₹ 9

Investment of Shail:
Face value of the share (F.V.) = ₹ 100
Market value of the share (M.V.) = ₹ 116
Dividend = 7%
Annual income from the share = \(\frac{7}{100}\) × 100 = ₹ 7

Since the rate of return for Tejas’s investment is greater than that for Shail’s, Tejas’s investment is better.

Question 8.
A 6% share yields 8%. Find the market value of a ₹ 100 share.
Solution:
Given that,
Face value of the share = ₹ 100
Dividend = 6%
Yield = 8%
Annual income on the share = \(\frac{6}{100}\) × 100 = ₹ 6

∴ The market value of the share = ₹ 75

Question 9.
Ashwini bought ₹ 40 shares at a premium of 40%. Find the income, if Ashwini invests ₹ 14,000 in these shares and receives a dividend at the rate of 8% on the nominal value of the shares.
Solution:
Given,
Face value of the shares (F.V.) = ₹ 40
Premium = 40%
Market value of the shares (M.V.) = 40 + (40 × \(\frac{40}{100}\))
= 40 + 16
= ₹ 56
Ashwini invests ₹ 14000 in these shares
∴ Number of shares bought by Ashwini = \(\frac{Amount Invested}{Market value of one share}\)
= \(\frac{14000}{56}\)
= 250
Dividend = 8%
∴ Annual income on one share = \(\frac{8}{100}\) × 40 = ₹ 3.2
∴ Income of Ashwini on 250 shares = 250 × 3.2 = ₹ 800
∴ Ashwini earns ₹ 800 on her investment.

Question 10.
Mr. Rutvik invests ₹ 30,000 in buying shares of a company that pays a 12% dividend annually on ₹ 100 shares selling at a premium of ₹ 50. Find
(i) The number of shares bought Mr. Rutvik and
(ii) His annual income from the shares.
Solution:
Given that,
Face value of a share (F.V.) = ₹ 100
Premium = ₹ 50
∴ Market value of a share (M.V.) = 100 + 50 = ₹ 150
Dividend =12%
Mr. Rutvik invests ₹ 30,000 in the shares.
(i) Number of shares bought by Mr. Rutvik = \(\frac{Amount invested}{Market value}\)
= \(\frac{30000}{150}\)
= 200

(ii) Dividend on the share = 12%
∴ Annual income from one share = \(\frac{12}{100}\) × 100 = ₹ 12
∴ His annual income from shares = number of shares × income from one share
= 200 × 12
= ₹ 2400

Question 11.
Rasika bought ₹ 40 shares at a discount of 40%. Find the income, if she invests ₹ 12,000 in these shares and receives a dividend at the rate of 11% on the nominal value of the shares.
Solution:
Given,
Face value of the shares (F.V.) = ₹ 40
Discount = 40%
∴ Market value of the shares (M.V.) = 40 – (40 × \(\frac{40}{100}\))
= 40 – 16
= ₹ 24
Rasika invests ₹ 12,000 in these shares.
∴ Number of shares bought by Rasika = \(\frac{Amount invested}{Market value of one share}\)
= \(\frac{12000}{24}\)
= 500
Dividend = 11%
∴ Annual income on one share = \(\frac{11}{100}\) × 40 = ₹ 4.4
∴ Rasika’s income on 200 such shares = 500 × 4.4 = ₹ 2200
∴ Rasika earns ₹ 2200 from her investment.

Question 12.
Nisha invests ₹ 15,840 in buying shares of nominal value ₹ 24 selling at a premium of 10%. The company pays a 15% dividend annually. Find
(i) The dividend she receives annually, and
(ii) The rate of return from her investment.
Solution:
Given that,
Face value of the share (F.V.) = ₹ 24
Premium = 10%
∴ Market value of the share (M.V.) = 24 + (24 × \(\frac{10}{100}\))
= 24 + 2.4
= ₹ 26.4
Dividend = 15%
∴ Annual income on the share = \(\frac{15}{100}\) × 24 = ₹ 3.6
Nisha invests ₹ 15,840 in these shares.
∴ Number of shares bought by Nisha

(i) Annual dividend received by Nisha = Number of shares × annual income from one share
= 600 × 3.6
= ₹ 2160

(ii) Rate of return from the investment

Question 13.
Ashutosh buys 80, ₹ 100 shares at a discount of 20% and receives a return of 12% on his money. Calculate
(i) The amount invested by Ashutosh.
(ii) The rate of dividend paid by the company.
Solution:
Given
Face value of the shares (F.V.) = ₹ 100
Discount = 20%
∴ Market value of the shares (M.V.) = 100 – (100 × \(\frac{20}{100}\)) = ₹ 80
(i) Amount invested by Ashutosh = number of shares × market value of the shares
= 80 × 80
= ₹ 6400

(ii) Ashutosh receives a return of 12% on his money.
∴ Ashutosh’s income from shares = \(\frac{12}{100}\) × 6400 = ₹ 768
∴ Ashutosh’s annual income from one share = \(\frac{768}{80}\) = ₹ 9.6
Annual income from one share = \(\frac{\text { Dividend }}{100} \times \text { Face value }\)
∴ 9 6 = \(\frac{\text { Dividend }}{100} \times 100\)
∴ Rate of dividend = 9.6%

Question 14.
Vaishnavi bought 1000, ₹ 100 shares from the stock market carrying 8% dividend quoted at ₹ 130. A few days later the market value of the shares went up by 10%. Vaishnavi sold all her shares. What was her total income from this transaction?
Solution:
Given that,
Face value of the shares (F.V.) = ₹ 100
The market value of the shares (M.V.) = ₹ 130
Dividend = 8%
Income from the each share = \(\frac{8}{100}\) × 100 = ₹ 8
Number of shares bought by Vaishnavi = 1000
∴ Vaishnavi’s income from dividend = 1000 × 8 = ₹ 8000
The price of the shares went up by 10%
New market value of the shares = 130 + (130 × \(\frac{10}{100}\)) = ₹ 143
Vaishnavi sold the shares at ₹ 143 which she bought at ₹ 130 each.
∴ Vaishnavi’s profit on one share =143 – 130 = ₹ 13
∴ Vaishnavi’s profit after selling all her shares =1000 × 13 = ₹ 13,000
Vaishnavi’s total income from this transaction = Income from dividend + income from sale of shares
= 8,000 + 13,000
= ₹ 21,000
∴ Vaishnavi’s total income from this transaction was ₹ 21,000.

Question 15.
Mr. Dinesh invests ₹ 20,800 in 6% ₹ 100 shares at ₹ 104, and ₹ 14,300 in 10.5% ₹ 100 shares at ₹ 143. What will be his annual income from the shares?
Solution:
For 1st kind of shares,
Face value of shares (F.V.) = ₹ 100
Dividend = 6%
∴ Annual income from one share = \(\frac{6}{100}\) × 100 = ₹ 6
Market value of the share (M.V.) = ₹ 104
Total amount invested = ₹ 20,800

∴ Total income from 1st kind of shares = 200 × 6 = ₹ 1200
For 2nd kind of shares,
Face value of shares (F.V.) = ₹ 100
Dividend = 10.5%
∴ Annual income from one share = \(\frac{10.5}{100}\) × 100 = ₹ 10.5
Market value of the share (M.V.) = ₹ 143
Total amount invested = ₹ 14300

∴ Total income from 2nd kind of shares = 100 × 10.5 = ₹ 1050
∴ Total annual income of Dinesh from both these shares = 1200 + 1050 = ₹ 2250

Question 16.
A company declares a semi-annual dividend of 5%. Daniel has 400 shares of the company. If Daniel’s annual income from the shares is ₹ 1,000, find the face value of each share.
Solution:
Given that,
Semi-annual dividend = 5%
∴ Annual dividend = 10%
Number of shares with Daniel = 400
Daniel’s annual income from the shares = ₹ 1000
∴ Annual income from one share = \(\frac{1000}{400}\) = ₹ 2.5
But annual income from one share = \(\frac{\text { Annualdividend }}{100} \times \text { Face value }\)
∴ 2.5 = \(\frac{10}{100}\) × Face value of the share
∴ Face value of the share = ₹ 25

Question 17.
Bhargav buys 400, ₹ 20 shares at a premium of ₹ 4 each and receives a dividend of 12%. Find
(i) The amount invested by Bhargav.
(ii) His total income from the shares.
(iii) Percentage return on his money.
Solution:
Given that,
Face value of the shares (F.V.) = ₹ 20
Premium = ₹ 4
∴ Market value of the shares (M.V.) = ₹ 24
Dividend = 12%
∴ Annual income from the share = \(\frac{12}{100}\) × 20 = ₹ 2.4
Bhargav buys 400 shares
(i) The amount invested by Bhargav = number of shares × market value
= 400 × 24
= ₹ 9600

(ii) Bhargav’s income from the shares = number of shares × annual income from one share
= 400 × 2.4
= ₹ 960

(iii) Percentage return on Bhargav’s money

∴ Bhargav gets 10% as the rate of return on his money.

Question 18.
Anil buys 350 ₹ 100 shares of a company at a premium of 20% from the market. The company pays 12% dividend annually. Find
(i) the investment made by Anil,
(ii) his annual income from the shares, and
(iii) the rate of return from the shares.
Solution:
Given that,
Face value of shares (F.V.) = ₹ 100
Premium = 20%
∴ Market value of shares (M.V.) = 100 + (\(\frac{20}{100}\) × 100) = ₹ 120
Dividend = 12%
∴ Annual income from one share = \(\frac{12}{100}\) × 100 = ₹ 12
Anil buys 350 shares.
(i) Amount invested by Anil = number of shares × market value
= 350 × 120
= ₹ 42,000

(ii) Anil’s annual income from the shares = number of shares × annual income from one share
= 350 × 12
= ₹ 4200

(iii) Rate of return from shares

∴ The rate of return from Anil’s shares is 10%.

11th Commerce Maths Digest Pdf

• 11th Commerce Maths Exercise 9.1 Solutions
• 11th Commerce Maths Exercise 9.2 Solutions
• 11th Commerce Maths Exercise 9.3 Solutions
• 11th Commerce Maths Exercise 9.4 Solutions
• 11th Commerce Maths Exercise 9.5 Solutions
• 11th Commerce Maths Exercise 9.6 Solutions
• 11th Commerce Maths Exercise 9.7 Solutions
• 11th Commerce Maths Miscellaneous Exercise 9 Solutions

Measures of Dispersion Class 11 Commerce Maths 2 Chapter 2 Exercise 2.2 Answers Maharashtra Board

Balbharati Maharashtra State Board 11th Commerce Maths Solution Book Pdf Chapter 2 Measures of Dispersion Ex 2.2 Questions and Answers.

Std 11 Maths 2 Exercise 2.2 Solutions Commerce Maths

Question 1.
Find the variance and S.D. for the following set of numbers.
7, 11, 2, 4, 9, 6, 3, 7, 11, 2, 5, 8, 3, 6, 8, 8, 2, 6
Solution:
Given data: 7, 11, 2, 4, 9, 6, 3, 7, 11, 2, 5, 8, 3, 6, 8, 8, 2, 6
The tabulated form of the above data is as given below:

We prepare the following table for the calculation of variance and S. D.

Question 2.
Find the variance and S.D. for the following set of numbers.
65, 77, 81, 98, 100, 80, 129
Solution:

Question 3.
Compute the variance and standard deviation for the following data:

Solution:
We prepare the following table for the calculation of variance and S.D.:

Question 4.
Compute the variance and S.D.

Solution:
We prepare the following table for the calculation of variance and S.D.:

Question 5.
The following data gives the age of 100 students in a school. Calculate variance and S.D.

Solution:
We prepare the following table for the calculation of variance and S.D:

Question 6.
The mean and variance of 5 observations are 3 and 2 respectively. If three of the five observations are 1, 3, and 5, find the values of the other two observations.
Solution:


∴ (x₄ – 4)(x₄ – 2) = 0
∴ x₄ = 4 or x₄ = 2
From (i), we get
x₅ = 2 or x₅ = 4
∴ The two numbers are 2 and 4.

Question 7.
Obtain standard deviation for the following data:

Solution:
We prepare the following table for the calculation of standard deviation.

Question 8.
The following distribution was obtained by change of origin and scale of variable X.

If it is given that mean and variance are 59.5 and 413 respectively, determine actual class intervals.
Solution:
Here, Mean = \(\bar{x}\) = 59.5, and
Var(X) = σ² = 413
Let x_i be a mid value of class and
d = \(\frac{x-a}{h}\), where a is assumed mean and h is class width.
We prepare the following table for calculation of mean and variance of d_i.


Now, Var(X) = h². Var(D)
∴ 413 = h² × 4.13
∴ h² = 100
∴ h = 10
Substituting h = 10 in (i), we get
-0.1 × 10 + a = 59.5
∴ -1 + a = 59.5
∴ a = 59.5 + 1
∴ a = 60.5
We prepare the following table to determine actual class intervals for corresponding values of d_i.

∴ The actual class intervals are 15.5 – 25.5, 25.5 – 35.5, …….., 95.5 – 105.5

11th Commerce Maths Digest Pdf

• 11th Commerce Maths Exercise 2.1 Solutions
• 11th Commerce Maths Exercise 2.2 Solutions
• 11th Commerce Maths Exercise 2.3 Solutions
• 11th Commerce Maths Miscellaneous Exercise 2 Solutions

Measures of Dispersion Class 11 Commerce Maths 2 Chapter 2 Miscellaneous Exercise 2 Answers Maharashtra Board

Balbharati Maharashtra State Board 11th Commerce Maths Solution Book Pdf Chapter 2 Measures of Dispersion Miscellaneous Exercise 2 Questions and Answers.

Std 11 Maths 2 Miscellaneous Exercise 2 Solutions Commerce Maths

Question 1.
Find the range for the following data.
116, 124, 164, 150, 149, 114, 195, 128, 138, 203, 144
Solution:
Here, largest value (L) = 203, smallest value (S) = 114
∴ Range = L – S
= 203 – 114
= 89

Question 2.
Given below the frequency distribution of weekly w ages of 400 workers. Find the range.

Solution:
Here, largest value (L) = 40, smallest value (S) = 10
∴ Range = L – S
= 40 – 10
= 30

Question 3.
Find the range of the following data.

Solution:
Here, upper limit of the highest class (L) = 175, lower limit of the lowest class (S) = 115
∴ Range = L – S
= 175 – 115
= 60

Question 4.
The city traffic police issued challans for not observing the traffic rules:

Find Q.D.
Solution:
The given data can be arranged in ascending order as follows:
24, 36, 40, 58, 62, 80
Here, n = 6
Q₁ = value of \(\left(\frac{n+1}{4}\right)^{\text {th }}\) observation
= value of \(\left(\frac{6+1}{4}\right)^{\text {th }}\) observation
= value of (1.75)th observation
= value of 1st observation + 0.75(value of 2nd observation – value of 1st observation)
= 24 + 0.75(36 – 24)
= 24 + 0.75(12)
= 24 + 9
∴ Q₁ = 33
Q₃ = value of 3\(\left(\frac{n+1}{4}\right)^{\text {th }}\) observation
= value of 3\(\left(\frac{6+1}{4}\right)^{\text {th }}\) observation
= value of (3 × 1.75)th observation
= value of (5.25)th observation
= value of 5th observation + 0.25(value of 6th observation – value of 5th observation)
= 62 + 0.25(80 – 62)
= 62 + 0.25(18)
= 62 + 4.5
= 66.5
∴ Q.D. = \(\frac{Q_{3}-Q_{1}}{2}=\frac{66.5-33}{2}=\frac{33.5}{2}\) = 16.75

Question 5.
Calculate Q.D. from the following data.

Solution:
We construct the less than cumulative frequency table as follows:

Here, N = 35
Q₁ class = class containing \(\left(\frac{N}{4}\right)^{\text {th }}\) observation
∴ \(\frac{\mathrm{N}}{4}=\frac{35}{4}\) = 8.75
Cumulative frequency which is just greater than (or equal to) 8.75 is 15.
∴ Q₁ lies in the class 20-30.
∴ L = 20, c.f. = 8, f = 7, h = 10

Question 6.
Calculate the appropriate measure of dispersion for the following data.

Solution:
Since open-ended classes are given, the appropriate measure of dispersion that we can compute is the quartile deviation.
We construct the less than cumulative frequency table as follows:

Here N = 250
Q₁ class class containing \(\left(\frac{N}{4}\right)^{t h}\) observation
∴ \(\frac{\mathrm{N}}{4}=\frac{250}{4}\) = 62.5
Cumulative frequency which is just greater than (or equal to) 62.5 is 65.
∴ Q₁ lies in the class 35-40.
∴ L = 35, c.f. = 15, f = 50, h = 5

The cumulative frequency which is just greater than (or equal to) 187.5 is 190.
∴ Q₃ lies in the class 45-50.
∴ L = 45, c.f. = 150, f = 40, h = 5

Question 7.
Calculate Q.D. of the following data.

Solution:
We construct the less than cumulative frequency table as follows:

Here, N = 120
Q₁ class = class containing \(\left(\frac{\mathrm{N}}{4}\right)^{\mathrm{th}}\) observation
∴ \(\frac{\mathrm{N}}{4}=\frac{120}{4}\) = 30
Cumulative frequency which is just greater than (or equal to) 30 is 35.
∴ Q₁ lies in the class 4-6.
∴ L = 4, c.f. = 15, f = 20, h = 2

Cumulative frequency which is just greater than (or equal to) 90 is 90.
∴ Q₃ lies in the class 10-12.
∴ L = 10, c.f. = 72, f = 18, h = 2

Question 8.
Find variance and S.D. for the following set of numbers.
25, 21, 23, 29, 27, 22, 28, 23, 27, 25 (Given √6.6 = 2.57)
Solution:
We prepare the following table for the calculation of variance and S.D.:

Question 9.
Following data gives no. of goals scored by a team in 90 matches.

Compute the variance and standard deviation for the above data.
Solution:
We prepare the following table for the calculation of variance and S.D:

Question 10.
Compute the arithmetic mean and S.D. and C.V. (Given √296 = 17.20)

Solution:
We prepare the following table for calculation of arithmetic mean and S.D.:

Question 11.
The mean and S.D. of 200 items are found to be 60 and 20 respectively. At the time of calculation, two items were wrongly taken as 3 and 67 instead of 13 and 17. Find the correct mean and variance.
Solution:
Here, n = 200, \(\bar{x}\) = Mean = 60, S.D. = 20
Wrongly taken items are 3 and 67.
Correct items are 13 and 17.
Now, \(\bar{x}\) = 60

Correct value of \(\sum_{i=1}^{n} x_{i}=\sum_{i=1}^{n} x_{i}\) (sum of wrongly taken items) + (sum of correct items)
= 12000 – (3 + 67) + (13 + 17)
= 12000 – 70 + 30
= 11960
Correct value of mean = \(\frac{1}{n}\) × correct value of \(\sum_{i=1}^{n} x_{i}\)
= \(\frac{1}{200}\) × 11960
= 59.8
Now, S.D. = 20
Variance = (S.D.)² = 20²
∴ Variance = 400
∴ \(\frac{1}{\mathrm{n}} \sum_{\mathrm{i}=1}^{\mathrm{n}} x_{\mathrm{i}}^{2}-(\bar{x})^{2}=400\)

Correct value of \(\sum_{i=1}^{n} x_{i}^{2}\)
= \(\sum_{i=1}^{n} x_{i}^{2}\) – (Sum of squares of wrongly taken items) + (Sum of squares of correct items)
= 800000 – (3² + 67²) + (13² + 17²)
= 800000 – (9 + 4489) + (169 + 289)
= 800000 – 4498 + 458
= 795960
∴ Correct value of Variance = (\(\frac{1}{n}\) × \(\sum_{i=1}^{n} x_{i}^{2}\)) – (correct value of \(\bar{x}\))²
= \(\frac{1}{200}\) × 795960 – (59.8)²
= 3979.8 – 3576.04
= 403.76
∴ The correct mean is 59.8 and correct variance is 403.76.

Question 12.
The mean and S.D. of a group of 48 observations are 40 and 8 respectively. If two more observations 60 and 65 are added to the set, find the mean and S.D. of 50 items.
Solution:

Question 13.
The mean height of 200 students is 65 inches. The mean heights of boys and girls are 70 inches and 62 inches respectively and the standard deviations are 8 and 10 respectively. Find the number of boys and combined S.D.
Solution:
Let n₁ and n₂ be the number of boys and girls respectively.
Let n = 200, \(\bar{x}_{\mathrm{c}}\) = 65, \(\bar{x}_{1}\) = 70, \(\bar{x}_{1}\) = 62, σ₁ = 8, σ₂ = 10
Here, n₁ + n₂ = n
∴ n₁ + n₂ = 200 …….(i)
Combined mean is given by

∴ 70n₁ + 62n₂ = 13000
∴ 35n₁ + 31n₂ = 6500 ……..(ii)
Solving (i) and (ii), we get
n₁ = 75, n₂ = 125
Combined standard deviation is given by,

Question 14.
From the following data available for 5 pairs of observations of two variables x and y, obtain the combined S.D. for all 10 observations,
where \(\sum_{i=1}^{n} x_{i}=30, \sum_{i=1}^{n} y_{i}=40, \sum_{i=1}^{n} x_{i}^{2}=225, \sum_{i=1}^{n} y_{i}^{2}=340\)
Solution:

Question 15.
The mean and standard deviations of two brands of watches are given below:

Calculate the coefficient of variation of the two brands and interpret the results.
Solution:

Since C.V. (I) > C.V. (II)
∴ the brand I is more variable.

Question 16.
Calculate the coefficient of variation for the data given below. [Given √3.3 = 1.8166]

Solution:

11th Commerce Maths Digest Pdf

• 11th Commerce Maths Exercise 2.1 Solutions
• 11th Commerce Maths Exercise 2.2 Solutions
• 11th Commerce Maths Exercise 2.3 Solutions
• 11th Commerce Maths Miscellaneous Exercise 2 Solutions

Partition Values Class 11 Commerce Maths 2 Chapter 1 Exercise 1.2 Answers Maharashtra Board

Balbharati Maharashtra State Board 11th Commerce Maths Solution Book Pdf Chapter 1 Partition Values Ex 1.2 Questions and Answers.

Std 11 Maths 2 Exercise 1.2 Solutions Commerce Maths

Question 1.
Calculate D₆ and P₈₅ for the following data:
79, 82, 36, 38, 51, 72, 68, 70, 64, 63
Solution:
The given data can be arranged in ascending order as follows:
36, 38, 51, 63, 64, 68, 70, 72, 79, 82
Here, n = 10
D₆ = value of 6\(\left(\frac{n+1}{10}\right)^{\text {th }}\) observation
= value of 6\(\left(\frac{10+1}{10}\right)^{\text {th }}\) observation
= value of (6 × 1.1)th observation
= value of (6.6)th observation
= value of 6th observation + 0.6(value of 7th observation – value of 6th observation)
= 68 + 0.6(70 – 68)
= 68 + 0.6(2)
= 68 + 1.2
∴ D₆ = 69.2
P₈₅ = value of \(\left(\frac{n+1}{100}\right)^{\text {th }}\) observation
= value of \(\left(\frac{10+1}{100}\right)^{\text {th }}\) observation
= value of (85 × 0. 11)th observation
= value of (9.35)th observation
= value of 9th observation + 0.35(value of 10th observation – value of 9th observation)
= 19 + 0.35(82 – 79)
= 79 + 0.35(3)
= 79 + 1.05
∴ P₈₅ = 80.05

Question 2.
The daily wages (in ₹) of 15 labourers are as follows:
230, 400, 350, 200, 250, 380, 210, 225, 375, 180, 375, 450, 300, 350, 250
Calculate D₈ and P₉₀.
Solution:
The given data can be arranged in ascending order as follows:
180, 200, 210, 225, 230, 250, 250, 300, 350, 350, 375, 375, 380, 400, 450
Here, n = 15
D₈ = value of 8\(\left(\frac{n+1}{10}\right)^{\text {th }}\) observation
= value of 8\(\left(\frac{15+1}{10}\right)^{\text {th }}\) observation
= value of (8 × 1.6)th observation
= value of (12.8)th observation
= value of 12th observation – 0.8(value of 13th observation – value of 12th observation)
= 375 + 0.8(380 – 375)
= 375 + 0.8(5)
= 375 + 4
∴ D₈ = 379
P₉₀ = value of 90\(\left(\frac{n+1}{10}\right)^{\text {th }}\) observation
= value of 90\(\left(\frac{15+1}{100}\right)^{\text {th }}\) observation
= value of (90 × 0.16)th observation
= value of (14.4)th observation
= value of 14th observation + 0.4 (value of 15th observation – value of 14th observation)
= 400 + 0.4(450 – 400)
= 400 + 0.4(50)
= 400 + 20
∴ P₉₀ = 420

Question 3.
Calculate 2nd decile and 65th percentile for the following:

Solution:
We construct the less than cumulative frequency table as given below:

Here, n = 200
D₂ = value of 2\(\left(\frac{n+1}{10}\right)^{\text {th }}\) observation
= value of 2\(\left(\frac{200+1}{10}\right)^{\text {th }}\) observation
= value of (2 × 20.1)th observation
= value of (40.2)th observation
Cumulative frequency which is just greater than (or equal to) 40.2 is 58.
∴ D₂ = 120
P₆₅ = value of 65\(\left(\frac{n+1}{100}\right)^{\text {th }}\) observation
= value of 65\(\left(\frac{200+1}{100}\right)^{\text {th }}\) observation
= value of (65 × 2.01)th observation
= value of (130.65)th observation
The cumulative frequency which is just greater than (or equal to) 130.65 is 150.
∴ P₆₅ = 280

Question 4.
From the following data calculate the rent of the 15th, 65th, and 92nd house.

Solution:
Arranging the given data in ascending order.

Here, n = 100
P₁₅ = value of 15
= value of 15\(\left(\frac{n+1}{100}\right)^{\text {th }}\) observation
= value of 15\(\left(\frac{100+1}{100}\right)^{\text {th }}\) observation
= value of (15 × 1.01 )th observation
= value of (15.15)th observation
Cumulative frequency which is just greater than (or equal to) 15.15 is 25.
∴ P₁₅ = 11000
P₆₅ = value of 65\(\left(\frac{n+1}{100}\right)^{\text {th }}\)observation
= value of 65\(\left(\frac{100+1}{100}\right)^{\text {th }}\) observation
= value of (65 × 1.01)th observation
= value of (65.65)th observation
Cumulative frequency which is just greater than (or equal to) 65.65 is 70.
∴ P₆₅ = 14000
P₉₂ = value of 92\(\left(\frac{n+1}{100}\right)^{\text {th }}\) observation
= value of 92\(\left(\frac{100+1}{100}\right)^{\text {th }}\) observation
= value of (92 × 1.01)th observation
= value of (92.92)th observation
Cumulative frequency which is just greater than (or equal to) 92.92 is 98.
∴ P₉₂ = 17000

Question 5.
The following frequency distribution shows the weight of students in a class.

(a) Find the percentage of students whose weight is more than 50 kg.
(b) If the weight column provided is of mid values then find the percentage of students whose weight is more than 50 kg.
Solution:
(a) Let the percentage of students weighing less than 50 kg be x.
∴ Px = 50

From the table, out of 20 students, 84 students have their weight less than 50 kg.
∴ Number of students weighing more than 50 kg = 120 – 84 = 36
∴ Percentage of students having there weight more than 50 kg = \(\frac{36}{120}\) × 100 = 30%

(b) The difference between any two consecutive mid values of weight is 5 kg.
The class intervals must of width 5, with 40, 45,….. as their mid values.
∴ The class intervals will be 37.5 – 42.5, 42.5 – 47.5, etc.
We construct the less than cumulative frequency table as given below:

Here, N = 120
Let P_x = 50
The value 50 lies in the class 47.5 – 52.5
∴ L = 47.5, h = 5, f = 29, c.f. = 55

∴ x = 58 (approximately)
∴ 58% of students are having weight below 50 kg.
∴ Percentage of students having weight above 50 kg is 100 – 58 = 42
∴ 42% of students are having weight above 50 kg.

Question 6.
Calculate D₄ and P₄₈ from the following data:

Solution:
The difference between any two consecutive mid values is 5, the width of class interval = 5
∴ Class interval with mid-value 2.5 is 0 – 5
Class interval with mid value 7.5 is 5 – 10, etc.
We construct the less than cumulative frequency table as given below:

Here, N = 100
D₄ class = class containing \(\left(\frac{4 \mathrm{N}}{10}\right)^{\text {th }}\) observation
∴ \(\frac{4 \mathrm{N}}{10}=\frac{4 \times 100}{10}\) = 40
Cumulative frequency which is just greater than (or equal to) 40 is 50.
∴ D₄ lies in the class 10 – 15.
∴ L = 10,h = 5, f = 25, c.f. = 25
∴ D₄ = \(\mathrm{L}+\frac{\mathrm{h}}{\mathrm{f}}\left(\frac{4 \mathrm{~N}}{10}-\text { c.f. }\right)\)
= 10 + \(\frac{5}{25}\) (40 – 25)
= 10 + \(\frac{1}{5}\) (15)
= 10 + 3
∴ D₄ = 13
P₄₈ class = class containing \(\left(\frac{48 \mathrm{~N}}{100}\right)^{\text {th }}\) observation
∴ \(\frac{48 \mathrm{~N}}{100}=\frac{48 \times 100}{100}\) = 48
Cumulative frequency which is just greater than (or equal to) 48 is 50.
∴ P₄₈ lies in the class 10 – 15.
∴ L = 10, h = 5, f = 25, c.f. = 25
∴ P₄₈ = \(\mathrm{L}+\frac{\mathrm{h}}{\mathrm{f}}\left(\frac{48 \mathrm{~N}}{100}-\text { c.f. }\right)\)
= 10 + \(\frac{5}{25}\) (48 – 25)
= 10 + \(\frac{1}{5}\) (23)
= 10 + 4.6
∴ P₄₈ = 14.6

Question 7.
Calculate D₉ and P₂₀ of the following distribution.

Solution:
We construct the less than cumulative frequency table as given below:

Here, N = 240
D₉ class = class containing \(\left(\frac{9 \mathrm{~N}}{10}\right)^{\mathrm{th}}\) observation
∴ \(\frac{9 \mathrm{~N}}{10}=\frac{9 \times 240}{10}\) = 216
Cumulative frequency which is just greater than (or equal to) 216 is 225.
∴ D₉ lies in the class 80 – 100.
∴ L = 80, h = 20, f = 90, c.f. = 135
∴ D₉ = \(L+\frac{h}{f}\left(\frac{9 N}{10}-c . f .\right)\)
= 80 + \(\frac{20}{90}\)(216 – 135)
= 80 + \(\frac{2}{9}\)(81)
= 80 + 18
∴ D₉ = 98
P₂₀ class = class containing \(\left(\frac{20 \mathrm{~N}}{100}\right)^{\text {th }}\) observation
∴ \(\frac{20 \mathrm{~N}}{100}=\frac{20 \times 240}{100}\) = 48
Cumulative frequency which is just greater than (or equal to) 48 is 50.
∴ P₂₀ lies in the class 40 – 60.
∴ L = 40, h = 20, f = 35, c.f. = 15

∴ P₂₀ = 58.86

Question 8.
Weekly wages for a group of 100 persons are given below:

D₃ for this group is ₹ 1100. Calculate the missing frequencies.
Solution:
Let a and b be the missing frequencies of class 500 – 1000 and class 2000 – 2500 respectively.
We construct the less than cumulative frequency table as given below:

Here, N = 62 + a + b
Since, N = 100
∴ 62 + a + b = 100
∴ a + b = 38 …..(i)
Given, D₃ = 1100
∴ D₃ lies in the class 1000 – 1500.
∴ L = 1000, h = 500, f = 25, c.f. = 7 + a
∴ \(\frac{3 \mathrm{~N}}{10}=\frac{3 \times 100}{10}=30\)
∴ D₃ = \(\mathrm{L}+\frac{\mathrm{h}}{\mathrm{f}}\left(\frac{3 \mathrm{~N}}{10}-\mathrm{c} . \mathrm{f} .\right)\)
∴ 1100 = 1000 + \(\frac{500}{25}\) [30 – (7 + a)]
∴ 1100 – 1000 = 20(30 – 7 – a)
∴ 100 = 20(23 – a)
∴ 100 = 460 – 20a
∴ 20a = 460 – 100
∴ 20a = 360
∴ a = 18
Substituting the value of a in equation (i), we get
18 + b = 38
∴ b = 38 – 18 = 20
∴ 18 and 20 are the missing frequencies of the class 500 – 1000 and class 2000 – 2500 respectively.

Question 9.
The weekly profit (in rupees) of 100 shops are distributed as follows:

Find the limits of the profit of middle 60% of the shops.
Solution:
To find the limits of the profit of the middle 60% of the shops, we have to find P₂₀ and P₈₀.
We construct the less than cumulative frequency table as given below:

Here, N = 100
P₂₀ class = class containing \(\left(\frac{20 \mathrm{N}}{100}\right)^{\text {th }}\) observation
∴ \(\frac{20 \mathrm{N}}{100}=\frac{20 \times 100}{100}=20\)
Cumulative frequency which is just greater than (or equal to) 20 is 26.
∴ P₂₀ lies in the class 1000 – 2000.
∴ L = 1000, h = 1000, f = 16, c.f. = 10
∴ P₂₀ = \(L+\frac{h}{f}\left(\frac{20 \mathrm{~N}}{100}-\text { c.f. }\right)\)
= 1000 + \(\frac{1000}{16}\) (20 – 10)
= 1000 + \(\frac{125}{2}\) (10)
= 1000 + 625
∴ P₂₀ = 1625
P₈₀ class = class containing \(\left(\frac{80 \mathrm{~N}}{100}\right)^{\text {th }}\) observation
∴ \(\frac{80 \mathrm{~N}}{100}=\frac{80 \times 100}{100}=80\)
Cumulative frequency which is just greater than (or equal to) 80 is 92.
∴ P₈₀ lies in the class 4000 – 5000.
∴ L = 4000, h = 1000, f = 20, c.f. = 72
∴ P₈₀ = \(L+\frac{h}{f}\left(\frac{80 \mathrm{~N}}{100}-\text { c.f. }\right)\)
= 4000 + \(\frac{1000}{20}\)(80 – 72)
= 4000 + 50(8)
= 4000 + 400
∴ P₈₀ = 4400
∴ the profit of middle 60% of the shops lie between the limits ₹ 1,625 to ₹ 4,400.

Question 10.
In a particular factory, workers produce various types of output units. The following distribution was obtained:

Find the percentage of workers who have produced less than 82 output units.
Solution:
Since the given data is not continuous, we have to convert it into a continuous form by subtracting 0.5 from the lower limit and adding 0.5 to the upper limit of every class interval.
∴ the class intervals will be 69.5 – 74.5, 74.5 – 79.5, etc.
We construct the less than cumulative frequency table as given below:

Here, N = 445
Let P_x = 82
The value 82 lies in the class 79.5 – 84.5
∴ L = 79.5, h = 5, f = 50, c.f. = 85

∴ 24.72% of workers produced less than 82 output units.

11th Commerce Maths Digest Pdf

• 11th Commerce Maths Exercise 1.1 Solutions
• 11th Commerce Maths Exercise 1.2 Solutions
• 11th Commerce Maths Exercise  1.3 Solutions
• 11th Commerce Maths Miscellaneous Exercise 1 Solutions

Measures of Dispersion Class 11 Commerce Maths 2 Chapter 2 Exercise 2.1 Answers Maharashtra Board

Balbharati Maharashtra State Board 11th Commerce Maths Solution Book Pdf Chapter 2 Measures of Dispersion Ex 2.1 Questions and Answers.

Std 11 Maths 2 Exercise 2.1 Solutions Commerce Maths

Question 1.
Find range of the following data:
575, 609, 335, 280, 729, 544, 852, 427, 967, 250
Solution:
Here, largest value (L) = 967, smallest value (S) = 250
∴ Range = L – S
= 967 – 250
= 717

Question 2.
The following data gives number of typing mistakes done by Radha during a week. Find the range of the data.

Solution:
Here, largest value (L) = 21, smallest value (S) = 10
∴ Range = L – S
= 21 – 10
= 11

Question 3.
Find range for the following data:

Solution:
Here, upper limit of the highest class (L) = 72, lower limit of the lowest class (S) = 62
∴ Range = L – S
= 72 – 62
= 10

Question 4.
Find the Q. D. for the following data.
3, 16, 8, 15, 19, 11, 5, 17, 9, 5, 3.
Solution:
The given data can be arranged in ascending order as follows:
3, 3, 5, 5, 8, 9, 11, 15, 16, 17, 19
Here, n = 11
Q₁ = value of \(\left(\frac{n+1}{4}\right)^{\text {th }}\) observation
= value of \(\left(\frac{11+1}{4}\right)^{\text {th }}\) observation
= value of 3rd observation
∴ Q₁ = 5
Q₃ = value of 3\(\left(\frac{n+1}{4}\right)^{\text {th }}\) observation
= value of 3\(\left(\frac{11+1}{4}\right)^{\text {th }}\) observation
= value of (3 × 3)th observation
= value of 9th observation
= 16
∴ Q.D.= \(\frac{\mathrm{Q}_{3}-\mathrm{Q}_{1}}{2}\)
= \(\frac{16-5}{2}\)
= \(\frac{11}{2}\)
= 5.5

Question 5.
Given below are the prices of shares of a company for the last 10 days. Find Q.D.:
172, 164, 188, 214, 190, 237, 200, 195, 208, 230.
Solution:
The given data can be arranged in ascending order as follows:
164, 172, 188, 190, 195, 200, 208, 214, 230, 237
Here, n = 10
Q₁ = value of \(\left(\frac{n+1}{4}\right)^{\text {th }}\) observation
= value of \(\left(\frac{10+1}{4}\right)^{\text {th }}\) observation
= value of (2.75)th observation
= value of 2nd observation + 0.75(value of 3rd observation – value of 2nd observation)
= 172 + 0.75(188 – 172)
= 172 + 0.75(16)
= 172 + 12
= 184
∴ Q₃ = value of 3\(\left(\frac{n+1}{4}\right)^{\text {th }}\) observation
= value of 3\(\left(\frac{10+1}{4}\right)^{\text {th }}\) observation
= value of (3 × 2.75)th observation
= value of (8.25)th observation
= value of 8th observation + 0.25(value of 9th observation – value of 8th observation)
= 214 + 0.25(230 – 214)
= 214 + 0.25(16)
= 214 + 4
= 218
∴ Q.D. = \(\frac{\mathrm{Q}_{3}-\mathrm{Q}_{1}}{2}\)
= \(\frac{218-184}{2}\)
= \(\frac{34}{2}\)
= 17

Question 6.
Calculate Q.D. for the following data.

Solution:
Since the given data is arranged in ascending order, we construct less than cumulative frequency table as follows:

Here, n = 30
Q₁ = value of \(\left(\frac{\mathrm{n}+1}{4}\right)^{\mathrm{th}}\) observation
= value of \(\left(\frac{30+1}{4}\right)^{\text {th }}\) observation
= value of (7.75)th observation
Cumulative frequency which is just greater than (or equal to) 7.75 is 11.
∴ Q₁ = 25
Q₃ = value of \(\left[3\left(\frac{\mathrm{n}+1}{4}\right)\right]^{\mathrm{th}}\) observation
= value of \(\left[3\left(\frac{30+1}{4}\right)\right]^{\text {th }}\) observation
= value of (3 × 7.75)th observation
= value of (23.25)th observation
Cumulative frequency which is just greater than (or equal to) 23.25 is 27.
∴ Q₃ = 29
∴ Q.D. = \(\frac{Q_{3}-Q_{1}}{2}\)
= \(\frac{29-25}{2}\)
∴ Q.D. = 2

Question 7.
Following data gives the age distribution of 240 employees of a firm. Calculate Q.D. of the distribution.

Solution:
We construct the less than cumulative frequency table as follows:

Here, N = 240
Q₁ class = class containing \(\left(\frac{\mathrm{N}}{4}\right)^{\mathrm{th}}\) observation
∴ \(\frac{N}{4}=\frac{240}{4}\) = 60
Cumulative frequency which is just greater than (or equal to) 60 is 70.
∴ Q₁ lies in the class 25 – 30.
∴ L = 25, c.f. = 30, f = 40, h = 5

Cumulative frequency which is just greater than (or equal to) 180 is 180.
∴ Q₃ lies in the class 35-40.
∴ L = 35, c.f. = 130, f = 50, h = 5

Question 8.
Following data gives the weight of boxes. Calculate Q.D. for the data.

Solution:

Here, N = 60
Q₁ class = class containing \(\left(\frac{\mathrm{N}}{4}\right)^{\text {th }}\) observation
∴ \(\frac{\mathrm{N}}{4}=\frac{60}{4}\) = 15
Cumulative frequency which is just greater than (or equal to) 15 is 26.
∴ Q₁ lies in the class 14 – 16.
∴ L = 14, c.f. = 10, f = 16, h = 2

Cumulative frequency which is just greater than (or equal to) 45 is 58.
∴ Q₃ lies in the class 18 – 20.
∴ L = 18, c.f. = 40, f = 18, h = 2

11th Commerce Maths Digest Pdf

• 11th Commerce Maths Exercise 2.1 Solutions
• 11th Commerce Maths Exercise 2.2 Solutions
• 11th Commerce Maths Exercise 2.3 Solutions
• 11th Commerce Maths Miscellaneous Exercise 2 Solutions

Probability Class 11 Commerce Maths 2 Chapter 7 Miscellaneous Exercise 7 Answers Maharashtra Board

Balbharati Maharashtra State Board 11th Commerce Maths Solution Book Pdf Chapter 7 Probability Miscellaneous Exercise 7 Questions and Answers.

Std 11 Maths 2 Miscellaneous Exercise 7 Solutions Commerce Maths

Question 1.
From a group of 2 men (M₁, M₂) and three women (W₁, W₂, W₃), two persons are selected. Describe the sample space of the experiment. If E is the event in which one man and one woman are selected, then which are the cases favourable to E?
Solution:
Let S be the sample space of the given event.
∴ S = {(M₁, M₂), (M₁, W₁), (M₁, W₂), (M₁, W₃), (M₂, W₁), (M₂, W₂), (M₂, W₃), (W₁, W₂) (W₁, W₃), (W₂, W₃)}
Let E be the event that one man and one woman are selected.
∴ E = {(M₁, W₁), (M₁, W₂), (M₁, W₃), (M₂, W₁), (M₂, W₂), (M₂, W₃)}
Here, the order is not important in which 2 persons are selected e.g. (M₁, M₂) is the same as (M₂, M₁)

Question 2.
Three groups of children contain respectively 3 girls and 1 boy, 2 girls and 2 boys and 1 girl and 3 boys. One child is selected at random from each group. What is the chance that the three selected consist of 1 girl and 2 boys?
Solution:

Let G₁, G₂, G₃ denote events for selecting a girl,
and B₁, B₂, B₃ denote events for selecting a boy from 1st, 2nd and 3rd groups respectively.
Then P(G₁) = \(\frac{3}{4}\), P(G₂) = \(\frac{2}{4}\), P(G₃) = \(\frac{1}{4}\)
P(B₁) = \(\frac{1}{4}\), P(B₂) = \(\frac{2}{4}\), P(B₃) = \(\frac{3}{4}\)
Where G₁, G₂, G₃, B₁, B₂ and B₃ are mutually exclusive events.
Let E be the event that 1 girl and 2 boys are selected
∴ E = (G₁ ∩ B₂ ∩ B₃) ∪ (B₁ ∩ G₂ ∩ B₃) ∪ (B₁ ∩ B₂ ∩ G₃)
∴ P(E) = P(G₁ ∩ B₂ ∩ B₃) + P(B₁ ∩ G₂ ∩ B₃) + P(B₁ ∩ B₂ ∩ G₃)

Question 3.
A room has 3 sockets for lamps. From a collection of 10 light bulbs, 6 are defective. A person selects 3 at random and puts them in every socket. What is the probability that the room, will be lit?
Solution:
Total number of bulbs = 10
Number of defective bulbs = 6
∴ Number of non-defective bulbs = 4
3 bulbs can be selected out of 10 light bulbs in ¹⁰C₃ ways.
∴ n(S) = ¹⁰C₃
Let A be the event that room is lit.
∴ A’ is the event that the room is not lit.
For A’ the bulbs should be selected from the 6 defective bulbs.
This can be done in ⁶C₃ ways.
∴ n(A’) = ⁶C₃
∴ P(A’) = \(\frac{\mathrm{n}\left(\mathrm{A}^{\prime}\right)}{\mathrm{n}(\mathrm{S})}=\frac{{ }^{6} \mathrm{C}_{3}}{{ }^{10} \mathrm{C}_{3}}\)
∴ P(Room is lit) = 1 – P(Room is not lit)

Question 4.
There are 2 red and 3 black balls in a bag. 3 balls are taken out at random from the bag. Find the probability of getting 2 red and 1 black ball or 1 red and 2 black balls.
Solution:
There are 2 + 3 = 5 balls in the bag and 3 balls can be drawn out of these in
⁵C₃ = \(\frac{5 \times 4 \times 3}{1 \times 2 \times 3}\) = 10 ways.
∴ n(S) = 10
Let A be the event that 2 balls are red and 1 ball is black
2 red balls can be drawn out of 2 red balls in ²C₂ = 1 way
and 1 black ball can be drawn out of 3 black balls in ³C₁ = 3 ways.
∴ n(A) = ²C₂ × ³C₁ = 1 × 3 = 3
∴ P(A) = \(\frac{\mathrm{n}(\mathrm{A})}{\mathrm{n}(\mathrm{S})}=\frac{3}{10}\)
Let B be the event that 1 ball is red and 2 balls are black
1 red ball out of 2 red balls can be drawn in ²C₁ = 2 ways
and 2 black balls out of 3 black balls can be drawn in ³C₂ = \(\frac{3 \times 2}{1 \times 2}\) = 3 ways.
∴ n(B) = ²C₁ × ³C₂ = 2 × 3 = 6
∴ P(B) = \(\frac{\mathrm{n}(\mathrm{B})}{\mathrm{n}(\mathrm{S})}=\frac{6}{10}\)
Since A and B are mutually exclusive and exhaustive events
∴ P(A ∩ B) = 0
∴ Required probability = P(A ∪ B) = P(A) + P(B)
= \(\frac{3}{10}+\frac{6}{10}\)
= \(\frac{9}{10}\)

Question 5.
A box contains 25 tickets numbered 1 to 25. Two tickets are drawn at random. What is the probability that the product of the numbers is even?
Solution:
Two tickets can be drawn out of 25 tickets in ²⁵C₂ = \(\frac{25 \times 24}{1 \times 2}\) = 300 ways.
∴ n(S) = 300
Let A be the event that product of two numbers is even.
This is possible if both numbers are even, or one number is even and other is odd.
As there are 13 odd numbers and 12 even numbers from 1 to 25.
∴ n(A) = ¹²C₂ + ¹²C₁ × ¹³C₁
= \(\frac{12 \times 11}{1 \times 2}\) + 12 × 13
= 66 + 156
= 222
∴ Required probability = P(A)
= \(\frac{\mathrm{n}(\mathrm{A})}{\mathrm{n}(\mathrm{S})}\)
= \(\frac{222}{300}\)
= \(\frac{37}{50}\)

Question 6.
A, B and C are mutually exclusive and exhaustive events associated with the random experiment. Find P(A), given that
P(B) = \(\frac{3}{2}\) P(A) and P(C) = \(\frac{1}{2}\) P(B)
Solution:
P(B) = \(\frac{3}{2}\) P(A) and P(C) = \(\frac{1}{2}\) P(B)
Since A, B, C are mutually exclusive and exhaustive events,

Question 7.
An urn contains four tickets marked with numbers 112, 121, 122, 222, and one ticket is drawn at random. Let A_i (i = 1, 2, 3) be the event that ith digit of the number of the ticket drawn is 1. Discuss the independence of the events A₁, A₂, and A₃.
Solution:
One ticket can be drawn out of 4 tickets in ⁴C₁ = 4 ways.
∴ n(S) = 4
According to the given information,
Let A₁ be the event that 1st digit of the number of tickets is 1
A₂ be the event that the 2nd digit of the number of tickets is 1
A₃ be the event that the 3rd digit of the number of tickets is 1
∴ A₁ = {112, 121, 122}, A₂ = {112}, A₃ = {121}

∴ A₁, A₂, A₃ are not pairwise independent
For mutual independence of events A₁, A₂, A₃
We require to have
P(A₁ ∩ A₂ ∩ A₃) = P(A₁) P(A₂) P(A₃)
and P(A₁) P(A₂) = P(A₁ ∩ A₂),
P(A₂) P(A₃) = P(A₂ ∩ A₃),
P(A₁) P(A₃) = P(A₁ ∩ A₃)
∴ From (iii),
A₁, A₂, A₃ are not mutually independent.

Question 8.
The odds against a certain event are 5 : 2 and the odds in favour of another independent event are 6 : 5. Find the chance that at least one of the events will happen.
Solution:
Let A and B be two independent events.
Odds against A are 5 : 2
∴ the probability of occurrence of event A is given by
P(A) = \(\frac{2}{5+2}=\frac{2}{7}\)
Odds in favour of B are 6 : 5
∴ the probability of occurrence of event B is given by
P(B) = \(\frac{6}{6+5}=\frac{6}{11}\)
∴ P(at least one event will happen) = P(A ∪ B)
= P(A) + P(B) – P(A ∩ B)
= P(A) + P(B) – P(A) P(B) ……[∵ A and B are independent events]

Question 9.
The odds against a husband who is 55 years old living till he is 75 is 8 : 5 and it is 4 : 3 against his wife who is now 48, living till she is 68. Find the probability that
(i) the couple will be alive 20 years hence
(ii) at least one of them will be alive 20 years hence.
Solution:
Let A be the event that husband would be alive after 20 years.
Odds against A are 8 : 5
∴ the probability of occurrence of event A is given by
P(A) = \(\frac{5}{8+5}=\frac{5}{13}\)
∴ P(A’) = 1 – P(A)
= 1 – \(\frac{5}{13}\)
= \(\frac{8}{13}\)
Let B be the event that wife would be alive after 20 years.
Odds against B are 4 : 3
∴ the probability of occurrence of event B is given by
P(B) = \(\frac{3}{4+3}=\frac{3}{7}\)
∴ P(B’) = 1 – P(B)
= 1 – \(\frac{3}{7}\)
= \(\frac{4}{7}\)
Since A and B are independent events
∴ A’ and B’ are also independent events
(i) Let X be the event that both will be alive after 20 years.
∴ P(X) = (A ∩ B)
∴ P(X) = P(A) . P(B)
= \(\frac{5}{13} \times \frac{3}{7}\)
= \(\frac{15}{91}\)

(ii) Let Y be the event that at least one will be alive after 20 years.
∴ P(Y) = P(at least one would be alive)
= 1 – P(both would not be alive)
= 1 – P(A’ ∩ B’)
= 1 – P(A’). P(B’)
= 1 – \(\frac{8}{13} \times \frac{4}{7}\)
= 1 – \(\frac{32}{91}\)
= \(\frac{59}{91}\)

Question 10.
Two throws are made, the first with 3 dice and the second with 2 dice. The faces of each die are marked with the number 1 to 6. What is the probability that the total in the first throw is not less than 15 and at the same time the total in the second throw is not less than 8?
Solution:
When 3 dice are thrown, then the sample space S₁ has 6 × 6 × 6 = 216 sample points.
∴ n(S₁) = 216
Let A be the event that the sum of the numbers is not less than 15.
∴ A = {(3, 6, 6), (4, 5, 6), (4, 6, 5), (4, 6, 6), (5, 4, 6), (5, 5, 5), (5, 5, 6), (5, 6, 4), (5, 6, 5), (5, 6, 6), (6, 3, 6), (6, 4, 5), (6, 4, 6), (6, 5, 4), (6, 5, 5), (6, 5, 6), (6, 6, 3), (6, 6, 4), (6, 6, 5), (6, 6, 6)}
∴ n(A) = 20
∴ P(A) = \(\frac{\mathrm{n}(\mathrm{A})}{\mathrm{n}\left(\mathrm{S}_{1}\right)}=\frac{20}{216}=\frac{5}{54}\)
When 2 dice are thrown, the sample space S₂ has 6 × 6 = 36 sample points.
∴ n(S₂) = 36
Let B be the event that sum of numbers is not less than 8.
∴ B = {(2, 6), (3, 5), (3,6), (4, 4), (4, 5), (4, 6), (5, 3), (5, 4), (5, 5), (5, 6), (6, 2), (6, 3), (6, 4), (6, 5), (6, 6)}
∴ n(B) = 15
∴ P(B) = \(\frac{\mathrm{n}(\mathrm{B})}{\mathrm{n}\left(\mathrm{S}_{2}\right)}=\frac{15}{36}=\frac{5}{12}\)
A ∩ B = Event that the total in the first throw is not less than 15 and at the same time the total in the second throw is not less than 8.
∴ A and B are independent events
∴ P(A ∩ B) = P(A) . P(B)
= \(\frac{5}{54} \times \frac{5}{12}\)
= \(\frac{25}{648}\)

Question 11.
Two-thirds of the students in a class are boys and the rest are girls. It is known that the probability of a girl getting first class is 0.25 and that of a boy getting is 0.28. Find the probability that a student chosen at random will get first class.
Solution:
Let A be the event that student chosen is a boy
B be the event that student chosen is a girl
C be the event that student gets first class
∴ P(A) = \(\frac{2}{3}\), P(B) = \(\frac{1}{3}\)
Probability of student getting first class, given that student is boy
Probability of student getting first class given that student is a girl, is
P(C/A) = 0.28 = \(\frac{28}{100}\)
and P(C/B) = 0.25 = \(\frac{25}{100}\)
∴ Required probability = P((A ∩ C) ∪ (B ∩ C))
Since A ∩ C and B ∩ C are mutually exclusive events
∴ Required probability = P(A ∩ C) + P(B ∩ C)
= P(A) . P(C/A) + P(B) . P(C/B)

Question 12.
A number of two digits is formed using the digits 1, 2, 3,……, 9. What is the probability that the number so chosen is even and less than 60?
Solution:
The number of two digits can be formed from the given 9 digits in 9 × 9 = 81 different ways.
∴ n(S) = 81
Let A be the event that the number is even and less than 60.
Since the number is even, the unit place of two digits can be filled in ⁴P₁ = 4 different ways by any one of the digits 2, 4, 6, 8.
Also the number is less than 60, so tenth place can be filled in ⁵P₁ = 5 different ways by any one of the digits 1, 2, 3, 4, 5.
∴ n(A) = 4 × 5 = 20
∴ Required probability = P(A) = \(\frac{\mathrm{n}(\mathrm{A})}{\mathrm{n}(\mathrm{S})}=\frac{20}{81}\)

Question 13.
A bag contains 8 red balls and 5 white balls. Two successive draws of 3 balls each are made without replacement. Find the probability that the first drawing will give 3 white balls and the second drawing will give 3 red balls.
Solution:
Total number of balls = 8 + 5 = 13.
3 balls can be drawn out of 13 balls in ¹³C₃ ways.
∴ n(S) = ¹³C₃
Let A be the event that all 3 balls drawn are white.
3 white balls can be drawn out of 5 white balls in ⁵C₃ ways.
∴ n(A) = ⁵C₃
∴ P(A) = \(\frac{n(A)}{n(S)}=\frac{{ }^{5} C_{3}}{{ }^{13} C_{3}}=\frac{5 \times 4 \times 3}{13 \times 12 \times 11}=\frac{5}{143}\)
After drawing 3 white balls which are not replaced in the bag, there are 10 balls left in the bag out of which 8 are red balls.
Let B be the event that the second draw of 3 balls are red.
∴ Probability of drawing 3 red balls, given that 3 white balls have been already drawn, is given by
P(B/A) = \(\frac{{ }^{8} \mathrm{C}_{3}}{{ }^{10} \mathrm{C}_{3}}=\frac{8 \times 7 \times 6}{10 \times 9 \times 8}=\frac{7}{15}\)
∴ Required probability = P(A ∩ B)
= P(A) . P(B/A)
= \(\frac{5}{143} \times \frac{7}{15}\)
= \(\frac{7}{429}\)

Question 14.
The odds against student X solving a business statistics problem are 8 : 6 and the odds in favour of student Y solving the same problem are 14 : 16
(i) What is the chance that the problem will be solved, if they try independently?
(ii) What is the probability that neither solves the problem?
Solution:
(i) Let A be the event that X solves the problem B be the event that Y solves the problem.
Since the odds against student X solving the problem are 8 : 6
∴ Probability of occurrence of event A is given by
P(A) = \(\frac{6}{8+6}=\frac{6}{14}\)
and P(A’) = 1 – P(A)
= 1 – \(\frac{6}{14}\)
= \(\frac{8}{14}\)
Also, the odds in favour of student Y solving the problem are 14 : 16
∴ Probability of occurrence of event B is given by
P(B) = \(\frac{14}{14+16}=\frac{14}{30}\) and
P(B’) = 1 – P(B)
= 1 – \(\frac{14}{30}\)
= \(\frac{16}{30}\)
Now A and B are independent events.
∴ A’ and B’ are independent events.
∴ A’ ∩ B’ = Event that neither solves the problem
= P(A’ ∩ B’)
= P(A’) . P(B’)
= \(\frac{8}{14} \times \frac{16}{30}\)
= \(\frac{32}{105}\)
A ∪ B = the event that the problem is solved
∴ P(problem will be solved) = P(A ∪ B)
= 1 – P(A ∪ B)’
= 1 – P(A’ ∩ B’)
= 1 – \(\frac{32}{105}\)
= 1 – \(\frac{73}{105}\)

(ii) P (neither solves the problem) = P(A’ ∩ B’)
= P(A’) P(B’)
= \(\frac{8}{14} \times \frac{16}{30}\)
= \(\frac{32}{105}\)

11th Commerce Maths Digest Pdf

• 11th Commerce Maths Exercise 7.1 Solutions
• 11th Commerce Maths Exercise 7.2 Solutions
• 11th Commerce Maths Exercise 7.3 Solutions
• 11th Commerce Maths Exercise7.4 Solutions
• 11th Commerce Maths Miscellaneous Exercise 7 Solutions

Permutations and Combinations Class 11 Commerce Maths 2 Chapter 6 Exercise 6.2 Answers Maharashtra Board

Balbharati Maharashtra State Board 11th Commerce Maths Solution Book Pdf Chapter 6 Permutations and Combinations Ex 6.2 Questions and Answers.

Std 11 Maths 2 Exercise 6.2 Solutions Commerce Maths

Question 1.
Evaluate:
(i) 8!
Solution:
8!
= 8 × 7 × 6 × 5 × 4 × 3 × 2 × 1
= 40320

(ii) 6!
Solution:
6!
= 6 × 5 × 4 × 3 × 2 × 1
= 720

(iii) 8! – 6!
Solution:
8! – 6!
= 8 × 7 × 6! – 6!
= 6! (8 × 7 – 1)
= 6! (56 – 1)
= 6 × 5 × 4 × 3 × 2 × 1 × 55
= 39,600

(iv) (8 – 6)!
Solution:
(8 – 6)!
= 2!
= 2 × 1
= 2

Question 2.
Compute:
(i) \(\frac{12 !}{6 !}\)
Solution:
\(\frac{12 !}{6 !}\)
= \(\frac{12 \times 11 \times 10 \times 9 \times 8 \times 7 \times 6 !}{6 !}\)
= 12 × 11 × 10 × 9 × 8 × 7
= 665280

(ii) \(\left(\frac{12}{6}\right) !\)
Solution:
\(\left(\frac{12}{6}\right) !\)
= 2!
= 2 × 1
= 2

(iii) (3 × 2)!
Solution:
(3 × 2)!
= 6!
= 6 × 5 × 4 × 3 × 2 × 1
= 720

(iv) 3! × 2!
Solution:
3! × 2!
= 3 × 2 × 1 × 2 × 1
= 12

Question 3.
Compute:
(i) \(\frac{9 !}{3 ! 6 !}\)
Solution:

(ii) \(\frac{6 !-4 !}{4 !}\)
Solution:

(iii) \(\frac{8 !}{6 !-4 !}\)
Solution:

(iv) \(\frac{8 !}{(6-4) !}\)
Solution:

Question 4.
Write in terms of factorials
(i) 5 × 6 × 7 × 8 × 9 × 10
Solution:
5 × 6 × 7 × 8 × 9 × 10
= 10 × 9 × 8 × 7 × 6 × 5
Multiplying and dividing by 4!, we get

(ii) 3 × 6 × 9 × 12 × 15
Solution:
3 × 6 × 9 × 12 × 15
= 3 × (3 × 2) × (3 × 3) × (3 × 4) × (3 × 5)
= (3⁵) (5 × 4 × 3 × 2 × 1)
= 3⁵ (5!)

(iii) 6 × 7 × 8 × 9
Solution:
6 × 7 × 8 × 9
= 9 × 8 × 7 × 6
Multiplying and dividing by 5!, we get

(iv) 5 × 10 × 15 × 20 × 25
Solution:
5 × 10 × 15 × 20 × 25
= (5 × 1) × (5 × 2) × (5 × 3) × (5 × 4) × (5 × 5)
= (5⁵) (5 × 4 × 3 × 2 × 1)
= (5⁵) (5!)

Question 5.
Evaluate: \(\frac{n !}{r !(n-r) !}\) for
(i) n = 8, r = 6
Solution:
n = 8, r = 6

(ii) n = 12, r = 12
Solution:
n = 12, r = 12

Question 6.
Find n, if
(i) \(\frac{n}{8 !}=\frac{3}{6 !}+\frac{1}{4 !}\)
Solution:

(ii) \(\frac{n}{6 !}=\frac{4}{8 !}+\frac{3}{6 !}\)
Solution:

(iii) \(\frac{1}{n !}=\frac{1}{4 !}-\frac{4}{5 !}\)
Solution:

Question 7.
Find n, if
(i) (n + 1)! = 42 × (n – 1)!
Solution:
(n + 1)! = 42(n – 1)!
∴ (n + 1) n (n – 1)! = 42(n – 1)!
∴ n² + n = 42
∴ n(n + 1) = 6 × 7
Comparing on both sides, we get
∴ n = 6

(ii) (n + 3)! = 110 × (n + 1)!
Solution:
(n + 3)! = 110 × (n + 1)!
∴ (n + 3) (n + 2) (n + 1)! = 110 (n + 1)!
∴ (n + 3) (n + 2) = (11) (10)
Comparing on both sides, we get
n + 3 = 11
∴ n = 8

Question 8.
Find n, if:
(i) \(\frac{n !}{3 !(n-3) !}: \frac{n !}{5 !(n-5) !}=5: 3\)
Solution:

∴ 12 = (n – 3)(n – 4)
∴ (n – 3)(n – 4) = 4 × 3
Comparing on both sides, we get
n – 3 = 4
∴ n = 7

(ii) \(\frac{n !}{3 !(n-5) !}: \frac{n !}{5 !(n-7) !}=10: 3\)
Solution:

∴ (n – 5) (n – 6) = 3 × 2
Comparing on both sides, we get
n – 5 = 3
∴ n = 8

Question 9.
Find n, if:
(i) \(\frac{(17-n) !}{(14-n) !}\) = 5!
Solution:
\(\frac{(17-n) !}{(14-n) !}\) = 5!
∴ \(\frac{(17-n)(16-n)(15-n)(14-n) !}{(14-n) !}\) = 5 × 4 × 3 × 2 × 1
∴ (17 – n) (16 – n) (15 – n) = 6 × 5 × 4
Comparing on both sides, we get
17 – n = 6
∴ n = 11

(ii) \(\frac{(15-n) !}{(13-n) !}\) = 12
Solution:
\(\frac{(15-n) !}{(13-n) !}\) = 12
∴ \(\frac{(15-\mathrm{n})(14-\mathrm{n})(13-\mathrm{n}) !}{(13-\mathrm{n}) !}\) = 12
∴ (15 – n) (14 – n) = 4 × 3
Comparing on both sides, we get
15 – n = 4
∴ n = 11

Question 10.
Find n if \(\frac{(2 n) !}{7 !(2 n-7) !}: \frac{n !}{4 !(n-4) !}\) = 24 : 1
Solution:

∴ (2n – 1) (2n – 3) (2n – 5) = 9 × 7 × 5
Comparing on both sides, we get
2n – 1 = 9
∴ n = 5

Question 11.
Show that \(\frac{n !}{r !(n-r) !}+\frac{n !}{(r-1) !(n-r+1) !}=\frac{(n+1) !}{r !(n-r+1)}\)
Solution:

Question 12.
Show that \(\frac{9 !}{3 ! 6 !}+\frac{9 !}{4 ! 5 !}=\frac{10 !}{4 ! 6 !}\)
Solution:

Question 13.
Find the value of:
(i) \(\frac{8 !+5(4 !)}{4 !-12}\)
Solution:

(ii) \(\frac{5(26 !)+(27 !)}{4(27 !)-8(26 !)}\)
Solution:

Question 14.
Show that
\(\frac{(2 n) !}{n !}\) = 2ⁿ (2n – 1) (2n – 3)…5.3.1
Solution:

11th Commerce Maths Digest Pdf

• 11th Commerce Maths Exercise 6.1 Solutions
• 11th Commerce Maths Exercise 6.2 Solutions
• 11th Commerce Maths Exercise 6.3 Solutions
• 11th Commerce Maths Exercise 6.4 Solutions
• 11th Commerce Maths Exercise 6.5 Solutions
• 11th Commerce Maths Exercise 6.6 Solutions
• 11th Commerce Maths Exercise 6.7 Solutions
• 11th Commerce Maths  Miscellaneous Exercise 6 Solutions

Bivariate Frequency Distribution and Chi Square Statistic Class 11 Commerce Maths 2 Chapter 4 Miscellaneous Exercise 4 Answers Maharashtra Board

Balbharati Maharashtra State Board 11th Commerce Maths Solution Book Pdf Chapter 4 Bivariate Frequency Distribution and Chi Square Statistic Miscellaneous Exercise 4 Questions and Answers.

Std 11 Maths 2 Miscellaneous Exercise 4 Solutions Commerce Maths

Question 1.
Following data gives the coded price (X) and demand (Y) of a commodity.

Classify the data by taking classes 0 – 4, 5 – 9, etc. for X and 5 – 8, 9 – 12, etc. for Y.
Also find
(i) marginal frequency distribution of X and Y.
(ii) conditional frequency distribution of Y when X is less than 10.
Solution:
Given, X = coded price
Y = demand
Bivariate frequency table can be prepared by taking class intervals 0 – 4, 5 – 9,… etc for X and 5 – 8, 9 – 12,… etc for Y.
Bivariate frequency distribution is as follows.

(i) Marginal frequency distribution of X:

Marginal frequency distribution of Y:

(ii) Conditional frequency distribution of Y when X < 10:

Question 2.
Following data gives the age in years and marks obtained by 30 students in an intelligence test.


Prepare a bivariate frequency distribution by taking class intervals 16 – 18, 18 – 20,…,etc. for age and 10 – 20, 20 – 30,…, etc. for marks.
Find
(i) marginal frequency distributions.
(ii) conditional frequency distribution of marks obtained when age of students is between 20 – 22.
Solution:
Let X = Age in years
Y = Marks
Bivariate frequency table can be prepared by taking class intervals 16 – 18, 18 – 20,…, etc for X and 10 – 20, 20 – 30,…, etc for Y.
Bivariate frequency distribution is as follows:

(i) Marginal frequency distribution of X:

Marginal frequency distribution of Y:

(ii) Conditional frequency distribution of Y when X is between 20 – 22:

Question 3.
Following data gives Sales (in Lakh ?) and Advertisement Expenditure (in Thousand ₹) of 20 firms.
(115, 61) (120, 60) (128, 61) (121, 63) (137, 62) (139, 62) (143, 63) (117, 65) (126, 64) (141, 65) (140, 65) (153, 64) (129, 67) (130, 66) (150, 67) (148, 66) (130, 69) (138, 68) (155, 69) (172, 68)
(i) Construct a bivariate frequency distribution table for the above data by taking classes 115 – 125, 125 – 135, ….etc. for sales and 60 – 62, 62 – 64, …etc. for advertisement expenditure.
(ii) Find marginal frequency distributions
(iii) Conditional frequency distribution of Sales when the advertisement expenditure is between 64 – 66 (Thousand ₹)
(iv) Conditional frequency distribution of advertisement expenditure when the sales are between 125 – 135 (lakh ₹)
Solution:
(i) Let X = Sales (in lakh ₹)
Y = Advertisement Expenditure (in Thousand ₹)
Bivariate frequency table can be prepared by taking class intervals 115 – 125, 125 – 135, …. etc for X and 60 – 62, 62 – 64, ….etc for Y.
Bivariate frequency distribution is as follows:

(ii) Marginal frequency distribution of X:

Marginal frequency distribution of Y:

(ii) Conditional frequency distribution of X when Y is between 64 – 66:

(iii) Conditional frequency distribution of Y when X is between 125 – 135:

Question 4.
Prepare a bivariate frequency distribution for the following data, taking class intervals for X as 35 – 45, 45 – 55, …. etc and for Y as 115 – 130, 130 – 145, … etc where, X denotes the age in years and Y denotes blood pressure for a group of 24 persons.
(55, 151) (36, 140) (72, 160) (38, 124) (65, 148) (46, 130) (58, 152) (50, 149) (38, 115) (42, 145) (41, 163) (47, 161) (69, 159) (60, 161) (58, 131) (57, 136) (43, 141) (52, 164) (59, 161) (44, 128) (35, 118) (62, 142) (67, 157) (70, 162)
Also find
(i) Marginal frequency distribution of X.
(ii) Conditional frequency distribution of Y when X < 45.
Solution:
Given X = Age in years
Y = Blood pressure
Bivariate frequency table can be prepared by taking class intervals 35 – 45, 45 – 55, …, etc for X and 115 – 130, 130 – 145, ….., etc for Y.
Bivariate frequency distribution is as follows:

(i) Marginal frequency distribution of X:

(ii) Conditional frequency distribution of Y when X < 45:

Question 5.
Thirty pairs of values of two variables X and Y are given below. Form a bivariate frequency table. Also find marginal frequency distributions of X and Y.

Solution:
Bivariate frequency table can be prepared by taking class intervals 80 – 90, 90 – 100, etc for X and 500 – 600, 600 – 700, …., etc for Y.
Bivariate frequency distribution is as follows:

Marginal frequency distribution of X:

Marginal frequency distribution of Y

Question 6.
The following table shows how the samples of Mathematics and Economics scores of 25 students are distributed:

Find the value of ϰ² statistic.
Solution:
Table of observed frequencies.

Expected frequencies are given by
E_ij = \(\frac{R_{i} \times C_{j}}{N}\)
E₁₁ = \(\frac{35 \times 25}{50}\) = 17.5
E₁₂ = \(\frac{35 \times 25}{50}\) = 17.5
E₂₁ = \(\frac{15 \times 25}{50}\) = 7.5
E₂₂ = \(\frac{15 \times 25}{50}\) = 7.5
Table of expected frequencies.

Question 7.
Compute ϰ² statistic from the following data:

Solution:
Table of observed frequencies.

Expected frequencies are given by
E_ij = \(\frac{\mathrm{R}_{\mathrm{i}} \times \mathrm{C}_{\mathrm{j}}}{\mathrm{N}}\)
E₁₁ = \(\frac{50 \times 60}{100}\) = 30
E₁₂ = \(\frac{50 \times 40}{100}\) = 20
E₂₁ = \(\frac{50 \times 60}{100}\) = 30
E₂₂ = \(\frac{50 \times 40}{100}\) = 20
Table of expected frequencies.

Question 8.
The attitude of 250 employees towards a proposed policy of the company is as observed in the following table. Calculate ϰ² statistic.

Solution:
Table of observed frequencies

Expected frequencies are given by
E_ij = \(\frac{\mathrm{R}_{\mathrm{i}} \times \mathrm{C}_{\mathrm{j}}}{\mathrm{N}}\)
E₁₁ = \(\frac{150 \times 95}{250}\) = 57
E₁₂ = \(\frac{150 \times 95}{250}\) = 57
E₁₃ = \(\frac{150 \times 60}{250}\) = 36
E₂₁ = \(\frac{100 \times 95}{250}\) = 38
E₂₂ = \(\frac{100 \times 95}{250}\) = 38
E₂₃ = \(\frac{100 \times 60}{250}\) = 24
Table of observed frequencies.

Question 9.
In a certain sample of 1000 families, 450 families are consumers of tea. Out of 600 Hindu families, 286 families consume tea. Calculate ϰ² statistic.
Solution:
The given data can be arranged in the following table.

Table of observed frequencies.

Expected frequencies are given by
E_ij = \(\frac{R_{i} \times C_{j}}{N}\)
E₁₁ = \(\frac{600 \times 450}{1000}\) = 270
E₁₂ = \(\frac{600 \times 550}{1000}\) = 330
E₂₁ = \(\frac{400 \times 450}{1000}\) = 180
E₂₂ = \(\frac{400 \times 550}{1000}\) = 220
Table of expected frequencies.

Question 10.
A sample of boys and girls were asked to choose their favourite sport, with the following results. Find the value of ϰ² statistic.

Solution:
Table of observed frequencies.

Expected frequencies are given by
E_ij = \(\frac{R_{i} \times C_{j}}{N}\)
E₁₁ = \(\frac{200 \times 126}{300}\) = 84
E₁₂ = \(\frac{200 \times 90}{300}\) = 60
E₁₃ = \(\frac{200 \times 69}{300}\) = 46
E₁₄ = \(\frac{200 \times 15}{300}\) = 10
E₂₁ = \(\frac{100 \times 126}{300}\) = 42
E₂₂ = \(\frac{100 \times 90}{300}\) = 30
E₂₃ = \(\frac{100 \times 69}{300}\) = 23
E₂₄ = \(\frac{100 \times 15}{300}\) = 5
Table of expected frequencies.

11th Commerce Maths Digest Pdf

• 11th Commerce Maths Exercise 4.1 Solutions
• 11th Commerce Maths Exercise 4.2 Solutions
• 11th Commerce Maths Miscellaneous Exercise 4 Solutions