Class 10

Balbharti Maharashtra State Board Class 10 Maths Solutions covers the Practice Set 2.3 Algebra 10th Class Maths Part 1 Answers Solutions Chapter 2 Quadratic Equations.

10th Standard Maths 1 Practice Set 2.3 Chapter 2 Quadratic Equations Textbook Answers Maharashtra Board

Class 10 Maths Part 1 Practice Set 2.3 Chapter 2 Quadratic Equations Questions With Answers Maharashtra Board

Question 1.
Solve the following quadratic equations by completing the square method.
1. x² + x – 20 = 0
2. x² + 2x – 5 = 0
3. m² – 5m = -3
4. 9y² – 12y + 2 = 0
5. 2y² + 9y + 10 = 0
6. 5x² = 4x + 7
Solution:
1. x² + x – 20 = 0
If x² + x + k = (x + a)², then
x² + x + k = x² + 2ax + a²
Comparing the coefficients, we get
1 = 2a and k = a²

∴ The roots of the given quadratic equation are 4 and -5.

2. x² + 2x – 5 = 0
If x² + 2x + k = (x + a)², then
x² + 2x + k = x² + 2ax + a²
Comparing the coefficients, we get
2 = 2a and k = a²
∴ a = 1 and k = (1)² = 1
Now, x² + 2x – 5 = 0
∴ x² + 2x + 1 – 1 – 5 = 0
∴ (x + 1)² – 6 = 0
∴ (x + 1)² = 6
Taking square root of both sides, we get
x + 1 = ± √6
∴ x + 1 √6 or x + 1 = √6
∴ x = √6 – 1 or x = -√6 – 1
∴ The roots of the given quadratic equation are √6 -1 and – √6 -1.

3. m² – 5m = -3
∴ m² – 5m + 3 = 0
If m² – 5m + k = (m + a)², then
m² – 5m + k = m² + 2am + a²
Comparing the coefficients, we get
-5 = 2a and k = a²

4. 9y² – 12y + 2 = 0

5. 2y² + 9y + 10 = 0

Taking square root of both sides, we get

∴ The roots of the given quadratic equation are -2 and \(\frac { -5 }{ 2 } \).

6. 5x² = 4x + 7
∴ 5x² – 4x – 7 = 0

Comparing the coefficients, we get

Class 10 Maths Digest

• Practice Set 2.1 Class 10 Answers
• Practice Set 2.2 Class 10 Answers
• Practice Set 2.3 Class 10 Answers
• Practice Set 2.4 Class 10 Answers
• Practice Set 2.5 Class 10 Answers
• Practice Set 2.6 Class 10 Answers
• Problem Set 2 Class 10 Answers

Balbharti Maharashtra State Board Class 10 Maths Solutions covers the Practice Set 2.6 Algebra 10th Class Maths Part 1 Answers Solutions Chapter 2 Quadratic Equations.

10th Standard Maths 1 Practice Set 2.6 Chapter 2 Quadratic Equations Textbook Answers Maharashtra Board

Class 10 Maths Part 1 Practice Set 2.6 Chapter 2 Quadratic Equations Questions With Answers Maharashtra Board

Question 1.
Product of Pragati’s age 2 years ago and years hence is 84. Find her present age.
Solution:
Let the present age of Pragati be x years.
∴ 2 years ago,
Age of Pragati = (x – 2) years
After 3 years,
Age of Pragati = (x + 3) years
According to the given condition,
(x – 2) (x + 3) = 84
∴ x(x + 3) – 2(x + 3) = 84
∴ x² + 3x – 2x – 6 = 84
∴ x² + x – 6 – 84 = 0
∴ x² + x – 90 = 0
x² + 10x – 9x – 90 = 0
∴ x(x + 10) – 9(x + 10) = 0
∴ (x + 10)(x – 9) = 0
By using the property, if the product of two numbers is zero, then at least one of them is zero, we get
∴ x + 10 = 0 or x – 9 = 0
∴ x = -10 or x = 9
But, age cannot be negative.
∴ x = 9
∴ Present age of Pragati is 9 years.

Question 2.
The sum of squares of two consecutive even natural numbers is 244; find the numbers.
Solution:
Let the first even natural number be x.
∴ the next consecutive even natural number will be (x + 2).
According to the given condition,
x² + (x + 2)2 = 244
∴ x² + x² + 4x + 4 = 244
∴ 2x² + 4x + 4 – 244 = 0
∴ 2x² + 4x – 240 = 0
∴ x² + 2x – 120 = 0 …[Dividing both sides by 2]
∴ x² + 12x – 10x – 120 = 0
∴ x(x + 12) – 10 (x + 12) = 0
∴ (x + 12) (x – 10) = 0
By using the property, if the product of two numbers is zero, then at least one of them is zero, we get
∴ x + 12 = 0 or x – 10 = 0
∴ x = -12 or x = 10
But, natural number cannot be negative.
∴ x = 10 and x + 2 = 10 + 2 = 12
∴ The two consecutive even natural numbers are 10 and 12.

Question 3.
In the orange garden of Mr. Madhusudan there are 150 orange trees. The number of trees in each row is 5 more than that in each column. Find the number of trees in each row and each column with the help of following flow chart.

Solution:
i. Number of trees in a column is x.
ii. Number of trees in a row = x + 5
iii. Total number of trees = x x (x + 5)
iv. According to the given condition,
x(x + 5) = 150
∴ x² + 5x = 150
∴ x² + 5x – 150 = 0
v. x² + 15x – 10x – 150 = 0
∴ x(x+ 15) – 10(x + 15) = 0
∴ (x + 15)(x – 10) = 0
By using the property, if the product of two numbers is zero, then at least one of them is zero, we get
∴ x + 15 = 0 or x – 10 = 0
∴ x = -15 or x = 10
But, number of trees cannot be negative.
∴ x = 10
vi. Number of trees in a column is 10.
vii. Number of trees in a row = x + 5 = 10 + 5 = 15
∴ Number of trees in a row is 15.

Question 4.
Vivek is older than Kishor by 5 years. The Find their present ages is \(\frac { 1 }{ 6 } \) Find their Present ages
Solution:
Let the present age of Kishor be x.
∴ Present age of Vivek = (x + 5) years
According to the given condition,

∴ 6(2x + 5) = x(x + 5)
∴ 12x + 30 = x2 + 5x
∴ x² + 5x – 12x – 30 = 0
∴ x² – 7x – 30 = 0
∴ x² – 10x + 3x – 30 = 0
∴ x(x – 10) + 3(x – 10) = 0
∴ (x – 10)(x + 3) = 0
By using the property, if the product of two numbers is zero, then at least one of them is zero, we get
∴ x – 10 = 0 or x + 3 = 0
∴ x = 10 or x = – 3
But, age cannot be negative.
∴ x = 10 andx + 5 = 10 + 5 = 15
∴ Present ages of Kishor and Vivek are 10 years and 15 years respectively.

Question 5.
Suyash scored 10 marks more in second test than that in the first. 5 times the score of the second test is the same as square of the score in the first test. Find his score in the first test.
Solution:
Let the score of Suyash in the first test be x.
∴ Score in the second test = x + 10 According to the given condition,
5(x + 10) = x²
∴ 5x + 50 = x²
∴ x² – 5x – 50 = 0
∴ x² – 10x + 5x – 50 = 0
∴ x(x – 10) + 5(x – 10) = 0
∴ (x – 10) (x + 5) = 0
By using the property, if the product of two numbers is zero, then at least one of them is zero, we get
∴ x – 10 = 0 or x + 5 = 0
∴ x = 10 or x = – 5
But, score cannot be negative.
∴ x = 10
∴ The score of Suyash in the first test is 10.

Question 6.
‘Mr. Kasam runs a small business of making earthen pots. He makes certain number of pots on daily basis. Production cost of each pot is ₹ 40 more than 10 times total number of pots, he makes in one day. If production cost of all pots per day is ₹ 600, find production cost of one pot and number of pots he makes per day.
Solution:
Let Mr. Kasam make x number of pots on daily basis.
Production cost of each pot = ₹ (10x + 40)
According to the given condition,
x(10x + 40) = 600
∴ 10x² + 40x = 600
∴ 10x² + 40x- 600 = 0
∴ x² + 4x – 60 = 0 …[Dividing both sides by 10]
∴ x² + 10x – 6x – 60 = 0
∴ x(x + 10) – 6(x + 10) = 0
∴ (x + 10) (x – 6) = 0
By using the property, if the product of two numbers is zero, then at least one of them is zero, we get
∴ x + 10 = 0 or x – 6 = 0
∴ x = – 10 or x = 6
But, number of pots cannot be negative.
∴ x = 6
∴ Production cost of each pot = 7(10 x + 40)
= ₹ [(10×6)+ 40]
= ₹(60 + 40) = ₹ 100
Production cost of one pot is ₹ 100 and the number of pots Mr. Kasam makes per day is 6.

Question 7.
Pratik takes 8 hours to travel 36 km downstream and return to the same spot. The speed of boat in still water is 12 km. per hour. Find the speed of water current.
Solution:
Let the speed of water current be x km/hr. Speed of boat is 12 km/hr. (x < 12)
In upstream, speed of the water current decreases the speed of the boat and it is the opposite in downstream.
∴ speed of the boat in upstream = (12 – x) km/hr and speed of the boat in downstream = (12 + x) km/hr.

∴ The speed of water current is 6 km/hr.

Question 8.
Pintu takes 6 days more than those of Nishu to complete certain work. If they work together they finish it in 4 days. How many days would it take to complete the work if they work alone.
Solution:
Let Nishu take x days to complete the work alone.
∴ Total work done by Nishu in 1 day = \(\frac { 1 }{ x } \)
Also, Pintu takes (x + 6) days to complete the work alone.
∴ Total work done by Pintu in 1 day = \(\frac { 1 }{ x+6 } \)
∴ Total work done by both in 1 day = (\(\frac { 1 }{ x } \) + \(\frac { 1 }{ x+6 } \))
But, both take 4 days to complete the work together.
∴ Total work done by both in 1 day = \(\frac { 1 }{ 4 } \)
According to the given condition,

∴ 4(2x + 6) = x(x + 6)
∴ 8x + 24 = x² + 6x
∴ x² + 6x – 8x – 24 = 0
∴ x² – 2x – 24 = 0
∴ x² – 6x + 4x – 24 = 0
∴ x(x – 6)+ 4(x – 6) = 0
∴ (x – 6) (x + 4) = 0
By using the property, if the product of two numbers is zero, then at least one of them is zero, we get
∴ x – 6 = 0 or x + 4 = 0
∴ x = 6 or x = -4
But, number of days cannot be negative,
∴ x = 6 and x + 6 = 6 + 6 = 12
∴ Number of days taken by Nishu and Pintu to complete the work alone is 6 days and 12 days respectively.

Question 9.
If 460 is divided by a natural number, quotient is 6 more than five times the divisor and remainder is 1. Find quotient and divisor.
Solution:
Let the natural number be x.
∴ Divisor = x, Quotient = 5x + 6
Also, Dividend = 460 and Remainder = 1
Dividend = Divisor × Quotient + Remainder

By using the property, if the product of two numbers is zero, then at least one of them is zero, we get
∴ x – 9 = 0 or 5x + 51 = 0
∴ x = 9 or x = \(\frac { -51 }{ 5 } \)
But, natural number cannot be negative,
∴ x = 9
∴ Quotient = 5x + 6 = 5(9) + 6 = 45 + 6 = 51
∴ Quotient is 51 and Divisor is 9.

Question 10.
In the given fig. []ABCD is a trapezium, AB || CD and its area is 33 cm2. From the information given in the figure find the lengths of all sides of the []ABCD. Fill in the empty boxes to get the solution.

Solution:

By using the property, if the product of two numbers is zero, then at least one of them is zero, we get

Class 10 Maths Digest

• Practice Set 2.1 Class 10 Answers
• Practice Set 2.2 Class 10 Answers
• Practice Set 2.3 Class 10 Answers
• Practice Set 2.4 Class 10 Answers
• Practice Set 2.5 Class 10 Answers
• Practice Set 2.6 Class 10 Answers
• Problem Set 2 Class 10 Answers

Balbharti Maharashtra State Board Class 10 Maths Solutions covers the Practice Set 1.3 Geometry 10th Class Maths Part 2 Answers Solutions Chapter 1 Similarity.

10th Standard Maths 2 Practice Set 1.3 Chapter 1 Similarity Textbook Answers Maharashtra Board

Class 10 Maths Part 2 Practice Set 1.3 Chapter 1 Similarity Questions With Answers Maharashtra Board

Practice Set 1.3 Question 1.
In the adjoining figure, ∠ABC = 75°, ∠EDC = 75°. State which two triangles are similar and by which test? Also write the similarity of these two triangles by a proper one to one correspondence.

Solution:
In ∆ABC and ∆EDC,
∠ABC ≅ ∠EDC [Each angle is of measure 75°]
∠ACB ≅ ∠ECD [Common angle]
∴ ∆ABC ~ ∆EDC [AA test of similarity]
One to one correspondence is
ABC ↔ EDC

Similarity Class 10 Practice Set 1.3 Question 2.
Are the triangles in the adjoining figure similar? If yes, by which test?

Solution:
In ∆PQR and ∆LMN,
\(\frac { PQ }{ LM } \) = \(\frac { 6 }{ 3 } \) = \(\frac { 2 }{ 1 } \) (i)
\(\frac { QR }{ MN } \) = \(\frac { 8 }{ 4 } \) = \(\frac { 2 }{ 1 } \) (ii)
\(\frac { PR }{ LN } \) = \(\frac { 10 }{ 5 } \) = \(\frac { 2 }{ 1 } \) (iii)
∴ \(\frac { PQ }{ LM } \) = \(\frac { QR }{ MN } \) = \(\frac { PR }{ LN } \) [From (i), (ii) and (iii)]
∴ ∆PQR – ∆LMN [SSS test of similarity]

Similarity Practice Set 1.3 Question 3.
As shown in the adjoining figure, two poles of height 8 m and 4 m are perpendicular to the ground. If the length of shadow of smaller pole due to sunlight is 6 m, then how long will be the shadow of the bigger pole at the same time?

Solution:
Here, AC and PR represents the bigger and smaller poles, and BC and QR represents their shadows respectively.
Now, ∆ACB – ∆PRQ [ ∵ Vertical poles and their shadows form similar figures]
∴ \(\frac { CB }{ RQ } \) = \(\frac { AC }{ PR } \) [Corresponding sides of similar triangles]
∴ \(\frac { x }{ 6 } \) = \(\frac { 8 }{ 4 } \)
∴ \(x=\frac{8 \times 6}{4}\)
∴ x = 12 m
∴ The shadow of the bigger pole will be 12 metres long at that time.

Practice Set 1.3 Geometry 10th Maharashtra Board Question 4.
In ∆ABC, AP ⊥ BC, BQ ⊥ AC, B – P – C, A – Q – C, then prove that ∆CPA – ∆CQB. If AP = 7, BQ = 8, BC = 12, then find AC.

Solution:
In ∆CPA and ∆CQB,
∠CPA ≅ ∠CQB [Each angle is of measure 90°]
∠ACP ≅ ∠BCQ [Common angle]
∴ ∆CPA ~ ∆CQB [AA test of similarity]
∴\(\frac { AC }{ BC } \) = \(\frac { AP }{ BQ } \) [Corresponding sides of similar triangles]
∴ \(\frac { AC }{ 12 } \) = \(\frac { 7 }{ 8 } \)
∴ AC = \(x=\frac{12 \times 7}{8}\)
∴ AC = 10.5 Units

10th Geometry Practice Set 1.3 Question 5.
Given: In trapezium PQRS, side PQ || side SR, AR = 5 AP, AS = 5 AQ, then prove that SR = 5 PQ.

Solution:
side PQ || side SR [Given]
and seg SQ is their transversal.
∴ ∠QSR = ∠SQP [Altemate angles]
∴ ∠ASR = ∠AQP (i) [Q – A – S]
In ∆ASR and ∆AQP,
∠ASR = ∠AQP [From (i)]
∠SAR ≅ ∠QAP [Vertically opposite angles]
∆ASR ~ ∆AQP [AA test of similarity]
∴ \(\frac { AS }{ AQ } \) = \(\frac { SR }{ PQ } \) (ii) [Corresponding sides of similar triangles]
But, AS = 5 AQ [Given]
∴ \(\frac { AS }{ AQ } \) = \(\frac { 5 }{ 1 } \) (iii)
∴ \(\frac { SR }{ PQ } \) = \(\frac { 5 }{ 1 } \) [From (ii) and (iii)]
∴ SR = 5 PQ

Practice Set 1.3 Geometry 10th Question 6.
Id trapezium ABCD (adjoining figure), side AB || side DC, diagonals AC and BD intersect in point O. If AB = 20, DC = 6, OB = 15, then find OD.

Solution:
side AB || side DC [Given]
and seg BD is their transversal.
∴ ∠DBA ≅ ∠BDC [Alternate angles]
∴ ∠OBA ≅ ∠ODC (i) [D – O – B]
In ∆OBA and ∆ODC
∠OBA ≅ ∠ODC [From (i)]
∠BOA ≅ ∠DOC [Vertically opposite angles]
∴ ∆OBA ~ ∆ODC [AA test of similarity]
∴ \(\frac { OB }{ OD } \) = \(\frac { AB }{ DC } \) [Corresponding sides of similar triangles]
∴ \(\frac { 15 }{ OD } \) = \(\frac { 20 }{ 6 } \)
∴ OD = \(x=\frac{15 \times 6}{20}\)
∴ OD = 4.5 units

Class 10 Geometry Practice Set 1.3 Question 7.
꠸ ABCD is a parallelogram. Point E is on side BC. Line DE intersects ray AB in point T. Prove that DE × BE = CE × TE.

Solution:
Proof:
꠸ ABCD is a parallelogram. [Given]
∴ side AB || side CD [Opposite sides of a parallelogram]
∴ side AT || side CD [A – B – T]
and seg DT is their transversal.
∴ ∠ATD ≅ ∠CDT [Alternate angles]
∴ ∠BTE ≅ ∠CDE (i) [A – B – T, T – E – D]
In ∆BTE and ∆CDE,
∠BTE ≅ ∠CDE [From (i)]
∠BET ≅ ∠CED [Vertically opposite angles]
∴ ∆BTE ~ ∆CDE. [AA test of similarity]
∴ \(\frac { TE }{ DE } \) = \(\frac { BE }{ CE } \) [Corresponding sides of similar triangles]
∴ DE × BE = CE × TE

Geometry Practice Set 1.3 Question 8.
In the adjoining figure, seg AC and seg BD intersect each other in point P and \(\frac { AP }{ CP } \) = \(\frac { BP }{ DP } \) Prove that, ∆ABP ~ ∆CDP

Solution:
Proof:
In ∆ABP and ∆CDP,
\(\frac { AP }{ CP } \) = \(\frac { BP }{ DP } \) [Given]
∠APB ≅ ∠CPD [Vertically opposite angles]
∴ ∆ABP ~ ∆CDP [SAS test of similarity]

Math 2 Practice Set 1.3 Question 9.
In the adjoining figure, in ∆ABC, point D is on side BC such that, ∠BAC = ∠ADC. Prove that, CA² = CB × CD,

Solution:
Proof:
In ∆BAC and ∆ADC,
∠BAC ≅ ∠ADC [Given]
∠BCA ≅ ∠ACD [Common angle]
∴ ∆BAC ~ ∆ADC [AA test of similarity]
∴ \(\frac { CA }{ CD } \) = \(\frac { CB }{ CA } \) [Corresponding sides of similar triangles]
∴ CA × CA = CB × CD
∴ CA² = CB × CD

Question 1.
In the adjoining figure, BP ⊥ AC, CQ ⊥ AB, A – P – C, A – Q – B, then prove that ∆APB and ∆AQC are similar. (Textbook pg. no. 20)

Solution:

2. SAS test for similarity of triangles:
For a given correspondence, if two pairs of corresponding sides are in the same proportion and the angle between them is congruent, then the two triangles are similar.

In the given figure, if \(\frac { AB }{ PQ } \) = \(\frac { BC }{ QR } \), and ∠B ≅∠Q, then ∆ABC ~ ∆PQR

3. SSS test for similarity of triangles:
For a given correspondence, if three sides of one triangle are in proportion with the corresponding three sides of the another triangle, then the two triangles are similar.

In the given figure, if \(\frac { AB }{ PQ } \) = \(\frac { BC }{ QR } \) = \(\frac { AC }{ PR } \), then ∆ABC ~ ∆PQR

Properties of similar triangles:

1. Reflexivity: ∆ABC ~ ∆ABC
2. Symmetry : If ∆ABC ~ ∆DEF, then ∆DEF ~ ∆ABC.
3. Transitivity: If ∆ABC ~ ∆DEF and ∆DEF ~ ∆GHI, then ∆ABC ~ ∆GHI.

Class 10 Maths Digest

• Practice Set 1.1 Class 10 Answers
• Practice Set 1.2 Class 10 Answers
• Practice Set 1.3 Class 10 Answers
• Practice Set 1.4 Class 10 Answers
• Problem Set 1 Class 10 Answers

Balbharti Maharashtra State Board Class 10 Maths Solutions covers the Practice Set 3.2 Algebra 10th Class Maths Part 1 Answers Solutions Chapter 3 Arithmetic Progression.

10th Standard Maths 1 Practice Set 3.2 Chapter 3 Arithmetic Progression Textbook Answers Maharashtra Board

Class 10 Maths Part 1 Practice Set 3.2 Chapter 3 Arithmetic Progression Questions With Answers Maharashtra Board

Question 1.
Write the correct number in the given boxes from the following A.P.

Solution:

Question 2.
Decide whether following sequence is an A.P., if so find the 20^th term of the progression.
-12, -5, 2, 9,16, 23,30,…
Solution:
i. The given sequence is
-12, -5,2, 9, 16, 23,30,…
Here, t₁ = -1₂, t₂ = -5, t₃ = 2, t₄ = 9
∴ t₂ – t₁ – 5 – (-12) – 5 + 12 = 7
t₃ – t₂ = 2 – (-5) = 2 + 5 = 7
∴ t₄ – t₃ – 9 – 2 = 7
∴ t₂ – t₁ = t₃ – t₂ = … = 7 = d = constant
The difference between two consecutive terms is constant.
∴ The given sequence is an A.P.

ii. t_n = a + (n – 1)d
∴ t₂₀ = -12 + (20 – 1)7 …[∵a = -12, d = 7]
= -12 + 19 × 7
= -12 + 133
∴ t₂₀ = 121
∴ 20^th term of the given A.P. is 121.

Question 3.
Given Arithmetic Progression is 12, 16, 20, 24, … Find the 24^th term of this progression.
Solution:
The given A.P. is 12, 16, 20, 24,…
Here, a = 12, d = 16 – 12 = 4 Since,
t_n = a + (n – 1)d
∴ t₂₄ = 12 + (24 – 1)4
= 12 + 23 × 4
= 12 + 92
∴ t₂₄ = 104
∴ 24th term of the given A.P. is 104.

Question 4.
Find the 19th term of the following A.P. 7,13,19,25…..
Solution:
The given A.P. is 7, 13, 19, 25,…
Here, a = 7, d = 13 – 7 = 6
Since, t_n = a + (n – 1)d
∴ t₁₉ = 7 + (19 – 1)6
= 7 + 18 × 6
= 7 + 108
∴ t₁₉ = 115
∴ 19^th term of the given A.P. is 115.

Question 5.
Find the 27^th term of the following A.P. 9,4,-1,-6,-11,…
Solution:
The given A.P. is 9, 4, -1, -6, -11,…
Here, a = 9, d = 4- 9 = -5
Since, t_n = a + (n – 1)d
∴ t₂₇ = 9 + (27 – 1)(-5)
= 9 + 26 × (-5)
= 9 – 130
∴ t₂₇ = -121
∴ 27^th term of the given A.P. is -121.

Question 6.
Find how many three digit natural numbers are divisible by 5.
Solution:
The three digit natural numbers divisible by
5 are 100, 105, 110, …,995
The above sequence is an A.P.
∴ a = 100, d = 105 – 100 = 5
Let the number of terms in the A.P. be n.
Then, t_n = 995
Since, t_n = a + (n – 1)d
∴ 995 = 100 +(n – 1)5
∴ 995 – 100 = (n – 1)5
∴ 895 = (n – 1)5
∴ n – 1 = \(\frac { 895 }{ 5 } \)
∴ n – 1 = 179
∴ n = 179 + 1 = 180
∴ There are 180 three digit natural numbers which are divisible by 5.

Question 7.
The 11th term and the 21st term of an A.P. are 16 and 29 respectively, then find the 41st term of that A.P.
Solution:
Bor an A.P., let a be the first term and d be the common difference,
t₁₁ = 16, t₂₁ = 29 …[Given]
t_n = a + (n – 1)d
∴ t₁₁, = a + (11 – 1)d
∴ 16 = a + 10d
i.e. a + 10d = 16 …(i)
Also, t₂₁ = a + (21 – 1)d
∴ 29 = a + 20d
i.e. a + 20d = 29 …(ii)
Subtracting equation (i) from (ii), we get a

Question 8.
8. 11, 8, 5, 2, … In this A.P. which term is number-151?
Solution:
The given A.P. is 11, 8, 5, 2,…
Here, a = 11, d = 8 – 11 = -3
Let the n^th term of the given A.P. be -151.
Then, t_n = – 151
Since, t_n = a + (n – 1)d
∴ -151= 11 + (n – 1)(-3)
∴ -151 – 11 =(n – 1)(-3)
∴ -162 = (n – 1)(-3)
∴ n – 1 = \(\frac { -162 }{ -3 } \)
∴ n – 1 = 54
∴ n = 54 + 1 = 55
∴ 55th term of the given A.P. is -151.

Question 9.
In the natural numbers from 10 to 250, how many are divisible by 4?
Solution:
The natural numbers from 10 to 250 divisible
by 4 are 12, 16, 20, …,248
The above sequence is an A.P.
∴ a = 12, d = 16 – 12 = 4
Let the number of terms in the A.P. be n.
Then, t_n = 248
Since, t_n = a + (n – 1)d
∴ 248 = 12 + (n – 1)4
∴ 248 – 12 = (n – 1)4
∴ 236 = (n – 1)4
∴ n – 1 = \(\frac { 236 }{ 4 } \)
∴ n – 1 = 59
∴ n = 59 + 1 = 60
∴ There are 60 natural numbers from 10 to 250 which are divisible by 4.

Question 10.
In an A.P. 17^th term is 7 more than its 10th term. Find the common difference.
Solution:
For an A.P., let a be the first term and d be the common difference.
According to the given condition,
t₁₇ = t₁₀ + 7
∴ a + (17 – 1)d = a + (10 – 1)d + 7 …[∵ t_n = a + (n – 1)d]
∴ a + 16d = a + 9d + 7
∴ a + 16d – a – 9d = 7
∴ 7d = 7
∴ d = \(\frac { 7 }{ 7 } \) = 1
∴ The common difference is 1.

Question 1.
Kabir’s mother keeps a record of his height on each birthday. When he was one year old, his height was 70 cm, at 2 years he was 80 cm tall and 3 years he was 90 cm tall. His aunt Meera was studying in the 10th class. She said, “it seems like Kabir’s height grows in Arithmetic Progression”. Assuming this, she calculated how tall Kabir will be at the age of 15 years when he is in 10th! She was shocked to find it. You too assume that Kabir grows in A.P. and find out his height at the age of 15 years. (Textbook pg. no. 63)
Solution:
Height of Kabir when he was 1 year old = 70 cm Height of Kabir when he was 2 years old = 80 cm
Height of Kabir when he was 3 years old = 90 cm The heights of Kabir form an A.P.
Here, a = 70, d = 80 – 70 = 10
We have to find height of Kabir at the age of 15years i.e. t₁₅.
Now, tn = a + (n – 1)d
∴ t₁₅ = 70 + (15 – 1)10
= 70 + 14 × 10 = 70 + 140
∴ t₁₅ = 210
∴ The height of Kabir at the age of 15 years will be 210 cm.

Question 2.
Is 5, 8, 11, 14, …. an A.P.? If so then what will be the 100^th term? Check whether 92 is in this A.P.? Is number 61 in this A.P.? (Textbook pg. no, 62)
Solution:
i. The given sequence is
5, 8,11,14,…
Here, t₁ = 5, t₂ = 8, t₃ = 11, t₄ = 14
∴ t₂ – t₁ = 8 – 5 = 3
t₃ – t₂ = 11 – 8 = 3
t₄ – t₃ = 14 – 11 = 3
∴ t₂ – t₁ = t₃ – t₂ = t₄ – t₃ = 3 = d = constant
The difference between two consecutive terms is constant
∴ The given sequence is an A.P.

ii. t_n = a + (n – 1)d
∴ t₁₀₀ = 5 + (100 – 1)3 …[∵ a = 5, d = 3]
= 5 + 99 × 3
= 5 + 297
∴ t₁₀₀ = 302
∴ 100^th term of the given A.P. is 302.

iii. To check whether 92 is in given A.P., let t_n = 92
∴ t_n = a + (n – 1)d
∴ 92 = 5 + (n – 1)3
∴ 92 = 5 + 3n – 3
∴ 92 = 2 + 3n
∴ 90 = 3n
∴ n = \(\frac { 90 }{ 3 } \) = 30
∴ 92 is the 30th term of given A.P.

iv. To check whether 61 is in given A.P., let t_n = 61
61 = 5 + (n – 1)3
∴ 61 = 5 + 3n – 3
∴ 61 = 2 + 3n
∴ 61 – 2 = 3n
∴ 59 = 3n
∴ n = \(\frac { 59 }{ 3 } \)
But, n is natural number 59
∴ n ≠ \(\frac { 59 }{ 3 } \)
∴ 61 is not in given A.P.

Class 10 Maths Digest

• Practice Set 3.1 Class 10 Answers
• Practice Set 3.2 Class 10 Answers
• Practice Set 3.3 Class 10 Answers
• Practice Set 3.4 Class 10 Answers
• Problem Set 3 Class 10 Answers

Balbharti Maharashtra State Board Class 10 Maths Solutions covers the Practice Set 2.1 Algebra 10th Class Maths Part 1 Answers Solutions Chapter 2 Quadratic Equations.

10th Standard Maths 1 Practice Set 2.1 Chapter 2 Quadratic Equations Textbook Answers Maharashtra Board

Class 10 Maths Part 1 Practice Set 2.1 Chapter 2 Quadratic Equations Questions With Answers Maharashtra Board

Question 1.
Write any two quadratic equations.
Solution:
i. y² – 7y + 12 = 0
ii. x² – 8 = 0

Question 2.
Decide which of the following are quadratic
i. x² – 7y + 2 = 0
ii. y² = 5y – 10
iii. y² + \(\frac { 1 }{ y } \) = 2
iv. x + \(\frac { 1 }{ x } \) = -2
v. (m + 2) (m – 5) = 03
vi. m³ + 3m² – 2 = 3m³
Solution:
i. The given equation is x² + 5x – 2 = 0
Here, x is the only variable and maximum index of the variable is 2.
a = 1, b = 5, c = -2 are real numbers and a ≠ 0.
∴ The given equation is a quadratic equation.

ii. The given equation is
y² = 5y – 10
∴ y² – 5y + 10 = 0
Here, y is the only variable and maximum index of the variable is 2.
a = 1, b = -5, c = 10 are real numbers and a ≠ 0.
∴ The given equation is a quadratic equation.

iii. The given equation is
y² + \(\frac { 1 }{ y } \) = 2
∴ y³ + 1 = 2y …[Multiplying both sides by y]
∴ y³ – 2y + 1 = 0
Here, y is the only variable and maximum index of the variable is not 2.
∴ The given equation is not a quadratic equation.

iv. The given equation is
x + \(\frac { 1 }{ x } \) = -2
∴ x² + 1 = -2x …[Multiplying both sides by x]
∴ x² + 2x+ 1 = 0
Here, x is the only variable and maximum index of the variable is 2.
a = 1, b = 2, c = 1 are real numbers and a ≠ 0.
∴ The given equation is a quadratic equation.

v. The given equation is
(m + 2) (m – 5) = 0
∴ m(m – 5) + 2(m – 5) = 0
∴ m² – 5m + 2m – 10 = 0
∴ m² – 3m – 10 = 0
Here, m is the only variable and maximum index of the variable is 2.
a = 1, b = -3, c = -10 are real numbers and a ≠ 0.
∴ The given equation is a quadratic equation.

vi. The given equation is
m³ + 3m² – 2 = 3m³
∴ 3m³ – m³ – 3m² + 2 = 0
∴ 2m³ – 3m² + 2 = 0
Here, m is the only variable and maximum
index of the variable is not 2.
∴ The given equation is not a quadratic equation.

Question 3.
Write the following equations in the form ax² + bx + c = 0, then write the values of a, b, c for each equation.
i. 2y = 10 – y²
ii. (x – 1)² = 2x + 3
iii. x² + 5x = – (3 – x)
iv. 3m² = 2m² – 9
v. P (3 + 6p) = – 5
vi. x² – 9 = 13
Solution:
i. 2y – 10 – y²
∴ y² + 2y – 10 = 0
Comparing the above equation with
ay² + by + c = 0, we get
a = 1, b = 2, c = -10

ii. (x – 1)² = 2x + 3
∴ x² – 2x + 12x + 3
x² – 2x + 1 – 2x – 30
∴ x² – 4x – 2 = 0
Comparing the above equation with
ax² + bx + c = 0, we get
a = 1, b = -4, c = -2

iii. x² + 5x = – (3 – x)
∴ x² + 5x = -3 + x
∴ x² + 5x – x + 3 = 0
∴ x² + 4x + 3 = 0
Comparing the above equation with
ax² + bx + c = 0, we get
a = 1, b = 4, c = 3

iv. 3m² = 2m² – 9
∴ 3m² – 2m² + 9 = 0
∴ m² + 9 = 0
∴ m² + 0m + 9 = 0
Comparing the above equation with
am² + bm + c = 0, we get
a = 1, b = 0, c = 9

v. p (3 + 6p) = – 5
∴ 3p + 6p² = -5
∴ 6p² + 3p + 5 = 0
Comparing the above equation with
ap² + bp + c = 0, we get
a = 6, b = 3, c = 5

vi. x² – 9 = 13
∴ x² – 9 – 13 = 0
∴ x² – 22 = 0
∴ x² + 0x – 22 = 0
Comparing the above equation with
ax² + bx + c = 0, we get
a = 1, b = 0, c = -22

Question 4.
Determine whether the values given against each of the quadratic equation are the roots of the equation.
i. x² + 4x – 5 = 0; x = 1,-1
ii. 2m² – 5m = 0; m = 2, \(\frac { 5 }{ 2 } \)
Solution:
i. The given equation is
x² + 4x – 5 = 0 …(i)
Putting x = 1 in L.H.S. of equation (i), we get
L.H.S. = (1)² + 4(1) – 5 = 1 + 4 – 5 = 0
∴ L.H.S. = R.H.S.
∴ x = 1 is the root of the given quadratic equation.
Putting x = -1 in L.H.S. of equation (i), we get
L.H.S. = (-1)² + 4(-1) – 5 = 1 – 4 – 5 = -8
∴ LH.S. ≠ R.H.S.
∴ x = -1 ¡s not the root of the given quadratic equation.

ii. The given equation is
2m² – 5m = 0 …(i)
Putting m = 2 in L.H.S. of equation (i), we get
L.H.S. = 2(2)² – 5(2) = 2(4) -10 = 8 – 10 = -2
∴ L.H.S. ≠ R.H.S.
∴ m = 2 is not the root of the given quadratic equation.
Putting m = \(\frac { 5 }{ 2 } \) in L.H.S. of equation (i), we get

Question 5.
Find k if x = 3 is a root of equation kx² – 10x + 3 = 0.
Solution:
x = 3 is the root of the equation kx² – 10x + 3 = 0.
Putting x = 3 in the given equation, we get
k(3)² – 10(3) + 3 = 0
∴ 9k – 30 +3 = 0
∴ 9k – 27 = 0
∴ 9k = 27
∴ k = \(\frac { 27 }{ 9 } \)
∴ k = 3

Question 6.
One of the roots of equation 5m² + 2m + k = 0 is \(\frac { -7 }{ 5 } \) Complete the following activity to find the value of ‘k’.
Solution:

Question 1.
x² + 3x – 5, 3x² – 5x, 5x²; Write the polynomials In the index form. Observe the coefficients and fill in the boxes. (Textbook p. no. 31)
Answer:
Index form of the given polynomials:
x² + 3x – 5, 3x² – 5x + 0, 5x² + 0x + 0
i. Coefficients of x2 are [1], [3] and [5] respectively, and these coefficients are non zero.
ii. Coefficients of x are 3, [-5] and [0] respectively.
iii. Constant terms are [-5], [0] and [0] respectively.
Here, constant terms of second and third polynomial is zero.

Question 2.
Complete the following table (Textbook p. no. 31)

Answer:

Question 3.
Decide which of the following are quadratic equations? (Textbook pg. no. 31)
i. 9y² + 5 = 0
ii. m³ – 5m² + 4 = 0
iii. (l + 2)(l – 5) = 0
Solution:
i. In the equation 9y² + 5 = 0, [y] is the only variable and maximum index of the variable is [2].
∴ It [is] a quadratic equation.

ii. In the equation m³ – 5m² + 4 = 0, [m] is the only variable and maximum index of the variable is not 2.
∴ It [is not] a quadratic equation.

iii. (l + 2)(l – 5) = 0
∴ l(l – 5) + 2(l – 5) = 0
∴ l² – 5l + 2l – 10 = 0
∴ l² – 3l – 10 = 0.
In this equation [l] is the only variable and maximum index of the variable is [2]
∴ it [is] a quadratic equation.

Question 4.
If x = 5 is a root of equation kx² – 14x – 5 = 0, then find the value of k by completing the following activity. (Textbook pg, no. 33)
Solution:

Class 10 Maths Digest

• Practice Set 2.1 Class 10 Answers
• Practice Set 2.2 Class 10 Answers
• Practice Set 2.3 Class 10 Answers
• Practice Set 2.4 Class 10 Answers
• Practice Set 2.5 Class 10 Answers
• Practice Set 2.6 Class 10 Answers
• Problem Set 2 Class 10 Answers

Balbharti Maharashtra State Board Class 10 Maths Solutions covers the Practice Set 1.5 Algebra 10th Class Maths Part 1 Answers Solutions Chapter 1 Linear Equations in Two Variables.

10th Standard Maths 1 Practice Set 1.5 Chapter 1 Linear Equations in Two Variables Textbook Answers Maharashtra Board

Class 10 Maths Part 1 Practice Set 1.5 Chapter 1 Linear Equations in Two Variables Questions With Answers Maharashtra Board

Question 1.
Two numbers differ by 3. The sum of twice the smaller number and thrice the greater number is 19. Find the numbers.
Solution:
Let the greater number be x and the smaller number be y.
According to the first condition, x – y = 3 …(i)
According to the second condition,
3x + 2y = 19 …(ii)
Multiplying equation (i) by 2, we get
2x – 2y = 6 …(iii)
Adding equations (ii) and (iii), we get

Substituting x = 5 in equation (i), we get
5 – y = 3
∴ 5 – 3 = y
∴ y = 2
∴ The required numbers are 5 and 2.

Question 2.
Complete the following.

Solution:
Opposite sides of a rectangle are equal.
∴ 2x + y + 8 = 4x – y
∴ 8 = 4x – 2x – y – y
∴ 2x – 2y = 8
∴ x – y = 4 …(i)[Dividingboth sides by 2]
Also, x + 4= 2y
∴ x – 2y = -4 …(ii)
Subtracting equation (ii) from (i), we get

Substituting y = 8 in equation (i), we get
x – 8 = 4
∴ x = 4 + 8
∴ x = 12
Now, length of rectangle = 4x – y
= 4(12) – 8
= 48 – 8
∴ Length of rectangle = 40
Breadth of rectangle = 2y = 2(8) = 16
Perimeter of rectangle = 2(length + breadth)
= 2(40 + 16)
= 2(56)
∴ Perimeter of rectangle =112 units
Area of rectangle = length × breadth
= 40 × 16
∴ Area of rectangle = 640 sq. units
∴ x = 12 and y = 8, Perimeter of rectangle is 112 units and area of rectangle is 640 sq. units.

Question 3.
The sum of father’s age and twice the age of his son is 70. If we double the age of the father and add it to the age of his son the sum is 95. Find their present ages.
Solution:
Let the present ages of father and son be x years and y years respectively.
According to the first condition,
x + 2y = 70 …(i)
According to the second condition,
2x + y = 95 …(ii)
Multiplying equation (i) by 2, we get
2x + 4y = 140 …(iii)
Subtracting equation (ii) from (iii), we get

Substituting y = 15 in equation (i), we get
x + 2(15) = 7O
⇒ x + 30 = 70
⇒ x = 70 – 30
∴ x = 40
∴ The present ages of father and son are 40 years and 15 years respectively.

Question 4.
The denominator of a fraction is 4 more than twice its numerator. Denominator becomes 12 times the numerator, if both the numerator and the denominator are reduced by 6. Find the fraction.
Solution:
Let the numerator of the fraction be x and the denominator be y.
∴ Fraction = \(\frac { x }{ y } \)
According to the first condition,
y = 2x + 4
∴ 2x – y = -4 …(i)
According to the second condition,
(y – 6)= 12(x – 6)
∴ y – 6 = 12x – 72
∴ 12x – y = 72 – 6
∴ 12x – y = 66 …(ii)
Subtracting equation (i) from (ii), we get

Question 5.
Two types of boxes A, B ,are to be placed in a truck having capacity of 10 tons. When 150 boxes of type A and 100 boxes of type B are loaded in the truck, it weights 10 tons. But when 260 boxes of type A are loaded in the truck, it can still accommodate 40 boxes of type B, so that it is fully loaded. Find the weight of each type of box.
Solution:
Let the weights of box of type A be x kg and that of box of type B be y kg.
1 ton = 1000 kg
∴ 10 tons = 10000 kg
According to the first condition,
150x + 100y = 10000
∴ 3x + 2y = 200 …(i) [Dividing both sides by 50]
According to the second condition,
260x + 40y = 10000
∴ 13x + 2y = 500 …(ii) [Dividing both sides by 20]
Subtracting equation (i) from (ii), we get

∴ The weights of box of type A is 30 kg and that of box of type B is 55 kg.

Question 6.
Out of 1900 km, Vishal travelled some distance by bus and some by aeroplane. Bus travels with average speed 60 km/hr and the average speed of aeroplane is 700 km/hr. It takes 5 hours to complete the journey. Find the distance Vishal travelled by bus.
Solution:
Let the distance Vishal travelled by bus be x km and by aeroplane be y km.
According to the first condition,
x + y = 1900 …(i)
\(\text { Time }=\frac{\text { Distance }}{\text { Speed }} \)
∴ Time required to cover x km by bus = \(\frac { x }{ 60 } \) hr
Time required to cover y km by aeroplane
= \(\frac { y }{ 700 } \) hr
According to the second condition,

Multiplying equation (i) by 6, we get
6x + 6y= 11400 …(iii)
Subtracting equation (iii) from (ii), we get

∴ The distance Vishal travelled by bus is 150 km.

Question 1.
There are some instructions given below. Frame the equations from the information and write them in the blank boxes shown by arrows. (Textbook pg. no. 20)
Answer:

Class 10 Maths Digest

• Practice Set 1.1 Class 10 Answers
• Practice Set 1.2 Class 10 Answers
• Practice Set 1.3 Class 10 Answers
• Practice Set 1.4 Class 10 Answers
• Practice Set 1.5 Class 10 Answers
• Problem Set 1. Class 10 Answers

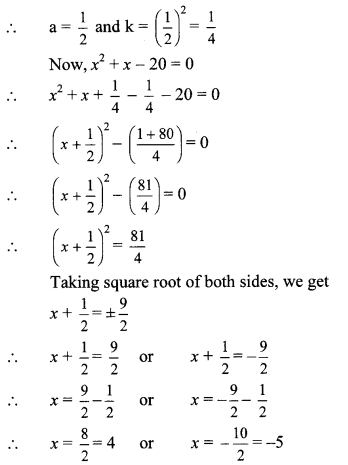
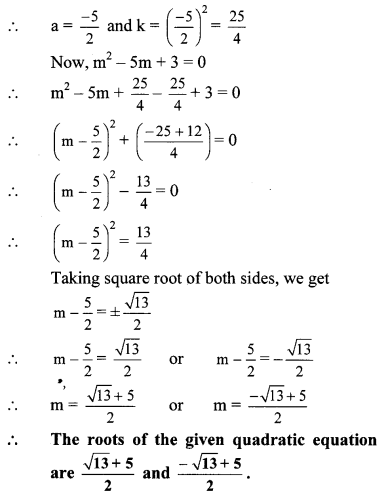
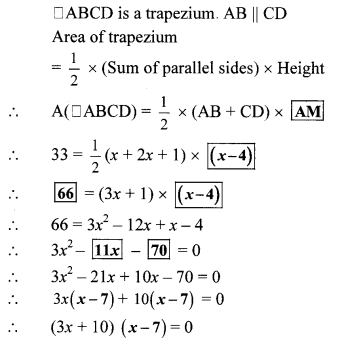
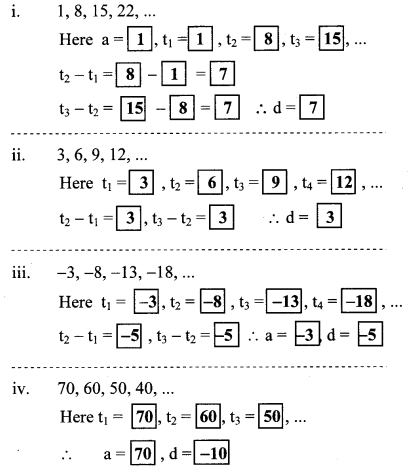
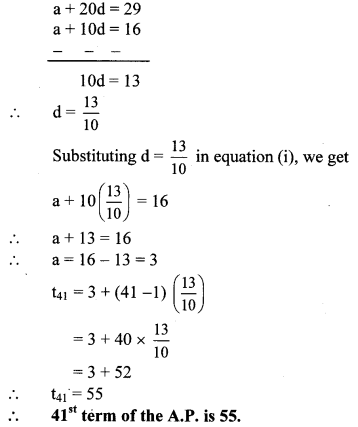
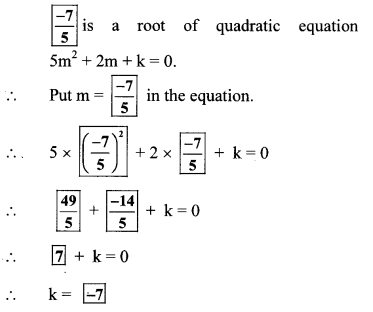
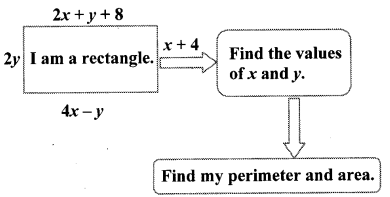
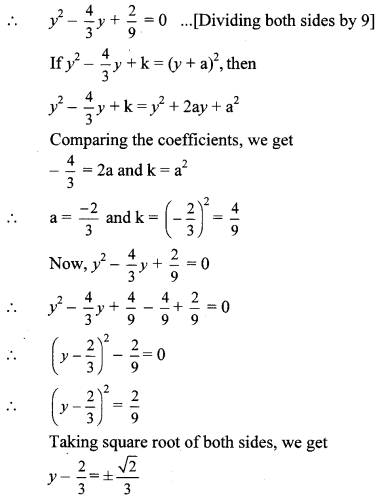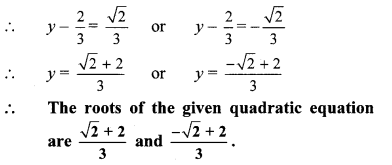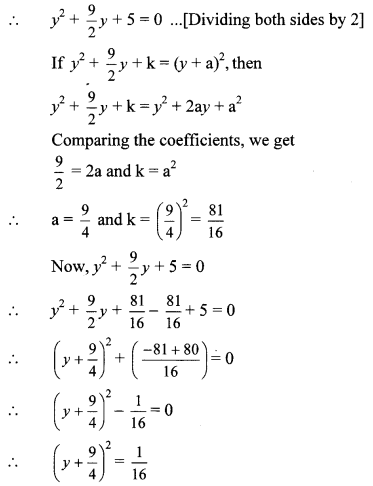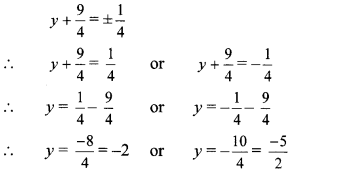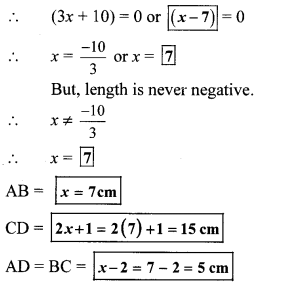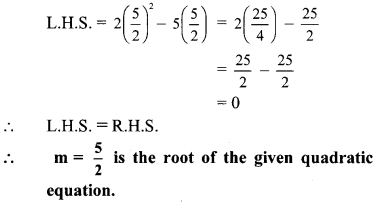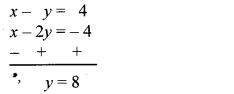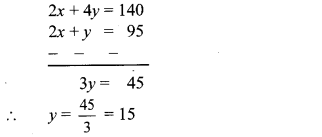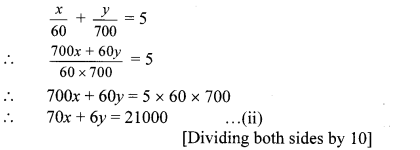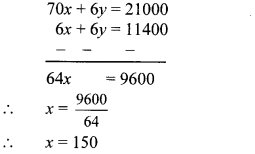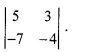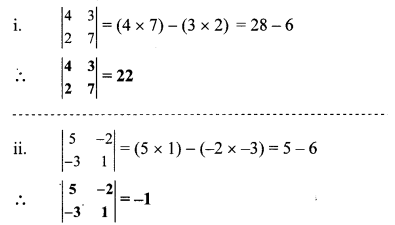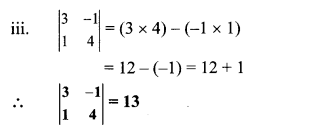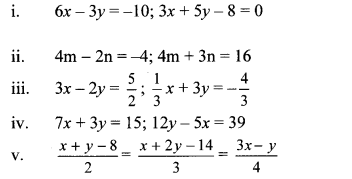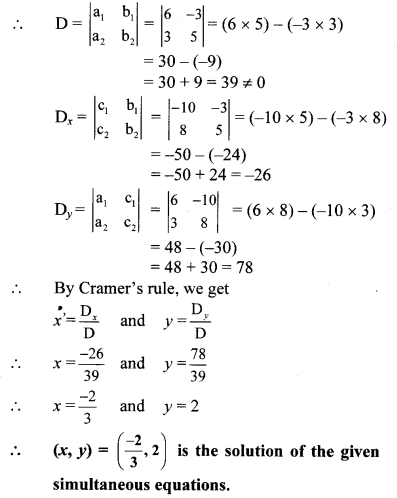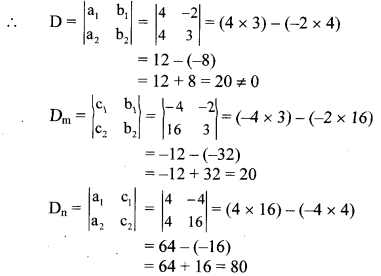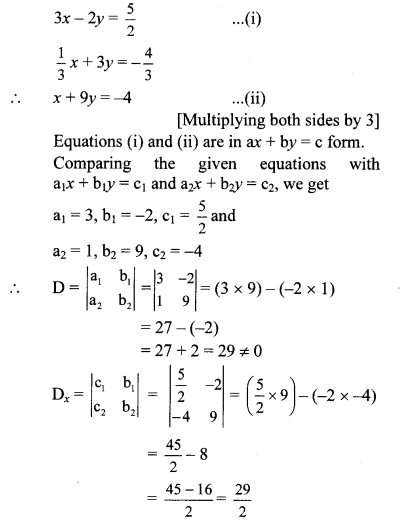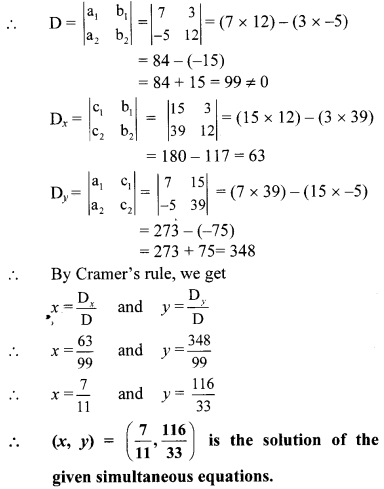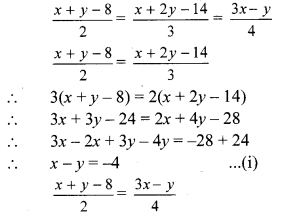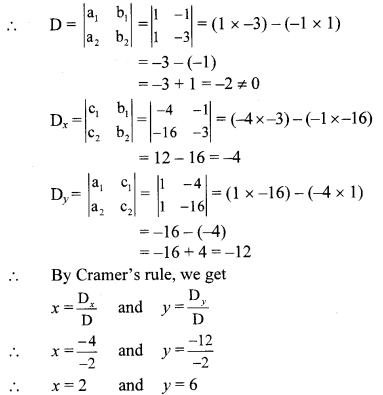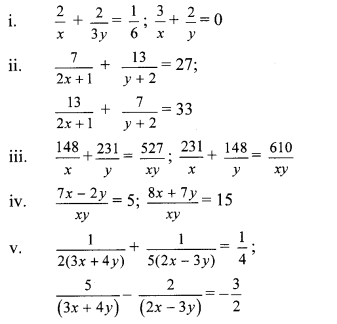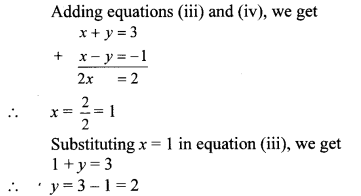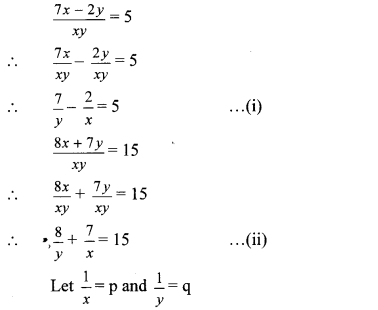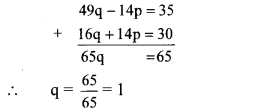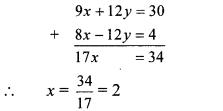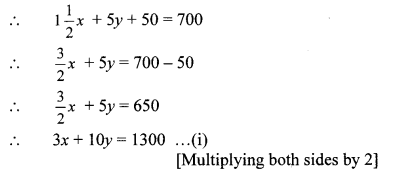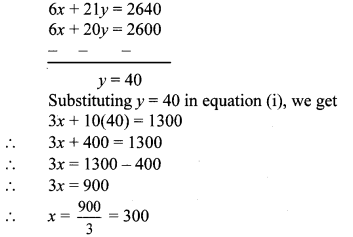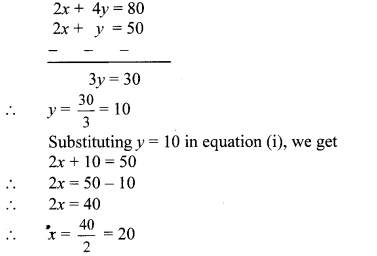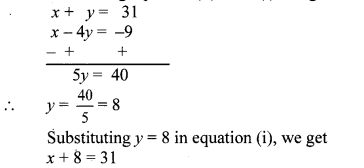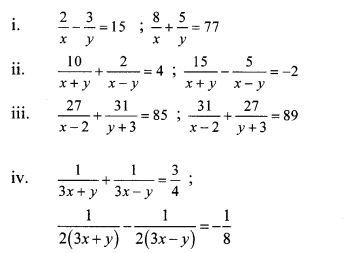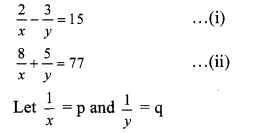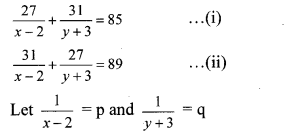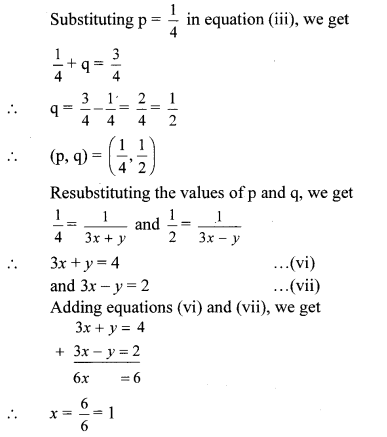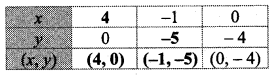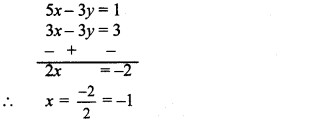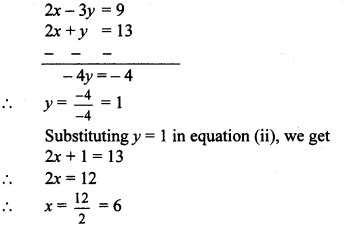Balbharti Maharashtra State Board Class 10 Maths Solutions covers the Problem Set 1 Algebra 10th Class Maths Part 1 Answers Solutions Chapter 1 Linear Equations in Two Variables.

10th Standard Maths 1 Problem Set 1 Chapter 1 Linear Equations in Two Variables Textbook Answers Maharashtra Board

Class 10 Maths Part 1 Problem Set 1 Chapter 1 Linear Equations in Two Variables Questions With Answers Maharashtra Board

Choose correct alternative for each of the following questions.

Question 1.
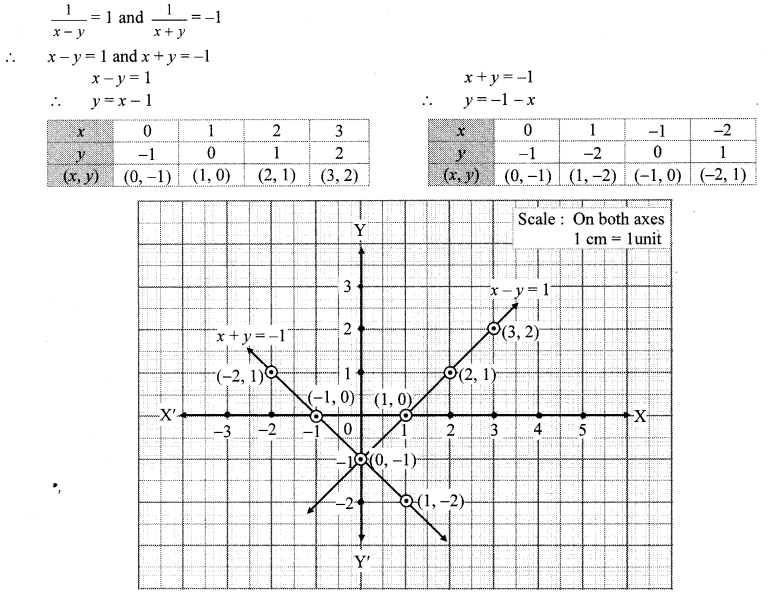
To draw graph of 4x + 5y = 19, find y when x = 1.
(a) 4
(b) 3
(c) 2
(d) -3
Answer:
(b)

Question 2.
For simultaneous equations in variables x and y, D_x = 49, Dy = – 63, D = 7 then what is x?
(a) 7
(b) -7
(c) \(\frac { 1 }{ 7 } \)
(d) \(\frac { -1 }{ 7 } \)
Answer:
(a)

Question 3.
Find the value of

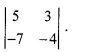
(a) -1
(b) -41
(c) 41
(d) 1
Answer:
(d)

Question 4.
To solvex + y = 3; 3x – 2y – 4 = 0 by determinant method find D.
(a) 5
(b) 1
(c) -5
(d) -1
Answer:
(c)

Question 5.
ax + by = c and mx + n y = d and an ≠ bm then these simultaneous equations have-
(a) Only one common solution
(b) No solution
(c) Infinite number of solutions
(d) Only two solutions.
Answer:
(a)

Question 2.
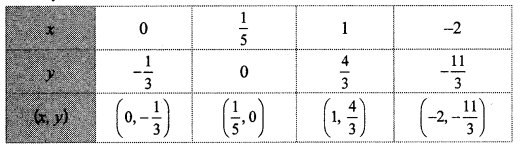
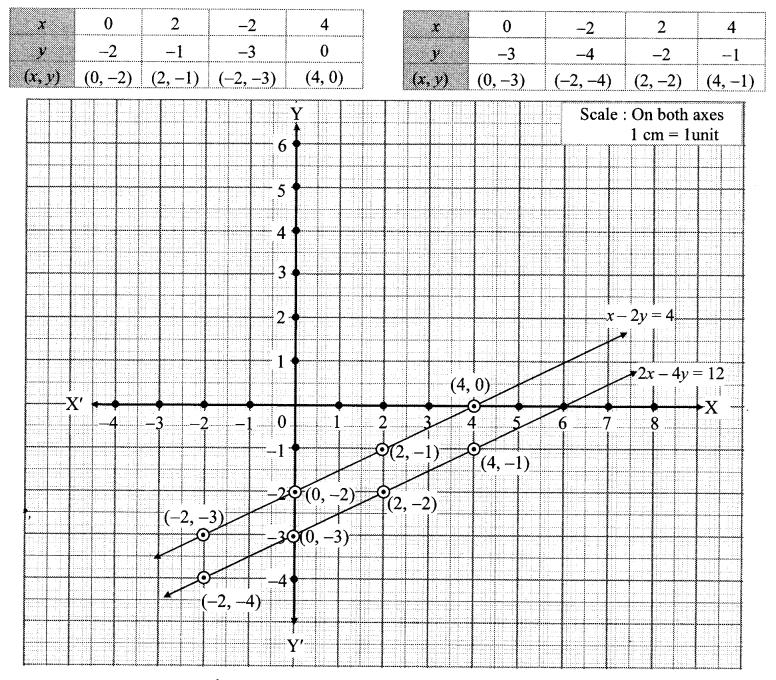
Complete the following table to draw the graph of 2x – 6y = 3.
Answer:

Question 3.
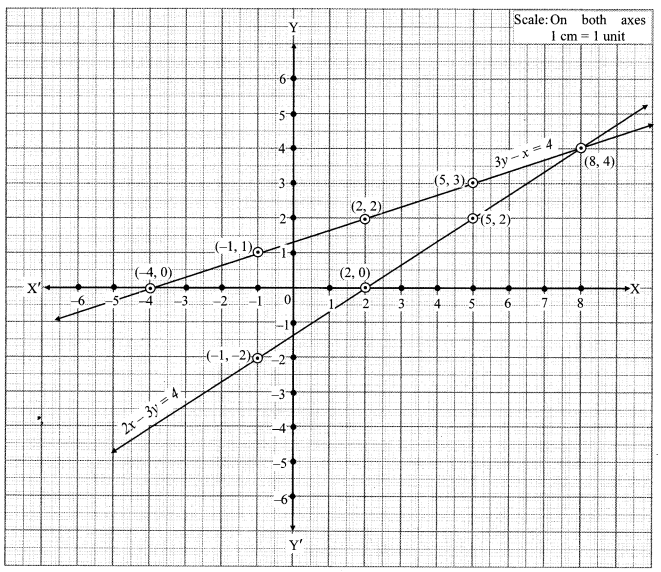
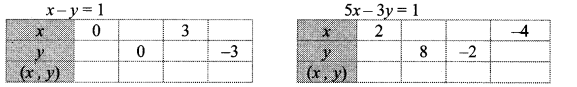
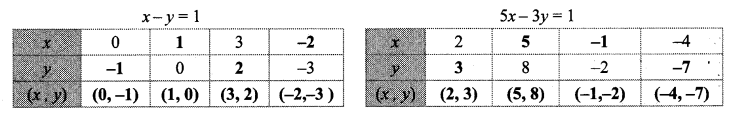
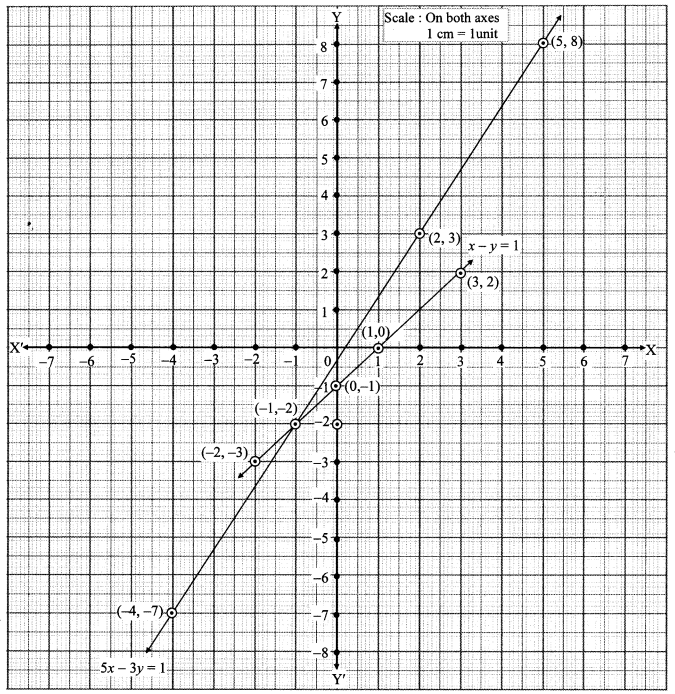
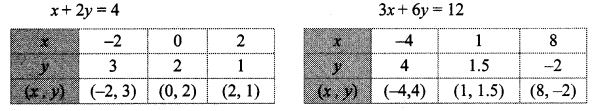
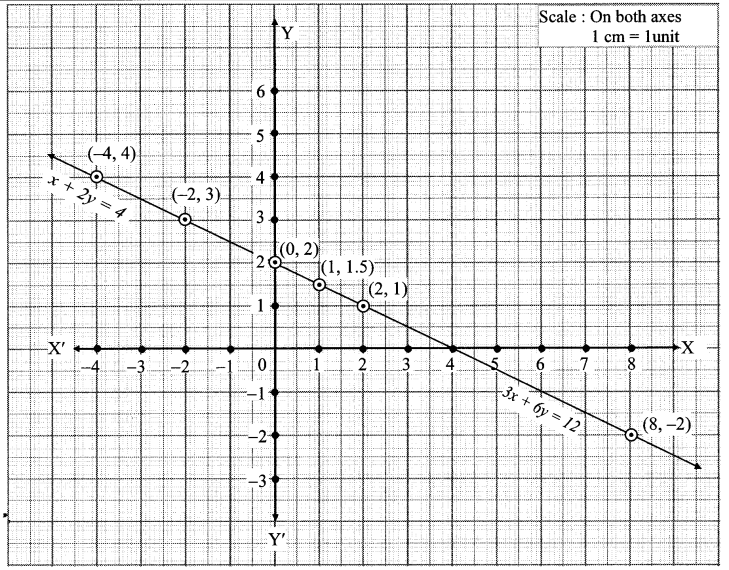
Solve the following simultaneous equations graphically.
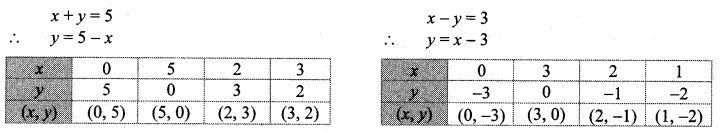
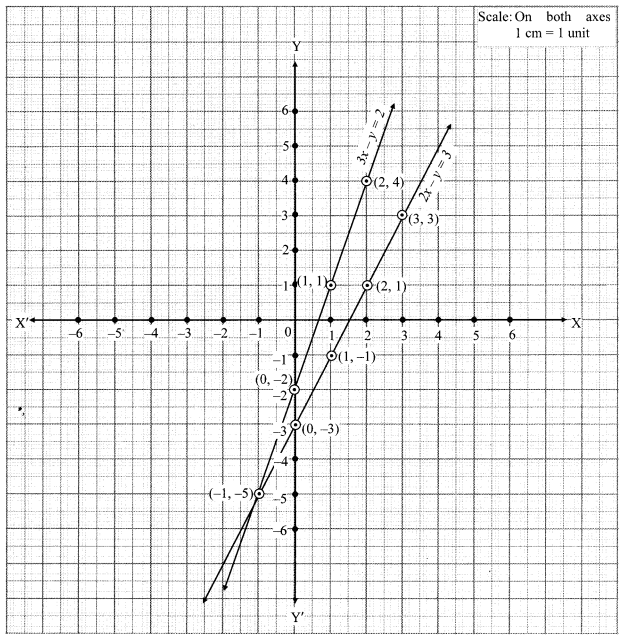
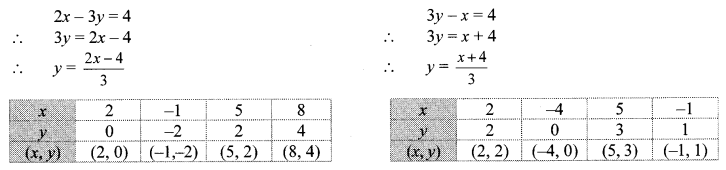
i. 2x + 3y = 12 ; x – y = 1
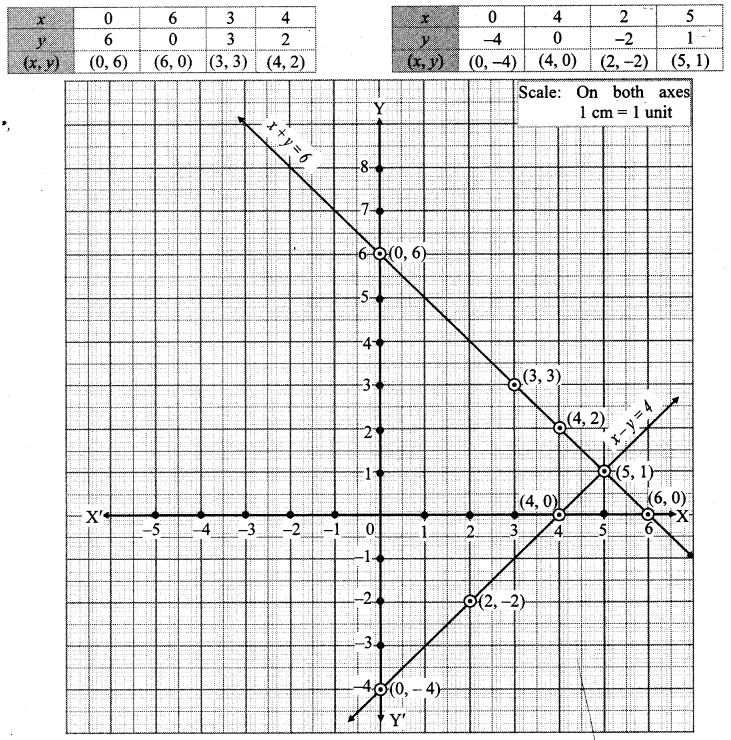
ii. x – 3y = 1 ; 3x – 2y + 4 = 0
iii. 5x – 6y + 30 = 0; 5x + 4y – 20 = 0
iv. 3x – y – 2 = 0 ; 2x + y = 8
v. 3x + y= 10 ; x – y = 2
Answer:
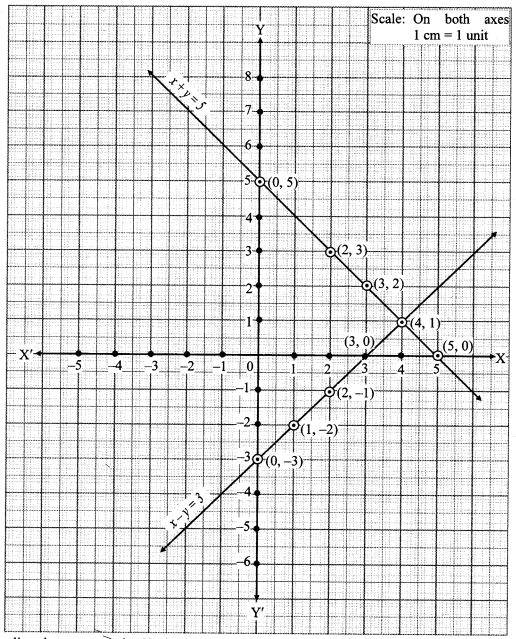
i. The given simultaneous equations are

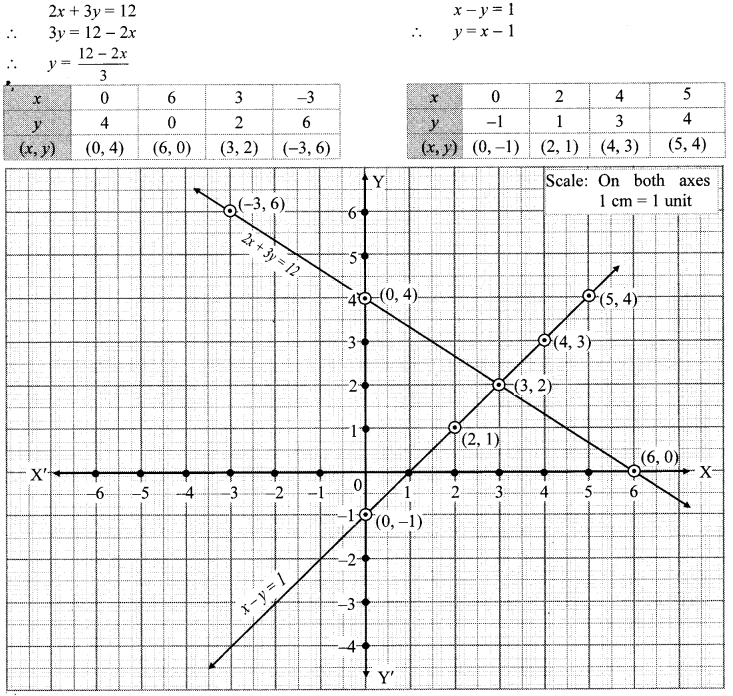
The two lines interest at point (3,2).
∴ x = 3 and y = 2 is the solution of the simultaneous equations 2x + 3y = 12 and x – y = 1.

ii. The given simultaneous equations are

The two lines intersect at point (-2, -1).
∴ x = -2 and y = -1 is the solution of the simultaneous equations x – 3y = 1 and 3x – 2p + 4 = 0.

iii. The given simultaneous equations are

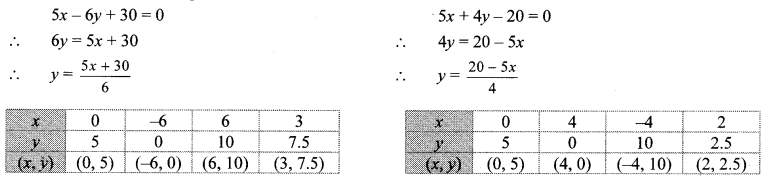
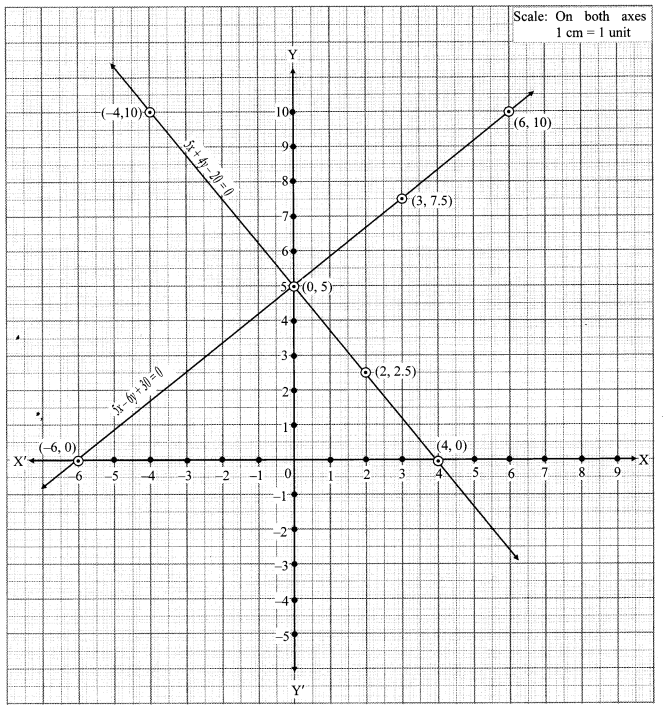
The two lines intersect at point (0, 5).
∴ x = 0 and y = 5 is the solution of the simultaneous equations 5x – 6y + 30 = 0 and 5x + 4y – 20 = 0.

iv. The given simultaneous equations are


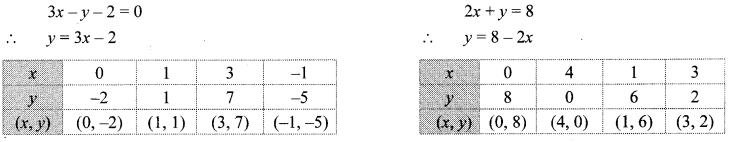
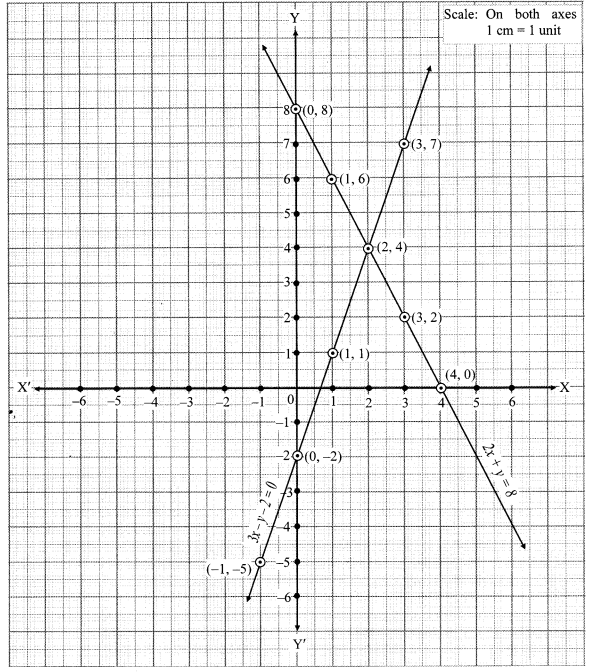
The two lines intersect at point (2, 4).
∴ x = 2 and y = 4 is the solution of the simultaneous equations 3x – y – 2 = 0 and 2x + y = 8.

v. The given simultaneous equations are

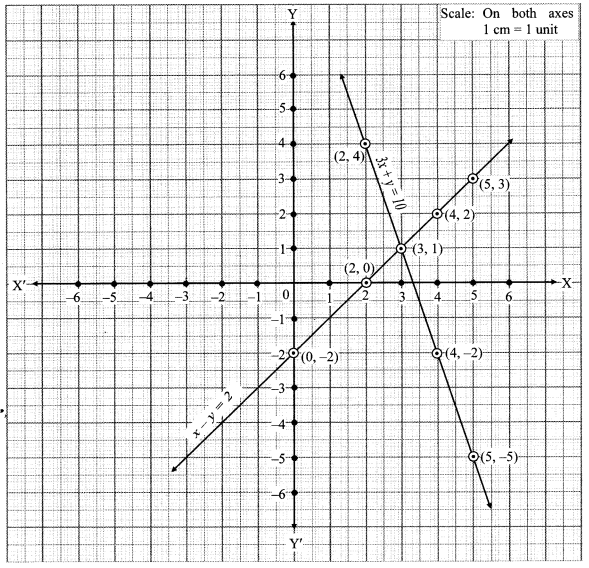
The two lines intersect at point (3, 1).
∴ x = 3 and y = 1 is the solution of the simultaneous equations 3x + y = 10 and x – y = 2.

Question 4.
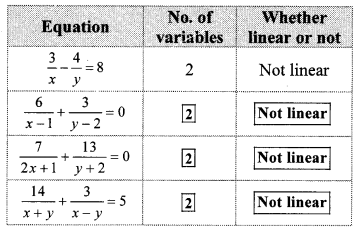
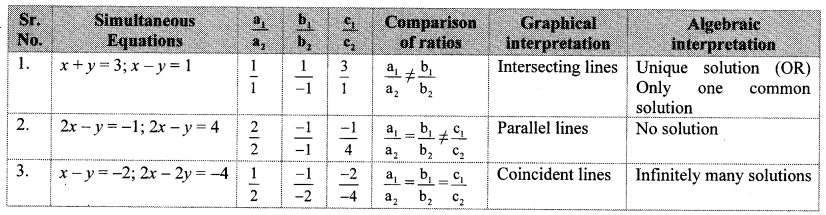
Find the values of each of the following determinants.

Solution:

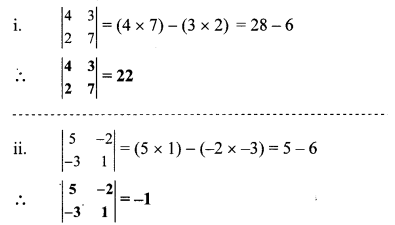
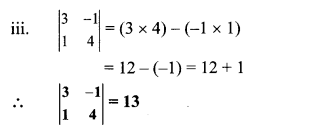
Question 5.
Solve the following equations by Cramer’s method.

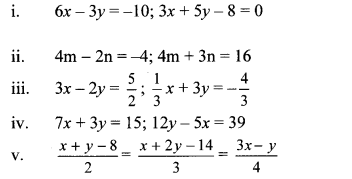
Solution:
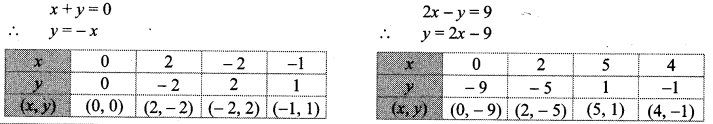
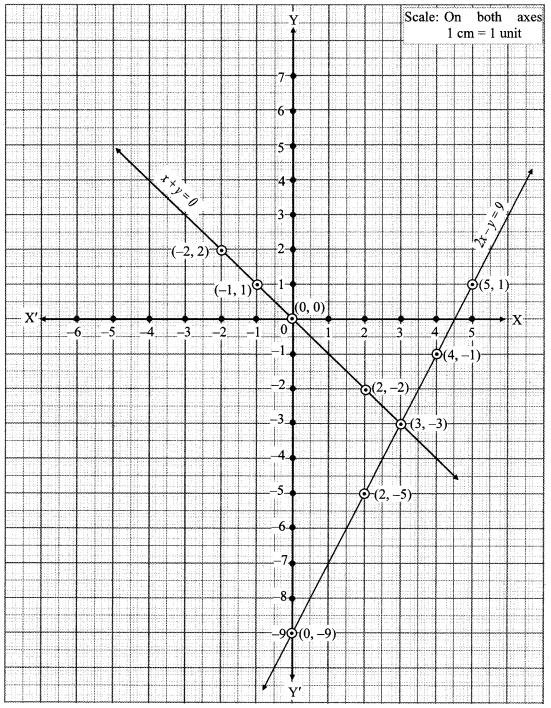
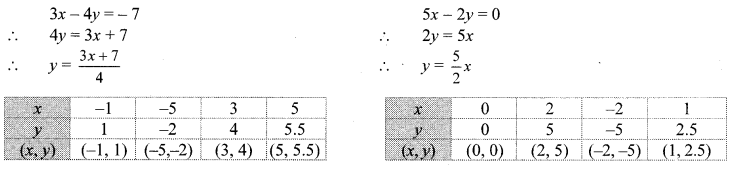
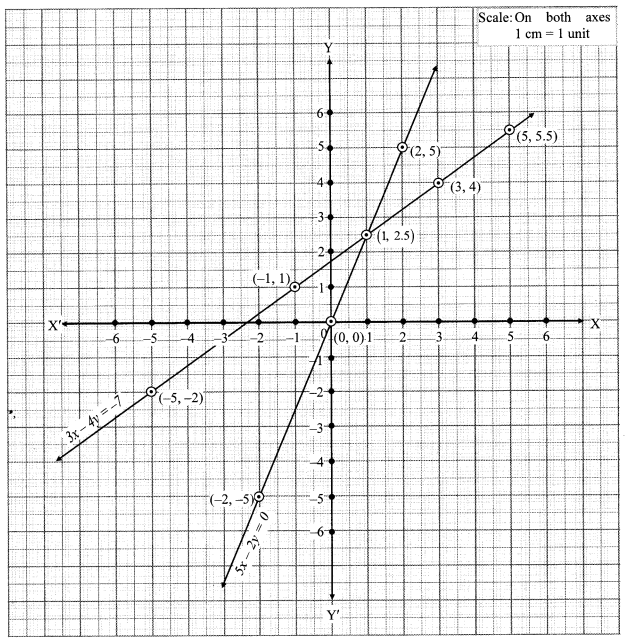
i. The given simultaneous equations are
6x – 3y = -10 …(i)
3x + 5y – 8 = 0
∴ 3x + 5y = 8 …(ii)
Equations (i) and (ii) are in ax + by = c form. Comparing the given equations with a₁x + b₁y = c₁ and a₂x + b₂y = c₂, we get
a₁ = 6, b₁ = -3, c₁ = 10 and
a₂ = 3, b₂ = 5, c₂ = 8

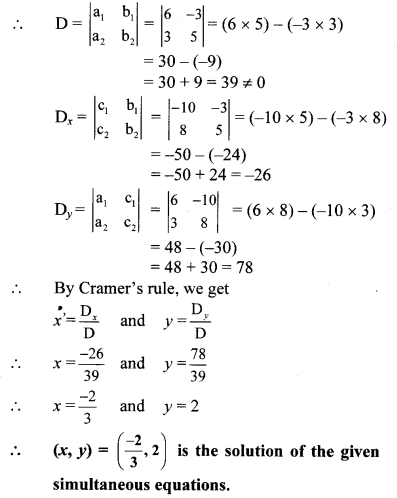
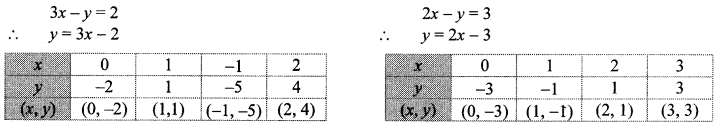
ii. The given simultaneous equations are
4m – 2n = -4 …(i)
4m + 3n = 16 …(ii)
Equations (i) and (ii) are in am + bn = c form.
Comparing the given equations with a₁m + b₁n = c₁ and a₂m + b₂n = c₂, we get
a₁ = 4, b₁ = -2, c₁ = -4 and
a₂ = 4, b₂ = 3, c₂ = 16


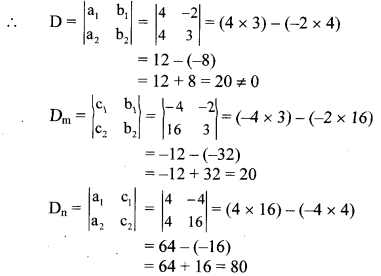
∴ (m, n) = (1, 4) is the solution of the given simultaneous equations.

iii. The given simultaneous equations are

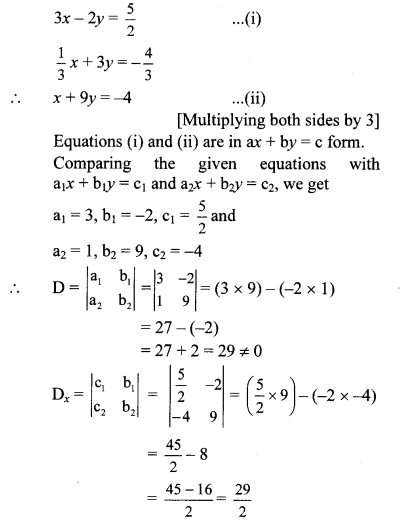
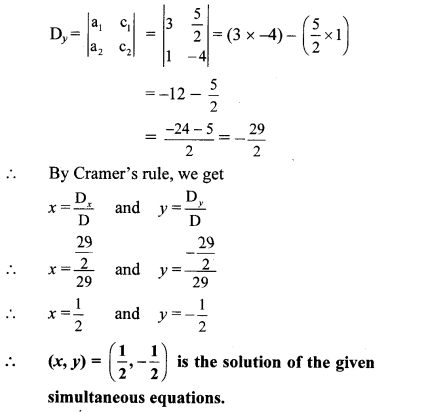
iv. The given simultaneous equations are
7x + 3y = 15 …(i)
12y – 5x = 39
i.e. -5x + 12y = 39 …(ii)
Equations (i) and (ii) are in ax + by = c form.
Comparing the given equations with
a₁x + b₁y = c₁ and a₂x + b₂y = c₂, we get
a₁ = 7, b₁ = 3, c₁ = 15 and
a₂ = -5, b₂ = 12, c₂ = 39

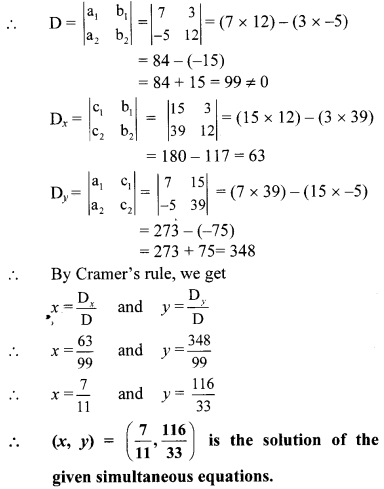
v. The given simultaneous equations are

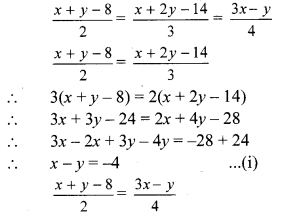
∴ 4(x + y – 8) = 2(3x – y)
∴ 4x + 4y – 32 = 6x – 2y
∴ 6x – 4x – 2y – 4y = -32
∴ 2x – 6y = -32
∴ x – 3y = -16 …(ii)[Dividing both sides by 2]
Equations (i) and (ii) are in ax + by = c form. Comparing the given equations with
a₁x + b₁y = c₁ and a₂x + b₂y = c₂, we get
a₁ = 1, b₁ = -1, c₁ = -4 and
a₂ = 1, b₂ = -3, c₂ = -16

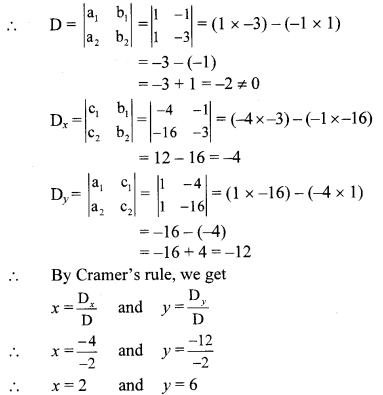
∴ (x, y) = (2, 6) is the solution of the given simultaneous equations.

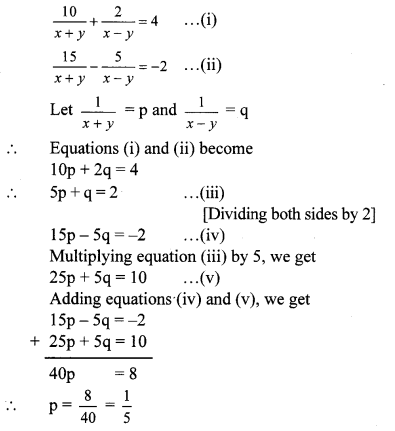
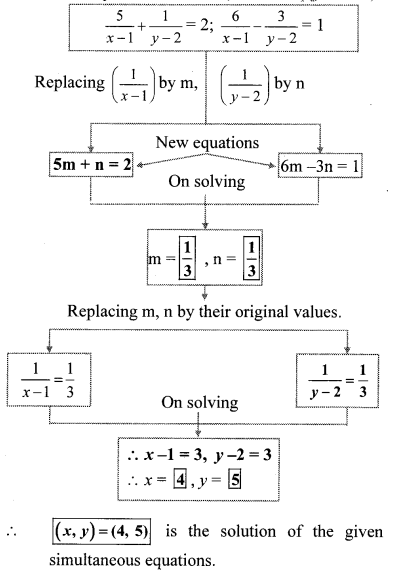
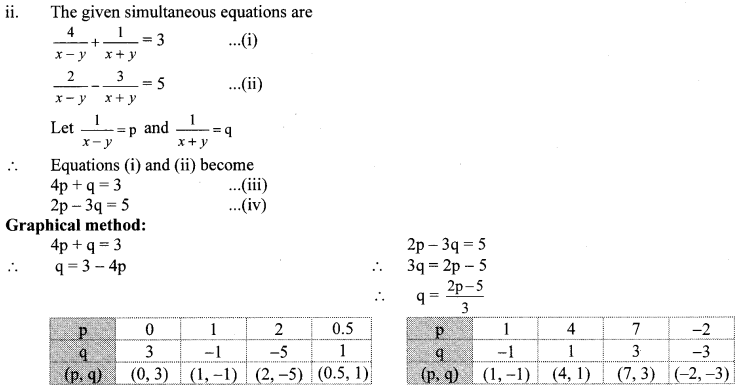
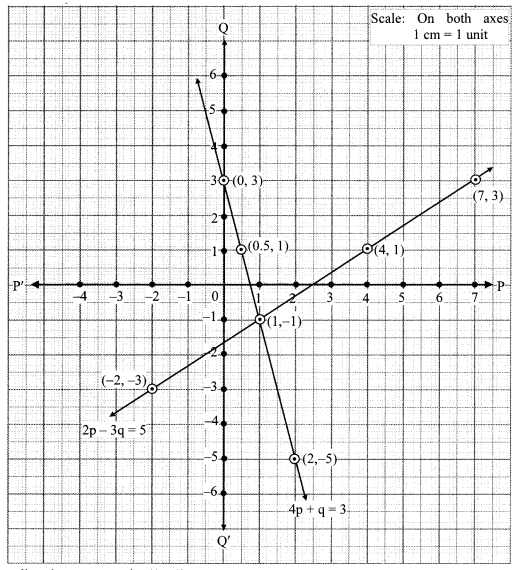
Question 6.
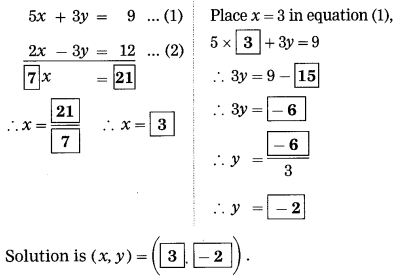
Solve the following simultaneous equations:

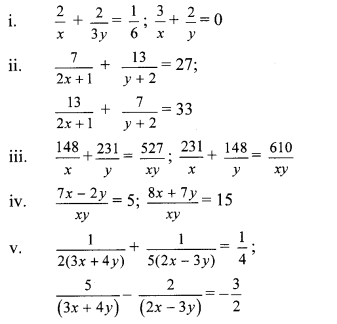
Answer:
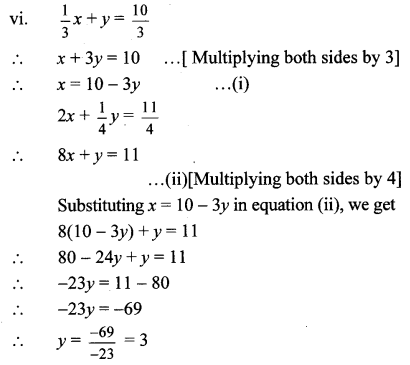
i. The given simultaneous equations are

Subtracting equation (iv) from (iii), we get

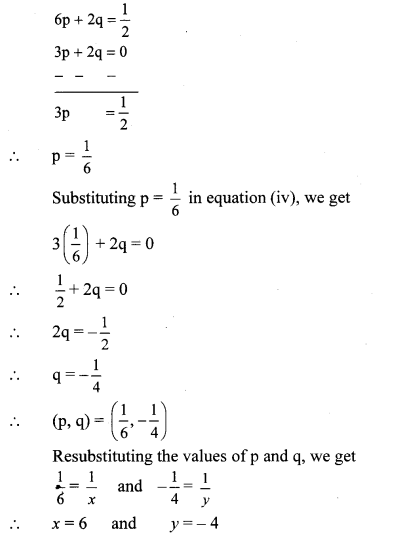
∴ (x, y) = (6, – 4) is the solution of the given simultaneous equations.

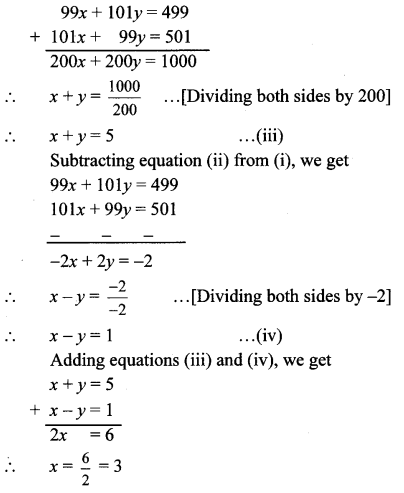
ii. The given simultaneous equations are

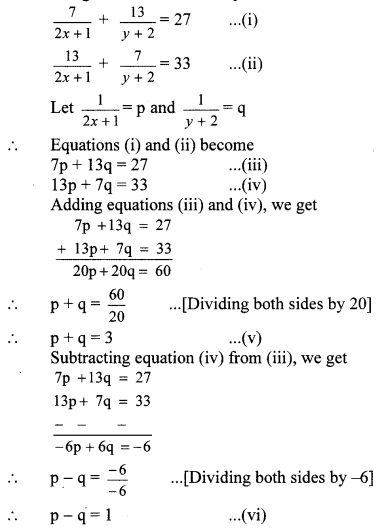
Adding equations (v) and (vi), we get

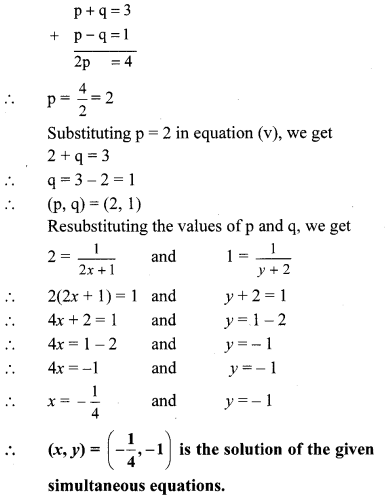
iii. The given simultaneous equations are


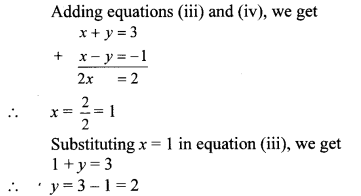
∴ (x, y) = (1, 2) is the solution of the given simultaneous equations.

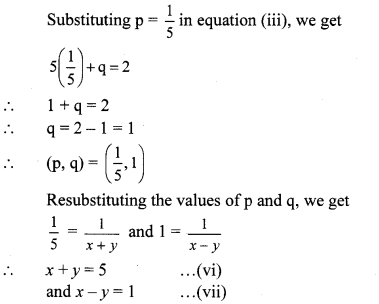
iv. The given simultaneous equations are

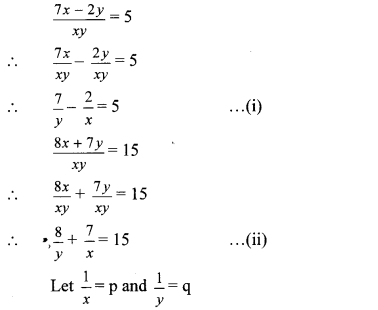
∴ Equations (i) and (ii) become 7q – 2p = 5 …(iii)
8q + 7p = 15 …(iv)
Multiplying equation (iii) by 7, we get
49q – 14p = 35 …(v)
Multiplying equation (iv) by 2, we get
16q + 14p = 30 …(vi)
Adding equations (v) and (vi), we get

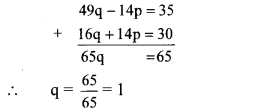
Substituting q = 1 in equation (iv), we get
8(1) + 7p = 15
∴ 8 + 7p = 15
∴ 7p = 15 – 8
∴ 7p = 7
∴ p = \(\frac { 7 }{ 7 } \) = 1
∴ (P, q) = (1,1)
Resubstituting the values of p and q, we get
1 = \(\frac { 1 }{ x } \) and 1 = \(\frac { 1 }{ y } \)
∴ x = 1 and y = 1
∴ (x, y) = (1, 1) is the solution of the given simultaneous equations.

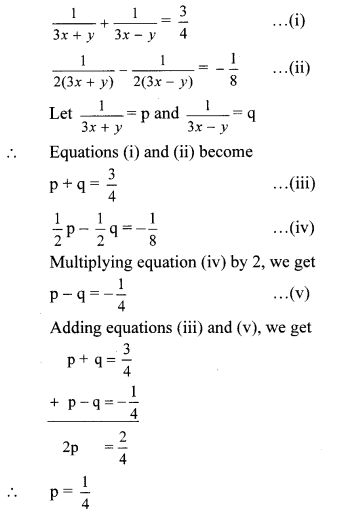
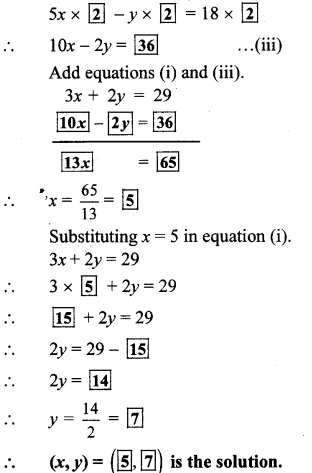
v. The given simultaneous equations are

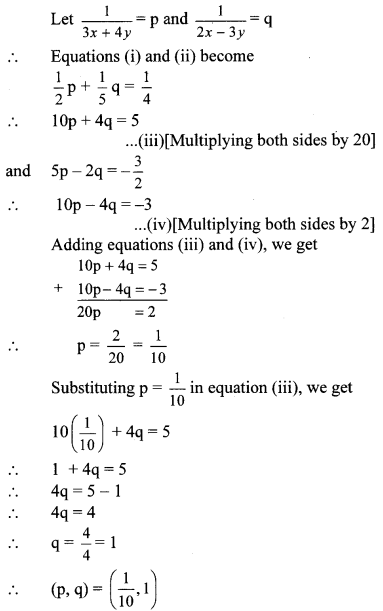
Resubstituting the values of p and q, we get

∴ 3x + 4y = 10 …(v)
and 2x – 3y = 1 …(vi)
Multiplying equation (v) by 3, we get
9x + 12y = 30 …(vii)
Multiplying equation (vi) by 4, we get
8x – 12y = 4 …(viii)
Adding equations (vii) and (viii), we get

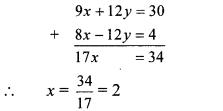
Substituting x = 2 in equation (v), we get
3(2) + 4y = 10
⇒ 6 + 4y = 10
⇒ 4y = 10 – 6
⇒ y = 4/4 = 1
∴ y = 1
∴ (x, y) = (2, 1) is the solution of the given simultaneous equations.

Question 7.
Solve the following word problems, i. A two digit number and the number with digits interchanged add up to 143. In the given number the digit in unit’s place is 3 more than the digit in the ten’s place. Find the original number.
Solution:
Let the digit in unit’s place be x
and that in the ten’s place be y.

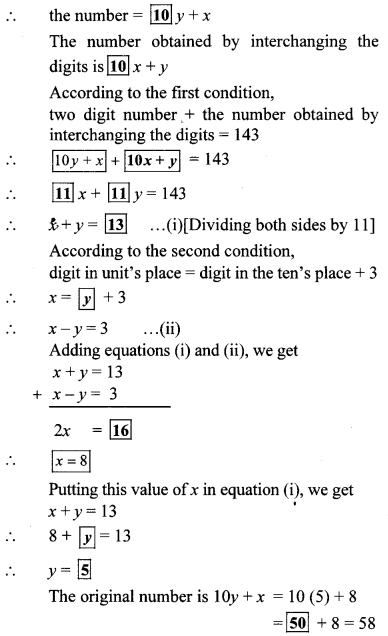
ii. Kantabai bought 1 \(\frac { 1 }{ 2 } \) kg tea and 5 kg sugar from a shop. She paid ₹ 50 as return fare for rickshaw. Total expense was ₹ 700. Then she realised that by ordering online the goods can be bought with free home delivery at the same price. So, next month she placed the order online for 2 kg tea and 7 kg sugar. She paid ₹ 880 for that. Find the rate of sugar and tea per kg.
Solution:
Let the rate of tea be ₹ x per kg and that of sugar be ₹ y per kg.
According to the first condition,
cost of 1 \(\frac { 1 }{ 2 } \) kg tea + cost of 5 kg sugar + fare for rickshaw = total expense

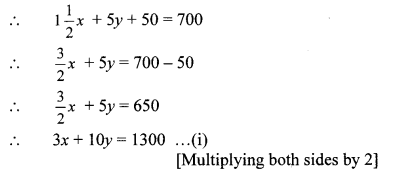
According to the second condition,
cost of 2 kg tea + cost of 7 kg sugar = total expense
2x + 7y = 880 …(ii)
Multiplying equation (i) by 2, we get
6x + 20y = 2600 …(iii)
Multiplying equation (ii) by 3, we get
6x + 21y = 2640 …(iv)
Subtracting equation (iii) from (iv), we get

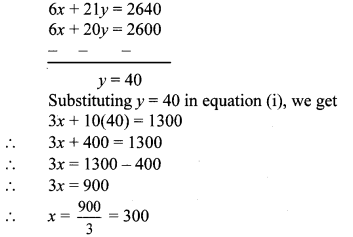
∴ The rate of tea is ₹ 300 per kg and that of sugar is ₹ 40 per kg.

iii. To find number of notes that Anushka had, complete the following activity.

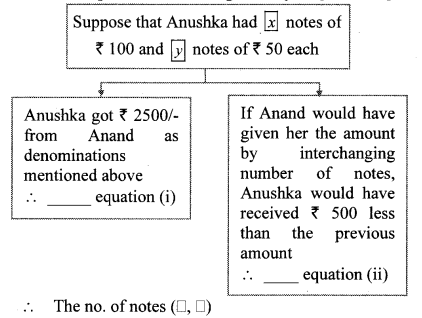
Solution:
Anushka had x notes of ₹ 100 and y notes of ₹ 50.
According to the first condition,
100x + 50y = 2500
∴ 2x + y = 50 …(i) [Dividing both sides by 50]
According to the second condition,
100y + 50x = 2000
∴ 2y + x = 40 … [Dividing both sides by 50]
i.e. x + 2y = 40
∴ 2x + 4y = 80 …(ii) [Multiplying both sides by 2]
Subtracting equation (i) from (ii), we get

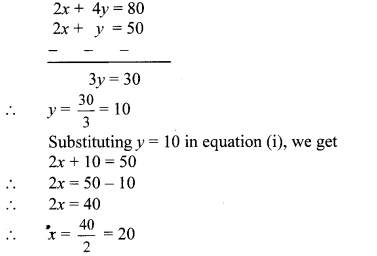
∴ Anushka had 20 notes of ₹ 100 and 10 notes of ₹ 50.

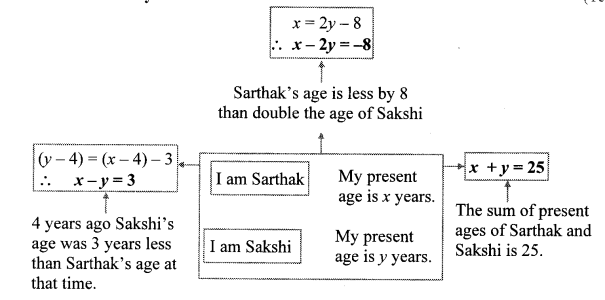
iv. Sum of the present ages of Manish and Savita is 31, Manish’s age 3 years ago was 4 times the age of Savita. Find their present ages.
Solution:
Let the present ages of Manish and Savita be x years and y years respectively.
According to the first condition,
x + y = 31 …(i)
3 years ago,
Manish’s age = (x – 3) years
Savita’s age = (y – 3) years
According to the second condition,
(x – 3) = 4 (y – 3)
∴ x – 3 = 4y – 12
∴ x – 4y = -12 + 3
∴ x – 4y = -9 …(ii)
Subtracting equation (ii) from (i), we get

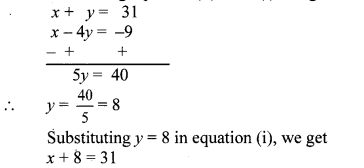
∴ x = 31 – 8
∴ x = 23
The present ages of Manish and Savita are 23 years and 8 years respectively.

v. In a factory the ratio of salary of skilled and unskilled workers is 5 : 3. Total salary of one day of both of them is ₹ 720. Find daily wages of skilled and unskilled workers.
Solution:
Let the daily wages of skilled workers be ₹ x
that of unskilled workers be ₹ y.
According to the first condition,

∴ The daily wages of skilled workers is ₹ 450 and that of unskilled workers is ₹ 270.

vi. Places A and B are 30 km apart and they are on a straight road. Hamid travels from A to B on bike. At the same time Joseph starts from B on bike, travels towards A. They meet each other after 20 minutes. If Joseph would have started from B at the same time but in the opposite direction (instead of towards A), Hamid would have caught him after 3 hours. Find the speed of Hamid and Joseph.
Solution:
Let the speeds of Hamid and Joseph be x km/hr andy km/hr respectively.
Distance travelled by Hamid in 20 minutes

According to the first condition,

∴ The speeds of Hamid and Joseph 50 km/hr and 40 km/hr respectively.

Class 10 Maths Digest

• Practice Set 1.1 Class 10 Answers
• Practice Set 1.2 Class 10 Answers
• Practice Set 1.3 Class 10 Answers
• Practice Set 1.4 Class 10 Answers
• Practice Set 1.5 Class 10 Answers
• Problem Set 1. Class 10 Answers

Balbharti Maharashtra State Board Class 10 Maths Solutions covers the Practice Set 1.4 Algebra 10th Class Maths Part 1 Answers Solutions Chapter 1 Linear Equations in Two Variables.

10th Standard Maths 1 Practice Set 1.4 Chapter 1 Linear Equations in Two Variables Textbook Answers Maharashtra Board

Class 10 Maths Part 1 Practice Set 1.4 Chapter 1 Linear Equations in Two Variables Questions With Answers Maharashtra Board

Question 1.
Solve the following simultaneous equations.

Solution:
i. The given simultaneous equations are

∴ Equations (i) and (ii) become
2p – 3q = 15 …(iii)
8p + 5q = 77 …(iv)
Multiplying equation (iii) by 4, we get
8p – 12q = 60 …(v)
Subtracting equation (v) from (iv), we get

ii. The given simultaneous equations are



Substituting x = 3 in equation (vi), we get
3 + y = 5
∴ y = 5 – 3 = 2
∴ (x, y) = (3, 2) is the solution of the given simultaneous equations.

iii. The given simultaneous equations are

∴ Equations (i) and (ii) become
27p + 31q = 85 …(iii)
31p + 27q = 89 …(iv)
Adding equations (iii) and (iv), we get

iv. The given simultaneous equations are


Substituting x = 1 in equation (vi), we get
3(1) + y = 4
∴ 3 + y = 4
∴ y = 4 – 3 = 1
∴ (x, y) = (1, 1) is the solution of the given simultaneous equations.

Question 1.
Complete the following table. (Textbook pg. no. 16)
Solution:

Question 2.
In the above table the equations are not linear. Can you convert the equations into linear equations? (Textbook pg. no. 17)
Answer:
Yes, the above given simultaneous equations can be converted to a pair of linear equations by making suitable substitutions.

Steps for solving equations reducible to a pair of linear equations.

• Step 1: Select suitable variables other than those which are in the equations.
• Step 2: Replace the given variables with new variables such that the given equations become linear equations in two variables.
• Step 3: Solve the new simultaneous equations and find the values of the new variables.
• Step 4: By resubstituting the value(s) of the new variables, find the replaced variables which are to be determined.

Question 3.
To solve given equations fill the below boxes suitably. (Text book pg.no. 19)
Answer:

Question 4.
The examples on textbook pg. no. 17 and 18 obtained by transformation are solved by elimination method. If you solve these equations by graphical method and by Cramer’s rule will you get the same answers? Solve and check it. (Textbook pg. no. 18)

Solution:

The two lines intersect at point (1,-1).
∴ p = 1 and q = -1 is the solution of the simultaneous equations 4p + q = 3 and 2p – 3q = 5.
Re substituting the values of p and q, we get

The two lines intersect at point (0, -1).
∴ x = 0 and y = -1 is the solution of the simultaneous equations x – y = 1 and x + y = -1.
∴ (x, y) = (0, -1) is the solution of the given simultaneous equations.

Class 10 Maths Digest

• Practice Set 1.1 Class 10 Answers
• Practice Set 1.2 Class 10 Answers
• Practice Set 1.3 Class 10 Answers
• Practice Set 1.4 Class 10 Answers
• Practice Set 1.5 Class 10 Answers
• Problem Set 1. Class 10 Answers

Balbharti Maharashtra State Board Class 10 Maths Solutions covers the Practice Set 1.2 Algebra 10th Class Maths Part 1 Answers Solutions Chapter 1 Linear Equations in Two Variables.

10th Standard Maths 1 Practice Set 1.2 Chapter 1 Linear Equations in Two Variables Textbook Answers Maharashtra Board

Class 10 Maths Part 1 Practice Set 1.2 Chapter 1 Linear Equations in Two Variables Questions With Answers Maharashtra Board

10th Maths 2 Practice Set 1.2 Question 1.
Complete the following table to draw graph of the equations.
i. x + y = 3
ii. x – y = 4
Answer:
i. x + y = 3

ii. x – y = 4

Linear Equations In Two Variables Practice Set 1.2  Question 2.
Solve the following simultaneous equations graphically.
i. x + y = 6 ; x – y = 4
ii. x + y = 5 ; x – y = 3
iii. x + y = 0 ; 2x – y = 9
iv. 3x – y = 2 ; 2x – y = 3
v. 3x – 4y = -7 ; 5x – 2y = 0
vi. 2x – 3y = 4 ; 3y – x = 4
Solution:
i. The given simultaneous equations are
x + y = 6                                                                                                        x – y = 4
∴ y = 6 – x                                                                                                     ∴ y = x – 4
The two lines intersect at point (5, 1).
∴ x = 5 and y = 1 is the solution of the simultaneous equations x + y = 6 and x – y = 4.

ii. The given simultaneous equations are


The two lines intersect at point (4, 1).
∴ x = 4 and y = 1 is the solution of the simultaneous equations x+y = 5 and x – y = 3.

iii. The given simultaneous equations are


The two lines intersect at point (3, -3).
∴ x = 3 and y = -3 is the solution of the simultaneous equations x + y = 0 and 2x – y = 9.

iv. The given simultaneous equations are


The two lines intersect at point (-1, -5).
∴ x = -1 and y = -5 is the solution of the simultaneous equations 3x- y = 2 and 2x- y = 3.

v. The given simultaneous equations are


The two lines intersect at point (1, 2.5).
∴ x = 1 and y = 2.5 is the solution of the simultaneous equations 3x – 4y = -7 and 5x – 2y = 0.

vi. The given simultaneous equations are


The two lines intersect at point (8, 4).
∴ x = 8 and y = 4 is the solution of the simultaneous equations 2x – 3y = 4 and 3y – x = 4.

10th Math Part 2 Practice Set 1.2  Question 1.
Solve the following simultaneous equations by graphical method. Complete the following tables to get ordered pairs.

i. Plot the above ordered pairs on the same co-ordinate plane.
ii. Draw graphs of the equations.
iii. Note the co-ordinates of the point of intersection of the two graphs. Write solution of these equations. (Textbook pg. no. 8)
Solution:

The two lines intersect at point (-1, -2).
∴ (x , y) = (-1, -2) is the solution of the given simultaneous equations.

Mathematics Part 1 Standard 9 Practice Set 1.2 Answer  Question 1.
Solve the above equations by method of elimination. Check your solution with the solution obtained by graphical method. (Textbook pg. no. 8)
Solution:
The given simultaneous equations are
x – y = 1 …(i)
5x – 3y = 1 …(ii)
Multiplying equation (i) by 3, we get
3x – 3y = 3 …(iii)
Subtracting equation (iii) from (ii), we get

Substituting x = -1 in equation (i), we get
-1 -y= 1
∴ -y = 1 + 1
∴ -y = 2
∴ y = -2
∴ (x,y) = (-1, -2) is the solution of the given simultaneous equations.
∴ The solution obtained by elimination method and by graphical method is the same.

1.2 Maths Class 10 Question 2.
The following table contains the values of x and y co-ordinates for ordered pairs to draw the graph of 5x – 3y = 1.

i. Is it easy to plot these points?
ii. Which precaution is to be taken to find ordered pairs so that plotting of points becomes easy? (Textbook pg. no. 8)
Solution:
i. No

The above numbers are non-terminating and recurring decimals.
∴ It is not easy to plot the given points.

ii. While finding ordered pairs, numbers should be selected in such a way that the co-ordinates obtained will be integers.

Linear Equations ¡n Two Variables Class 10 Maths Question 3.
To solve simultaneous equations x + 2y = 4; 3x + 6y = 12 graphically, following are the ordered pairs.

Plotting the above ordered pairs, graph is drawn. Observe it and find answers of the following questions.

i. Are the graphs of both the equations different or same?
ii. What are the solutions of the two equations x + 2y = 4 and 3x + 6y = 12? How many solutions are possible?
iii. What are the relations between coefficients of x, coefficients of y and constant terms in both the equations?
iv. What conclusion can you draw when two equations are given but the graph is only one line? (Textbook pg. no. 9)
Solution:
i. The graphs of both the equations are same.
ii. The solutions of the given equations are (-2, 3), (0, 2), (1, 1.5), etc.
∴ Infinite solutions are possible.
iii. Ratio of coefficients of x = \(\frac { 1 }{ 3 } \)
Ratio of coefficients of y = \(\frac { 2 }{ 6 } \) = \(\frac { 1 }{ 3 } \)
Ratio of constant terms = \(\frac { 4 }{ 12 } \) = \(\frac { 1 }{ 3 } \)
∴ Ratios of coefficients of x = ratio of coefficients of y = ratio of the constant terms
iv. When two equations are given but the graph is only one line, the equations will have infinite solutions.

Class 10 Maths Part 1 Practice Set 1.2 Question 4.
Draw graphs of x- 2y = 4, 2x – 4y = 12 on the same co-ordinate plane. Observe it. Think of the relation between the coefficients of x, coefficients ofy and the constant terms and draw the inference. (Textbook pg. no. 10)
Solution:

ii. Ratio of coefficients of x =\(\frac { 1 }{ 2 } \)
Ratio of coefficients of y = \(\frac { -2 }{ -4 } \) = \(\frac { 1 }{ 2 } \)
Ratio of constant terms = \(\frac { 4 }{ 12 } \) = \(\frac { 1 }{ 3 } \)
∴ Ratio of coefficients of x = ratio of coefficients of y ratio of constant terms
iii. If ratio of coefficients of x = ratio of coefficients of y ≠ ratio of constant terms, then the graphs of the two equations will be parallel to each other.

Condition of consistency in Equations:

Class 10 Maths Digest

• Practice Set 1.1 Class 10 Answers
• Practice Set 1.2 Class 10 Answers
• Practice Set 1.3 Class 10 Answers
• Practice Set 1.4 Class 10 Answers
• Practice Set 1.5 Class 10 Answers
• Problem Set 1. Class 10 Answers

Balbharti Maharashtra State Board Class 10 Maths Solutions covers the Practice Set 1.1 Algebra 10th Class Maths Part 1 Answers Solutions Chapter 1 Linear Equations in Two Variables.

10th Standard Maths 1 Practice Set 1.1 Chapter 1 Linear Equations in Two Variables Textbook Answers Maharashtra Board

Class 10 Maths Part 1 Practice Set 1.1 Chapter 1 Linear Equations in Two Variables Questions With Answers Maharashtra Board

Question 1.
Complete the following activity to solve the simultaneous equations.
5x + 3y = 9 …(i)
2x-3y=12 …(ii)
Solution:
5x + 3y = 9 …(i)
2x-3y=12 …(ii)
Add equations (i) and (ii).

Question 2.
Solve the following simultaneous equations.
i. 3a + 5b = 26; a + 5b = 22
ii. x + 7y = 10; 3x – 2y = 7
iii. 2x – 3y = 9; 2x + y = 13
iv. 5m – 3n = 19; m – 6n = -7
v. 5x + 2y = -3;x + 5y = 4
vi. \(\frac { 1 }{ 3 } \) x+ y = \(\frac { 10 }{ 3 } \) ; 2x + \(\frac { 1 }{ 4 } \) y = \(\frac { 11 }{ 4 } \)
vii. 99x + 101y = 499 ; 101x + 99y = 501
viii. 49x – 57y = 172; 57x – 49y = 252
Solution:
i. 3a + 5b = 26 …(i)
a + 5b = 22 …(ii)
Subtracting equation (ii) from (i), we get

Substituting a = 2 in equation (ii), we get
2 + 5b = 22
∴ 5b = 22 – 2
∴ 5b = 20
∴ b = \(\frac { 20 }{ 5 } \) =4
∴ (a, b) = (2, 4) is the solution of the given simultaneous equations.

ii. x + 7y = 10
∴ x = 10 – 7y …(i)
3x – 2y = 7 …1(ii)
Substituting x = 10 – ly in equation (ii), we get
3 (10 – 7y) – 2y = 7
∴ 30 – 21y – 2y = 7
∴ -23y = 7 – 30
∴ -23y = -23
∴ y = \(\frac { -23 }{ -23 } \)
Substituting y = 1 in equation (i), we get
x = 10 – 7 (1)
= 10 – 7 = 3
∴ (x, y) = (3, 1) is the solution of the given simultaneous equations.

iii. 2x – 3y = 9 …(i)
2x + y = 13 …(ii)
Subtracting equation (ii) from (i), we get

∴ (x, y) = (6, 1) is the solution of the given simultaneous equations.

iv. 5m – 3n = 19 …(i)
m – 6n = -7
∴ m = 6n – 7 …(ii)
Substituting m = 6n – 7 in equation (i), we get
5(6n – 7) – 3n = 19
∴ 30n – 35 – 3n = 19
∴ 27n = 19 + 35
∴ 27n = 54
∴ n = \(\frac { 54 }{ 27 } \) = 2
Substituting n = 2 in equation (ii), we get
m = 6(2) – 7
= 12 – 7 = 5
∴ (m, n) = (5, 2) is the solution of the given simultaneous equations.

v. 5x + 2y = -3 …(i)
x + 5y = 4
∴ x = 4 – 5y …(ii)
Substituting x = 4 – 5y in equation (i), we get
5(4 – 5y) + 2y = -3
∴ 20 – 25y + 2y = -3
∴ -23y = -3 – 20
∴ -23y = -23
∴ y = \(\frac { -23 }{ -23 } \) = 1
Substituting y = 1 in equation (ii), we get
x = 4 – 5(1)
= 4 – 5 = -1
∴ (x, y) = (-1, 1) is the solution of the given simultaneous equations.

Substituting y = 3 in equation (i), we get
x = 10 – 3(3)
= 10 – 9 = 1
∴ (x, y) = (1, 3) is the solution of the given simultaneous equations.

vii. 99x + 101 y = 499 …(i)
101 x + 99y = 501 …(ii)
Adding equations (i) and (ii), we get

Substituting x = 3 in equation (iii), we get
3 + y = 5
∴ y = 5 – 3 = 2
∴ (x, y) = (3, 2) is the solution of the given simultaneous equations.

viii. 49x – 57y = 172 …(i)
57x – 49y = 252 …(ii)
Adding equations (i) and (ii), we get

Substituting x = 7 in equation (iv), we get
7 + y = 10
∴ y = 10 – 7 = 3
∴ (x, y) = (7, 3) is the solution of the given simultaneous equations.

Complete the following table. (Textbook pg. no. 1)

Question 1.
Solve: 3x+ 2y = 29; 5x – y = 18 (Textbook pg. no. 3)
Solution:
3x + 2y = 29 …(i)
and 5x- y = 18 …(ii)
Let’s solve the equations by eliminating ‘y’.
Fill suitably the boxes below.
Multiplying equation (ii) by 2, we get

Class 10 Maths Digest

• Practice Set 1.1 Class 10 Answers
• Practice Set 1.2 Class 10 Answers
• Practice Set 1.3 Class 10 Answers
• Practice Set 1.4 Class 10 Answers
• Practice Set 1.5 Class 10 Answers
• Problem Set 1. Class 10 Answers