Important Questions

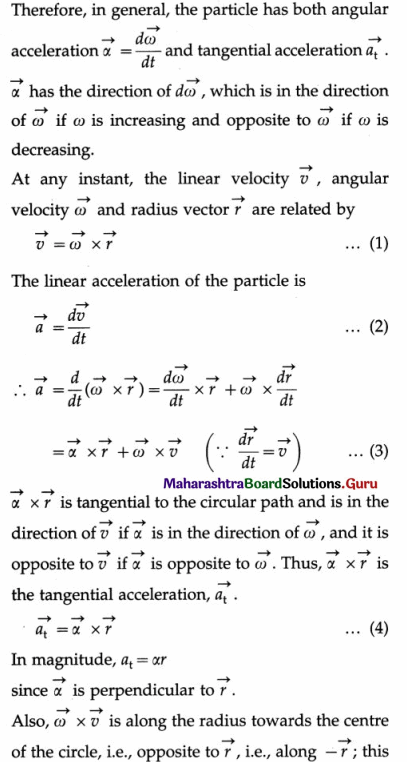
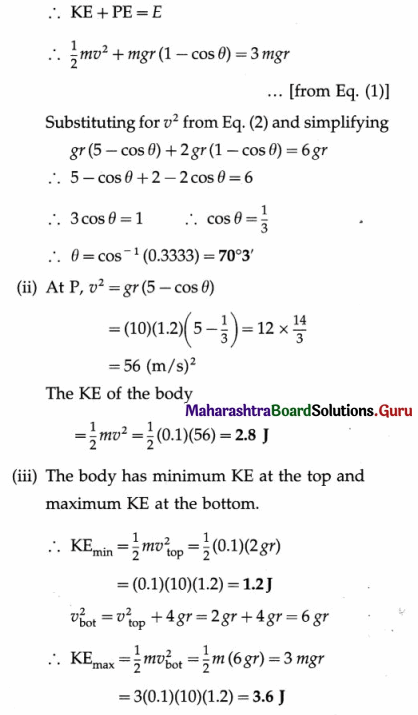
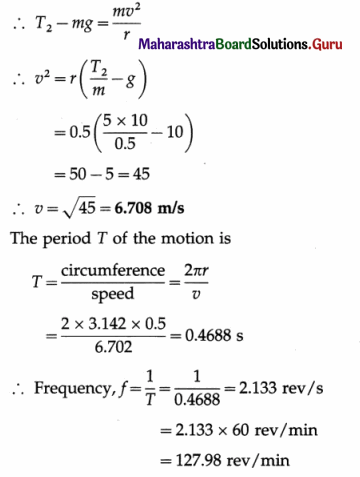
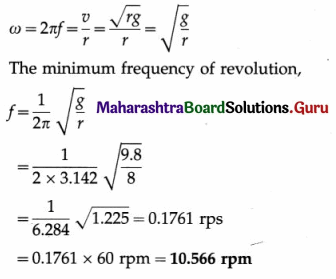
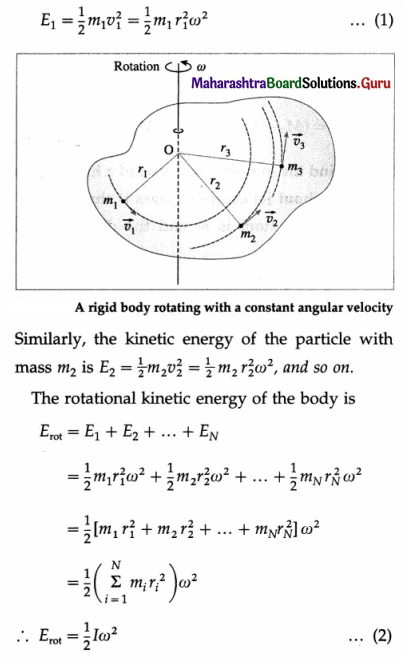
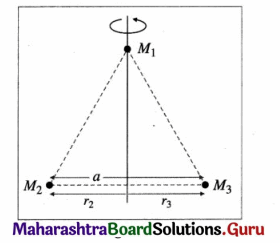
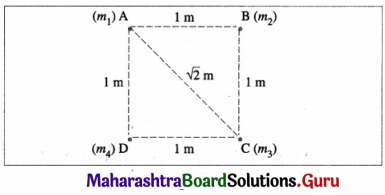
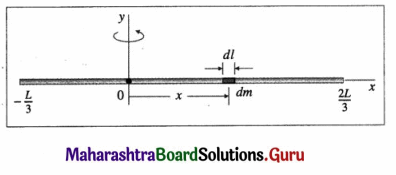
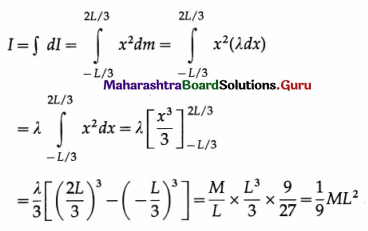
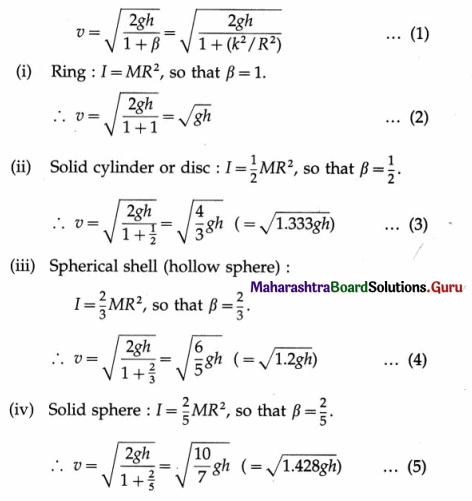
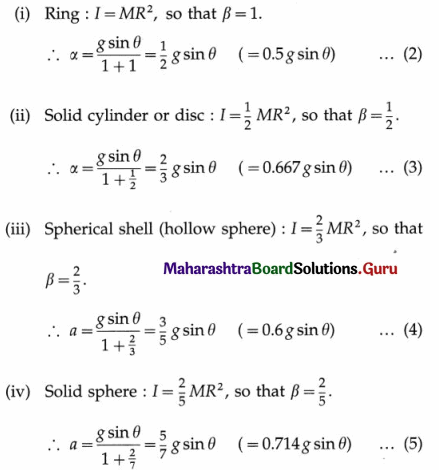
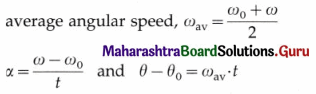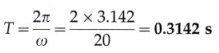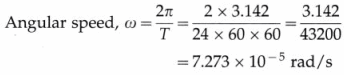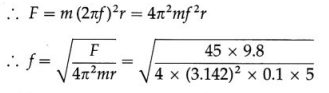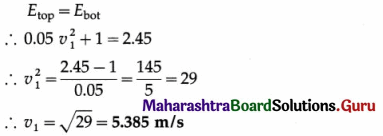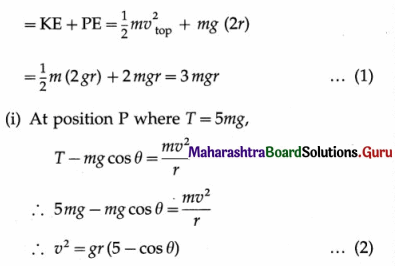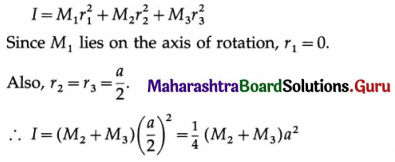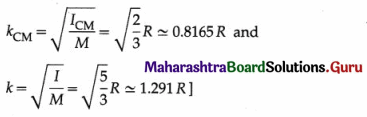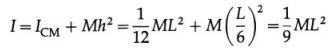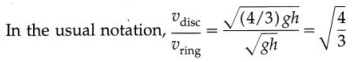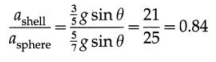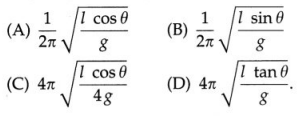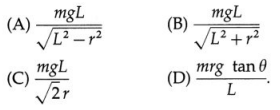Balbharti Maharashtra State Board 12th Physics Important Questions Chapter 2 Mechanical Properties of Fluids Important Questions and Answers.

Maharashtra State Board 12th Physics Important Questions Chapter 2 Mechanical Properties of Fluids

Question 1.
What is fluid? Give two examples.
Answer:
A fluid is a substance that can flow. A fluid has shear modulus O and yields to shear. Under shear stress and a pressure gradient, fluid begins to flow. Liquids, gases, and plasmas are collectively called fluids.

Examples: All gases, all liquids, molten glass and lava, honey, etc.

Question 2.
What is an ideal fluid?
OR
State the characteristics of an ideal fluid.
Answer:
An ideal fluid is one that has the following properties:

1. It is incompressible, i.e., its density has a constant value throughout the fluid.
2. Its flow is irrotational, i.e., the flow is steady or laminar. In an irrotational flow, the fluid doesn’t rotate like in a whirlpool arid the velocity of the moving fluid at a specific point doesn’t change over time. (Many fluids change from laminar to turbulent flow as the speed of the fluid increases above some specific value. This can dramatically change the properties of the fluid.)
3. Its flow is nonviscous or inviscid, i.e., internal friction or viscosity is zero so that no energy lost due the motion of the fluid.

Question 3.
How does a fluid differ from a solid ?
Answer:
In response to a shear as well as normal force, a solid deforms and develops a restoring force. Within the elastic limit, both types of deformation is reversible. A solid changes its shape under a shear. A normal force causes a change in its length or volume. If the elastic limit is exceeded, the solid gets an irreversible deformation called a permanent set.

A fluid, on the other hand, can only be subjected to normal compressive stress, called pressure. A fluid does not have a definite shape, so that under a shear it begins to flow, Real fluids, with non-zero viscosity, display a weak resistance to shear.

Question 4.
State the properties of a fluid.
Answer:
Properties of a fluid :

1. They do not resist deformation and get permanently deformed.
2. They are capable of flowing.
3. They take the shape of the container.

Question 5.
Define pressure. State its SI and CGS units and dimensions.
Answer:
Definition : The pressure at a point in a fluid in hydrostatic equilibrium is defined as the normal force per unit area exerted by the fluid on a surface of infinitesimal area containing the point.
Thus, the pressure, p = \(\lim _{\Delta A \rightarrow 0} \frac{F}{\Delta A}\)
where F is the magnitude of the normal force on a surface of area ∆A. The pressure is defined to be a scalar quantity.

SI unit: the pascal (Pa), 1 Pa = 1 N∙m^-2
CGS unit: the dyne per square centimetre (dyn/cm²)
Dimensions : [p] = [F][A^-1] = [MLT^-2, L^-2]
= [ML^-1 T^-2]

Question 6.
State two non-SI units of pressure.
Answer:
Two non-SI units, which are either of historical interest, or are still used in specific fields are the bar and the torr.
1 bar = 0.1 MPa = 100 kPa = 1000 hPa = 10⁵Pa
1 torr = (101325/760) Pa = 133.32 Pa
[Note : Their use in modern scientific and technical work is strongly discouraged.]

Question 7.
If a force of 200 N is applied perpendicular to a surface of area 10 cm², what is the corresponding pressure ?
Answer:
Pressure, p = \(\frac{F}{A}=\frac{200 \mathrm{~N}}{10 \times 10^{-6} \mathrm{~m}^{2}}\) = 2 × 10⁷ N/m²

Question 8.
Explain why the forces acting on any surface within a fluid in hydrostatic equilibrium must be normal to the surface.
Answer:
In a fluid, the molecules are in a state of random motion and the intermolecular cohesive forces are weak. If a fluid is subjected to a tangential force (shear) anywhere within it, the layers of the fluid slide over one another, i.e., the fluid begins to flow. Thus, a fluid cannot sustain a tangential force. So, in

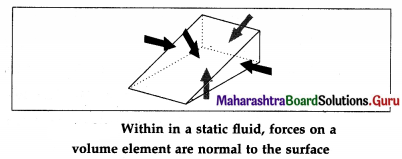
turn, a fluid at rest cannot exert a tangential force on any surface with which it is in contact. It can exert only a force normal to the surface. Hence, if a fluid is in hydrostatic equilibrium (i.e., at rest), the force acting on any surface within the fluid must be normal to the surface.

Question 9.
Would you rather have someone wearing studs step on your foot or have someone wearing tennis shoes step on your foot ?
Answer:
A person would exert the same downward force regardless of whether he or she was wearing studs or tennis shoes. However, if the person were wearing studs, the force would be applied over a much smaller area, so the pressure would be greater (and so would be more painful).

Question 10.
Would you rather have an elephant stand on your foot directly or have an elephant balance on a thumbtack on top of your foot?
Answer:
The downward force of the elephant’s weight would be applied over a much smaller area if it were balancing on a thumbtack, so the pressure would be greater.

Question 11.
Derive an expression for pressure exerted by a liquid column.
Answer:
At a point at depth h below the surface of a liquid of uniform density ρ, the pressure due to the liquid is due to the weight per unit area of a liquid column of height h above that point.

In above figure to find the pressure due to the liquid at point P, consider the cylindrical liquid column, of cross section A and height h, above that point.

The weight of this liquid column = volume × density × acceleration due to gravity
= (Ah)(ρ)(g)
∴ Pressure due to the liquid at depth h
= \(\frac{\text { weight of the liquid column }}{\text { cross sectional area }}\)
= \(\frac{A h \rho g}{A}\) = hpg
If the free surface of the liquid is open to the atmosphere, the pressure on the surface is the atmosphere pressure p₀. Then, the absolute pressure within the liquid at a depth h is p = p₀ + hρg

Question 12.
State the characteristics of pressure due to a liquid at rest at a point within it.
Answer:
Characteristics of pressure due to a liquid at rest at a point within it:

1. Within a liquid of constant density, the pressure is directly proportional to the depth.
2. At the same depth within liquids of different densities, the pressure is directly proportional to the density of the liquid.
3. Within a liquid of constant density, the pressure at a given depth is directly proportional to the acceleration due to gravity.
4. The pressure at a point within a given liquid is the same in all directions.
5. The pressure at all points at the same horizontal level within a given liquid is the same.

Question 13.
How much force is exerted on one side of an 8.50 cm by 11.0 cm sheet of paper by the atmosphere? How can the paper withstand such a force ?
Answer:
Pressure p = F/A. Therefore, the force on one side is F = ρ ∙ A = (1.013 × 10⁵ Pa) (8.50 × 11.0 × 10^-4 m²) = 947.2 N.

The pressure at a point within a fluid being the same in all directions, the same force acts on the other side of the paper. Thus, the net force on the paper is zero.

Question 14.
What is the pressure exerted by a water column of height 1 m?[ρ = 10³ kg/m³, g = 9.8 m/s²]
Answer:
Pressure exerted by the water column = hρg
= 1 m(10³ kg/m³) × (9.8 m/s²)
= 9.8 × 10³Pa

Question 15.
Would you rather breathe through a 2 m long tube to the surface in 1.5 m of water in the ocean or breathe at the beach near the ocean?
Answer:
The pressure on one’s lungs would be much greater under water than standing on the beach because the force exerted by the water on the lungs ‘ is greater than the force exerted by the air. Because the pressure of the water on the lungs is so much greater than the outward pressure of the air inside, it would be difficult to take a breath under 1.5 m of water than on the beach.

Question 16.
What is atmospheric pressure ? Define standard atmospheric pressure.
Answer:
The Earth’s surface is covered with a layer of atmosphere, with more than 99% of the atmosphere lying within 31 km of the surface. The weight of the atmosphere exerts a downward thrust on any surface lying within it. This gives rise to atmospheric pressure. The atmospheric pressure at any height above the Earth’s surface is the weight of a column of air of unit cross section from that altitude to the top of the atmosphere.

Definition : Standard atmospheric pressure, or one atmosphere of pressure, is defined as the pressure equivalent of a column of mercury that is exactly 0.7600 m in height at 0 °C.

We can calculate this equivalent pressure in SI unit by using the density of mercury
ρ = 13.6 × 10³ kg/m³ and g = 9.80 m/s².
1 atm = (0.76 m) . (13.6 × 10³ kg/m³) . (9.80 m/s²)
= 1.013 × 10⁵ Pa = 101.3 kPa
[Note : 1000 mbar = 100 kPa. Therefore, 1 atm = 1013 mbar.]

Question 17.
Explain gauge pressure and absolute pressure within a liquid open to the atmosphere.
OR
Explain the effect of gravity on fluid pressure.
Answer:
Consider a cylindrical fluid column of uniform density ρ, area of cross section A and height h,

The mass of the fluid within the column is
m = density × volume
= ρAh

If p₁ and p₂ are the pressures at the top and bottom faces of the column, the forces on the top and bottom faces are respectively.
F₁ = p₁A + mg (downward)
and F₂ = p₂A (upward)
If the column is in equilibrium,
F₂ = F₁
∴ p₂A = p₁A + mg = p₁A + ρAhg
∴ P₂ ~ P₁ = ρhg
If p₁ = p₀ = atmospheric pressure, the gauge pressure
P₂ – P₀ = ρhg

In the absence of gravity, p₂ = p₀ But since atmospheric pressure is equal to the weight per unit area of the entire air column above, even p0 will be zero in the absence of gravity.

Question 18.
Define gauge pressure.
When is gauge pressure (i) positive (ii) negative ?
Give two examples where gauge pressure is more relevant.
Answer:
Definition : Gauge pressure is the pressure exerted by a fluid relative to the local atmospheric pressure.

Gauge pressure, p_g = p – p₀

where p is the absolute pressure and p₀ is the local atmospheric pressure.

When the pressure inside a closed container or tank is greater than atmospheric pressure, the pressure reading on a pressure gauge is positive. The pressure inside a ‘vacuum chamber’-a rigid chamber from which some of the air is pumped out-is less than the atmospheric pressure, so a pressure gauge on the chamber designed to measure negative pressure reads a negative value.

At a depth within a liquid of density ρ, the gauge pressure is p_g = p – p₀ = hpg

Examples : Tyre pressure gauge, blood pressure gauge, pressure gauge on an oxygen or scuba tank.

Question 19.
Define absolute pressure.
Answer:
Definition : The absolute pressure, or total press-ure, is measured relative to absolute zero on the pressure scale-which is a perfect vacuum-and is the sum of gauge pressure and atmospheric press-ure. It is the same as the thermodynamic pressure.

Absolute pressure accounts for the atmospheric pressure, which in effect adds to the pressure in any fluid which is not enclosed in a rigid container i.e., the fluid is open to the atmosphere.
p = p₀ + P_g
where p₀ and p_g are respectively atmospheric pressure and the gauge pressure.

Absolute pressure can be never negative.

Question 20.
If your tyre gauge reads 2.31 atm (234.4 kPa), what is the absolute pressure ?
Answer:
The absolute pressure, p = p₀ + p_g = 1 atm + 2.31 atm = 3.31 atm (≅ 335 kPa).

Question 21.
State and explain the hydrostatic paradox. OR Explain hydrostatic paradox.
Answer:
Hydrostatic paradox : The normal force exerted by a liquid at rest on the bottom of the containing vessel is independent of the amount of liquid or the shape of the container, but depends only on the area of the base and its depth from the liquid surface.

Consider several vessels of the same base area as shown in figure (a). A liquid is poured into them to the same level, so that the pressure is the same at the bottom of each vessel. Then it must follow that the normal force on the base of each vessel is also the same. However, when placed on a scale balance they are found to have different weights. Herein lies the paradox.

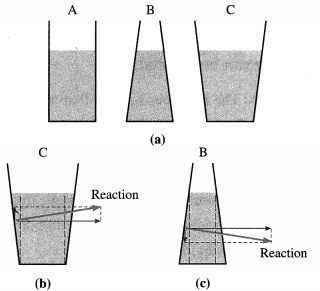
Explanation : Since a liquid always exerts a normal force on a wall of the container, in turn, the wall exerts an equal and opposite reaction on the liquid. In the case of tube A, this reaction is everywhere horizontal; so that the normal force at the base of A is only due to the weight of the liquid column above.

The reaction of the slanted wall of vessel C has a – vertical component, as shown in figure (b), which supports the weight of the liquid above the slanted side. Hence, the normal force at the base of C is only due to the weight of the vertical liquid column above the base, shown by dashed lines. Since the vessels A and C are filled to the same height and have the same base area, the pressures at the bases of the two vessels are also same. However, the volume of the liquid being clearly different, they have different weights.

In the case of vessel B, the downward vertical component of the reaction of the wall provides an extra normal force at the base, as shown in figure (c).

Question 22.
Can pressure in a fluid be increased by pushing directly on the fluid ? Give an example.
Answer:
Yes, but it is much easier if the fluid is enclosed.

Examples : (1) The heart increases the blood pressure by pushing on the blood in an enclosed ventricle.
(2) Hydraulic brakes, lifts and cranes operate by pushing on oil in an enclosed system.

Question 23.
State Pascal’s law.
Answer:
Pascal’s law : A change in the pressure applied to an enclosed fluid at rest is transmitted un-diminished to every point of the fluid and to the walls of the container, provided the effect of gravity can be ignored.

[Note : The law does not say that ‘the pressure is the same at all points of a fluid’ – rightly so, since the pressure in a fluid near Earth varies with height. Rather, the law applies to the change in pressure. According to Pascal’s law, if the pressure on an enclosed static fluid is changed by a certain amount, the pressure at all points within the fluid changes by the same amount.

The above law is due to Blaise Pascal (1623 – 62), French mathematician and physicist.]

Question 24.
Describe an experimental proof of Pascal’s law.
Answer:
Consider a spherical vessel having four cylindrical tubes A, B, C and D each fitted with air-tight

frictionless pistons of areas of cross section A, A/2, 2A and 3A, respectively, as shown in above figure. The vessel is filled with an incompressible liquid such that there is no air between the liquid and the pistons.

If the piston A is pushed with a force F, the pressure on the piston and the liquid in the vessel is p_A = F/A. It is seen that the other three pistons are pushed outwards. To keep these pistons at their respective original positions, forces of F/2, IF and 3F, respectively are required to be applied on pistons B, C and D respectively to hold them. Then, the pressures on the respective pistons are
p_B = \(\frac{F / 2}{A / 2}\) = F/A, p_C = 2F/2A = F/A, and
p_D = 3F/3A = F/A
∴ p_A = p_B = p_C = p_D = F/A

This indicates that the pressure applied is trans-mitted equally to all parts of liquid. This proves Pascal law.

Question 25.
Explain the principle of multiplication of thrust.
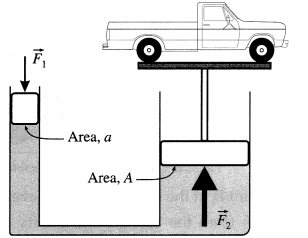
Answer:
Principle of multiplication of thrust by transmission of fluid pressure : The normal force exerted by a fluid on any surface in contact with it is called the thrust. Consider two hydraulically connected cylinders, one of cross section a and the other A, as in figure. If a force F_a is exerted on the smaller piston, pressure p = \(\frac{F_{\mathrm{a}}}{a}\) is produced and transmitted undiminished throughout the liquid. Then, the thrust F_A on the larger piston is
F_A = pA = \(\frac{A}{a}\) F_a
If A = na, F_A = nF_a, i.e., the thrust on the larger piston is multiplied n times. This is known as the principle of multiplication of thrust by transmission of fluid pressure.

Question 26.
State any two applications of Pascal’s law.
Briefly explain their working.
Ans.
Applications of Pascal’s law :

1. Hydraulic car lift and hydraulic press
2. Hydraulic brakes.

All the above applications work on the principle of multiplication of thrust by transmission of fluid pressure.

(1) Working of a hydraulic lift : Two hydraulically connected cylinders, one of cross section a and the other A, are such that A is many times larger than a : A = na. If a force F_a is exerted on the smaller piston, a pressure p = \(\frac{F_{\mathrm{a}}}{a}\) is produced and transmitted undiminished throughout the liquid. Then, the thrust F_A on the larger piston
F_A = pA = \(\frac{A}{a}\) F_a = nF_a
is n times greater than that on the smaller piston. A platform attached to the larger piston can lift a car (as in a hydraulic car lift), or press bales of cotton or paper against a fixed rigid frame (as in Brahma’s.)

(2) Working of hydraulic brakes in a car: Brakes which are operated by means of hydraulic pressure are called hydraulic brakes. An automobile hydraulic brake system, shown schematically in below figure, has fluid-filled master and slave cylinders connected by pipes. When the brake pedal is pushed, it depresses the piston of the pedal or master cylinder through a lever. The change in pressure in the master cylinder is transmitted to the four wheel or slave cylinders. Since the brake fluid is incompressible, the pistons of the slave cylinders are pushed out, pressing braking pads onto the braking discs on the wheels. Note that we can add as many wheel cylinders as we wish.

The master cylinder has a much smaller area of cross section A_m compared to the combined area A_s

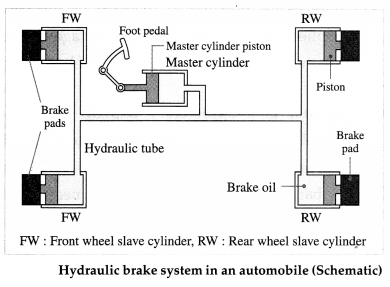
of the slave cylinders. Hence, with a small force F_m on the master cylinder, a force F_s = \(\frac{A_{\mathrm{s}}}{A_{\mathrm{m}}}\) F_m which is greater than F_m is applied on each slave cylinder. Consequently, the master piston has to travel sev-eral inches to move the slave pistons the fraction of an inch it takes to apply the brakes. But the arrangement allows great force to be exerted at the brake pads.

[Note : (1) Pascal’s law laid the foundation for hydraulics, the use of a liquid under pressure to transfer force or motion, or to increase an applied force. It is one of the most important branches in modern engineering. (2) A hydraulic system, as an example of a simple machine, can increase force but cannot do more work than is done on it. Work being force times the distance moved, the piston in a wheel cylinder moves through a smaller distance than that in the pedal cylinder. Power brakes in modern automobiles have a motorized pump that does most of the work in the system.]

Question 27.
Why are liquids used in hydraulic systems but not gases?
Answer:
Liquids are used in a hydraulic system because liquids are incompressible and transmit a change in pressure undiminished to all parts of the system. On the other hand, on increasing the pressure, a gas will be compressed into a smaller volume due to which there will be no transmission of force or motion.

Question 28.
State one advantage of hydraulic brakes in an automobile.
Answer:
Advantages of

1. By Pascal’s law, equal braking effort is applied to all the wheels.
2. It is easily possible to increase or decrease the applied force-during the design stage-by changing the size of piston and cylinder relative to other.

Question 29.
What is a barometer? Explain the use of a simple mercury barometer to measure atmospheric pressure.
Answer:
A barometer is an instrument to measure atmospheric pressure. The mercury barometer was in-vented by Evangelista Torricelli (1609-47). Italian physicist and mathematician.

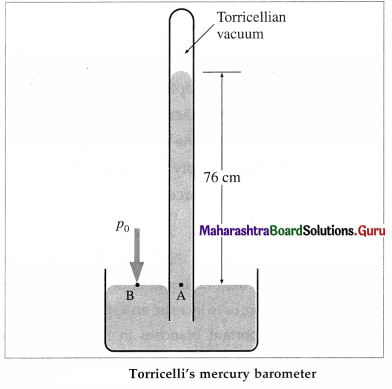
A strong glass tube, about one metre long and closed at one end, is filled with mercury. With a finger over the open end, the tube is inverted and the open end is immersed into a bowl of mercury. When the finger is removed, the mercury level in the tube drops. The mercury column in the tube stands at a height h for which the pressure at point A inside the tube due to the weight of the mercury column is equal to the atmospheric pressure p0 outside (at point B).

The space at the closed end of the tube, after the mercury level drops, is nearly a vacuum, known as the Torricellian vacuum, so the pressure there can be taken as zero. It, therefore, follows that p₀ = pgh Where p is the density of mercury and h is the height of the mercury column.

Question 30.
What is an open tube manometer? Briefly describe its function with a neat diagram.
Answer:
An open tube manometer is a device to measure the pressure of a gas in a vessel. It consists of a U-shaped tube containing a liquid (say, mercury) of density p, as shown in below figure.

One end of the tube is connected to the vessel while the other end is open to the atmosphere. The pressure p at point A is the (unknown) pressure of the gas in the vessel. The pressure on the mercury column in the open tube is the atmospheric pressure p₀.

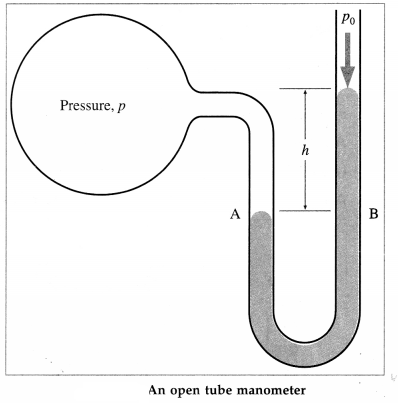
A point B, at the same horizontal level as A, is at a depth h from the surface of mercury in the open tube. Therefore, the pressure at B is p₀ + ρgh.

The pressures at points A and B at the same liquid level being the same, equating the unknown pressure p (at A) to the pressure at B.
p = p₀ + ρgh
The pressure p is called the absolute pressure, and the difference in pressure p – p₀ is called the gauge pressure.

Question 31.
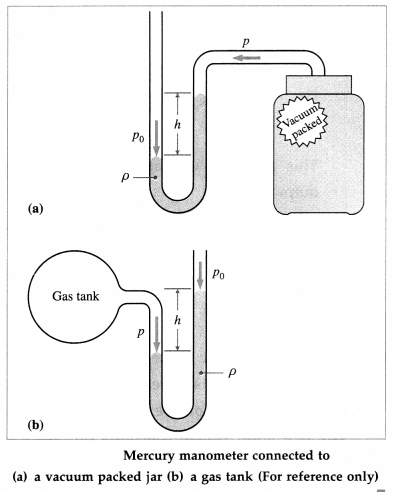
An open tube manometer is connected to (i) a vacuum-packed candy jar, with the atmospheric pressure in the open tube supporting a column of fluid of height h (ii) a gas tank, with the absolute pressure in the tank supporting a column of fluid of height h. Is the absolute pressure in the jar and the gas tank greater than or less than the atmospheric pressure ? By how much ?
Answer:
In the first case, p_abs is less than the atmospheric pressure, whereas in the second case, p_abs is greater than the atmospheric pressure. In both cases, p_abs differs from the atmospheric pressure by the gauge pressure hρg, where ρ is the density of the fluid in the manometer.

32. Solve the following

Question 1.
For diver’s safety, a 10 m platform diving pool should be 5 m deep. However, with an excellent dive, a diver usually reaches a maximum depth of 2.5 m.
(i) Calculate the pressure due to the weight of the water at the depth of 2.5 m.
(ii) Calculate the depth below the surface of water at which the pressure due to the weight of the water equals 1.0 atm. [Density of water = 10³ kg/m³, 1 atm = 101.3 kPa]
Solution:
Data : h = 250 m, ρ = 1000 kg/m³, g = 9.8 m/s², 1 atm = 101.3 kPa
(i) ρ = hpg = (250)(1000)(9.8) = 2.45 mPa
= \(\frac{2.45 \times 10^{6}}{1.013 \times 10^{5}}\) = 24.18 atm
This gives the pressure at a depth of 250 m.

(ii) h = \(\frac{p}{\rho g}=\frac{1.013 \times 10^{5}}{10^{3} \times 9.8}\) = 10.34 m
This gives the required depth.

Question 2.
Suppose a dam is 250 m wide and the water is 40 m deep at the dam. What is
(i) the average pressure on the dam
(ii) the force exerted against the dam due to the water?
Solution :
Data : Width, L = 250 m, depth H = 40 m, ρ = 1000 kg/m³, g = 9.8 m/s²
Since pressure increases linearly with depth, the average pressure p_av due to the weight of the water is the pressure at the average depth h of 20 m. The force exerted on the dam by the water is the average pressure times the area of contact, F = p_av A = p_av LH.
(i) p _av = hρg = (20)(1000)(9.8) = 1.96 × 10⁵Pa
(ii) F = p_avA = p_avLH = (1.96 × 10⁵)(250)(40)
= 1.96 × 10⁵ N

Question 3.
A car lift at a service station has a piston of diameter 30 cm. The lift and piston weigh 800 kg wt. What pressure (in excess of the atmospheric pressure) must be exerted on the piston to raise a car weighing 1700 kg wt at a constant speed? [g = 9.8 m/s²]
Solution:
Data : Piston diameter, D = 30 cm = 0.3 m. mass of lift and piston, m = 800 kg, mass of car, M = 1700 kg
Cross-sectional area of the piston.
A = \(\frac{\pi D^{2}}{4}=\frac{3.142(0.3 \mathrm{~m})^{2}}{4}\) = 7.07 × 10^-2 m ²
Total weight of the car and lift,
W = (m + M)g
= (800 kg + 1700 kg) (9.8 m/s²)
= 2.45 × 10⁴ N
Therefore, the pressure on the piston
p = \(\frac{F}{A}=\frac{W}{A}=\frac{2.45 \times 10^{4} \mathrm{~N}}{7.07 \times 10^{-2} \mathrm{~m}^{2}}\)
= 3.465 × 10⁵ Pa
A pressure of 3.465 × 10⁵ Pa must be exerted on the piston.

Question 4.
The diameters of two pistons in a hydraulic press are 5 cm and 25 cm respectively. A force of 20 N is applied to the smaller piston. Find the force exerted on the larger piston.
Solution:
Data : D₁ = 5 cm, D₂ = 25 cm, F₁ = 20 N
By Pascal’s law,

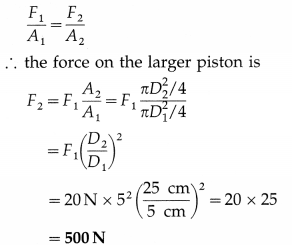
Question 5.
In a hydraulic lift, the input piston has surface area 20 cm². The output piston has surface area 1000 cm². If a force of 50 N is applied to the input piston, it raises the output piston by 2 m. Calculate the weight of the support on the output piston and the work done by it.
Solution:
Data : A₁ = 20 cm² = 2 × 10^-3 m²,
A₂ = 1000 cm² = 10^-1 m², F₁ = 50 N, s₂ = 2m
(i) By Pascal’s law,

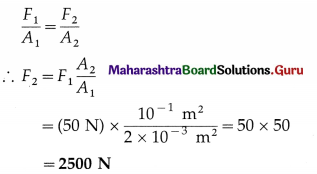
This gives the weight of the support on the output piston.

(ii) The work done by the force transmitted to the output piston is
F₂S₂ = (2500 N) (2 m)
= 5000 J

Question 6.
A driver pushes the brake pedal of a car exerting a force of 100 N that is increased by the simple lever to a force of 500 N on the pedal (master) cylinder. The hydraulic system transmits this force to the four wheel (slave) cylinders. If the pedal cylinder has a diameter of 0.5 cm and each wheel cylinder has a diameter of 2.5 cm, calculate the magnitude of the force F_s on each of the wheel cylinder.
Solution:
Data : F_m = 500 N, D_m = 1 cm, D_s = 2.5 cm
\(\frac{F_{\mathrm{s}}}{A_{\mathrm{s}}}=\frac{F_{\mathrm{m}}}{A_{\mathrm{m}}}\)
∴ The magnitude of the force on each of the wheel cylinders,
F_s = \(\frac{A_{\mathrm{s}}}{A_{\mathrm{m}}}\) F_m = (\(\frac{D_{\mathrm{s}}}{D_{\mathrm{m}}}\))² F_m = (\(\frac{2.5}{0.5}\))² (500)
= 25 × 500 = 12.5 kN

Question 7.
Mercury manometers are often used to measure arterial blood pressure. The typical blood pressure of a young adult raises the mercury to a height of 120 mm at systolic and 80 mm at diastolic. Express these values in pascal and bar. [Density of mercury = 13600 kg/m³, 1 mbar = 100 Pa]
Solution:
Data : p_max = p_syst = 120 mm of Hg, p_min = p_dias = 80 mm of Hg, ρ = 13600 kg/m³, g = 9.8 m/s², 1 mbar = 100 Pa
p = hρg
∴ p_syst = (0.120)(1.36 × 10⁴)(9.8)
= 1.6 × 10⁴ Pa = 16 kPa
= 1600 mbar = 1.5 bar

and P_dias = (0.08)(1.36 × 10⁴)(9.8)
= 1.066 × 10⁴ Pa = 10.66 kPa
= 1066 mbar = 1.066 bar

Question 33.
Describe the phenomenon of surface tension, giving four examples.
Answer:
Surface tension is a unique property of liquids that arises because the net intermolecular force of attraction on the liquid molecules at or near a liquid surface differs from that on molecules deep in the interior of the liquid. This results in the tendency of the free surface of a liquid to minimize its surface area and behave somewhat like a stressed elastic membrane.

Surface tension is important in understanding the peculiar behaviour of the free surface of a liquid in many cases as illustrated below :

1. Small quantities of liquids assume the form of spherical droplets, as in mist, or a mercury droplet on a flat surface. This is because the stressed surface ‘skin’ tends to contract and mould the liquid into a shape that has minimum surface area for its volume, i.e., into a sphere.
2. Surface tension is responsible for the spherical shape of freely-falling raindrops and the behaviour of bubbles and soap films.
3. The bristles of a paint brush cling together when it is drawn out of water or paint.
4. A steel needle or a razor blade can, with care, be supported on a still surface of water which is much less dense than the metal from which these objects are made of.
5. Many insects like ants, mosquitoes, water striders, etc., can walk on the surface of water.

Question 34.
Define (1) cohesive force (2) adhesive force.
Give one example in each case.
Answer:
(1) Cohesive force : The intermolecular force of attraction between two molecules of the same material is called the cohesive force.
Example : The force of attraction between two water molecules.

(2) Adhesive force : The intermolecular force of attraction between two molecules of different materials is called the adhesive force.
Example : The force of attraction between a water molecule and a molecule of the solid surface which is in contact with water.

Question 35.
Define (1) range of molecular attraction or molecular range (2) sphere of influence.
Answer:
1) Range of molecular attraction or molecular range : Range of molecular aftraction or molecular range is defined as the maximum distance between two molecules up to which the intermolecular force of attraction is appreciable.

[Note : The intermolecular force is a short range force, LeV, it is effective over a very short range-about 10^-9 m. Beyond this distance, the force is negligible. The inter molecular force does not obey inverse square law.]

2) Sphere of influence : The sphere of influence of a molecule is defined as an imaginary sphere with the molecule as the centre and radius equal to the range of molecular attraction.

[Note : All molecules lying within the sphere of influence of a molecule are attracted by (as well as attract) the molecule at the centre. For molecules which lie outside this sphere, the intermolecular force due to the molecule at the centre is negligible.]

Question 36.
What is meant by a surface film?
Answer:
The layer of the liquid surface of thickness equal to the range of molecular attraction is called a surface film.

Question 37.
What is meant by free surface of a liquid ?
Answer:
The surface of a liquid open to the atmosphere is called the free surface of the liquid.

Question 38.
Explain the phenomenon of surface tension on the basis of molecular theory.
Answer:
The phenomenon of surface tension arises due to the cohesive forces between the molecules of a liquid. The net cohesive force on the liquid molecules within the surface film differs from that on molecules deep in the interior of the liquid.

Consider three molecules of a liquid : A molecule A well inside the liquid, and molecules B and C lying within the surface film, shown in figure. The figure also shows their spheres of influence of radius R.

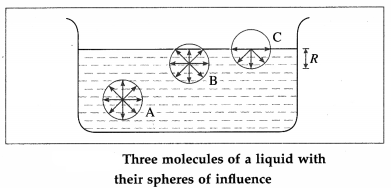
(1) The sphere of influence of molecule A is entirely inside the liquid and the molecule is surrounded by its nearest neighbours on all sides. Hence, molecule A is equally attracted from all sides, so that the resultant cohesive force acting on it is zero. Hence, it is free to move anywhere within the liquid.

(2) For molecule B, a part of its sphere of influence is outside the liquid surface. This part contains air molecules whose number is negligible compared to the number of molecules in an equal volume of the liquid. Therefore, molecule B experiences a net cohesive force downward.

(3) For molecule C, the upper half of its sphere of influence is outside the liquid surface. Therefore, the resultant cohesive force on molecule C in the
downward direction is maximum.

(4) Thus, all molecules lying within a surface film of thickness equal to R experience a net cohesive force directed into the liquid.

(5) The surface area is proportional to the number of molecules on the surface. To increase the surface area, molecules must be brought to the surface from within the liquid. For this, work must be done against the cohesive forces. This work is stored in the liquid surface in the form of potential energy. With a tendency to have minimum potential energy, the liquid tries to reduce the number of molecules on the surface so as to have minimum surface area. This is why the surface of a liquid behaves like a stressed elastic membrane.

Question 39.
Define surface tension.
State its formula and CGS and SI units.
Answer:
The surface tension of a liquid is defined as the tangential force per unit length, acting at right angles on either side of an imaginary line on the free surface of the liquid.

If F is the force on one side of a line of length Z, drawn on the free surface of a liquid, the surface tension (T) of the liquid is

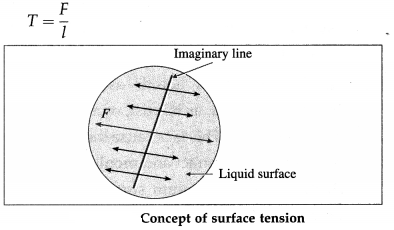
The CGS unit of surface tension : The dyne per centimetre (dyn/cm) or, equivalently, the erg per square centimetre (erg/cm²).

The SI unit of surface tension : The newton per metre (N/m) or, equivalently, the joule per square metre (J/m²).

Question 40.
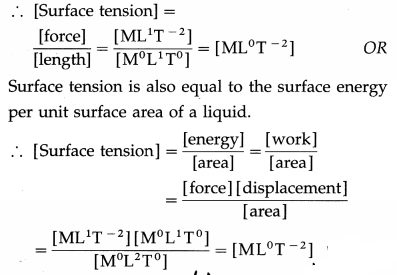
Obtain the dimensions of surface tension.
Answer:
Surface tension is a force per unit length.

Question 41.
Define and explain surface energy of a liquid.
OR
Define surface energy.
OR
State its dimensions and SI unit.
OR
Why do molecules of a liquid in the surface film possess extra energy?
Answer:
Surface energy : The surface energy is defined as the extra (or increased) potential energy possessed by the molecules in a liquid surface with an isothermal increase in the surface area of the liquid.

A liquid exerts a resultant cohesive force on every molecule of its surface, trying to pull it into the liquid. To increase the surface area, it is necessary to bring more molecules from inside the liquid to the liquid surface. For this, external work must be done against the net cohesive forces on the molecules. This work is stored in the liquid surface in the form of potential energy.

This extra potential energy that the molecules in the liquid surface have is called the surface energy. Thus, the molecules of a liquid in the surface film possess extra energy.
Dimensions : [surface energy] = [ML²T^-2]
SI unit: the joule (J).

Question 42.
Why is the surface tension of paints and lubricating oils kept low?
Answer:
For better wettability (surface coverage), the surface tension and angle of contact of paints and lubricating oils must be low.

Question 43.
Derive the relation between the surface tension and surface energy of a liquid.
OR
Derive the relation between surface tension and surface energy per unit area.
OR
Show that the surface tension of a liquid is numerically equal to the surface energy per unit area.
Answer:
Suppose a soap film is isothermally stretched over the area enclosed by a U-shaped frame ABCD and a w cross-piece PQ that can slide smoothly along the frame, as shown in the figure. Let T be the surface tension of the soap solution and l, the length of wire PQ in contact with the soap film.

The film has two surfaces, both of which are in contact with the wire. The film tends to contract by exerting a force on wire PQ. As each surface exerts a force Tl, the net force on the wire is 2Tl.

Suppose that wire PQ is pulled outward very slowly through a distance dx to the position P’Q’ by an external force of magnitude 2T l. The work done by the external force against the force due to the film is
W = applied force × displacement
∴ W = Fdx = ITldx (∵ F = 2Tl)
This work is stored in the unit surface area in the form of potential energy. This potential energy is called the surface energy.

Due to the displacement dx, the surface area of the film increases. As the film has two surfaces, the increase in its surface area is
A = 2ldx
Thus, the work done per unit surface area is
\(\frac{W}{A}=\frac{2 T l d x}{2 l d x}\) = T
Thus, the surface energy per unit area of a liquid is equal to its surface tension.

Question 44.
Two soap bubbles of the same soap solution have diameters in the ratio 1 : 2. What is the ratio of work done to blow these bubbles ?
Answer:
Work done oc surface area.
∴ W₁/W₂ = (r₁/r₂)² = (\(\frac{1}{2}\))² = \(\frac{1}{4}\)
∴ W₁ : W₁ = 1 : 4.

Question 45.
If the surface tension of a liquid is 70 dyn/cm, what is the total energy of the free surface of the liquid drop of radius 0.1 cm ?
Answer:
E = 4πr²T = 4 × \(\frac{22}{7}\) × (0.1)² × 70
= 88 × 10^-2 × 10 = 8.8 ergs

Question 46.
The total energy of the free surface of a liquid drop of radius 1 mm is 10 ergs. What is the total energy of a liquid drop (of the same liquid) of radius 2 mm ?
Answer:
E = 4πr²T ∴ \(\frac{E_{2}}{E_{1}}=\left(\frac{r_{2}}{r_{1}}\right)^{2}=\left(\frac{2}{1}\right)^{2}\) = 4
∴ E₂ = 4E₁ = 4 × 10 = 40 ergs is the required

47. Solve the following

Question 1.
Calculate the work done in blowing a soap bubble of radius 4 cm. The surface tension of the soap solution is 25 × 10^-3 N/m.
Solution:
Data : r = 4 cm = 4 × 10^-2 m, T = 25 × 10^-3 N/m
Initial surface area of soap bubble = 0
Final surface area of soap bubble = 2 × 4πr²
Increase in surface area = 2 × 4πr² The work done
= surface tension x increase in surface area
= T × 2 × 4πr²
= 25 × 10^-3 × 2 × 4 × 3.142 × (4 × 10^-2)2
= 1.005 × 10^-3 J

Question 2.
Two soap bubbles have radii in the ratio 4 : 3. What is the ratio of work done to blow these bubbles?
Solution:
Data : \(\frac{r_{1}}{r_{2}}=\frac{4}{3}\)
Work done, W = 2TdA
∴ W₁ = 2T(4πr₁²), W₂ = 2T(4πr₂²)
∴\(\frac{W_{1}}{W_{2}}=\frac{2 T\left(4 \pi r_{1}^{2}\right)}{2 T\left(4 \pi r_{2}^{2}\right)}=\left(\frac{r_{1}}{r_{2}}\right)^{2}\)
= (\(\frac{4}{3}\))² = \(\frac{16}{9}\)

Question 3.
Calculate the work done in increasing the radius of a soap bubble in air from 1 cm to 2 cm. The surface tension of the soap solution is 30 dyn/cm.
Solution:
Data : r₁ = 1 cm, r₂ = 2 cm, T = 30 dyn/cm
Initial surface area = 2 × 4πr₁²
Final surface area = 2 × 4πr₂²
∴ Increase in surface area
= 2 × 4πr₂² – 2 × 4πr₁² = 8π(r₂² – r₁²)
∴ The work done
= surface tension × increase in surface area
= T × 8π(r₂² – r₁²)
= 30 × 8 × 3.142 × [(2)² – (1)²]
= 2262 ergs

Question 4.
A mercury drop of radius 0.5 cm falls from a height on a glass plate and breaks into one million droplets, all of the same size. Find the height from which the drop fell. [Density of mercury = 13600 kg/m3, surface tension of mercury = 0.465 N/m]
Solution:
Data : R = 0.5 cm = 0.5 × 10^-2 m, n = 10⁶, ρ = 13600 kg m³, T = 0.465 N/m, g = 9.8 m/s²
\(\frac{4}{3}\) πR³ = n × \(\frac{4}{3}\) πr³
as the volume of the mercury remains the same.
∴ r = \(\frac{R}{\sqrt[3]{n}}=\frac{0.5 \times 10^{-2}}{\sqrt[3]{10^{6}}}\) = 0.5 × 10^-4 m
This gives the radius of a droplet.
By energy conservation, if h is the height from which the drop of mass m falls,

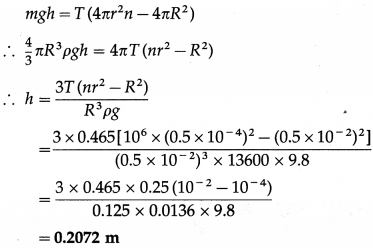
This gives the required height.

Question 5.
Eight droplets of mercury, each of radius 1 mm, coalesce to form a single drop. Find the change in the surface energy. [Surface tension of mercury = 0.472 J/m²]
Solution:
Data : r = 1 mm = 1 × 10^-3 m, T = 0.472 J/m²
Let R be the radius of the single drop formed due to the coalescence of 8 droplets of mercury.
Volume of 8 droplets = volume of the single drop as the volume of the liquid remains constant.
∴ 8 × \(\frac{4}{3}\) πr³ = \(\frac{4}{3}\) πR³
∴ 8r³ = R³
∴ 2r = R
Surface area of 8 droplets = 8 × 4πr²
Surface area of single drop = 4πR²
∴ Decrease in surface area = 8 × 4πr² – 4πR²
= 4π(8r² – R²)
= 4π[8r² – (2r)²]
= 4π × 4r²
∴ The energy released
= surface tension × decrease in surface area
= T × 4π × 4r²
= 0.472 × 4 × 3.142 × 4 × (1 × 10^-3)²
= 2.373 × 10^-5 J

Question 6.
The total energy of the free surface of a liquid drop is 2 × 10^-4 π times the surface tension of the liquid. What is the diameter of the drop ? (Assume all terms in SI unit.)
Solution:
Data : 4πr²T = 2 × 10^-4 πT (numerically)
∴ 2r² = 10^-4
∴ r = \(\frac{10^{-2}}{\sqrt{2}}=\frac{10^{-2}}{1 \cdot 414}\)
= 0.7072 × 10^-2 m
∴ d = 2r = 2 × 0.7072 × 10^-2
= 1.4144 × 10^-2 m
This gives the diameter of the liquid drop.

Question 48.
Define angle of contact.
Answer:
The angle of contact for a liquid-solid pair (a liquid in contact with a solid) is defined as the angle between the surface of the solid and the tangent drawn to the free surface of the liquid at the extreme edge of the liquid, as measured through the liquid.

Question 49.
Draw neat diagrams to show the angle of contact in the case of a liquid which
(i) completely wets
(ii) partially wets
(iii) does not wet the solid. State the characteristics of the angle of contact in each case, giving one example of each.
Answer:
Characteristics :
(1) For a liquid, which completely wets the solid, the angle of contact is zero.
For example, pure water completely wets clean glass. Therefore, the angle of contact at the water glass interface is zero [from figure (a)].

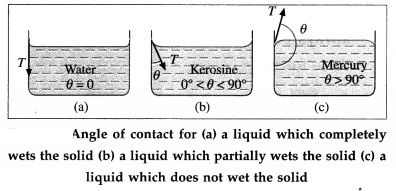
(2) For a liquid which partially wets the solid, the angle of contact is an acute angle. For example, kerosine partially wets glass, so that the angle of contact is an acute angle at the kerosine glass interface [from figure (b)].
(3) For a liquid which does not wet the solid, the angle of contact is an obtuse angle. For example, mercury does not wet glass at all, so that the angle of contact is an obtuse angle at the mercury-glass interface [from figure (c)].
(4) The angle of contact for a given liquid solid pair is constant at a given temperature, provided the liquid is pure and the surface of the solid is clean.

Question 50.
State any two characteristics of angle of contact.
Answer:
Characteristics of angle of contact:

1. It depends upon the nature of the liquid and solid in contact, and is constant for a given liquid-solid pair, other factors remaining unchanged.
2. It depends upon the medium (gas) above the free surface of the liquid.
3. It is independent of the inclination of the solid to the liquid surface.
4. It changes with surface tension and, hence, with the temperature and purity of the liquid.

Question 51.
Explain why the free surface of some liquids in contact with a solid is not horizontal.
OR
Explain the formation of concave and covex surface of a liquid on the basis of molecular theory.
Answer:
For a molecule in the liquid surface which is in contact with a solid, the forces on it are largely the solid-liquid adhesive force \(\vec{F}_{\mathrm{A}}=\overrightarrow{P A}\) and the liquid- liquid cohesive force \(\vec{F}_{\mathrm{C}}=\overrightarrow{P C} \vec{F}_{A}\) is normal to the solid surface and \(\vec{F}_{\mathrm{C}}\) is at 45° with the horizontal, from figure (a). The free surface of a liquid at rest is always perpendicular to the resultant \(\vec{F}_{\mathrm{R}}=\overrightarrow{P R}\) of these forces.

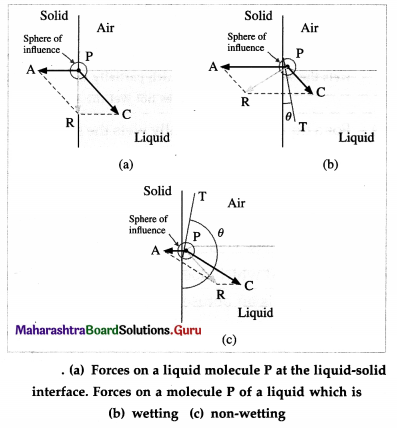
If F_C = \(\sqrt{2} F_{\mathrm{A}}, \vec{F}_{\mathrm{R}}\) is along the solid surface, the contact angle is 90° and the liquid surface is horizontal at the edge where it meets the solid, as in figure (a). In general this is not so, and the liquid surface is not horizontal at the edge.

For a liquid which completely wets the solid (e.g., pure water in contact with clean glass), F_C << F_A. For a liquid which partially wets the solid (e.g., kerosine . or impure water in contact with glass), F_C < \(\sqrt{2} F_{\mathrm{A}}\). If F_C << F_A or if F_C < \(\sqrt{2} F_{\mathrm{A}}\), the contact angle is correspondingly zero or acute and the liquid surface curves up and acquires a concave shape until the tangent PT is tangent to \(\vec{F}_{\mathrm{R}}\) fron figure (b).

If F_C > \(\sqrt{2} F_{\mathrm{A}}\), the contact angle is obtuse and the liquid surface curves down and acquires a convex shape until the tangent PT is tangent to \(\vec{F}_{\mathrm{R}}\), from figure (c).

Question 52.
State the conditions for concavity and convexity of a liquid surface where it is in contact with a solid.
Answer:
For a molecule in the liquid surface which is in contact with a solid, the forces on it are largely
(i) the solid-liquid adhesive force \(\vec{F}_{\mathrm{A}}\) normal and into the solid surface and
(ii) the liquid-liquid cohesive force \(\vec{F}_{\mathrm{C}}\) at nearly 45° with the horizontal.

If F_C << F_A or if F_C < \(\sqrt {2}\)F_A , the contact angle is correspondingly zero or acute and the liquid sur-face is concave with the solid.

If F_C > \(\sqrt {2}\)F_A, the contact angle is obtuse and the liquid surface curves down, i.e., convex, with the Solid.

Question 53.
Draw neat labelled diagrams to show angle of contact between (a) pure water and clean glass . (b) mercury and clean glass.
Answer:

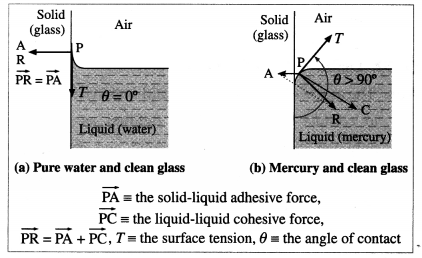
Question 54.
Explain the shape of a liquid drop on a solid surface in terms of interfacial tensions.
OR
Account for the angle of contact in terms of interfacial tensions.
OR
Draw diagram showing force due to surface tension at the liquid-solid, air-solid, air-liquid interface, in case of (i) a drop of mercury on a plane solid surface and (ii) a drop of water on a plane solid surface. Discuss the variation of angle of contact.
Answer:
A liquid surface, in general, is curved where it meets a solid. The angle between the solid surface and the tangent to the liquid surface at the extreme edge of the liquid, as measured through the liquid, is called the angle of contact.

Above figure shows the interfacial tensions that act in equilibrium at the common point of the liquid, solid and gas (air + vapour).
T₁ = the liquid-solid interfacial tension
T₂ = the solid-gas interfacial tension
T₃ = the liquid-gas interfacial tension
θ = the angle of contact for the liquid-solid pair is the angle between T₁ and T₃
The equilibrium force equation (along the solid surface) is
T₃ Cos θ + T₁ – T₂ = 0
∴ cos θ = \(\frac{T_{2}-T_{1}}{T_{3}}\) …………… (1)

Case (1) : If T₂ > T₁, cos θ is positive and contact angle θ < 900, so that the liquid wets the surface.
Case (2) : If T₂ < T₁, cos θ is negative and θ is obtuse, so that the liquid is non-wetting.
Case (3): If T₂ – T₁ T₃, cos θ = 1 and θ ≅ 0°.
Case (4) : If T₂ – T₁ ≅ T₃, cos θ will be greater than 1 which is impossible, so that there will be no equilibrium and the liquid will spread over the solid surface.

Question 55.
State the expression for the angle of contact in terms of interfacial tensions?
Answer:
cos θ = \(\frac{T_{2}-T_{1}}{T_{3}}\), where θ is the angle of contact for a liquid-solid pair, T₁ is the liquid-solid interfacial tension, T₂ is the solid-gas (air + vapour) inter-facial tension and T₃ is the liquid-gas interfacial tension.

Question 56.
In terms of interfacial tension, when is the angle of contact acute ?
Answer:
The angle of contact is acute when the solid-gas (air + vapour) interfacial tension is greater than the liquid-solid interfacial tension.

Question 57.
In terms of interfacial tensions, when is the angle of contact obtuse ?
Answer:
The angle of contact is obtuse when the solid-gas (air + vapour) interfacial tension is less than the liquid-solid interfacial tension.

Question 58.
State the factors affecting a liquid-solid angle of contact.
Answer:
Factors affecting a liquid-solid angle of contact:

1. the nature of the liquid and the solid in contact,
2. impurities in the liquid,
3. temperature of the liquid.

Question 59.
Explain the effect of impurity on the angle of contact (or surface tension of a liquid).
Answer:
Effect of impurity :
(i) The angle of contact or the surface tension of a liquid increases with dissolved impurities like common salt. For dissolved impurities, the angle of contact (or surface tension) increases linearly with the concentration of the dissolved materials.

(ii) It decreases with sparingly soluble substances like phenol or alcohol. A detergent is a surfactant whose molecules have hydrophobic and hydrophilic ends; the hydrophobic ends decrease the surface tension of water. With reduced surface tension, the water can penetrate deep into the fibres of a cloth and remove stubborn stains.

(iii) It decreases with insoluble surface impurities like oil, grease or dust. For example, mercury surface contaminated with dust does not form perfect spherical droplets till the dust is removed.

Question 60.
Explain the effect of temperature on the angle of contact (or surface tension of a liquid).
Answer:
Effect of temperature : The surface tension of a liquid decreases with increasing temperature of the liquid. For small temperature differences, the decrease in surface tension is nearly directly proportional to the temperature rise.

If T and T₀ are the surface tensions of a liquid at temperatures θ and 0 °C, respectively, then T = T₀(1 – αθ) where α is a constant for a given liquid. The surface tension of a liquid becomes zero at its critical temperature. The surface tension increases with increasing temperature only in case of molten copper and molten cadmium.

Question 61.
Why cold wash is recommended for new cotton fabrics while hot wash for removing stains?
Answer:
Cold wash is recommended for new/coloured cotton fabrics. Cold water, due to its higher surface tension, does not penetrate deep into the fibres and thus does not fade the colours. Hot water, because of its lower surface tension, can penetrate deep into fabric fibres and remove tough stains.

Question 62.
Explain in brief the pressure difference across a curved liquid surface.
Answer:
Every molecule lying within the surface film of a static liquid is pulled tangentially by forces due to surface tension. The direction of their resultant, \(\vec{F}_{\mathrm{T}}\), on a molecule depends upon the shape of that liquid surface and decides the cohesion pressure at a point just below the liquid surface.

Consider two molecules, A and B, respectively just above and below the free surface of a liquid. So, the level difference between them is negligibly small and the atmospheric pressure on both is the same, p₀, Let \(\vec{F}_{\mathrm{atm}}\) be the downward force on A and B due to the atmospheric pressure.

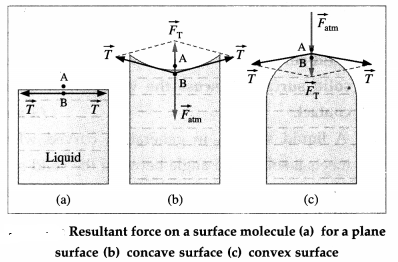
If the free surface of a liquid is horizontal, the resultant force \(\vec{F}_{\mathrm{T}}\) on molecule B is zero, from figure (a). Then, the cohesion pressure is negligible and the net force on A and B is \(\vec{F}_{\mathrm{atm}}\). The pressure difference on the two sides of the liquid surface is zero.

If the free surface of a liquid is concave, the resultant force \(\vec{F}_{\mathrm{T}}\) on molecule B is outwards (away from the liquid), from figure (b), opposite to \(\vec{F}_{\mathrm{atm}}\). Then, the net force on B is less than \(\vec{F}_{\mathrm{atm}}\) and the cohesion pressure is decreased. The pressure above the concave liquid surface is greater than that just below the liquid surface.

If the free surface of a liquid is convex, the resultant force \(\vec{F}_{\mathrm{T}}\) on molecule B acts inwards (into the liquid), from figure (c), in the direction of \(\vec{F}_{\mathrm{atm}}\). Then, the net force on B is greater than \(\vec{F}_{\mathrm{atm}}\) and the cohesion pressure is increased. The pressure below the convex liquid surface is greater than that just above the liquid surface.

Question 63.
Derive an expression for the excess pressure inside a soap bubble.
OR
Derive Laplace’s law for spherical membrane of a bubble due to surface tension.
Answer:
Consider a small, spherical, thin-filmed soap bubble with a radius R. Let the pressure outside the drop be Po and that inside be p. A soap bubble in air is like a spherical shell and has two gas-liquid interfaces. Hence, the surface area of the bubble is
A = 8πR² ………. (1)
Hence, with a hypothetical increase in radius by an infinitesimal amount dR, the differential increase in surface area and surface energy would be
dA = 16πR ∙ dR and
dW = T ∙ dA = 16πTRdR ………….. (2)
We assume that dR is so small that the pressure inside remains the same, equal to p. All parts of the surface of the bubble experiences an outward force per unit area equal to p – p_o. Therefore, the work done by this outward pressure-developed force against the surface tension force during the increase in radius dR is
dW = (excess pressure × surface area) ∙ dR
= (p – p_o) × 4πR² ∙ dR ………. (3)
From Eqs. (2) and (3),
(p – p_o) × 4πR² ∙ dR = 16πTRdR
∴ p – p_o = \(\frac{4 T}{R}\) …………… (4)
which is the required expression.

[Note : The excess pressure inside a drop or bubble is inversely proportional to its radius : the smaller the bubble radius, the greater the pressure difference across its wall.]

Question 64.
What is the excess of pressure inside a soap bubble of radius 3 cm if the surface tension of the soap solution is 30 dyn/cm ?
Answer:
Excess of pressure, p – p_o = \(\frac{4 T}{R}=\frac{4 \times 30}{3}\)
= 40 dyn/cm²

Question 65.
Two soap bubbles of the same soap solution have radii 3 cm and 1.5 cm. If the excess pressure inside the bigger bubble is 40 dyn/cm², what is the excess pressure inside the smaller bubble ?
Answer:
Excess pressure ∝ T/R. In this case, the surface tension is the same in the two cases. Hence, the excess pressure inside the smaller bubble will be 80 dyn/cm².

Question 66.
Explain : In the absence of gravity or other external forces, a liquid drop assumes a spherical shape.
Answer:
A spherical shape has the minimum surface area-to-volume ratio of all geometric forms. If any . external force distorts the sphere, molecules must be brought from the interior to the surface in order to provide for the increased surface area. This process requires work to be done in order to raise the potential energy of a molecule. The change in free surface energy is equal to the net work done to alter the surface area of the liquid.

However, spontaneous processes are associated with a decrease in free energy. Hence, in the absence of external forces, a liquid drop will spontaneously assume a spherical shape in order to minimize its exposed surface area and thereby its free surface energy.

[ Note : The spontaneous coalescence of two similar liquid droplets into one large drop when brought into contact is a dramatic demonstration of the decrease in free surface energy brought about by the decrease in total surface area by the formation of a single larger drop.]

Question 67.
A small air bubble of radius r in water is at a depth h below the water surface. If p₀ is the atmospheric pressure, ρ is the density of water and T is the surface tension of water, what is the pressure inside the bubble?
Answer:
The absolute pressure within the liquid at a depth h is p = p₀ + ρgh.
Since the excess pressure inside a bubble is \(\frac{2 T}{R}\), the pressure inside the bubble is
P_in = p + \(\frac{2 T}{R}\) = p₀ + ρgh + \(\frac{2 T}{R}\)

68. Solve the following

Question 1.
What is the excess pressure (in atm) inside a soap bubble with a radius of 1.5 cm and surface tension of 3 × 10^-2 N/m? [1 atm = 101.3 kPa]
Solution:
Data : R = 1.5 × 10^-2 m, T = 3 × 10^-2 N/m,
1 atm = 1.013 × 10⁵ Pa
The excess pressure inside a soap bubble is
p – p₀ = \(\frac{4 T}{R}\)
= \(\frac{4 \times 3 \times 10^{-2}}{1.5 \times 10^{-2}}\) = 8Pa
= \(\frac{8}{1.013 \times 10^{5}}\) atm = 7.897 × 10^-5 atm

Question 2.
A raindrop of diameter 4 mm is about to fall on the ground. Calculate the pressure inside the rain drop. [Surface tension of water T = 0.072 N/m, atmospheric pressure = 1.013 × 10⁵ N/m²)
Solution:
Data : D = 4 × 10^-3 m, T = 0.072N/m,
p₀ = 1013 × 10⁵ N/m²
R = \(\frac{D}{2}\) = 2 × 10^-3 m
The excess pressure inside the raindrop is
p – p₀ = \(\frac{2 I}{R}=\frac{2(0.072)}{2 \times 10^{-3}}\) = 72 N/m²
∴ p = 101300 + 72 = 101372 N/m²

Question 3.
What should be the diameter of a soap bubble such that the excess pressure inside it is 51.2 Pa? [Surface tension of soap solution = 3.2 × 10^-2 N/m]
Solution:
Data : p – p₀ = 51.2 Pa, T = 3.2 × 10^-2 N/m
Forasoapbubb1e, p – p₀ = \(\frac{4T}{R}\)
∴ The radius of the soap bubble should be
R = \(\frac{4 T}{p-p_{0}}=\frac{4 \times 3.2 \times 10^{-2}}{51.2}\) = 2.5 × 10^-3 m = 2.5 mm
∴ the diameter of the soap bubble should be 2 × 2.5 = 5 mm.

Question 4.
The lower end of a capillary tube of diameter 1 mm is dipped 10 cm below the water surface in a beaker. What pressure is required to blow a hemispherical air bubble at the lower end of the tube? Present your answer rounded off to 4 significant figures. [Surface tension = 0.072 N/m, density = 10³ kg/m³, atmospheric pressure = 101.3 kPa, g = 9.8 m/s²]
Solution:
Data : r = 0.5 mm = 5 × 10^-4 m, d = 10 cm = 0.1 m,
T = 0.072 N/m, p = 10³ kg/m³, g = 9.8 m/s²,
P = 1 atm = 1.013 × 10⁵ Pa
The pressure outside the bubble at the depth d is
p₀ = P + dρg
= 1.013 × 10⁵ +0.1 × 10³ × 9.8
= (1.013 + 0.0098) × 10⁵ = 1.0228 × 10⁵ Pa
Since a bubble within water has only one, gas-liquid interface, the excess pressure inside the bubble is
p – p₀ = \(\frac{2 T}{r}=\frac{2 \times 0.072}{5 \times 10^{-4}}\) = 0.0288 × 10⁴ Pa
= 0.00288 × 10⁵ Pa
∴ P = (1.0228 + 0.00288) × 10⁵ = 1.02568 × 10⁵ Pa
The pressure inside the air bubble is 1.026 × 10⁵ Pa (or 102.6 kPa), rounded off to four significant figures.

Question 5.
There is an air bubble of radius 1.0 mm in a liquid of surface tension 0.072 N/m and density 10³ kg/m³. The bubble is at a depth of 10 cm below the free surface of the liquid. By what amount is the pressure inside the bubble greater than the’ atmospheric pressure?
Solution:
Data : R = 10^-3 m, T = 0.072 N/m, ρ = 10³ kg/m³, h = 0.1 m
Let the atmospheric pressure be p0. Then, the absolute pressure within the liquid at a depth h is
p = p₀ + ρgh
Hence, the pressure inside the bubble is
p_in = p₀ + \(\frac{2 T}{R}\) = p₀ + ρgh + \(\frac{2 T}{R}\)
The excess pressure inside the bubble over the atmospheric pressure is
p_in – p₀ = ρgh + \(\frac{2 T}{R}\)
= (10³) (9.8) (0.1) + \(\frac{2(0.072)}{10^{-3}}\)
= 980 + 144 = 1124 Pa

Question 6.
Two soap bubbles A and B, of radii 2 cm and 4 cm, respectively, are in a closed chamber where air pressure is maintained at 8 N/m ². If n_A and n_B are the number of moles of air in bubbles A and B, respectively, then find the ratio n_B : n_A. [Surface tension of soap solution = 0.04 N/m. Ignore the effect of gravity.]
Solution:
Data : R_A = 0.02 m, R_B = 0.04 m, p₀ = 8 N/m², T = 0.04 N/m

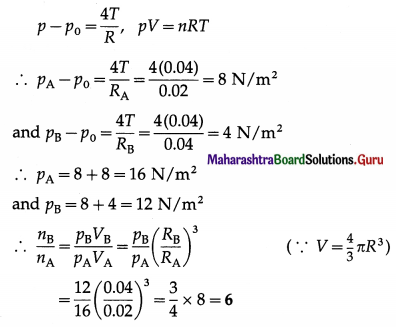
This is the required ratio.

Question 69.
What is capillary? What is capillarity or capillary action?
Answer:
(1) A tube of narrow bore (i.e. very small diameter) is called a capillary tube. The word capillary is derived from the Latin capillus meaning hair, capillaris in Latin means ‘like a hair’.

(2) If a capillary tube is just partially immersed in a wetting liquid the liquid rises in the capillary tube. This is called capillary rise.

If a capillary tube is just partially immersed in a non-wetting liquid, the liquid falls in the capillary tube. This is called capillary depression.

The rise of a wetting liquid and fall of a non-wetting liquid in a capillary tube is called capillarity.

Question 70.
State any four applications of capillarity.
Answer:
Applications of capillarity:

1. A blotting paper or a cotton cloth absorbs water; ink by capillary action.
2. Oil rises up the wick of an oil lamp and sap rises up xylem tissues of a tree by capillarity.
3. Ground water rises to the open surface through the capillaries formed in the soil. In summer, the farmers plough their fields to break these capillaries and prevent excessive evaporation.
4. Water rises up the crevices in rocks by capillary action. Expansion and contraction of this water due to daily and seasonal temperature variations cause the rocks to crumble.

[Note: The rise of sap is due to the combined action of capilarity and transpiration. The transpiration pull, is considered to be the major driving force for water transport throughout a plant.]

Question 71.
Two capillary tubes have radii in the ratio 1: 2. If they are dipped in the same liquid, what will be the ratio of capillary rise in the two tubes ?
Answer:
T = \(\frac{h r \rho g}{2 \cos \theta}\)
In this case, hr = constant
∴ h₁ : h₂ = r₂ : r₁ = 2 : 1.

Question 72.
The radii of two columns of a U-tube are r₁ and r₂. When a liquid of density ρ and angle of contact θ = 0° is filled in it, the level difference of the liquid in the two columns is h. Find the surface tension of the liquid.
Answer:
Capillary rise, h = \(\frac{2 T \cos \theta}{r \rho g}\), where θ is the angle of contact.
Assuming the two columns of the U-tube to be sufficiently thin,

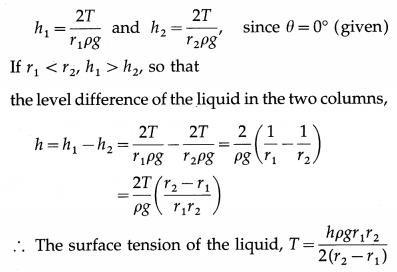
73. Solve the following

Question 1.
A liquid of density 900 kg/m³ rises to a height of 9 mm in a capillary tube of 2.4 mm diameter. If the angle of contact is 25°, find the surface tension of the liquid.
Solution:
Data : ρ = 900 kg/m³, h = 9 mm = 9 × 10^-3 m,
θ = 25°, g = 9.8 m/s²
r = \(\frac{1}{2}\) × diameter = \(\frac{2.4}{2}\) = 1.2 mm = 1.2 × 10^-3 m
cos θ = cos 25° = 0.9063
The surface tension of the liquid,
T = \(\frac{r h \rho g}{2 \cos \theta}\)
= \(\frac{1.2 \times 10^{-3} \times 9 \times 10^{-3} \times 900 \times 9.8}{2 \times 0.9063}\)
= 5.257 × 10^-2 N/m

Question 2.
A capilary tube of uniform bore is dipped vertically in water which rises by 7 cm in the tube. Find the radius of the capillary tube if the surface tension of water is 70 dyn/cm. [g = 980 cm/s²]
Solution:
Data : h = 7 cm, T = 70 dyn/cm, g = 980 cm/s², ρ = 1 g/cm³ and θ = 0° (for water)
∴ cos θ = 1 .
Surface tension, T = \(\frac{r h \rho g}{2 \cos \theta}\)
∴ The radius of the capillary tube,
r = \(\frac{2 T \cos \theta}{h \rho g}\)
= \(\frac{2 \times 70 \times 1}{7 \times 1 \times 980}\) = 0.02041 cm

Question 3.
A liquid rises to a height of 9 cm in a glass capillary tube of radius 0.02 cm. What will be the height of the liquid column in a glass capillary tube of radius 0.03 cm ?
Solution:
Data : h₁ = 9 cm, r₁ = 0.02 cm, r₂ = 0.03 cm
For the first capillary, T = \(\frac{r_{1} h_{1} \rho g}{2 \cos \theta}\)
For the second capillary, T = \(\frac{r_{2} h_{2} \rho g}{2 \cos \theta}\)
∴ \(\frac{r_{1} h_{1} \rho g}{2 \cos \theta}=\frac{r_{2} h_{2} \rho g}{2 \cos \theta}\)
∴ r₁h₁ = r₂h₂
The height of the liquid column in the second capillary,
h₂ = \(\frac{r_{1} h_{1}}{r_{2}}=\frac{0.02 \times 9}{0.03}\) = 6 cm

Question 4.
Water rises to a height of 5 cm in a certain capillary tube. In the same capillary tube, mercury is depressed by 2.02 cm. Compare the surface tensions of water and mercury.
[Density of water = 1000 kg/m³, density of mercury = 13600 kg/m³, angle of contact for water = 0°, angle of contact for mercury = 148°]
Solution:
Let T_w, θ_w, h_w and ρ_w be the surface
tension, angle of contact, capillary rise and density of water respectively. Let T_m, θ_m, h_m and ρ_m be the corresponding quantities for mercury. The radius (r) of the capillary is the same in both cases.

Data : h_w = 5 cm = 5 × 10^-2 m, θ_w = 0°, ρ_w = 1000 kg/m³, h_m = -2.02 cm
= -2.02 × 10^-2 m,
ρ_m = 13600 kg/m³, θ_m= 148°
[Note : h_m is taken to be negative because for mercury there is capillary depression.]
cos θ_w = cos 0° = 1
cos θ_m = cos 148° = – cos 32° = -0.8480

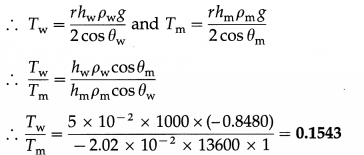
Question 5.
When a glass capillary tube of radius 0.4 mm is dipped into mercury, the level of mercury inside the capillary stands 1.50 cm lower than that outside. Calculate the surface tension of mercury. [Angle of contact of mercury with glass = 148 °, density of mercury = 13600 kg/m³]
Solution:
Data : r = 0.4 mm = 4 × 10^-4 m, h = -1.50 cm = – 1.50 × 10^-2 m,
ρ = 13.6 × 10³ kg/m³, g = 9.8 m/s², θ = 148°
cos θ = cos 148° = – cos 32 ° = – 0.8480
The surface tension of mercury is
T = \(\frac{r h \rho g}{2 \cos \theta}\)
= \(\frac{\left(4 \times 10^{-4}\right)\left(-1.50 \times 10^{-2}\right)\left(13.6 \times 10^{3}\right)(9.8)}{2(-0.8480)}\)
= 0.4715 N/m

Question 6.
The tube of a mercury barometer is 1 cm in diameter. What correction due to capillarity is to be applied to the barometric reading if the surface tension of mercury is 435.5 dyn/cm and the angle of contact of mercury with glass is 140° ? [Density of mercury = 13600 kg/m³]
Solution:
Data : d = 1 cm, T = 435.5 dyn/cm, θ = 140°, ρ = 13660 kg/m³ = 13.66 g/cm³,
g = 9.8 m/s² = 980 cm/s²

∴ The correction due to capillarity = -0.1001 cm

Question 7.
Calculate the density of paraffin oil, if within a glass capillary of diameter 0.25 mm dipped in paraffin oil of surface tension 0.0245 N/m, the oil rises to a height of 4 cm. [Angle of contact of paraffin oil with glass = 28°, acceleration due to gravity = 9.8 m/s²]
Solution :
Data : d = 0.25 mm, T = 0.0245 N/m, h = 4 cm = 4 × 10^-2 m, θ = 28°, g = 9.8 m/s²

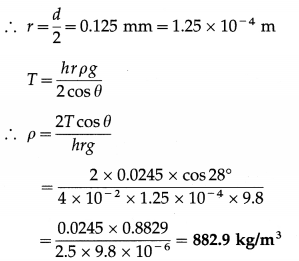
This gives the density of paraffan oil.

Question 8.
A capillary tube of radius r can support a liquid column of weight 6.284 × 10^-4 N. Calculate the radius of the capillary if the surface tension of the liquid is 4 × 10^-2 N/m.
Solution:
Data : mg = 6.284 × 10^-4 N, T = 4 × 10^-2 N/m
Net upward force = weight of liquid column
∴ 2πrT cos θ = mg
Assuming the angle of contact, θ = 0° (∵ data not given), the radius of the capillary is
r = \(\frac{m g}{2 \pi T}=\frac{6.284 \times 10^{-4}}{2(3.142)\left(4 \times 10^{-2}\right)}\) = 2.5 × 10^-3 m

Question 9.
Two vertical glass plates are held parallel 0.5 mm apart, dipped in water. If the surface tension of water is 70 dyn/cm , calculate the height to which water rises between the two plates.
Solution:
Data : x = 0.5 mm = 5 × 10^-4 m, T = 0.07 N/m, ρ(water) = 10³ kg/m³

Let the width of each glass plate be b and the height to which the water rises between the plates be h.

Then, the upward force on the water between the plates due to surface tension = 2Tb cos θ

where θ is the angle of contact of water with glass. The weight of the water between the plates = mg = (bxhρ) = g

where x is the separation between the plates and p is the density of water.
Equating, (bxhρ)g = 2Tb cosθ
∴ The height to which water rises between the two plates,
h = \(\frac{2 T \cos \theta}{x \rho g}=\frac{2\left(7 \times 10^{-2}\right)(1)}{\left(5 \times 10^{-4}\right)\left(10^{3}\right)(9.8)}=\frac{0.2}{7}\)
= 0.02857 m = 2.857 cm

Question 10.
A glass capillary of radius 0.4 mm is inclined at 60° with the vertical in water. Find the length of water column in the capillary tube. [Surface tension of water = 7 × 10^-2 N/m]
Solution:
Data : r = 4 × 10^-4 m, Φ = 60°, T = 7 × 10^-2 N/m
Let h be the capillary rise when the capillary tube is immersed vertically in water. Let l be the length of the water column in the capillary tube above that of the outside level.

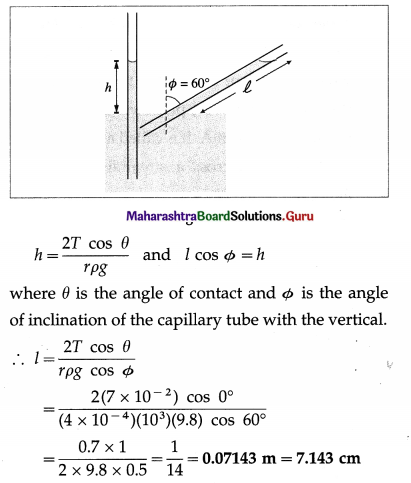
Question 74.
What is hydrodynamics?
Answer:
Hydrodynamics is the branch of physics that deals with fluid dynamics, i.e., the study of fluids in motion. Since the most basic fluid motion is highly complex, we consider only ideal fluids-non-viscous and incompressible, i.e., fluids whose internal friction is negligible and density is constant throughout.

Question 75
What is meant by a steady flow ?
Answer:
When a liquid flows slowly over a surface or through a pipe such that its velocity or pressure at any point within the fluid is constant, it is said to be in steady flow.

Question 76.
Explain a streamline and streamline flow.
Answer:
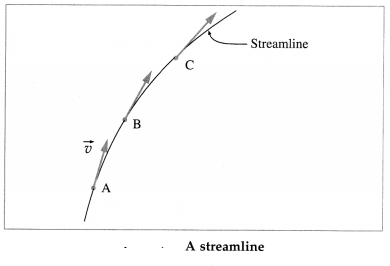
Streamline : Consider point A, from figure, within a fluid. The velocity \(\vec{v}\) at A does not change with time. Hence, every particle passes point A with the same speed and in the same direction. The same is true about the other points such as B and C. A curve which is tangent or parallel to the velocity of the fluid particles at every point will be the path of every particle arriving at A. It is called a streamline. A fluid particle cannot cross a streamline but only flow along it.

Streamline flow : When a liquid flows slowly over a surface or through a pipe with a velocity less than a certain critical velocity, the motion of its molecules is orderly. All molecules passing a given point proceed with the same velocity. This kind of fluid motion is called streamline or steady flow.

Question 77.
Explain a flow tube.
Answer:
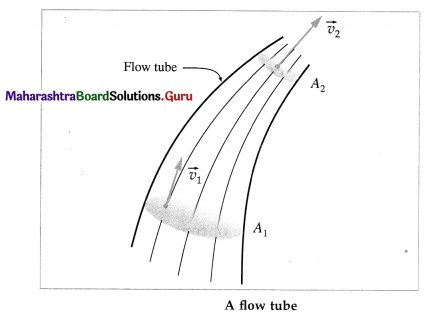
A bundle of adjacent streamlines form a tube of flow or flow tube through which the fluid is flowing. In a flow tube, where the streamlines are close together the velocity is high, and where they are widely separated, the fluid is moving slowly. No fluid can cross the boundary of a tube of flow.

Question 78.
Explain turbulent flow.
Answer:
Turbulent flow or turbulence is a non-steady fluid flow in which streamlines and flowtubes change continuously. It has two main causes. First, any obstruction or sharp edge, such as in a tap, creates

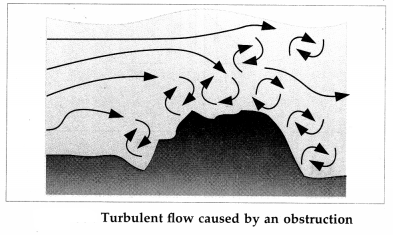
turbulence by imparting velocities perpendicular to the flow. Second, if the speed with which a fluid moves relative to a solid body is increased beyond a certain critical velocity the flow becomes unstable or one of extreme disorder. In both cases, the fluid particles still move in general towards the main direction as before. But now all sorts of secondary motions cause them to cross and recross the main direction continuously. The orderly streamlines break up into eddies or vertices and the result is turbulence. In a turbulent flow, regions of fluid move in irregular, colliding paths, resulting in mixing and swirling.

Question 79.
Distinguish between streamline flow and turbulent flow.
Answer:

Streamline flow	Turbulent flow
1. The steady flow of a fluid, with velocity less than certain critical velocity is called streamline or laminar flow.	1. A non-steady irregular fluid flow in which stream­lines and flowtubes change continuously with a veloc­ity greater than certain critical velocity.
2. In a streamline flow, the velocity of a fluid at a given point is always constant.	2. In a turbulent flow, the vel­ocity of a fluid at any point does not remain constant.
3. Streamlines do not change and never intersect.	3. Streamlines and flowtubes change continuously.
4. over a surface, and is in the form of coaxial cylinders through a pipe.	4. Fluid particles still move in general towards the main direction as before. But now all sorts of secondary motions cause eddies or vortices.

Question 80.
Explain the Reynolds number.
OR
What is Reynolds number?
Answer:
Osborne Reynolds found that if the free-stream velocity of a fluid increases when it moves relative to a solid body, a point is reached where the steady flow becomes turbulent. From experiments, he found that the transition from steady to turbulent flow depends on the value of the quantity \(\frac{v_{0} d}{\eta / \rho}\), where v₀ is the free-stream velocity, d is some characteristic dimension of the system, ρ the density of the fluid and η its coefficient of viscosity. For a sphere in a fluid stream, d is its diameter; for water in a pipe, d is the pipe diameter.

This dimensionless number, defined as
Re = \(\frac{v_{0} d \rho}{\eta}\) is called the Reynolds number.
In a system of particular geometry, transition from a steady to turbulent flow is given by a certain value of the Reynolds number called the critical Reynolds number. The free-stream velocity for this critical Reynolds number is called the critical velocity, v_critical = \(\frac{n R_{\mathrm{e}}}{\rho d}\). For a given system geometry, the free stream velocity beyond which a streamline flow becomes turbulent is called critical velocity.

Steady flow takes place for Re up to about 1000. For 1000 < Re < 2000, there is a transition region in which the flow is extremely sensitive to all sorts of small disturbances. For Re > 2000, the flow is completely turbulent.

[Notes : (1) See Q. 95 for “free-stream velocity”. (2) The dimensionless number is named after Osborne Reynolds (1842-1912), British physicist.]

Question 81.
Explain the term viscosity.
Answer:
Suppose a constant tangential force is applied to the surface of a liquid. Under this shearing force, the liquid begins to flow. The motion of a thin layer of the liquid at the surface, relative to a layer below, is opposed by fluid friction. Because of this internal fluid friction, horizontal layers of the liquid flow with varying velocities.

This also happens in a gas. When a solid surface is moved through a gas, a thin layer of the gas moves with the surface. But its motion relative to a layer away is opposed by fluid friction.

The resistance to relative motion between the adjacent layers of a fluid is known as viscosity.

It is a property of the fluid. The resistive force in fluid motion is called the viscous drag.

Question 82.
When a-liquid contained in a bucket is stirred and left alone, it comes to rest after some time. Why?
Answer:
This happens due to the internal friction (viscosity) and friction with the walls and bottom of the bucket.

Question 83.
What do you mean by viscous drag?
Answer:
When a fluid flows past a solid surface, or when a solid body moves through a fluid, there is always a force of fluid friction opposing the motion. This force of fluid friction is called the drag force or viscous drag.

Question 84.
What causes viscous drag in fluids?
Answer:
In liquids, the viscous drag is due to short range molecular cohesive forces while in gases it is due to collisions between fast moving molecules. For laminar flow in both liquids and gases, the viscous drag is proportional to the relative velocity between the layers, provided the relative velocity is small. For turbulent flow, the viscous drag increases rapidly and is proportional to some higher power of the relative velocity.

Question 85.
Define and explain velocity gradient in a steady flow.
Answer:
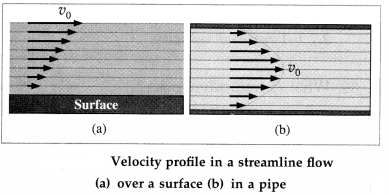
Definition : In a steady flow of a fluid past a solid surface, the rate at which the velocity changes with distance within a limiting distance from the surface is called the velocity gradient.

When a fluid flows past a surface with a low velocity, within a limiting distance from the surface, its velocity varies with the distance from the surface, from below figure. The layer in contact with the surface is at rest relative to the surface. Starting outwards from the surface, the next layer has an extremely small velocity; each successive layer has a slightly higher velocity than its inner neighbour, as shown. Finally, a layer is reached which has approximately the full, or free-stream, velocity v₀ of the fluid. The situation is reversed if a body is moving in a stationary fluid : the fluid velocity reduces as the distance of a layer from the body increases. Thus, the velocity in each layer increases with its distance from the surface.

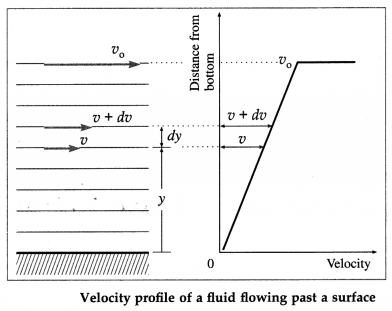
Consider the layer of thickness dy at y from the solid surface. Let v and v + dv be the velocities of the fluid at the base and upper edge of this layer. The change in velocity across the layer is dv. Therefore, the rate at which the velocity changes dv between the layers is \(\frac{d v}{d y}\). This is called the velocity gradient.

Question 86.
State and explain Newton’s law of viscosity.
Answer:
Newton’s law of viscosity: In a steady flow of a fluid past a solid surface, a velocity profile is set up such that the viscous drag per unit area on a layer is directly proportional to the velocity gradient.

When a fluid flows past a solid surface in a streamline flow or when a solid body moves through a fluid, the force of fluid friction opposing the motion is called the viscous drag. The magnitude of the viscous drag of a fluid is given by Newton’s law of viscosity.

If \(\frac{d v}{d y} \) is the velocity gradient, the viscous drag per unit area on a layer,
\(\frac{F}{A} \propto \frac{d v}{d y}\)
∴ \(\frac{F}{A}=\eta \frac{d v}{d y}\)
where the constant of proportionality, y, is called the coefficient of viscosity of the fluid.

Question 87.
Define coefficient of viscosity.
Answer:
Coefficient of viscosity : The coefficient of viscosity of a fluid is defined as the viscous drag per unit area acting on a fluid layer per unit velocity gradient established in a steady flow.

Question 88.
Find the dimensions of the coefficient of viscosity. State its SI and CGS units.
Answer:
By Newton’s law of viscosity,
\(\frac{F}{A}=\eta \frac{d v}{d y}\)
where \(\frac{F}{A}\) is the viscous drag per unit area, \(\frac{d v}{d y}\) is the velocity gradient and y is the coefficient of viscosity of the fluid. Rewriting the above equation as
η = \(\frac{(F / A)}{(d v / d y)}\)
[η] = \(\frac{\left[F A^{-1}\right]}{[d v / d y]}\) = [ML^-1T^-2][T¹] = [ML^-1T^-1]
SI unit: the pascal ∙ second (abbreviated Pa ∙ s), 1 Pa ∙ s = 1 N ∙ m^-2 ∙ s

CGS unit: dyne ∙ cm^-2 ∙ s, called the poise [symbol P, named after Jean Louis Marie Poiseuille (1799-1869), French physician].

[Note : The most commonly used submultiples are the millipascal-second (mPa ∙ s) and the centipoise (cP). 1 mPa ∙ s = 1 cP.]

Question 89.
Define the SI and CGS units of coefficient of viscosity.
Answer:
The SI unit of coefficient of viscosity is the pascal- second.

Definition : If a tangential force per unit area of one newton per square metre is required to maintain a difference in velocity of one metre per second between two parallel layers of a fluid in streamline flow separated by one metre, the coefficient of viscosity of the fluid is one pascal-second.

The CGS unit of coefficient of viscosity is the poise.

Definition : If a tangential force per unit area of one dyne per square centimetre is required to maintain a difference in velocity of one centimetre per second between two parallel layers of a fluid in streamline flow separated by one centimetre, the coefficient of viscosity of the fluid is one poise.

Question 90.
Find the conversion factor between the SI and CGS units of coefficient of viscosity using dimensional analysis.
Answer:
The dimensions of the coefficient of viscosity η are
[η] = [ML^-1T^-1]
The SI and CGS units of coefficient of viscosity are the pascal-second and poise, respectively.
1 Pa ∙ s = 1 N ∙ m^-2 ∙ s = 1 kg-m^-1 ∙ s^-1
1 P = 1 dyn ∙ cm^-2 ∙ s = l g-cm^-1 ∙ s^-1
Let 1 Pa ∙ s = xP
∴ 1[M₁L₁^-1T₁^-1] = x[M₂L₂^-1T₂^-1]
where subscripts 1 and 2 pertain to SI and CGS units.

Question 91.
State Stokes’ law. Derive Stokes’ law using dimensional analysis.
Answer:
Stokes’ law : If a fluid flows past a sphere or a sphere moves through a fluid, for small enough
∴ Viscous force = gravitational force-buoyant force
= mg – m_Lg
where m = mass of the sphere = \(\frac{4}{3} \pi r^{3} \rho\) and m_{L</sub. = mass of the liquid displaced = \(\frac{4}{3} \pi r^{3} \rho_{\mathrm{L}}\).}

At its terminal speed v_t, the magnitude of the viscous force by Stokes’ law is

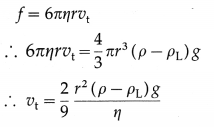
[Note : Theoretically, v → v_t as time t → ∞. In practice, if η is appreciable, then v tends to v_t in a very small time interval.]

[Data: g = 9.8 m/s²]

92. Solve the following

Question 1.
The relative velocity between two layers of a fluid, separately by 0.1 mm, is 2 cm/s. Calculate the velocity gradient.
Solution :
Data : dy = 0.1 mm = 10^-2 cm, dv = 2 cm/s
The velocity gradient, \(\frac{d v}{d y}=\frac{2 \mathrm{~cm} / \mathrm{s}}{10^{-2} \mathrm{~cm}}\) = 200 s^-1

Question 2.
Calculate the force required to move a flat glass plate of area of 10 cm² with a uniform velocity of 1 cm/s over the surface of a liquid 2 mm thick, if the coefficient of viscosity of the liquid is 2 Pa.s.
Solution :
Data : η = 2 Pa.s; A = 10 cm² = 10^-3 m²;
dv = 1 cm/s = 0.01 m/s; dy = 2 mm = 2 × 10^-3 m
According to Newton’s formula,
viscous force f = \(\eta A \frac{d v}{d y}\)
= \(\frac{(2 \mathrm{~Pa} \cdot \mathrm{s})\left(10^{-3} \mathrm{~m}^{2}\right)(0.01 \mathrm{~m} / \mathrm{s})}{2 \times 10^{-3} \mathrm{~m}}\)
= 0.01 N

This force retards the motion of the glass plate. Therefore, in order to keep the plate moving with a uniform velocity, an equal force must be exerted on the plate in the forward direction.

The required force to move the glass plate is 0.01 N

Question 3.
A metal plate of length 10 cm and breadth 5 cm is in contact with a layer of oil 1 mm thick. The horizontal force required to move it with a velocity of 4 cm/s along the surface of the oil is 0.32 N. Find the coefficient of viscosity of the oil. Also express it in poise.
Solution:

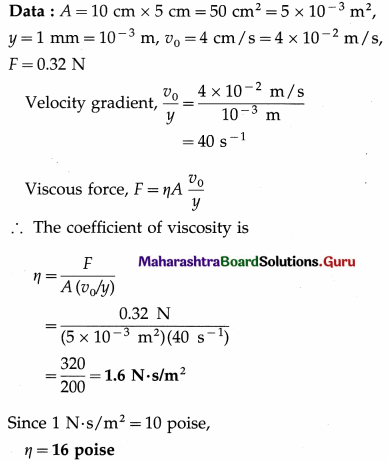
Question 4.
A spherical liquid drop of diameter 2 × 10^-4 m is falling with a constant velocity through air, under gravity. If the density of the liquid is 500 kg/m3 and the coefficient of viscosity of air is 2 × 10^-5 Pa.s, determine the terminal velocity of the drop and the viscous force acting on it. Ignore the density of air.
Solution :
Data : r = 1 × 10^-4 m, ρ = 500 kg/m³, ρ_air \(\ll \rho\) η = 2 × 10^-5 Pa.s

(i) The terminal velocity,

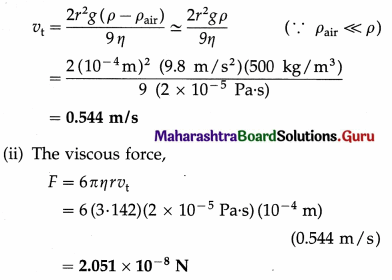
Question 5.
If the speed at which water flows through a long cylindrical pipe of radius 8 mm is 10 cm/s, find the Reynolds number. [Density of water = 1 g/cm³, coefficient of viscosity of water = 0.01 poise]
Solution :
Data : v₀ = 10 cm/s, ρ = 1 g/cm³, r = 8 mm
∴ d = 2r = 16 mm = 1.6 cm, η = 0.01 poise

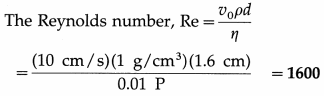
Question 93.
Define volume flow rate or volume flux. Explain how it is related to the velocity of fluid.
OR
What is the difference between flow rate and fluid velocity ? How are they related ?
Answer:
Definition : The volume of fluid passing by a given location per unit time through an area is called the volume flow rate, or simply flow rate, Q.Q = dV/dt

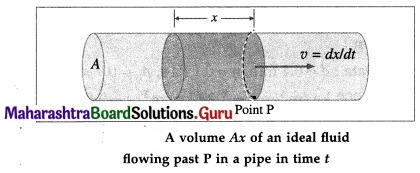
Consider an ideal fluid flowing with velocity v through a uniform flow tube of cross section A. If, as shown in Fig. 2.34, the shaded cylinder of fluid of length x and volume V flows past point P in time t,

which is the required relation.

Question 94.
State the SI unit for volume flow rate.
Answer:
The SI unit for volume flow rate is the cubic metre per second (m³/s).

[Note : Another common unit accepted in SI is the litre per minute (L/min). 1 L = 10^-3 m³ = 10³ cm³. An old non-SI unit from FPS system still used is the cubic feet per second (symbol, cusec).]

Question 95.
Define mass flow rate or mass flux. Explain how it is related to the velocity of fluid.
Answer:
Definition : The mass of fluid passing by a given point per unit time through an area is called the mass flow rate, dmldt.

Consider an ideal fluid of density ρ flowing with velocity v through a uniform flow tube of cross section A. If, as shown in Fig. 2.34, the shaded cylinder of fluid of length x and volume V flows past point P in time t,

which is the required relation.

Question 96.
Explain the continuity condition for a flow tube. Show that the flow speed is inversely proportional to the cross-sectional area of a flow tube.
Answer:
Consider a fluid in steady or streamline flow. The velocity of the fluid within a flow tube, while everywhere parallel to the tube, may change its magnitude. Suppose the velocity is \(\overrightarrow{v_{1}}\) at point P and \(\overrightarrow{v_{2}}\) at point Q. If A₁ and A₂ are the cross-sectional areas of the tube and ρ₁ and ρ₂ are the densities of the fluid at these two points, the mass of the fluid passing per unit time across A₁ is A₁ρ₁v₁ and that passing across A₂ is A₂ρ₂v₂. Since no fluid can enter or leave through the boundary of the tube, the conservation of mass requires
A₁ρ₁v₁ = A₂ρ₂v₂ …………. (1)

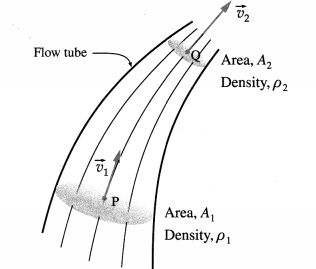
Equation (1) is called the equation of continuity of flow. It holds true for a compressible fluid, (like all gases) for which the density of the fluid may differ from point to point in a tube of flow. For an incompressible fluid (like all liquids), ρ₁ = ρ₂ and Eq. (1) takes the simpler form
A₁v₁ = A₂v₂ ………… (2)
∴ \(\frac{v_{1}}{v_{2}}=\frac{A_{2}}{A_{1}}\) …………… (3)
that is, the flow speed is inversely proportional to the cross-sectional area of a flow tube. Where the area is large, the speed of flow is small, and vice versa.

Equations (2) is the equation of continuity for an incompressible fluid for which density is constant throughout.

Question 97.
Explain why flow speed is greatest where streamlines are closest together.
Answer:
By the equation of continuity, the flow speed is inversely proportional to the area of cross section of a flow tube. Where the area of cross section is small, i. e., streamlines are close, the flow speed is large and vice versa.

Question 98.
You can squirt water a considerably greater distance by placing your thumb over the end of a garden hose. Explain.
Answer:
Placing one’s thumb over the end of a garden hose constricts the open end. By the continuity condition, the speed of water increases as it passes through the constriction. Hence, water squirts out and reaches a longer distance.

99. Solve the following

Question 1.
A liquid is flowing through a horizontal pipe of varying cross section. At a certain point, where the diameter of the pipe is 5 cm, the flow velocity is 0.25 m/s. What is the flow velocity where the diameter is 1 cm ?
Solution:
Data : d₁ = 5 cm, v₁ = 0.25 m/s, d₂ = 1 cm According to the equation of continuity of flow,
A₁ρ₁v₁ = A₂ρ₂v₂
where A₁ and ρ₁ are the cross-sectional area and density of the liquid where the flow velocity is v₁; A₂ and ρ₂ are the corresponding quantities where the flow velocity is v₂.
Assuming the liquid is incompressible,

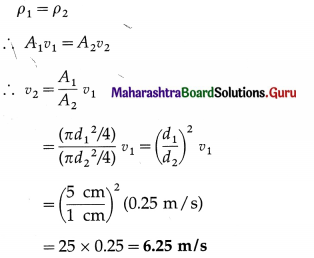
Question 100.
State Bernoulli’s principle.
Answer:
Where the velocity of an ideal fluid in streamline flow is high, the pressure is low, and where the velocity of a fluid is low, the pressure is high. OR At every point in the streamline flow of an ideal (i.e., nonviscous and incompressible) fluid, the sum of the pressure energy, kinetic energy and potential energy of a given mass of the fluid is constant at every point.

[Note : The above principle is equivalent to a statement of the law of conservation of mechanical energy as applied to fluid mechanics. It was published in 1738 by Daniel Bernoulli (1700 – 82), Swiss mathematician.]

Question 101.
Explain Bernoulli’s equation of fluid flow.
Answer:
Consider an ideal fluid incompressible and nonviscous of density ρ flowing along a flow tube of varying cross section. The system under consideration is the flow tube between points 1 and 2, and the Earth (below figure). From the continuity equation it follows that pressure and speed must be different in regions of different cross section. If the height also changes, there is an additional pressure difference.

The fluid enters the system at point 1 through a. surface of cross section A₁ at speed v₁. The point 1 lies at a height h₁, with respect to an arbitrary reference level y = 0, and the local pressure there is p₁. The fluid leaves the system at point 2 where the corresponding quantities are A₂, v₂, h₂ and p₂.

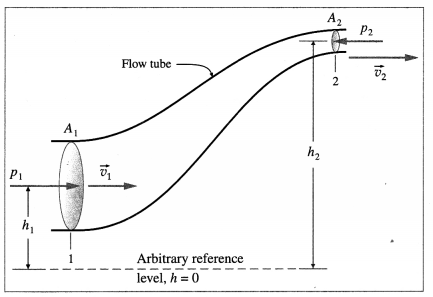
Consider a small fluid element, of volume ∆V and mass ∆m = ρ∆V, that enters at point 1 and leaves at point 2 during small time interval ∆t. In the absence of internal fluid friction, it can be shown that the work done on the fluid element by the surrounding fluid is
∆W= (p₁ – p₂)∆V
This is sometimes called the pressure energy. During At, the changes in the kinetic energy and potential energy are
∆KE = \(\frac{1}{2}\) ∆m (V₂² – V₁²) = \(\frac{1}{2}\) ρ∆V(v₂² – v₁²)
∆PE = ∆mg(h₂ – h₁) = ρ∆Vg(h₂ – h₁)
Since ∆W is the work done by a non-conservative force,
∆W = ∆KE + ∆PE
∴ (p₁ – p₂)∆V = \(\frac{1}{2}\) ρ∆V(v₂2² – v₁²) + ρ∆Vg(h₂ – h₁) ……….. (1)
∴ p₁ – p₂ = \(\frac{1}{2}\) ρ(v₂² – v₁²) + ρg(h₂ – h₁)
∴ p₁ + \(\frac{1}{2}\) ρv₁² + ρgh₁ = p₂ + \(\frac{1}{2}\) ρv₂² + ρgh₂
or p + \(\frac{1}{2}\) ρv² + ρgh = constant …………. (2)
This is known as Bernoulli’s equation.

[Notes : Equation (1) can be rewitten as
p₁∆V + \(\frac{1}{2}\)ρ∆Vv₁² + ρ∆Vgh₁
p₂∆V + \(\frac{1}{2}\) ρ∆v₂² + ρ∆Vgh₂
or p∆V + \(\frac{1}{2}\)ρ∆v² + ρ∆Vgy = constant ………. (3)
i.e., pressure energy + KE + PE = constant
Dividing Eq. (3) by ∆m = ρ∆V,
\(\frac{p}{\rho}\) + \(\frac{1}{2}\) v² + gy constant

i.e., pressure energy per unit mass + KE per unit mass + PE per unit mass = constant, which is Bernoulli’s principle. Note that in writing

∆W = ∆KE + ∆PE, we have assumed principle of conservation of energy.

Dimensionally, pressure is energy per unit volume. Both terms on the right side of Eq. (2) also have the same dimensions. Hence, the term (p₁ – p₂) is often referred to as pressure energy per unit volume or pressure head. The first term on the right, \(\frac{1}{2}\) p (v₂² – v₁²) head and the second term, pg(h₂ – h₁), is called the potential head.]

Question 102.
State the limitations of Bernoulli’s principle.
Answer:
Limitations: Bernoulli’s principle and his equation for fluid flow is valid only for
(1) an ideal fluid, i.e., one that is incompressible and nonviscous, so that the density remains constant throughout a flow tube and there is no viscous drag which results in energy dissipation or loss,
(2) streamline flow.

Question 103.
State the applications of Bernoulli’s principle.
Answer:
Applications :

1. Venturi meter : It is a horizontal constricted tube that is used to measure flow speed in a gas.
2. Atomizer : It is a hydraulic device used for spraying insecticide, paint, air perfume, etc.
3. Aerofoil : The aerofoil shape of the wings of an aircraft produces aerodynamic lift.
4. Bunsen’s burner : Bernoulli effect is used to admit air into the burner to produce an oxidising flame.

Question 104.
State the law of efflux. Derive an expression for the speed of efflux for a tank discharging through an opening at a depth h below the liquid surface. Hence or otherwise show that the speed of efflux for an open tank is \(\sqrt{2 g h}\).
Answer:
Law of efflux (Torricelli’s theorem) : The speed of efflux for an open tank through an orifice at a depth h below the liquid surface is equal to the speed acquired by a body falling freely through a vertical distance h.

Consider a tank with cross-sectional area A₁ holding a static liquid of density ρ. The tank discharges through an opening (of cross-sectional area A₂) in the side wall at a depth h below the surface of the liquid. The flow speed at which the liquid leaves the tank is called the speed of efflux.

The pressure at point 2 it is the atmospheric pressure p₀. Let the pressure of the air above the liquid at point 1 be ρ. We assume that the tank is large in cross section compared to the opening (A₁ >> A₂), so that the upper surface of the liquid will drop very slowly. That is, we may regard the liquid surface to be approximately at rest v₁ ≈ 0). Bernoulli’s equation, in usual notation, states
p₁ + \(\frac{1}{2}\) ρv₁² + ρgy₁ = p₂ + \(\frac{1}{2}\) ρv₂² + ρgy₂
Substituting p₁ = p, p₂ = p₀, v₁ ≈ 0 and (y₁ – y₂) = h,
v₂² = 2 \(\frac{p-p_{0}}{\rho}\) + 2gh
If the tank is open to the atmosphere, then p = p₀,
v₂ = \(\sqrt{2 g h}\).
which is the law of efflux.

[Note : For an open tank, the speed of the liquid, v₂, leaving a hole a distance h below the surface is equal to that acquired by an object falling freely through a vertical distance h.]

Question 105.
What is a Venturi tube? Explain the working of a Venturi tube.
OR
What is a Venturi meter? Briefly explain its use to determine the flow rate in a pipe.
Answer:
A Venturi meter is a horizontal constricted tube that is used to measure the flow speed through a pipeline. The constricted part of the tube is called the throat. Although a Venturi meter can be used for a gas, they are most commonly used for liquids. As the fluid passes through the throat, the higher speed results in lower pressure at point 2 than at point 1. This pressure difference is measured from the difference in height h of the liquid levels in the U-tube manometer containing a liquid of density ρ_m (from below figure). The following treatment is limited to an incompressible fluid.

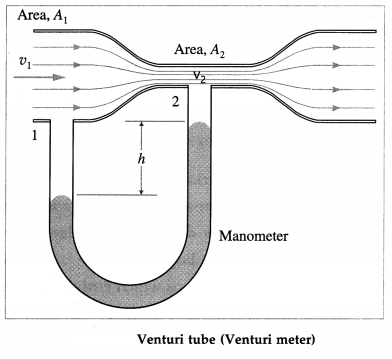
Let A₁ and A₂ be the cross-sectional areas at points 1 and 2, respectively. Let v₁ and v₂ be the corresponding flow speeds, p is the density of the fluid in the pipeline. By the equation of continuity,
v₁A₁ = v₂A₂ …………. (1)
Since the meter is assumed to be horizontal, from Bernoulli’s equation we get,
p₁ = \(\frac{1}{2}\) ρv₁² = p₂ + \(\frac{1}{2}\) ρv₂²
∴ p₁ = \(\frac{1}{2}\) ρv₁² = p₂ + \(\frac{1}{2}\) ρv₁² \(\left(\frac{A_{1}}{A_{2}}\right)^{2}\) [from Eq. (1)]
∴ p₁ – p₁ = \(\frac{1}{2}\) ρv₁² [\(\left(\frac{A_{1}}{A_{2}}\right)^{2}\) – 1] …………. (2)
The pressure difference is equal to pm gh, where h is the differences in liquid levels in the manometer. Then,

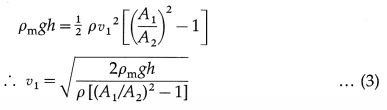
Equation (3) gives the flow speed of an incom-pressible fluid in the pipeline. The flow rates of practical interest are the mass and volume flow ‘ rates through the meter.
Volume flow rate = A₁v₁
and mass flow rate = density x volume flow rate = ρA₁v₁
[Note ; When a Venturi meter is used in a liquid pipeline, the pressure difference is measured from the difference in height h of the levels of the same liquid in the two vertical tubes, as shown in below figure. Then, the pressure difference is equal to ρgh.

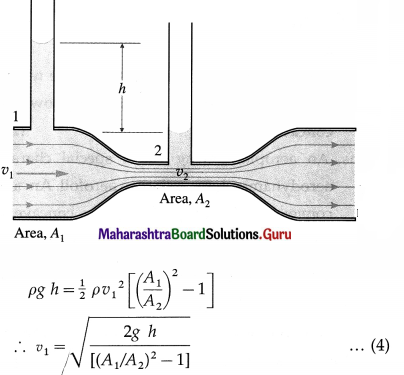
The flow meter is named after Giovanni Battista Venturi (1746-1822), Italian physicist.]

Question 106.
Explain aerodynamic lift on the wings of an aeroplane.
OR
Explain why the upper surface of the wings of an aeroplane is made convex and the lower surface concave.
Answer:
An aeroplane wing has a special characteristic aerodynamic shape called an aerofoil. An aerofoil is convex on the top and slightly concave on the bottom. Its leading edge is well rounded while the trailing edge is sharp. As an aeroplane moves through air, the aerofoil shape makes the air moving over the top and along the bottom of a wing in a certain way.

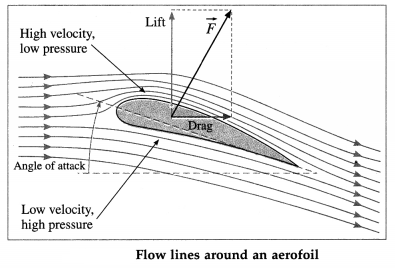
If the air over the top surface travels faster than the air below the wing, this decreases the air pressure above the wing. The air flowing below the wing moves almost in a straight line, so its speed and air pressure remain the same. The air under the wings therefore pushes upward more than the air on top of the wings pushes downward, thus producing an upward force \(\vec{F}\). It is the pressure difference that generates this force. The component of \(\vec{F}\) perpendicular to the direction of motion is called the aerodynamic lift or, simply, the lift. The component parallel to the direction of flight is the drag. The lift is the force that allows an aeroplane to get off the ground and stay in the air. For an aeroplane to stay in level flight, the lift is equal in magnitude and opposite in direction to the force of gravity.

[Note: For an airborne aeroplane to get to the ground, the direction of \(\vec{F}\) must be reversed. Then, the upper surface should be more concave than the lower surface such that air above the wing travels slower than the air below it, decreasing the air pressure below the wing. This is achieved by small flaps, called ailerons, attached at the trailing end of each wing.]

Question 107.
Explain the working of an atomizer.
OR
A perfume bottle or atomizer sprays a fluid that is in the bottle. How does the fluid rise up in the vertical tube in the bottle?
Answer:
An atomizer is a device which entraps or entrains liquid droplets in a flowing gas. Its working is based on Bernoulli’s principle. A squeeze bulb or a pump is used to create a jet of air over an open tube dipped into a liquid. By Bernoulli’s principle, the high-velocity air stream creates low pressure at the open end of the tube. This causes the liquid to rise in the tube. The liquid is then dispersed into a fine spray of droplets. This type of system is used in a perfume bottle, a paint sprayer, insect and perfume sprays and an automobile carburetor.

[Notes : A Bunsen burner uses an adjustable gas nozzle to entrain air into the gas stream for proper combustion. Aspirators, used as suction pumps, in dental and surgical situations (for draining body fluids) or for draining a flooded basement, is another similar applica-tion. Some chimney pipes have a T-shape, with a cross-piece on top that helps draw up gases whenever there is even a slight breeze.]

Question 108.
Roofs are sometimes blown off vertically during a tropical cyclone, and houses sometimes explode outward when hit by a tornado. Use Bernoulli’s principle to explain these phenomena.
Answer:
A cyclonic high wind blowing over a roof creates a low pressure above it, in accordance with Bernoulli’s principle. The pressure below the roof is equal to the atmospheric pressure which is now greater than the pressure above the roof. This pressure difference causes an aerodynamic lift that lifts the roof up. Once the roof is lifted up, it blows off in the direction of the wind.

Wind speeds in a tornado may be much higher and thus create much greater pressure differences. Sometimes, wooden houses hit by a tornado explode.

Question 109.
Describe what happens : (1) Hold the short edge of a paper strip (2″ × 6″) up to your lips-the strip slanting downward over your finger-and blow over the top of the strip. (2) Hold two strips of paper up to your lips, separated by your fingers and blow between the strips.
Answer:
(1) The air stream over the top surface travels faster than the air stream below the paper strip. This decreases the air pressure above the strip relative o that below. This produces an aerodynamic lift in accordance with Bernoulli’s principle and the paper strip will lift up.

(2) Air passing between the paper strips flows in a narrower channel and, in accordance with Be rnoulli’s principle, must increase its speed, causing the pressure between them to drop. This will pinch the two strips together.

110. Solve the following

Question 1.
Water is flowing through a horizontal pipe of varying cross section. At a certain point where the velocity is 0.12 m/s, the pressure of water is 0.010 m of mercury. What is the pressure at a point where the velocity is 0.24 m/s?
Solution:
Data : v₁ = 0.12 m/s, p₁ = 0.010 m of Hg, v₂ = 0.24 m/s, y₁ = y₂ (horizontal pipe), ρ_Hg = 13600 kg/m², ρ_w = 1000 kg/m³, g = 9.8 m/s²
p₁ = 0.010 m of Hg .
= (0.010 m) ρ_Hgg
= (0.010 m) (13600 kg/m³) (9.8 m/s²)
= 1332.8 Pa ≅ 1333 Pa
According to Bernoulli’s principle,

This gives the pressure of the water in the pipe where the flow velocity is 0.24 m / s.

Question 2.
A building receives its water supply through an undergound pipe 2 cm in diameter at an absolute pressure of 4 × 10⁵ Pa and flow velocity 4 m/s. The pipe leading to higher floors is 1.5 cm in diameter. Find the flow velocity and pressure at the floor inlet 10 m above.
Solution:
Data : d₁ = 3 cm, p₁ = 4 × 10⁵ Pa, v₁ = 4 m/s,
d₂ = 2 cm, h₂ – h₁ = 10 m
By continuity equation, the flow velocity at the higher floor inlet

Question 3.
Calculate the total energy per unit mass possessed by water at a point where the pressure is 0.1 × 10⁵ N/m², the velocity is 0.02 m/s and the height of the water level from the ground is 10 cm. Density of water = 1000 kg/m³.
Solution:
Data : p = 0.1 × 10⁵ N/m² = 10⁴ Pa, v = 0.02 m/s, y = 10 cm = 0.1 m, ρ = 1000 kg/m³, g = 9.8 m/s²
The total energy per unit mass of water
= \(\frac{p}{\rho}\) + \(\frac{1}{2}\)v² + gy
= \(\frac{10^{4} \mathrm{~Pa}}{10^{3} \mathrm{~kg} / \mathrm{m}^{3}}\) + \(\frac{1}{2}\) (2 × 10^-2 m/s)² + (9.8 m/ s²) (0.1 m)
= 10 + 0.0002 + 0.98 = 10.9802 J/kg

Question 4.
A horizontal wind with a speed of 11 m/s blows past a tall building which has large windowpanes of plate glass of dimensions 4 m × 1.5 m. The air inside the building is at atmospheric pressure. What is the total force exerted by the wind on a window pane ? [Density of air = 1.3 kg/m³]
Solution:
Data : v₁ (inside) = 0 m/s, v₂ (outside) = 11 m/s, ρ = 1.3 kg/m³, p₁ = p₀ = atmospheric pressure,
A = 4m × 1.5 m = 6m
Let p₂ be the air pressure outside a window. At the same height, Bernoulli’s equation gives
∴ p₁ + \(\frac{1}{2}\) ρv₁² = p₂ + \(\frac{1}{2}\)ρv₂²
∴ p₀ + 0 = p₂ + \(\frac{1}{2}\) ρv₂²
∴ The difference in pressure across a windowpane is
p₀ – p₂ = \(\frac{1}{2}\) ρv₂²
Since the right hand side is positive,
p₀ > p₂ and p₀ – p₂ is directed outward.
∴ The total force on a window pane is
F = (p₀ – p₂)A = \(\frac{1}{2}\) ρv₂² A
= \(\frac{1}{2}\) (1.3 kg/m³) (11 m/s)² (6 m²)
= 3.9 × 121 = 471.9 N (outward)

Question 5.
A water tank has a hole at a distance x from the free surface of water in the tank. If the radius of the hole is 2 mm and the velocity of efflux is 11 m/s, find x.
Solution:
Data: r = 2 mm, v = 11 m/s, g = 9.8 m/s²
By Torricelli’s law of efflux, the velocity of efflux,
v = \(\sqrt{2 g x}\)
∴ x = \(\frac{v^{2}}{2 g}=\frac{(11 \mathrm{~m} / \mathrm{s})^{2}}{2\left(9.8 \mathrm{~m} / \mathrm{s}^{2}\right)}\)
= \(\frac{121}{19.6}\) = 6.173 m

Multiple Choice Questions

Question 1.
Consider the following statements:
I. A fluid in hydrostatic equilibrium exerts only normal force on any surface within the fluid.
II. A fluid can resist a tangential force. Of these,
(A) only (I) is correct
(B) only (II) is correct
(C) both are correct
(D) both are false.
Answer:
(A) only (I) is correct

Question 2.
Which of the following is correct ?
(A) The free surface of a liquid at rest is horizontal.
(B) The pressure at a point within a liquid at rest is the same in all directions.
(C) The pressure at all points within a liquid at rest is the same.
(D) Both (A) and (B).
Answer:
(D) Both (A) and (B).

Question 3.
The surface of a liquid (of uniform density p) in a container is open to the atmosphere. The atmospheric pressure is ρ₀. The pressure ρgh, at a depth h below the surface of the liquid, is called the
(A) absolute pressure
(B) normal pressure
(C) gauge pressure
(D) none of these.
Answer:
(C) gauge pressure

Question 4.
Three vessels having the same base area are filled with water to the same height, as shown. The force exerted by water on the base is

(A) largest for vessel P
(B) largest for vessel Q
(C) largest for vessel R
(D) the same in all three.
Answer:
(D) the same in all three.

Question 5.
Rain drops or liquid drops are spherical in shape, especially when small, because
(A) cohesive force between the molecules of water have spheres of influence
(B) for a given volume, a spherical drop has the least surface energy
(C) for a given volume, a spherical drop has the maximum surface energy
(D) the pressure inside a drop is many times the atmospheric pressure outside.
Answer:
(B) for a given volume, a spherical drop has the least surface energy

Question 6.
The surface tension acts
(A) perpendicular to the surface and vertically up-wards
(B) perpendicular to the surface and vertically into the liquid
(C) tangential to the surface
(D) only at the liquid-solid interface.
Answer:
(C) tangential to the surface

Question 7.
A thin ring of diameter 8 cm is pulled out of water (surface tension 0.07 N/m). The force required to break free the ring from water is
(A) 0.0088 N
(B) 0.0176 N
(C) 0.0352 N
(D) 3.52 N.
Answer:
(C) 0.0352 N

Question 8.
A matchstick 5 cm long floats on water. The water film has a surface tension of 70 dyn/cm. A little comphor put on one side of stick reduces the surface tension there to 50 dyn/cm. The net force on the matchstick is
(A) 4 dynes
(B) 20 dynes
(C) 100 dynes
(D) 600 dynes.
Answer:
(C) 100 dynes

Question 9.
A big drop of radius R is formed from 1000 droplets of water. The radius of a droplet will be
(A) 10 R
(B) \(\frac{R}{10}\)
(C) \(\frac{R}{100}\)
(D) \(\frac{R}{1000}\)
Answer:
(B) \(\frac{R}{10}\)

Question 10.
The work done in breaking a spherical drop of a liquid (surface tension T) of radius R into 8 equal drops is
(A) πR²T
(B) 2πR²T
(C) 3πR²T
(D) 4πR²T.
Answer:
(D) 4πR²T.

Question 11.
If for a liquid in a vessel, the force of cohesion is more than the force of adhesion,
(A) the liquid does not wet the solid
(B) the liquid wets the solid
(C) the surface of the liquid is plane
(D) the angle of contact is zero.
Answer:
(A) the liquid does not wet the solid

Question 12.
If a liquid does not wet a solid surface, its angle of contact with the solid surface is
(A) zero
(B) acute
(C) 90°
(D) obtuse.
Answer:
(D) obtuse.

Question 13.
The pressure within a bubble is higher than that outside by an amount proportional
(A) directly to both the surface tension and the bubble size
(B) directly to the surface tension and inversely to the bubble size
(C) directly to the bubble size and inversely to the surface tension
(D) inversely to both the surface tension and the bubble size.
Answer:
(B) directly to the surface tension and inversely to the bubble size

Question 14.
The pressure difference across the surface of a spherical water drop of radius 1 mm and surface tension 0.07 N/m is
(A) 28 Pa
(B) 35 Pa
(C) 140 Pa
(D) 280 Pa.
Answer:
(C) 140 Pa

Question 15.
An air bubble just inside a soap solution and a soap bubble blown using the same solution have their radii in the ratio 3 : 2. The ratio of the excess pressure inside them is
(A) 1 : 12
(B) 1 : 6
(C) 1 : 3
(D) 1 : 2.
Answer:
(C) 1 : 3

Question 16.
A liquid rises to a height of 5 cm in a glass capillary tube of radius 0.02 cm. The height to which the liquid would rise in a glass capillary tube of radius 0.04 cm is
(A) 2.5 cm
(B) 5 cm
(C) 7.5 cm
(D) 10 cm.
Answer:
(A) 2.5 cm

Question 17.
In a gravity free space, the liquid in a capillary tube will rise to
(A) the same height as that on the Earth
(B) a lesser height than on the Earth
(C) slightly more height than that on the Earth
(D) the top and overflow.
Answer:
(D) the top and overflow.

Question 18.
In which of the following substances, does the surface tension increase with an increase in tempera¬ture?
(A) Copper
(B) Molten copper
(C) Iron
(D) Molten iron
Answer:
(B) Molten copper

Question 19.
A fluid flows in steady flow through a pipe. The pipe has a circular cross section, but its radius varies along its length. The mass of the fluid passing per second at the entrance point (radius R) of the pipe is Q₁ while that at the exit point (radius R/2) is Q₂. Then, Q₂ is equal to
(A) \(\frac{1}{4}\) Q₁
(B) Q₁
(C) 2Q₁
(D) 4Q₁.
Answer:
(B) Q₁

Question 20.
The dimensions of coefficient of viscosity are
(A) [ML^-1T^-2]
(B) [M^-1LT^-2]
(C) [MLT^-2]
(D) [ML^-1T^-1].
Answer:
(D) [ML^-1T^-1].

Question 21.
The unit of coefficient of viscosity is
(A) the pascal-second
(B) the pascal
(C) the poise-second
(D) both (A) and (C).
Answer:
(A) the pascal-second

Question 22.
A fluid flows past a sphere in streamline flow. The viscous force on the sphere is directly proportional to
(A) the radius of the sphere
(B) the speed of the flow
(C) the coefficient of viscosity of the fluid
(D) all of these.
Answer:
(D) all of these.

Question 23.
Water flows in a streamlined flow through the pipe shown in the following figure. The pressure

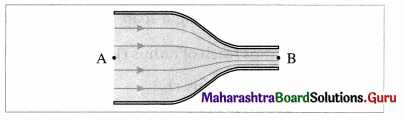
(A) is greater at A than at B
(B) at A equals that at B
(C) is less at A than at B
(D) at A is unrelated to that at B.
Answer:
(A) is greater at A than at B

Question 24.
Two steel marbles (of radii R and \(\frac{R}{3}\)) are released in a highly viscous liquid. The ratio of the terminal velocity of the larger marble to that of the smaller is
(A) 9
(B) 3
(C) 1
(D) \(\frac{1}{9}\)
Answer:
(A) 9

Question 25.
A large tank, filled with a liquid, is open to the atmosphere. If the tank discharges through a small hole at its bottom, the speed of efflux does NOT depend on
(A) cross-sectional area of the hole
(B) depth of the hole from the liquid surface
(C) acceleration due to gravity
(D) all of these.
Answer:
(A) cross-sectional area of the hole

Balbharti Maharashtra State Board 12th Physics Important Questions Chapter 1 Rotational Dynamics Important Questions and Answers.

Maharashtra State Board 12th Physics Important Questions Chapter 1 Rotational Dynamics

Question 1.
What is circular motion?
Answer:
The motion of a particle along a complete circle or a part of it is called circular motion.

Question 2.
What is a radius vector in circular motion?
Answer:
For a particle performing circular motion, its position vector with respect to the centre of the circle is called the radius vector.
[Note : The radius vector has a constant magnitude, equal to the radius of the circle. However, its direction changes as the position of the particle changes along the circumference.]

Question 3.
What is the difference between rotation and revolution?
Answer:
There is no physical difference between them. It is just a question of usage. Circular motion of a body about an axis passing through the body is called rotation. Circular motion of a body around an axis outside the body is called revolution.

Question 4.
State the characteristics of circular motion.
Answer:

1. It is an accelerated motion : As the direction of velocity changes at every instant, it is an accelerated motion.
2. It is a periodic motion : During the motion, the particle repeats its path along the same trajectory. Thus, the motion is periodic.

Question 5.
Explain angular displacement in circular motion.
Answer:
The change in the angular position of a particle performing circular motion with respect to a reference line in the plane of motion of the particle and passing through the centre of the circle is called the angular displacement.

As the particle moves in its circular path, its angular position changes, say from θ₁ at time t to θ₂ at a short time δt later. In the interval δt, the position vector \(\vec{r}\) sweeps out an angle δθ = θ₂ – θ₁. δθ is the magnitude of the change in the angular position of the particle.

Infinitesimal angular displacement \(\overrightarrow{\delta \theta}\) in an infinitesimal time interval δt → 0, is given a direction perpendicular to the plane of revolution by the right hand thumb rule.

Question 6.
Explain angular velocity. State the right hand thumb rule for the direction of angular velocity.
Answer:
Angular velocity : The time rate of angular displacement of a particle performing circular motion is called the angular velocity.

1. If the particle has an angular displacement \(\delta \vec{\theta}\) in a short time interval δt, its angular velocity
   
2. \(\vec{\omega}\) is a vector along the axis of rotation, in the direction of \(d \vec{\theta}\), given by the right hand thumb rule.

Right hand thumb rule : If the fingers of the right hand are curled in the sense of revolution of the particle, then the outstretched thumb gives the direction of the angular displacement.

[Note : Angular speed, ω = |\(\vec{\omega}\)| = \(\frac{d \theta}{d t}\) is also called angular frequency. ]

Question 7.
Explain the linear velocity of a particle performing circular motion.
OR
Derive the relation between the linear velocity and the angular velocity of a particle performing circular motion.
Answer:
Consider a particle performing circular motion in an anticlockwise sense, along a circle of radius r. In a very small time interval δt, the particle moves from point A to point B through a distance δs and its angular position changes by δθ.

Since is tangential, the instantaneous linear velocity \(\vec{v}\) of a particle performing circular motion is along the tangent to the path, in the sense of motion of the particle.

\(\vec{v}\), \(\vec{\omega}\) and \(\vec{r}\) are mutually perpendicular, so that in magnitude, v = ωr.

Question 8.
State the relation between the linear velocity and the angular velocity of a particle in circular motion.
Answer:
Linear velocity, \(\vec{v}\) = \(\vec{\omega}\) × \(\vec{r}\) where ω is the angular velocity and r is the radius vector.

At every instant, \(\vec{v}\), \(\vec{\omega}\) and \(\vec{r}\) are mutually perpendicular, so that in magnitude v = ωr.

Question 9.
Define uniform circular motion (UCM).
Answer:
A particle is said to perform uniform circular motion if it moves in a circle or a circular arc at constant linear speed or constant angular velocity.

Question 10.
A stone tied to a string is rotated in a horizontal circle (nearly). If the string suddenly breaks, in which direction will the stone fly off ?
Answer:
In a circular motion, the instantaneous velocity \(\vec{v}\) is always tangential, in the sense of the motion. Hence, an inertial observer will see the stone fly off tangentially, in the direction of \(\vec{v}\) at the instant the string breaks.

Question 11.
What is the angular speed of a particle moving in a circle of radius r centimetres with a constant speed of v cm/s ?
Answer:
Angular speed, ω = \(\frac{v \mathrm{~cm} / \mathrm{s}}{r \mathrm{~cm}}\) = \(\frac{v}{r}\) rad/s.

Question 12.
Define the period and frequency of revolution of a particle performing uniform circular motion (UCM) and state expressions for them. Also state their SI units.
Answer:
(1) Period of revolution : The time taken by a particle performing UCM to complete one revolution is called the period of revolution or the period (T) of UCM.
T = \(\frac{2 \pi r}{v}\) = \(\frac{2 \pi}{\omega}\)
where v and ω are the linear and angular speeds, respectively.
SI unit: the second (s)
Dimensions : [M°L°T¹].

(2) Frequency of revolution : The number of revolutions per unit time made by a particle in UCM is called the frequency of revolution (f).

The particle completes 1 revolution in periodic time T. Therefore, it completes 1/T revolutions per unit time.
∴ Frequency f = \(\frac{1}{T}\) = \(\frac{v}{2 \pi r}\) = \(\frac{\omega}{2 \pi}\)
SI unit : the hertz (Hz), 1 Hz = 1 s^-1
Dimensions : [M°L°T^-1]

Question 13.
If the angular speed of a particle in UCM is 20π rad/s, what is the period of UCM of the particle?
Answer:
The period of UCM of the particle,
T = \(\frac{2 \pi}{\omega}\) = \(\frac{2 \pi}{20 \pi}\) = 0.1 s

Question 14.
Why is UCM called a periodic motion?
Answer:
In a uniform motion, a particle covers equal distances in equal intervals of time. Any motion which repeats itself in equal intervals of time is called a periodic motion. In a uniform circular motion (UCM), the particle takes the same time to complete each revolution, a distance equal to the circumference of the circle. Therefore, it is a periodic motion.

Question 15.
Give one example of uniform circular motion.
Answer:

1. Circular motion of every particle of the blades of a fan or the dryer drum of a washing machine when the fan or the drum is rotating with a constant angular speed.
2. Motion of the hands of a clock.
3. Motion of an Earth-satellite in a circular orbit.

Question 16.
What can you say about the angular speed of an hour hand as compared to that of the Earth’s rotation about its axis ?
Answer:
The periods of rotation of an hour hand and the Earth are T_h = 12 h and T_E = 24 h, respectively, so that their angular speeds are ω_h = \(\frac{2 \pi}{12}\) rad/h and ω_E = \(\frac{2 \pi}{24}\) rad/h.
∴ ω_h = 2ω_E

Question 17.
Explain the acceleration of a particle in UCM. State an expression for the acceleration.
Answer:
A particle in uniform circular motion (UCM) moves in a circle or circular arc at constant linear speed v. The instantaneous linear velocity \(\vec{v}\) of the particle is along the tangent to the path in the sense of motion of the particle. Since \(\vec{v}\) changes in direction, without change in its magnitude, there must be an acceleration that must be

1. perpendicular to \(\vec{v}\)
2. constant in magnitude
3. at every instant directed radially inward, i.e., towards the centre of the circular path.

Such a radially inward acceleration is called a centripetal acceleration.
∴ \(\vec{a}\) = \(\frac{d \vec{v}}{d t}\) = \(\overrightarrow{a_{\mathrm{r}}}\)

If \(\vec{\omega}\) is the constant angular velocity of the particle and r is the radius of the circle,
\(\overrightarrow{a_{\mathrm{r}}}\) = –\(\omega^{2} \vec{r}\)
where ω = |\(\vec{\omega}\)| and the minus sign shows that the direction of \(\vec{a}_{\mathrm{r}}\) is at every instant opposite to that of the radius vector \(\vec{r}\). In magnitude,
a_r = ω²r = \(\frac{v^{2}}{r}\) = ωv

[Note : The word centripetal comes from Latin for ‘centre-seeking’.]

Question 18.
Draw a diagram showing the linear velocity, angular velocity and radial acceleration of a particle performing circular motion with radius r.
Answer:

Question 19.
If a particle in UCM has linear speed 2 m/s and angular speed 5 rad/s, what is the magnitude of the centripetal acceleration of the particle ?
Answer:
The magnitude of the centripetal acceleration of the particle is a_r = ωv = (5)(2) = 10 m/s²

Question 20.
State any two quantities that are uniform in UCM.
Answer:
Linear speed and angular speed. (Also, kinetic energy, angular speed and angular momentum.)

Question 21.
State any two quantities that are nonuniform in UCM.
Answer:
Velocity and acceleration are nonuniform in UCM.
(Also, centripetal force.)

Question 22.
What is a nonuniform circular motion?
Answer:
Consider a particle moving in a plane along a circular path of constant radius. If the particle is speeding up or slowing down, its angular speed ω and linear speed v both change with time. Then, the particle is said to be in a non uniform circular motion.

Question 23.
Explain angular acceleration.
Answer:
Angular acceleration : The time rate of change of angular velocity of a particle performing circular motion is called the angular acceleration.

(i) If \(\delta \vec{\omega}\) is the change in angular Velocity in a short time interval St, the angular acceleration

(ii) The direction of \(\vec{\alpha}\) is the same as that of \(d \vec{\omega}\). We consider the case where a change in \(\vec{\omega}\) arises due to a change in its magnitude only. If the particle is speeding up, i.e., ω is increasing with time, then \(\vec{\alpha}\) is in the direction of \(\vec{\omega}\). If the particle is slowing down, i.e., ω is decreasing with time, then \(\vec{\alpha}\) is directed opposite to \(\vec{\omega}\).

(iii) If the angular speed changes from ω₁ to ω₂ in time f, the magnitude (α) of the average angular acceleration is
α = \(\frac{\omega_{2}-\omega_{1}}{t}\)

Question 24.
Explain the tangential acceleration of a particle in non uniform circular motion.
Answer:
Tangential acceleration : For a particle performing circular motion, the linear acceleration tangential to the path that produces a change in the linear speed of the particle is called the tangential acceleration.
Explanation :
(i) If a particle performing circular motion is speeding up or slowing down, its angular speed co and linear speed v both change with time.

The linear acceleration that produces a change only in the linear speed must be along \(\vec{v}\). Hence, it is called the tangential acceleration, \(\overrightarrow{a_{\mathrm{t}}}\). In magnitude, a_t = dv/dt

(ii) If the linear speed v of the particle is increasing, \(\overrightarrow{a_{\mathrm{t}}}\) is in the direction of \(\vec{v}\). If v is decreasing, \(\overrightarrow{a_{\mathrm{t}}}\) is directed opposite to \(\vec{v}\).

Question 25.
Obtain the relation between the magnitudes of the linear (tangential) acceleration and angular acceleration in non uniform circular motion.
Answer:
Consider a particle moving along a circular path of constant radius r. If the particle is speeding up or slowing down, its motion is nonuniform, and its angular speed ω and linear speed v both change with time. At any instant, v, ω and r are related by v = ωr
The angular acceleration of the particle is
α = \(\frac{d \omega}{d t}\)

The tangential acceleration \(\overrightarrow{a_{\mathrm{t}}}\) is the linear acceleration that produces a change in the linear speed of the particle and is tangent to the circle. In magnitude,

This is the required relation.

Question 26.
Obtain an expression for the acceleration of a particle performing circular motion. Explain its two components.
OR
For a particle performing uniform circular motion, \(\vec{v}\) = \(\vec{\omega}\) × \(\vec{r}\). Obtain an expression for the linear acceleration of a particle performing non-uniform circular motion.
OR
In circular motion, assuming \(\vec{v}\) = \(\vec{\omega}\) × \(\vec{r}\), obtain an expression for the resultant acceleration of a particle in terms of tangential and radial components.
Answer:
Consider a particle moving along a circular path of constant radius r. If its motion is nonuniform, then its angular speed ω and linear speed v both change with time.

acceleration is called the radial or centripetal acceleration \(\overrightarrow{a_{\mathrm{r}}}\).
\(\overrightarrow{a_{\mathrm{r}}}\) = \(\vec{\omega}\) × \(\vec{v}\) … (5)
In magnitude, a_r = ωv
since \(\vec{\omega}\) is perpendicular to \(\vec{v}\).
∴ \(\vec{a}\) = \(\overrightarrow{a_{\mathrm{t}}}\) + \(\overrightarrow{a_{\mathrm{r}}}\) …. (6)
This is the required expression.

Question 27.
What is the angle between linear acceleration and angular acceleration of a particle in nonuniform circular motion ?
Answer:
In a nonu niform circular motion, the angular acceleration is an axial vector, perpendicular to the plane of the motion. The linear acceleration is in the plane of the motion. Hence, the angle between them is 90°.

Question 28.
What are the differences between a nonuniform circular motion and a uniform circular motion? (Two points of distinction) Give examples.
Answer:
(i) Nonuniform circular motion :

1. The angular and tangential accelerations are non-zero, so that linear and angular speeds both change with time.
   
2. The net linear acceleration, being the resultant of the radial and tangential accelerations, is not radial, \(\vec{a}\) = \(\overrightarrow{a_{\mathrm{c}}}\) + \(\overrightarrow{a_{t}}\),
3. The magnitudes of the centripetal acceleration and the centripetal force are not constant.
4. Example : Motion of the tip of a fan blade when the fan is speeding up or slowing down.

(ii) Uniform circular motion :

1. The angular and tangential accelerations are zero, so that linear speed and angular velocity are constant.
2. The net linear acceleration is radially inward, i.e., centripetal.
3. The magnitudes of the centripetal acceleration and the centripetal force are also constant.
4. Example : Motion of the tips of the hands of a mechanical clock.

Question 29.
Write the kinematical equations for circular motion in analogy with linear motion.
Answer:
For circular motion of a particle with constant angular acceleration α,

where ω₀ and ω are the initial and final angular speeds, t is the time, ω_av the average angular speed and θ_o and θ the initial and final angular positions of the particle.

Then, the angular kinematical equations for the circular motion are (in analogy with linear kinematical equations for constant linear acceleration)
ω = ω₀ + αt
θ – θ₀ = ω₀t + \(\frac{1}{2} \alpha t^{2}\)
ω² = \(\omega_{0}^{2}\) + 2α (θ – θ₀)

Question 30.
Solve the following :

Question 1.
Certain stars are believed to be rotating at about 1 rot/s. If such a star has a diameter of 40 km, what is the linear speed of a point on the equator of the star?
Solution :
Data : d = 40 km, /= 1 rot/s
∴ r = \(\frac{d}{2}\) = \(\frac{40 \mathrm{~km}}{2}\) = 20 km = 2 × 10⁴ m
Linear speed, v = ωr = (2πf)r
= (2 × 3.142 × 1)(2 × 10⁴)
= 6.284 × 2 × 10⁴
= 1.257 × 10⁵ m/s (or 125.6 km/s)

Question 2.
A body of mass 100 grams is tied to one end of a string and revolved along a circular path in the horizontal plane. The radius of the circle is 50 cm. If the body revolves with a constant angular speed of 20 rad/s, find the

1. period of revolution
2. linear speed
3. centripetal acceleration of the body.

Solution :
Data : m = 100 g = 0.1 kg, r = 50 cm = 0.5 m, ω = 20 rad/s

1. The period of revolution of the body,
   
2. Linear speed, v = ωr = 20 × 0.5 = 10 m/s
3. Centripetal acceleration,
   a_c = w²r = (20)² × 0.5 = 200 m/s²

Question 3.
Calculate the angular speed of the Earth due to its spin (rotational motion).
Solution :
Data : T = 24 hours = 24 × 60 × 60 s

The angular speed of the Earth due to its spin (rotational motion) is 7.273 × 10^-5 rad/s.

Question 4.
Find the angular speed of rotation of the Earth so that bodies on the equator would feel no weight. [Radius of the Earth = 6400 km, g = 9.8 m/s²]
Solution :
Data : Radius of the Earth = r = 6400 km = 6.4 × 10⁶ m, g = 9.8 m/s²

As the Earth rotates, the bodies on the equator revolve in circles of radius r.

Question 31.
Write the kinematical equations for circular motion in analogy with linear motion.
Answer:
For circular motion of a particle with constant angular acceleration α,

where ω₀ and ω are the initial and final angular speeds, t is the time, ω_av the average angular speed and 0o and 0 the initial and final angular positions of the particle.

Then, the angular kinematical equations for the circular motion are (in analogy with linear kinematical equations for constant linear acceleration)
ω = ω₀ + αt
θ – θ_o = ω₀t + \(\frac{1}{2} \alpha t^{2}\)
ω² = \(\omega_{0}^{2}\) + 2α (θ – θ_o)

Question 32.
Solve the following :

Question 1.
Certain stars are believed to be rotating at about 1 rot/s. If such a star has a diameter of 40 km, what is the linear speed of a point on the equator of the star?
Solution :
Data : d = 40 km, f= 1 rot/s
∴ r = \(\frac{d}{2}\) = \(\frac{40 \mathrm{~km}}{2}\) = 20 km = 2 × 10⁴ m
Linear speed, v = ωr = (2πf)r
= (2 × 3.142 × 1)(2 × 10⁴)
= 6.284 × 2 × 10⁴
= 1.257 × 10⁵ m/s (or 125.6 km/s)

Question 2.
A body of mass 100 grams is tied to one end of a string and revolved along a circular path in the horizontal plane. The radius of the circle is 50 cm. If the body revolves with a constant angular speed of 20 rad/s, find the

1. period of revolution
2. linear speed
3. centripetal acceleration of the body.

Solution :
Data : m = 100 g = 0.1 kg, r = 50 cm = 0.5 m, ω = 20 rad/s

1. The period of revolution of the body,
   
2. Linear speed, v = ωr = 20 × 0.5 = 10 m/s
3. Centripetal acceleration,
   a_c = ω² = (20)² × 0.5 = 200 m/s²

Question 3.
Calculate the angular speed of the Earth due to its spin (rotational motion).
Solution :
Data : T = 24 hours = 24 × 60 × 60 s

The angular speed of the Earth due to its spin (rotational motion) is 7.273 × 10^-5 rad/s.

Question 4.
Find the angular speed of rotation of the Earth so that bodies on the equator would feel no weight. (Radius of the Earth = 6400 km, g = 9.8 m/s²]
Solution :
Data : Radius of the Earth = r = 6400 km
= 6.4 × 10⁶ m, g = 9.8 m/s²
As the Earth rotates, the bodies on the equator revolve in circles of radius r.
These bodies would not feel any weight if their centripetal acceleration (ωr) is equal to the acceleration due to gravity (g).
∴ ω²r = g
The angular speed of the Earth’s rotation,

Question 5.
To simulate the acceleration of large rockets, astronauts are seated in a chamber and revolved in a circle of radius 9.8 m. What angular speed is required to generate a centripetal acceleration 8 times the acceleration due to gravity? [g = 9.8 m/s²]
Solution :
Data : r = 9.8 m, g = 9.8 m/s², a = 8g
Centripetal acceleration = ω²r
∴ ω²r = 8g
∴ 9.8 ω² = 8(9.8)
∴ ω² = 8
The required angular speed, ω = \(\sqrt{8}\) = 2\(\sqrt{2}\) = 2.828 rad/s

Question 6.
The angular position of a rotating object is given by θ(t) = (1.55t² – 7.75 t + 2.87) rad, where t is measured in second.
(i) When is the object momentarily at rest ?
(ii) What is the magnitude of its angular acceleration at that time ?
Solution :

Question 7.
A motor part at a distance of 1.5 m from the motor’s axis of rotation has a constant angular acceleration of 0.25 rad/s². Find the magnitude of its linear acceleration at the instant when its angular speed is 0.5 rad/s.
Solution :
Data : r = 1.5 m, α = 0.25 rad/s², ω = 0.5 rad/s² a_r = ω²r = (0.5)²(1.5) = 0.25 × 1.5 = 0.375 m/s² a_t = αr = 0.25 × 1.5 = 0.375 m/s²
The linear acceleration,

Question 8.
A coin is placed on a stationary disc at a distance of 1 m from the disc’s centre. At time t = 0 s, the disc begins to rotate with a constant angular acceleration of 2 rad/s² around a fixed vertical axis through its centre and perpendicular to its plane.
Find the magnitude of the linear acceleration of the coin at t = 1.5 s. Assume the coin does not slip.
Solution :

Question 9.
A railway locomotive enters a stretch of track, which is in the form of a circular arc of radius 280 m, at 10 m/s and with its speed increasing uniformly. Ten seconds into the stretch its speed is 14m/s and at 18s its speed is 19 m/s. Find
(i) the magnitude of the locomotive’s linear acceleration when its speed is 14 m/s
(ii) the direction of this acceleration at that point with respect to the locomotive’s radial acceleration
(iii) the angular acceleration of the locomotive.
Answer:
Data : r = 280 m, v₁ = 10 m/s at t₁ = 0, v₂ = 14 m/s at t₂ = 10 s, v₃ = 19 m/s at t₃ = 18 s
(i) At t = t₂, the radial acceleration is

Since the tangential acceleration is constant, it may be found from the data for any two times.

(ii) If θ is the angle between the resultant linear acceleration and the radial acceleration,
tan θ = \(\frac{a_{\mathrm{t}}}{a_{\mathrm{r}}}\) = \(\frac{0.5}{0.7}\) = 0.7142
∴ θ = tan^-1 0.7142 = 35°32′

(iii) a_t = αr
The angular acceleration,
α = \(\frac{a_{\mathrm{t}}}{r}\) = \(\frac{0.5}{280}\)
= 1.785 × 10^-3 rad/s²
= 1.785 mrad/s²

Question 10.
The frequency of revolution of a particle performing circular motion changes from 60 rpm to 180 rpm in 20 seconds. Calculate the angular acceleration of the particle.
Solution :
Data : f₁ =60 rpm = \(\frac{60}{60}\) rev/s = 1 rev/s, f₂ = 180 rpm = \(\frac{180}{60}\) rev/s = 3 rev/s, t = 20 s
The angular acceleration in SI units,

OR
Using non SI units, the angular frequencies are ω₁ = 60 rpm = 1 rps and ω₂ = 180 rpm = 3 rps.

Question 11.
The frequency of rotation of a spinning top is 10 Hz. If it is brought to rest in 6.28 s, find the angular acceleration of a particle on its surface.
Solution:
Data: f₁ = 10Hz, f₂ = 0 Hz, t = 6.28s
The angular acceleration,

Question 12.
A wheel of diameter 40 cm starts from rest and attains a speed of 240 rpm in 4 minutes. Calculate its angular displacement in this time interval.
Solution:

Question 13.
A flywheel slows down uniformly from 1200 rpm to 600 rpm in 5 s. Find the number of revolutions made by the wheel in 5 s.
Solution :
Data : ω₀ = 1200 rpm, ω = 600 rpm, f = 5 s
Since the flywheel slows down uniformly, its angular acceleration is constant. Then, its average angular speed,

Its angular displacement in time t,
θ = ω_av.t = 15 × 5 = 75 revolutions

Question 33.
Define and explain centripetal force.
Answer:
Definition : In the uniform circular motion of a particle, the centripetal force is the force on the particle which at every instant points radially towards the centre of the circle and produces the centripetal acceleration to move the particle in its circular path.

Explanation : A uniform circular motion is an accelerated motion, with a radially inward (i.e., centripetal) acceleration –\(\frac{v^{2}}{r} \hat{\mathrm{r}}\) or \(-\frac{v^{2}}{r} \hat{\mathrm{r}}\), where \(\vec{r}\) is the radius vector and \(\hat{\mathbf{r}}\) is a unit vector in the direction of \(\vec{r}\). Hence, a net real force must act on the particle to produce this acceleration. This force, which at every instant must point radially towards the centre of the circle, is called the centripetal force. If m is the mass of the particle, the centripetal force is

Notes :

1. As viewed from an inertial frame of reference, the centripetal force is necessary and sufficient for the particle to perform UCM. At any instant, if the centripetal force suddenly vanishes, the particle would fly off in the direction of its linear velocity at that instant.
2. In case the angular or linear speed changes with time, as in nonuniform circular motion, the force is not purely centripetal but has a tangential component which accounts for the tangential acceleration.

Question 34.
Give any two examples of centripetal force.
Answer:
Examples of centripetal force :

1. For an Earth-satellite in a circular orbit, the centripetal force is the gravitational force exerted by the Earth on the satellite.
2. In the Bohr atom, the centripetal force on an electron in circular orbit around the nucleus is the attractive Coulomb force of the nucleus.
3. When an object tied at the end of a string is revolved in a horizontal circle, the centripetal force is the tension in the string.
4. When a car takes a turn in a circular arc on a horizontal road with constant speed, the force of static friction between the car tyres and road surfaces is the centripetal force.

Note : The tension in a string or the force of friction is electromagnetic in origin.

Question 35.
Define and explain centrifugal force.
Answer:
Definition : In the reference frame of a particle performing circular motion, centrifugal force is defined as a fictitious, radially outward force on the particle and is equal in magnitude to the particle’s mass times the centripetal acceleration of the reference frame, as measured from an inertial frame of reference.

Explanation : A uniform circular motion is an accelerated motion, with a centripetal acceleration of magnitude v²/r or ω²r. A frame of reference attached to the particle also has this acceleration and, therefore, is an accelerated or noninertial reference frame. The changing direction of the linear velocity appears in this reference frame as a tendency to move radially outward. This is explained by assuming a fictitious centrifugal, i.e., radially outward, force acting on the particle. Since the particle is stationary in its reference frame, the magnitude of the centrifugal force is mv²/r or mω²r, the same as that of the centripetal force on the particle.

Note : The word ‘centrifugal’ comes from the Latin for ‘fleeing from the centre’. The word has the same root fuge from the Latin ‘to flee’ as does refugee.

Question 36.
Give any two examples of centrifugal force.
Answer:
Examples of centrifugal force :

1. A person in a merry-go-round experiences a radially outward force.
2. Passengers of a car taking a turn on a level road experience a force radially away from the centre of the circular road.
3. A coin on a rotating turntable flies off for some high enough angular speed of the turntable.
4. As the Earth rotates about its axis, the centrifugal force on its particles is directed away from the axis. The force increases as one goes from the poles towards the equator. This leads to the bulging of the Earth at the equator.

Question 37.
Explain why centrifugal force is called a pseudo force.
Answer:
A force which arises from gravitational, electromagnetic or nuclear interaction between matter is called a real force. The centrifugal force does not arise due to any of these interactions. Therefore, it is not a real force.

The centrifugal force in the noninertial frame of reference of a particle in circular motion is the effect of the acceleration of the frame of reference. Therefore, it is called a pseudo or fictitious force.

Question 38.
Distinguish between centripetal force and centrifugal force. State any two points of distinction.
Answer:

Centripetal force	Centrifugal force
1. Centripetal force is the force required to provide centri­petal acceleration to a par­ticle to move it in a circular path.	1. The centrifugal tendency of the particle, in its acceler­ated, i.e., non-inertial, frame of reference, is explained by assuming a centrifugal force acting on it.
2. At every instant, it is directed radially towards the centre of the circular path.	2. At every instant, it is directed radially away from the centre of the circular path.
3. It is a real force arising from gravitational or electromag­netic interaction between matter.	3. It is a pseudo force since it is the effect of the acceleration of the reference frame of the revolving particle.

Question 39.
Solve the following :

Question 1.
An object of mass 0.5 kg is tied to a string and revolved in a horizontal circle of radius 1 m. If the breaking tension of the string is 50 N, what is the maximum speed the object can have?
Solution :
Data : m = 0.5 kg, r = 1 m, F = 50 N
The maximum centripetal force that can be applied is equal to the breaking tension.

This is the maximum speed the object can have.

Question 2.
A certain string 500 cm long breaks under a tension of 45 kg wt. An object of mass 100 g is attached to this string and whirled in a horizontal circle. Find the maximum number of revolutions that the object can make per second without breaking the string, [g = 9.8 m/s²]
Solution :
Data : m = 100 g = 0.1 kg, r = 500 cm = 5 m, g = 9.8 m/s², F = 45 kg wt = 45 × 9.8 N
The breaking tension is equal to the maximum centripetal force that can be applied.
∴ F = mω²r ,
But ω = 2πf, where/is the corresponding frequency of revolution.

The maximum number of revolutions per second, f = 4.726 Hz

Question 3.
A disc of radius 15 cm rotates with a speed of 33\(\frac{1}{3}\) rpm. Two coins are placed on it at 4 cm and 14 cm from its centre. If the coefficient of friction between the coins and the disc is 0.15, which of the two coins will revolve with the disc ?
Solution :
Data : r = 15 cm = 0.15 m,
f = 33\(\frac{1}{3}\) rpm = \(\frac{100}{3 \times 60}\)rev/s = \(\frac{5}{9}\) Hz, µ_s = 0.15, r₁ = 4 cm = 0.04 m, r₂ = 14 cm = 0.14 m

To revolve with the disc without slipping, the necessary centripetal force must be less than or equal to the limiting force of static friction.

Limiting force of static friction, f_s = µ_s N = µ_s (mg) where m is the mass of the coin and N = mg is the normal force on the coin.
∴ mω²r ≤ µ_s(mg) or ω²r ≤ µ_sg
µ_sg = 0.15 × 9.8 = 1.47 m/s²
For the first coin, r₁ = 0.04 m.
∴ ω²r₁ = (3.491)² × 0.04 = 12.19 × 0.04 = 0.4876 m/s²
Since, ω²r₁ < µ_sg, this coin will revolve with the disc. For the second coin, r₂ = 0.14 m.
∴ ω²r₂ = (3.491)² × 0.14 = 12.19 × 0.14 = 1.707 m/s²
Since, ω²r₂ > µ_sg, this coin will not revolve with the disc.
Thus, only the coin placed at 4 cm from the centre will revolve with the disc.

Question 40.
Derive an expression for the maximum safe speed for a vehicle on a horizontal circular road without skidding off. State its significance.
Answer:
Consider a car of mass m taking a turn of radius r along a level road. If µ_s is the coefficient of static friction between the car tyres and the road surface, the limiting force of friction is f_s = µ_sN = µ_smg where N = mg is the normal reaction. The forces on the car, as seen from an inertial frame of reference are shown in below figure.

Then, the maximum safe speed v_max with which the car can take the turn without skidding off is set by maximum centripetal force = limiting force of static friction

This is the required expression.
Significance : The above expression shows that the maximum safe speed depends critically upon friction which changes with circumstances, e.g., the nature of the surfaces and presence of oil or water on the road. If the friction is not sufficient to provide the necessary centripetal force, the vehicle is likely to skid off the road.

[Note : At a circular bend on a level railway track, the centrifugal tendency of the railway carriages causes the flange of the outer wheels to brush against the outer rail and exert an outward thrust on the rail. Then, the reaction of the outer rail on the wheel flange provides the necessary centripetal force.]

Question 41.
Derive an expression for the maximum safe speed for a vehicle on a circular horizontal road without toppling/overturning/rollover.
Answer:
Consider a car of mass m taking a turn of radius r along a level road. As seen from an inertial frame of reference, the forces acting on the car are :

1. the lateral limiting force of static friction \(\overrightarrow{f_{\mathrm{s}}}\) on the wheels-acting along the axis of the wheels and towards the centre of the circular path which provides the necessary centripetal force.
2. the weight \(m \vec{g}\) acting vertically downwards at the centre of gravity (C.G.)
3. the normal reaction \(\vec{N}\) of the road on the wheels, acting vertically upwards effectively at the C.G. Since maximum centripetal force = limiting force of static friction,
   

In a simplified rigid-body vehicle model, we consider only two parameters-the height h of the C.G. above the ground and the average distance b between the left and right wheels called the track width.

The friction force \(\overrightarrow{f_{\mathrm{s}}}\) on the wheels produces a torque T, that tends to overturn/rollover the car about the outer wheel. Rotation about the front-to-back axis is called roll

When the inner wheel just gets lifted above the ground, the normal reaction \(\vec{N}\) of the road acts on the outer wheels but the weight continues to act at the C.G. Then, the couple formed by the normal reaction and the weight produces a opposite torque \(\tau_{\mathrm{r}}\) which tends to restore the car back on all four wheels
\(\tau_{\mathrm{r}}\) = mg.\(\frac{b}{2}\) … (3)

The car does not topple as long as the restoring torque \(\tau_{\mathrm{r}}\) counterbalances the toppling torque \(\tau_{\mathrm{t}}\). Thus, to avoid the risk of rollover, the maximum speed that the car can have is given by

Thus, vehicle tends to roll when the radial acceleration reaches a point where inner wheels of the four-wheeler are lifted off of the ground and the vehicle is rotated outward. A rollover occurs when the gravitational force \(m \vec{g}\) passes through the pivot point of the outer wheels, i.e., the C.G. is above the line of contact of the outer wheels. Equation (3) shows that this maximum speed is high for a car with larger track width and lower centre of gravity.

Question 42.
A carnival event known as a “well of death” consists of a large vertical cylinder inside which usually a stunt motorcyclist rides in horizontal circles. Show that the minimum speed necessary to keep the rider from falling is given by v = \(\sqrt{r g / \mu_{s}}\), in usual notations.
Answer:
The forces exerted on the rider are

1. the normal force \(\vec{N}\) exerted by the wall, directed radially inward, is the centripetal force,
2. the upward frictional force \(\overrightarrow{f_{\mathrm{s}}}\) exerted by the wall, since the motorcycle has a tendency to slide down,
3. the downward gravitational force \(m \vec{g}\).

which is the required expression.

Question 43.
A road at a bend should be banked for an optimum or most safe speed v₀. Derive an expression for the required angle of banking.
OR
Obtain an expression for the optimum or most safe speed with which a vehicle can be driven along a curved banked road. Hence show that the angle of banking is independent of the mass of a vehicle.
Answer:
Consider a car taking a left turn along a road of radius r banked at an angle θ for a designed optimum or most safe speed v₀. Let m be the mass of the car. In general, the forces acting on the car are
(a) its weight \(m \vec{g}\), acting vertically down
(b) the normal reaction of the road \(\vec{N}\), perpendicular to the road surface
(c) the frictional force \(\overrightarrow{f_{s}}\) along the inclined surface of the road.

At the optimum speed, frictional force is not relied upon to contribute to the necessary lateral centripetal force. Thus, ignoring \(\overrightarrow{f_{\mathrm{s}}}\), resolve \(\vec{N}\) into two perpendicular components : N cos θ vertically up and N sin θ horizontally towards the centre of the circular path. Since there is no acceleration in the vertical direction, N cos θ balances mg and N sin θ provides the necessary centripetal force.

Equation (3) gives the expression for the required angle of banking. From EQ. (3), we can see that θ depends upon v₀, r and g. The angle of banking is independent of the mass of a vehicle negotiating the curve. Also, for a given r and θ, the recommended optimum speed is
v₀ = \(\sqrt{r g \tan \theta}\) … (4)

Question 44.
State any two factors on which the most safe speed of a car in motion along a banked road depends.
Answer:
The angle of banking of the road and the radius of the curved path.

Question 45.
A curved horizontal road must be banked at an angle θ’ for an optimum speed v. What will happen to a vehicle moving with a speed v along this road if the road is banked at an angle θ such that
(i) θ < θ’
(ii) θ > θ’?
Answer:
(i) For θ < θ’, the horizontal component of the normal reaction would be less than the optimum value and will not be able to provide the necessary centripetal force. Then, the vehicle will tend to skid outward, up the inclined road surface.

(ii) For θ > θ’, the horizontal component of the normal reaction would be more than the necessary centripetal force. Then, the vehicle will tend to skid down the banked road.

Question 46.
A banked circular road is designed for traffic moving at an optimum or most safe speed v₀. Obtain an expression for
(a) the minimum safe speed
(b) the maximum safe speed with which a vehicle can negotiate the curve without skidding.
Answer:
Consider a car taking a left turn along a road of radius r banked at an angle θ for a designed optimum speed v. Let m be the mass of the car. In general, the forces acting on the car are
(a) its weight \(m \vec{g}\), acting vertically down
(b) the normal reaction of the road \(\vec{N}\), perpendicular to the road surface
(c) the frictional force \(\overrightarrow{f_{\mathrm{s}}}\) along the inclined surface of the road.
If µ_s is the coefficient of static friction between the tyres and road, f_s = µ_sN.

(a) For minimum safe speed : If the car is driven at a speed less than the optimum speed v₀, it may tend to slide down the inclined surface of the road so that \(\overrightarrow{f_{\mathrm{s}}}\) is up the incline.

Resolve \(\vec{N}\) and \(\overrightarrow{f_{\mathrm{s}}}\) into two perpendicular components : Ncos θ and f_s sin θ vertically up; N sin θ horizontally towards centre of the circular path. So long as the car takes the turn without skidding off, the horizontal components N sin θ and f_s cos θ together provide the necessary centripetal force, and N cos θ balances the sum mg + f_s sin θ. If v_max is the maximum safe speed without skidding,

(b) For maximum safe speed : If the car is driven fast enough, at a speed greater than the optimum speed v, it may skid off up the incline so that \(\overrightarrow{f_{\mathrm{s}}}\) is down the incline.

Resolve \(\vec{N}\) and \(\overrightarrow{f_{\mathrm{s}}}\) into two perpendicular components : N cos θ vertically up and f_s sin θ vertically down; N sin θ and f_s cos θ horizontally towards the centre of the circular path. So long as the car takes the turn without skidding off, the horizontal components N sin θ and f cos θ together provide the necessary centripetal force, and N cos θ balances the sum mg + f sin θ. If v is the maximum safe
speed without skidding,

Ignoring few special cases, the maximum value of µ_s = 1. Thus, for θ ≥ 45°, v_max = ∞, i.e., on a heavily banked road a car is unlikely to skid up the incline and the minimun limit is more important.

Question 47.
Solve the following :

Question 1.
Find the maximum speed with which a car can be safely driven along a curve of radius 100 m, if the coefficient of friction between its tyres and the road is 0.2 [g = 9.8 m/s²].
Solution :
Data : r = 100 m, µ_s = 0.2, g = 9.8 m/s²
The maximum speed, v = \(\sqrt{r \mu_{s} g}\)
= \(\sqrt{100 \times 0.2 \times 9.8}\) = 14 m/s

Question 2.
A flat curve on a highway has a radius of curvature 400 m. A car goes around the curve at a speed of 32 m/s. What is the minimum value of the coefficient of friction that will prevent the car from sliding?
Solution:
Data : r = 400 m, v = 32 m/s, g = 10 m/s²

Question 3.
A car can be driven on a flat circular road of radius r at a maximum speed v without skidding. The same car is now driven on another flat circular road of radius 2r on which the coefficient of friction between its tyres and the road is the same as on the first road. What is the maximum speed of the car on the second road such that it does not skid?
Solution:
Data: v₁ = v, r₁ = r, r₂ = 2r
On a flat circular road, the maximum safe speed is

Question 4.
On a dry day, the maximum safe speed at which a car can be driven on a curved horizontal road without skidding is 7 m/s. When the road is wet, the frictional force between the tyres and road reduces by 25%. How fast can the car safely take the turn on the wet road ?
Solution:
Let subscripts 1 and 2 denote the values of a quantity under dry and wet conditions, respectively.
Data : v₁ = 7 m/s, f₂ = f₁, – 0.25f₁ = 0.75f₁

On a dry horizontal curved road, the frictional force between the tyres and road is f₁ = µ₁mg, where m is the mass of the car and g is the gravitational acceleration.

The maximum safe speed for taking a turn of radius r on a dry horizontal curved road is

Question 5.
A coin kept at a distance of 5 cm from the centre of a turntable of radius 1.5 m just begins to slip when the turntable rotates at a speed of 90 rpm. Calculate the coefficient of static friction between the coin and the turntable. [g = π² m/s²]
Solution:
Data: r = 5 cm = 0.05 m, f = 90 rpm = \(\frac{90}{60}\) rps = 1.5 rps, g = π² m/s²

The centripetal force for the circular motion of the coin is provided by the friction between the coin and the turntable. The coin is just about to slip off the turntable when the limiting force of friction is equal to the centripetal force.

Question 6.
A thin cylindrical shell of inner radius 1.5 m rotates horizontally, about a vertical axis, at an angular speed ω. A wooden block rests against the inner surface and rotates with it. If the coefficient of static friction between block and surface is 0.3, how fast must the shell be rotating if the block is not to slip and fall ?

Solution :
Data : r = 1.5 m, µ_s = 0.3
The normal force \(\vec{N}\) of the shell on the block is the centripetal force which holds the block in place. \(\vec{N}\) determines the friction on the block, which in turn keeps it from sliding downward. If the block is not to slip, the friction force \(\overrightarrow{f_{\mathrm{s}}}\) must balance the weight \(m \vec{g}\) of the block.
∴ N = mω²r and f_s = μ_sN = mg
∴ μ_s(ω²r) = mg

This gives the required angular speed.

Question 7.
A motorcyclist rounds a curve of radius 25 m at 36 km/h. The combined mass of the motorcycle and the man is 150 kg.

1. What is the centripetal force exerted on the motorcyclist ?
2. What is the upward force exerted on the motorcyclist?

Solution :
Data : r = 25m, v = 36 km/h = 36 × \(\frac{5}{18}\)m/s = 10 m/s, m = 150 kg, g = 10 m/s²

1. Centripetal force, F = \(\frac{m v^{2}}{r}\) = \(\frac{150 \times(10)^{2}}{25}\) = 600 N
2. Upward force = normal reaction of the road surface = mg = 150 × 10 = 1500 N

Question 8.
A motorcyclist is describing a circle of radius 25 m at a speed of 5 m/s. Find his inclination with the vertical. What is the value of the coefficient of friction between the tyres and ground ?
Solution :
Data : v = 5 m/s, r = 25 m, g = 10 m/s²

Question 9.
A motor van weighing 4400 kg (i.e., a motor van of mass 4400 kg) rounds a level curve of radius 200 m on an unbanked road at 60 km/h. What should be the minimum value of the coefficient of friction to prevent skidding ? At what angle should the road be banked for this velocity?
Solution :
Data : m = 4400 kg, r = 200 m,
v = 60 km/h = 60 × \(\frac{5}{18}\)m/s = \(\frac{50}{3}\) m/s, g = 10 m/s²

[Note : In part (ii), v is to be taken as the optimum speed.]

Question 10.
An amusement park ride (known variously as the Rotor, the Turkish Twist and the Gravitron) consists of a large vertical cylinder that is spun about it axis fast enough such that the riders remain pinned against its inner wall. The floor drops away once the cylinder has attained its full rotational speed. The radius of the cylinder is R and the coefficient of static friction between a rider and the wall is μ_s.
(i) Show that the minimum angular speed necessary to keep a rider from falling is given by ω = \(\sqrt{g / \mu_{s} R} \text {. }\).
(ii) Obtain a numerical value for the frequency of rotation of the cylinder in rotations per minute if R =4 m and
µ_s = 0.4.
Solution:
Data: R = 4 m, µ_s = 0.4, g = 10 m/s²
The forces exerted on the rider, when the floor
drops away, are

1. the normal force \(\vec{N}\) exerted by the wall, directed radially inward, is the centripetal force
2. the upward frictional force \(\overrightarrow{f_{\mathrm{s}}}\) exerted by the wall
3. the downward gravitational force mg .

∴ N = mω²R and f_s = µ_sN = µ_s (mω²R) where m is the mass of the rider and ω is the angular speed of the Rotor cylinder. For the rider not to fall, \(\overrightarrow{f_{\mathrm{s}}}\) must balances \(m \vec{g}\).

This is the minimum angular speed necessary. Since ω = 2πf, the corresponding frequency of rotation of the cylinder is

Question 11.
The two rails of a broad-gauge railway track are 1.68 m apart. At a circular curve of radius 1.6 km, the outer rail is raised relative to the inner rail by 8.4 cm. Find the angle of banking of the track and the optimum speed of a train rounding the curve.
Solution :
Data : l = 1.68 m = 168 cm, r = 1.6 km = 1600 m, h = 8.4 cm, g = 10 m/s²

Question 12.
A metre gauge train is moving at 72 kmph along a curved railway track of radius of curvature 500 m. Find the elevation of the outer rail above the inner rail so that there is no side thrust on the outer rail.
Solution :
Data : r = 500 m, v = 72 kmph = 72 × \(\frac{5}{18}\) m/s = 20 m/s, g = 10 m/s², l = 1 m
tan θ = \(\frac{v^{2}}{r g}\)
= \(\frac{(20)^{2}}{500 \times 10}\) = 0.08
The required angle of banking,
θ = tan^-1 (0.08) = 4°4′
The elevation of the outer rail relative to the inner rail,
h = l sin θ
= (1)(sin 4°4′) = 0.0709 m = 7.09 cm

Question 13.
A circular race course track has a radius of 500 m and is banked at 10°. The coefficient of static friction between the tyres of a vehicle and the road surface is 0.25. Compute
(i) the maximum speed to avoid slipping
(ii) the optimum speed to avoid wear and tear of the tyres.
Solution :
Data : r = 500 m, θ = 10°, µ_s = 0.25, g = 9.8 m/s², tan 10° = 0.1763

(i) On the banked track, the maximum speed of the vehicle without slipping (skidding) is

(ii) The optimum speed of the vehicle on the track is

Question 48.
Define a conical pendulum.
Answer:
A conical pendulum is a small bob suspended from a string and set in UCM in a horizontal plane with the centre of its circular path below the point of suspension such that the string makes a constant angle θ with the vertical.
OR
A conical pendulum is a simple pendulum whose bob revolves in a horizontal circle with constant speed such that the string describes the surface of an imaginary right circular cone.

Question 49.
Derive an expression for the angular speed of the bob of a conical pendulum.
OR
Derive an expression for the frequency of revolution of the bob of a corical pendulum.
Answer:
Consider a conical pendulum of string length L with its bob of mass m performing UCM along a circular path of radius r.
At every instant of its motion, the bob is acted upon by its weight \(m \vec{g}\) and the tension \(\vec{F}\) in the string. If the constant angular speed of the bob is ω, the necessary horizontal centripetal force is F_c = mω²r

F_c is the resultant of the tension in the string and the weight. Resolve \(\vec{F}\) into components F cos θ vertically opposite to the weight of the bob and F sin θ horizontal. F cos θ balances the weight. F sin θ is the necessary centripetal force.
∴ F sin θ = mω²r … (1)
and F cos θ = mg … (2)

is the required expression for ω.
[Note : From Eq. (4), cos θ = g/ω²L. Therefore, as ω increases, cos θ decreases and θ increases.
If n is the frequency of revolution of the bob,

is the required expression for the frequency.

Question 50.
What will happen to the angular speed of a conical pendulum if its length is increased from 0.5 m to 2 m, keeping other conditions the same?
Answer:
The angular speed of the conical pendulum will become half the original angular speed.

Question 51.
Write an expression for the time period of a conical pendulum. State how the period depends on the various factors.
Answer:
If T is the time period of a conical pendulum of string length L which makes a constant angle θ with the vertical,
T = 2π\(\sqrt{\frac{L \cos \theta}{g}}\)
is the required expression
(Note: L cos θ = OC = h, where h is the axial height of the cone.
∴ T = 2π\(\sqrt{\frac{h}{g}}\)

where g is the acceleration due to gravity at the place.

From the above expression, we can see that

1. T ∝ \(\sqrt{L}\)
2. T ∝ \(\sqrt{\cos \theta}\) if θ increases, cos θ and T decrease
3. T ∝ \(\frac{1}{\sqrt{g}}/latex]
4. The period is independent of the mass of the bob.

Question 52.
Solve the following :

Question 1.
A stone of mass 2 kg is whirled in a horizontal circle attached at the end of a 1.5 m long string. If the string makes an angle of 30° with the vertical, compute its period.
Solution :
Data : L = 1.5 m, θ = 30°, g = 10 m/s²
The period of the conical pendulum,

Question 2.
A string of length 0.5 m carries a bob of mass 0. 1 kg at its end. If this is to be used as a conical pendulum of period 0.4πs, calculate the angle of inclination of the string with the vertical and the tension in the string.
Solution :
Data : L = 0.5m, m = 0.1 kg, T = 0.4πs, g = 10 m/s²

Question 3.
In a conical pendulum, a string of length 120 cm is fixed at a rigid support and carries a bob of mass 150 g at its free end. If the bob is revolved in a horizontal circle of radius 0.2m around a vertical axis, calculate the tension in the string. [g = 9.8 m/s²]
Solution:
Data : L = 120 cm = 1.2 m, m = 150 g = 0.15 kg,
r = 0.2 m, g = 9.8 m/s²

Question 4.
A stone of mass 1 kg, attached at the end of a 1 m long string, is whirled in a horizontal circle. If the string makes an angle of 30° with the vertical, calculate the centripetal force acting on the stone.
Solution :
Data : m = 1 kg, L = 1 m, θ = 30°, g = 10 m/s²

Question 53.
What is vertical circular motion? Comment on its two types.
Answer:
A body revolving in a vertical circle in the gravitational field of the Earth is said to perform vertical circular motion.

A vertical circular motion controlled only by gravity is a nonuniform circular motion because the linear speed of the body does not remain constant although the motion can be periodic.

In a controlled vertical circular motion, such as that a body attached to a rod, the linear speed of the body can be constant (including zero) so that such a motion can be uniform and periodic.

Question 54.
A body, tied to a string, performs circular motion in a vertical plane such that the tension in the string is zero at the highest point. What is the linear speed of the body at the

1. lowest position
2. highest position ?

Answer:

1. [latex]\sqrt{5 r g}\)
2. \(\sqrt{r g}\) in the usual notation.

Question 55.
A body, tied to a string, performs circular motion in a vertical plane such that the tension in the string is zero at the highest point. What is the angular speed of the body at the

1. highest position
2. lowest position ?

Answer:

1. \(\sqrt{g / r}\)
2. \(\sqrt{5 g / r}\) in the usual notation.

Question 56.
In a vertical circular motion controlled by gravity, derive an expression for the speed at an arbitrary position. Hence, show that the speed decreases while going up and increases while coming down.
OR
In a nonuniform vertical circular motion, derive expressions for the speed and tension/normal force at an arbitrary position.
OR
Show that a vertical circular motion controlled by gravity is a non uniform circular motion.
Answer:
Consider a small body of mass m tied to a string and revolved in a vertical circle of radius r. At every instant of its motion, the body is acted upon by its weight \(m \vec{g}\) and the tension \(\vec{T}\) in the string. At any instant, when the body is at the position P, let the string make an angle θ with the vertical, \(m \vec{g}\) is resolved into components, mg cos θ (radial) and mg sin θ (tangential).

At point P shown, the net force on the body towards the centre, T-mg cos θ, is the necessary centripetal force on the body. If v is its speed at P,


Let v₂ be the speed of the body at the lowest point B, which is the reference level for zero potential energy. Then, the body has only kinetic energy

As the body goes from B to P, it rises through a height h = r – r cos θ = r(1 – cos θ).
Total energy at P = KE + PE

Assuming that the total energy of the body is conserved, total energy at any point = total energy at the bottom.
Then, from Eqs. (2) and (3),

From the above expression, it can be seen that the linear speed v changes with θ. Thus, as θ increases, (while going up) cos θ decreases, 1 – cos θ increases, and v decreases. While coming down, θ decreases and v increases. Hence, a vertical circular motion controlled by gravity is a nonuniform circular motion.

Substituting for v² from Eq. (4) in Eq. (1),

Equation (6) is the expression for the tension in the string at any instant in terms of the speed at the lowest point.

Question 57.
A body at the end of a rod is revolved in a non-uniform vertical circular motion. Show that
(i) it must have a minimum speed 2\(\sqrt{g r}\) at the bottom
(ii) the difference in tensions in the rod at the highest and lowest positions is 6 mg.
Answer:
Consider a body of mass m attached to a rod and revolved in a vertical circle of radius r at a place where the acceleration due to gravity is g. We shall assume that the rod is not rigid so that the tension in the rod changes. As the rod is rotated in a nonuniform circular motion, the tension in the rod changes from a minimum value T₁ when the body is at the highest point to a maximum value T₂ when the body is at the bottom of the circle. At every instant, the body is acted upon by two forces, namely/its weight \(m \vec{g}\) and the tension \(\vec{T}\) in the string.

Question 58.
You may have seen in a circus a motorcyclist driving in vertical loops inside a hollow globe (sphere of death). Explain clearly why the motor-cyclist does not fall down when at the highest point of the chamber.
Answer:
A motorcyclist driving in vertical loops inside a hollow globe performs vertical circular motion. Suppose the mass of the motorcycle and motorcyclist is m and the radius of the chamber is r. At every instant of the motion, the motorcyclist is acted upon by the weight \(m \vec{g}\) and the normal reaction \(\vec{N}\).

At the highest point, let v₁ be the speed and \(\vec{N}_{1}\) the normal reaction. Here, both \(\vec{N}_{1}\) and \(m \vec{g}\) are parallel, vertically downward. Hence, the net force on the motorcyclist towards the centre O is N₁ + mg. If this force is able to provide the necessary centripetal force at the highest point, the motorcycle does not lose contact with the globe and fall down.

The minimum value of this force is found from the limiting case when N, just becomes zero and the weight alone provides the necessary centripetal force :
\(\frac{m v_{1}^{2}}{r}\) = mg
This requires that the motorcycle has a minimum speed at the highest point given by \(v_{1}^{2}\) = gr or v₁ = \(\sqrt{g r}\)

[Note : The ‘globe of death’ is a circus stunt in which stunt drivers ride motorcycles inside a mesh globe. Starting from small horizontal circles, they eventually perform revolutions along vertical circles. The linear speed is more for larger circles but angular speed is more for smaller circles as in conical pendulum.]

Question 59.
A car crosses over a bridge which is in the form of a convex arc with a uniform speed,
(i) State the expression for the normal reaction on the car.
OR
How does the normal reaction on the car vary with speed?
(ii) Hence show that the maximum speed with which the car can cross the bridge without losing contact with the road is equal to \(\sqrt{r g}\).
Answer:
Suppose a car of mass m, travelling with a uniform speed v, crosses over a bridge which is in the form of a convex arc of radius r.
(i) The forces acting on it at the highest point are as shown in below figure. Their resultant mg-N provides the centripetal force.

is the required expression. It shows that as v increases, N decreases.

(ii) Equation (1) shows that for g – \(\frac{v^{2}}{r}\) = 0, i.e., for centripetal acceleration equalling the gravitational acceleration, N = 0. That is, for \(\frac{v^{2}}{r}\) = g or v = \(\sqrt{r g}\), the
car just loses contact with the road. Therefore, this is the maximum speed with which a car can cross the bridge, irrespective of its mass.

[Data : Take g = 10 m/s² unless specified otherwise]

Question 60.
Solve the following :

Question 1.
An object of mass 1 kg tied to one end of a string of length 9 m is whirled in a vertical circle. What is the minimum speed required at the lowest position to complete the circle ? [g = 9.8 m/s²]
Solution :
Data : m = 1 kg, r = 9 m, g = 9.8 m/s²
The minimum speed of the object at the lowest position is

Question 2.
A stone of mass 5 kg, tied at one end of a rope of length 0.8 m, is whirled in a vertical circle. Find the minimum velocity at the highest point and at the midway point, [g = 9.8 m/s²]
Solution:
Data : m = 5 kg, r = l = 0.8 m, g = 9.8 m/s²

1. The minimum velocity of the stone at the highest point in its path,
   v = \(\sqrt{r g}\) = \(\sqrt{0.8 \times 9.8}\) = 2.8 m/s
2. The minimum velocity of the stone at the midway point in its path,
   v = \(\sqrt{3 r g}\) = \(\sqrt{3 \times 0.8 \times 9.8}\) = 4.85 m/s

Question 3.
A small body of mass 0.3 kg oscillates in a vertical plane with the help of a string 0.5 m long with a constant speed of 2 m/s. It makes an angle of 60° with the vertical. Calculate the tension in the string.
Solution :
Data : m = 0.3 kg, r = 0.5 m, v = 2 m/s, θ = 60°, g = 10 m/s²

Question 4.
A bucket of water is whirled in a vertical circle at an arm’s length. Find the minimum speed at the top so that no water spills out. Also find the corresponding angular speed. [Assume r = 0.75 m]
Solution :
Data : r = 0.75 m, g = 10 m/s²
At the highest point the minimum speed required is v = \(\sqrt{r g}\) = \(\sqrt{0.75 \times 10}\) = 2.738 m/s
The corresponding angular speed is 2.738
ω = \(\frac{v}{r}\) = \(\frac{2.738}{0.75}\) = 3.651 rad/s

Question 5.
A pendulum, with a bob of mass m and string length l, is held in the horizontal position and then released into a vertical circle. Show that at the lowest position the velocity of the bob is \(\sqrt{2 g l}\) and the tension in the string is 3 mg.
Solution :
Taking the reference level for zero potential energy to be the bottom of the vertical circle, the initial potential energy of the bob at the horizontal position = mgh = mgl.

Hence, at the bottom where the speed of the bob is v, it has only kinetic energy = \(\frac{1}{2}\)mv².

This gives the required velocity at the lowest position.
Also, at the bottom, the tension (T) and the centripetal acceleration are upward while the force of gravity is downward.

Equations (1) and (2) give the required expressions for the velocity and tension at the lowest position.

Question 6.
A stone of mass 100 g attached to a string of length 50 cm is whirled in a vertical circle by giving it a velocity of 7 m/s at the lowest point. Find the velocity at the highest point.
Solution :
Data : m = 0.1 kg, r = l = 0.5 m, v₂ = 7 m/s, g = 10 m/s²
The total energy at the bottom, E_bot
= KE + PE = \(\frac{1}{2} m v_{2}^{2}\) + 0 = \(\frac{1}{2}\)(0.1) (7)² = 2.45 J
The total energy at the top, E_top = KE + PE = \(\frac{1}{2} m v_{1}^{2}\) + mg (2r)
= \(\frac{1}{2}\)(0.1)\(v_{1}^{2}\) + (0.1) (10) (2 × 0.5)
= 0.05\(v_{1}^{2}\) + 1
By the principle of conservation of energy,

Question 7.
A pilot of mass 50 kg in a jet aircraft executes a “loop-the-loop” manoeuvre at a constant speed of 250 m/s. If the radius of the vertical circle is 5 km, compute the force exerted by the seat on the pilot at
(i) the top of the loop
(ii) the bottom of the loop.
Solution :
Data: m = 50 kg, v = 250m/s, r = 5 km = 5 × 10³ m, g = 10 m/s²

(i) At the top of the loop : The forces on the pilot are the gravitational force \(m \vec{g}\) and the normal force \(\vec{N}_{1}\), exerted by the seat, both acting downward. So the net force downward that causes the centripetal acceleration has a magnitude

(ii) At the bottom of the loop : The forces on the pilot are the downward gravitational force \(m \vec{g}\) and the upward normal force \(\vec{N}_{2}\) exerted by the seat. So the net upward force that causes the centripetal acceleration has a magnitude N₂ – mg.

The forces exerted by the seat on the pilot at the top and bottom of the loop are 125 N and 1125 N, respectively.

Question 8.
A ball released from a height h along an incline, slides along a circular track of radius R (at the end of the incline) without falling vertically downwards. Show that h_min = \(\frac{5}{2}\) R.
Solution:
To just loop-the-loop, the ball must have a speed v₂ = \(\sqrt{5 R g}\) at the bottom of the circular track.

If h_min is the minimum height above the bottom of the circular track from which the ball must be released, by the principle of conservation of energy, we have,
mgh_min = \(\frac{1}{2} m v_{2}^{2}\) = \(\frac{1}{2} m(5 R g)\)

Note : 1f the ball rolls all along the track without slipping, its total energy at the top of the circular track should take into account the rotational kinetic energy of the ball.

Question 9.
A block of mass 1 kg is released from P on a frictionless track which ends with a vertical quarter circular turn.

What are the magnitudes of the radial acceleration and total acceleration of the block when it arrives at Q ?
Solution :
Data : m = 1 kg, h = 6 m, r = 2 m, g = 10 m/s²
Let v be the speed of the block at Question Then, the total energy of the block at Q is
E = KE + PE = \(\frac{1}{2} m v^{2}\) + mgr
By the principle of conservation of energy,

The radial acceleration has a magnitude 40 m/s². The total acceleration has a magnitude 41.23 m/s² and makes an angle of 14°2′ with the radial acceleration.

Question 10.
A loop-the-loop cart runs down an incline into a vertical circular track of radius 3 m and then describes a complete circle. Find the minimum height above the top of the circular track from which the cart must be released.
Solution :

Data : r = 3 m
To just loop-the-loop, the cart must have a speed V₁ = \(\sqrt{r g}\) at the top of the loop.

If h is the minimum height above the top of the loop from which the cart must be released, by the principle of conservation of energy, we have, mgh = \(\frac{1}{2} m v_{1}^{2}\) = \(\frac{1}{2} m g r\)
∴ h = \(\frac{r}{2}\) = \(\frac{3}{2}\) = 1.5 m

Question 11.
A motorcyclist rides in vertical circles in a hollow sphere of radius 5 m. Find the required minimum speed and minimum angular speed, so that he does not lose contact with the sphere at the highest point. [g = 9.8 m/s²]
Solution :
Data : r = 5 m, g = 9.8 m/s²
Let v and ω be respectively the required minimum speed and angular speed at the highest point.

Question 12.
The vertical section of a road over a bridge in the direction of its length is in the form of an arc of a circle of radius 4.4 m. Find the maximum speed with which a vehicle can cross the bridge without losing contact with the road at the highest point, if the centre of gravity of the vehicle is 0.5 m from the ground.
Solution :
Data: While travelling along the bridge, the vehicle moves along a vertical circle of radius r = 4.4 + 0.5 = 4.9 m, g = 10 m/s².
If m is the mass and v is the maximum speed of the vehicle, then at the highest point,

Question 13.
A small body tied to a string is revolved in a vertical circle of radius r such that its speed at the top of the circle is \(\sqrt{2 g r}\). Find
(i) the angular position of the string when the tension in the string is numerically equal to 5 times the weight of the body.
(ii) the KE of the body at this position
(iii) the minimum and maximum KEs of the body.
[Take m = 0.1 kg, r = 1.2 m, g = 10 m/s²]
Solution :
Data : v_top = \(\sqrt{2 g r}\), T = 5 mg, m = 0.1 kg, r = 1.2 m, g = 10 m/s²

Let the angular position of the string, θ = 0° when the body is at the bottom of the circle.

We assume total energy to be conserved and take the reference level for zero potential energy to be the bottom of the circle.

Total energy at the top, E

At P, the vertical displacement of the body from the bottom is r(1 – cos θ). Its total energy there is also E.

Question 14.
An object of mass 0.5 kg attached to a rod of length 0.5 m is whirled in a vertical circle at a constant angular speed. If the maximum tension in the rod is 5 kg wt, calculate the linear speed of the object and the maximum number of revolutions it can complete in a minute.
Solution :
Data : m = 0.5 kg, r = l = 0.5 m, g = 10 m/s²,
T₂ = 5 kg wt = 5 × 10 N

As the rod is rotated in a vertical circle at a constant angular speed, the linear speed of the object at the end of the rod is constant, say v. However, the tension in the rod changes from a minimum value T₁ when the object is at the highest point to a maximum value T₂ when the object is at the bottom of the circle.

At the bottom of the circle, the tension and acceleration are upward while the force of gravity is downward.

∴ The maximum number of revolutions the object can complete in a minute is 127.98.

Question 15.
A small body of mass m = 0.1 kg at the end of a cord 1 m long swings in a vertical circle. Its speed is 2 mIs when the cord makes an angle θ = 30° with the vertical. Find the tension in the cord.
Solution:
Data: m = 0.1 kg, r = 1 m, y = 2 m/s, θ = 30°,
g = 9.8 m/s²
When the cord makes an angle θ with the vertical, the centripetal force on the body is

Question 16.
A bucket of water is tied to one end of a rope 8 m long and rotated about the other end in a vertical circle. Find the number of revolutions per minute such that water does not spill.
Solution:
[Important note : The circular motion of the bucket in a vertical plane under gravity is not a uniform circular motion. Assuming the critical case of the motion such that the bucket has the minimum speed at the highest point required for the water to stay put in the bucket, we can find the minimum frequency of revolution. ]
Data :r = 8m, g = 9.8 m/s², π = 3.142
Assuming the bucket has a minimum speed v = \(\sqrt{r g}\) at the highest point, the corresponding angular speed is

Question 61.
Derive an expression for the kinetic energy of a body rotating with constant angular velocity.
Answer:
Consider a rigid body rotating with a constant angular velocity \(\vec{\omega}\) about an axis passing through the point O and perpendicular to the plane of the figure. Suppose that the body is made up of N particles of masses m₁, m₂, …, m_N situated at perpendicular distances r₁, r₂, , r_N, respectively, from the axis of rotation as shown in below figure.

As the body rotates, all the particles perform uniform circular motion with the same angular velocity \(\vec{\omega}\). However, they have different linear speeds depending upon their distances from the axis of rotation.

The linear speed of the particle with mass ml is v₁ = r₁ω. Therefore, its kinetic energy is

where I = \(\sum_{i=1}^{N} m_{i} r_{i}^{2}\) is the moment of inertia of the body about the axis of rotation.
Equation (2) gives the required expression.

Question 62.
Define moment of inertia. State the factors which it depends on. Obtain its dimensions and state its SI unit.
OR
Define moment of inertia. State its dimensions and SI units.
Answer:
(1) Moment of inertia : The moment of inertia of a body about a given axis of rotation is defined as the sum of the products of the masses of the particles of the body and the squares of their respective distances from the axis of rotation.

If the body is made up of N discrete particles of masses m₁, m₂, …,m_N situated at respective distances r₁, r₂, …, r_N from the axis of rotation, the moment of inertia of the body is

For a rigid body, having a continuous and uniform distribution of mass, the moment of inertia is
I = \(\int r^{2} d m\) …(2)
where dm is the mass of an infinitesimal element, situated at distance r from the axis of rotation.

(2) The moment of inertia of a rigid body depends on

1. the mass and shape of the body
2. orientation and position of the rotation axis
3. distribution of the mass about the rotation axis.

(3) Dimensions :
[Moment of inertia] = [mass] [distance]²
= [M] [L²] = [M¹L²T⁰]

(4) SI unit : The kilogram-metre² (kg.m²).

Question 63.
Explain the physical significance of moment of inertia.
Answer:
(1) The physical significance of moment of inertia can be understood by comparing the formulae in the following table.

Linear motion	Rotational motion
1. Momentum = mass × velocity	1. Angular momentum = moment of inertia × angular velocity
2. Force = mass × acceleration	2. Torque = moment of inertia × angular acceleration
3. Kinetic energy = \(\frac{1}{2} M v^{2}\)	3. Kinetic energy = \(\frac{1}{2} I \omega^{2}\)

(2) Force produces acceleration, while torque produces angular acceleration. Force and torque are analogous quantities. Also, momentum and angular momentum are analogous quantities.
(3) By comparing the above formulae, we find that moment of inertia plays the same role in rotational motion as that played by mass in linear motion. The moment of inertia of a body is its rotational inertia, that which opposes any tendency to change its angular velocity. In the absence of a net torque, the body continues to rotate with a uniform angular velocity.

Question 64.
Three point masses M₁, M₂ and M₃ are located at the vertices of an equilateral triangle of side a. What is the moment of inertia of the system about an axis along the altitude of the triangle passing through M₁ ?
Answer:

The moment of inertia of the system about the altitude passing through M₁ is

Question 65.
Find the moment of inertia of a hydrogen molecule about its centre of mass if the mass of each hydrogen atom is m and the distance between them is R.
Answer:
We assume the rotation axis to be a transverse axis through the centre of mass of the linear molecule H₂. Then, each of the hydrogen atom is a distance \(\frac{1}{2}\)R from the CM. Therefore, the MI of the molecule about this axis,

Notes :

1. For a H₂ molecule, mH = 1.674 × 10^-27 kg and bond length = 7.774 × 10^-11 m, so that I = 5.065 × 10^-48 kg.m².
2. As atoms are treated as particles, we do not consider rotation about the line passing through the atoms.

Question 66.
Solve the following :

Question 1.
Four particles of masses 0.2 kg, 0.3 kg, 0.4 kg and 0.5 kg respectively are kept at comers A, B, C and D of a square ABCD of side 1 m. Find the moment of inertia of the system about an axis passing through point A and perpendicular to the plane of the square.
Solution :
Data : m₁ = 0.2 kg, m₂ = 0.3 kg, m₃ = 0.4 kg, m₄ = 0.5 kg

The axis of rotation passes through point A and is perpendicular to the plane of the square. Hence the distance (r₁) of mass ml from the axis is r₁ =0, that of mass m₂ is r₂ = AB = 1 m, that of mass m₃ is r₃ = \(\sqrt{2}\)AC m and that of mass m₄ is r₄ = AD = 1 m.
The moment of inertia,

Question 2.
The moment of inertia of the Earth about its axis of rotation is 9.83 × 10 kg.m² and its angular speed is 7.27 × 10^-5 rad/s. Calculate its
(i) kinetic energy of rotation
(ii) radius of gyration. [ Mass of the Earth = 6 × 10²⁴ kg]
Solution :
Data : I = 9.83 × 10³⁷ kg.m², ω = 7.27 × 10^-5 rad/s, M = 6 × 10²⁴ kg

(i) The kinetic energy of rotation of the Earth,

Question 67.
State an expression for the moment of inertia of a thin ring about its transverse symmetry axis.
Answer:
A thin uniform ring (or hoop) has all its mass uniformly distributed along the circumference of a circle. It is taken to be a two-dimensional body. It is also assumed that the radial thickness of the ring is so small as to be completely negligible in comparison to its radius.

Consider a thin ring (or hoop) of radius R and mass M. The axis of rotation through its centre C is perpendicular to its plane. C is also its centre of mass (CM).

The MI of the ring about the transverse symmetry axis is
I_CM = MR²

Question 68.
Derive an expression for the moment of inertia of a thin uniform disc about its transverse symmetry axis.
Answer:
A thin uniform disc has all its mass homogeneously distributed over its circular surface area. It is taken to be a two-dimensional body, i.e., its axial thickness is small as to be completely negligible in comparison to its radius. Consider a thin disc of radius R and mass M. Its mass per unit area is

The axis of rotation is the transverse symmetry axis, through its centre of mass (CM) and perpendicular to its plane. For rotation about this axis, we consider the disc to consist of a large number of thin concentric rings, having the same rotation axis as the transverse symmetry axis of the disc. One such elemental ring at a distance r from the rotation axis shown in below figure, has mass dm and radial width dr.

Since the disc is uniform, the area and mass of this elemental ring are

and its moment of inertia (MI) about the given axis is dm.r².
Therefore, the MI of the disc is

This gives the required expression.

Question 69.
Is radius of gyration of a rigid body a constant quantity?
Answer:
Radius of gyration of a rigid body depends on the distribution of mass of the body about a rotation axis and, therefore, changes with the choice of the rotation axis. Hence, unlike the mass of the body which is constant, radius of gyration and moment of inertia of the body are not constant.

Question 70.
State an expression for the radius of gyration of
(i) a thin ring
(ii) a thin disc, about respective transverse symmetry axis.
OR
Show that for rotation about respective transverse symmetry axis, the radius of gyration of a thin disc is less than that of a thin ring.
Answer:
(i) The MI of the ring about the transverse symmetry axis is
I_CM = MR² … (1)
Radius of gyration : The radius of gyration of the ring about the transverse symmetry axis is
K = \(\sqrt{I_{\mathrm{CM}} / M}\) = \(\sqrt{R^{2}}\) = R …… (2)

(ii) The MI of the disc about the transverse symmetry axis is
I_CM = \(\frac{1}{2}\)MR<² … (3)
Radius of gyration : The radius of gyration of the disc for the given rotation axis is

Question 71.
State and prove the theorem of parallel axis.
Answer:
Theorem of parallel axis : The moment of inertia of a body about an axis is equal to the sum of

1. its moment of inertia about a parallel axis through its centre of mass and
2. the product of the mass of the body and the square of the distance between the two axes.

Proof : Let I_CM be the moment of inertia (MI) of a body of mass M about an axis through its centre of mass C, and I be its MI about a parallel axis through any point O. Let h be the distance between the two axes.

Consider an infinitesimal mass element dm of the body at a point P. It is at a perpendicular distance CP from the rotation axis through C and a perpendicular distance OP from the parallel axis through O. The MI of the element about the axis through C is CP²dm. Therefore, the MI of the body about the axis through the CM is I_CM = \(\int \mathrm{CP}^{2} d m\). Similarly, the MI of the body about the parallel axis through O is I = \(\int \mathrm{OP}^{2} d m\).

∴ I = I_CM + Mh²
This proves the theorem of parallel axis.

Question 72.
State and prove the theorem of perpendicular axes about moment of inertia.
Answer:
Theorem of perpendicular axes : The moment of inertia of a plane lamina about an axis perpendicular to its plane is equal to the sum of its moments of inertia about two mutually perpendicular axes in its plane and through the point of intersection of the perpendicular axis and the lamina.

Proof : Let Ox and Oy be two perpendicular axes in the plane of the lamina and Oz, an axis perpendicular to its plane. Consider an infinitesimal mass element dm of the lamina at the point P(Y, y). MI of the lamina about the z-axis, I_z = \(\int \mathrm{OP}^{2} d m\)

The element is at perpendicular distance y and x from the x- and y- axes respectively. Hence, the moments of inertia of the lamina about the x- and y-axes are, respectively,

This proves the theorem of perpendicular axes.

Question 73.
About which axis of rotation is the radius of gyration of a body the least ?
Answer:
The radius of gyration of a body is the least about an axis through the centre of mass (CM) of the body.

From the parallel axis theorem, we know that a given body has the smallest possible moment of inertia about an axis through its CM. The radius of gyration of a body about a given axis is directly proportional to the square root of its moment of inertia about that axis. Hence, the conclusion.
{OR I = I_CM + Mh². ∴ Mk² = \([/latexM k_{\mathrm{CM}}^{2}] + Mh².
∴ k² = [latex]k_{\mathrm{CM}}^{2}\) + h², which shows that k is minimum, equal to k_CM when h = 0.}

Question 74.
State an expression for the moment of inertia of a thin uniform rod about an axis through its centre and perpendicular to its length. Hence deduce the expression for its moment of inertia about an axis through its one end and perpendicular to its length.
OR
State an expression for the moment of inertia of a thin uniform rod about its transverse symmetry axis. Hence, deduce the expression for its moment of inertia about a parallel axis through one end. Also deduce the expressions for the corresponding radii of gyration.
Answer:
(1) MI about a transverse axis through centre : Consider a thin uniform rod AB of mass M and length L, rotating about a transverse axis through its centre C. C is also its centre of mass (CM).

(2) MI about a transverse axis through one end : Let I be its MI about a transverse axis through its end A. By the theorem of parallel axis,
I = I_CM + Mh² … (2)
In this case,

(3) Radii of gyration : The radius of gyration of the rod about its transverse symmetry axis is

The radius of gyration of the rod about the transverse axis through an end is

Question 75.
State the expression for the MI of a thin spherical shell (i.e., a thin-walled hollow sphere) about its diameter. Hence obtain the expression for its MI about a tangent.
Answer:
Consider a uniform, thin-walled hollow sphere radius R and mass M. An axis along its diameter is an axis of spherical symmetry through its centre of mass. The MI of the thin spherical shell about its diameter is
I_CM = \(\frac{2}{3}\)MR²

Let I be its MI about a tangent parallel to the diameter. Here, h = R = distance between the two axes. Then, according to the theorem of parallel axis,

Question 76.
Calculate the moment of inertia by direct integration of a thin uniform rod of mass M and length L about an axis perpendicular to the rod and passing through the ród at L/3, as shown below.
Check your answer with the parallel-axis theorem.

Answer:
Method of direct integration : Consider a thin uniform rod of mass M and length L. The axis of rotation is perpendicular to the rod and passing through the rod at L/3. We consider the origin of coordinates to be at this point and the x-axis to be along the rod,

Since the mass density is constant, the linear mass density is
λ = M/L
An element of the rod has mass dm and length dl = dx.

If the distance of each mass element from the axis is given by the variable x, the moment of inertia of an element about the axis of rotation is dI = x²dm
Since the rod extends from x= – L/3 to x = 2L/3, the MI of the rod about the axis is

Method of parallel-axis : The MI of the thin rod about a transverse axis through its CM is
I_CM = \(\frac{1}{12} M L^{2}\)
The given axis of rotation is at a distance h = \(\frac{L}{2}\) – \(\frac{L}{3}\) = \(\frac{L}{6}\) from the transverse symmetry axis.
Therefore, the MI of the rod about the given axis is

the same as arrived at by direct integration method.

Question 77.
State an expression for the moment of inertia of a thin ring about its transverse symmetry axis. Hence deduce the expression for its moment of inertia about a tangential axis perpendicular to its plane. Also deduce the expressions for the corresponding radius of gyration.
Answer:
(1) MI about the transverse symmetry axis : Consider a thin ring (or hoop) of radius R and mass M. The axis of rotation through its centre C is perpendicular to its plane. C is also its centre of mass (CM). It is assumed that the radial thickness of the ring is so small as to be completely negligible in comparison to radius R.

The MI of the ring about the transverse symmetry axis is
I_CM = MR² …(1)
Radius of gyration : The radius of gyration of the ring about the transverse symmetry axis is
k = \(\sqrt{I_{\mathrm{CM}} / M}\) = \(\sqrt{R^{2}}\) = R …(2)

(2) MI about a tangent perpendicular to its plane : Let I be its MI about a parallel axis, tangent to the ring. Here, h = R = distance between the two axes.
By the theorem of parallel axis,
I = I_CM + Mh² … (3)
= MR² + MR² = 2MR² …(4)
Radius of gyration : The radius of gyration of the ring about a transverse tangent is
k = \(\sqrt{I / M}\) = \(\sqrt{2 R^{2}}\) = \(\sqrt{2} R\) …(5)

Question 78.
Assuming the expression for the moment of inertia of a ring about its transverse symmetry axis, obtain the expression for its moment of inertia about
(1) a diameter
(2) a tangential axis in its plane. Also deduce the expressions for the corresponding radii of gyration.
Answer:
Let M be the mass of a thin ring of radius R. Let /CM be the moment of inertia (MI) of the ring about its transverse symmetry axis. Then,
I_CM = MR … (1)

(1) MI about a diameter : Let x- and y-axes be along two perpendicular diameters of the ring as shown in below figure. Let I_x, I_y and I_z be the moments of inertia of the ring about the x, y and z axes, respectively.

Both I_x and I_y represent the moment of inertia of the ring about its diameter and, by symmetry, the MI of the ring about any diameter is the same.
∴ I_x = I_y ….. (2)
Also, I_z being the MI of the ring about its transverse symmetry axis,

(2) MI about a tangent in its plane: Let I be its MI about an axis in plane of the ring, i.e., parallel to a diameter, and tangent to it. Here, h = R and
I_CM = I_x = \(\frac{1}{2}\)MR².
By the theorem of parallel axis,
= \(\frac{1}{2} M R^{2}\) + MR² = \(\frac{3}{2} M R^{2}\) … (7)

Question 79.
State an expression for the MI of a thin uniform disc about a transverse axis through its centre. Hence, derive an expression for the MI of the disc about its tangent perpendicular to the plane. Deduce the expressions for the corresponding radii of gyration.
Answer:
(1) MI about the transverse symmetry axis : Consider a thin uniform disc of radius R and mass M. The axis of rotation through its centre C is perpendicular to its plane. C is also its centre of mass (CM).

Radius of gyration : The radius of gyration of the disc for the given rotation axis is

(2) MI about a tangent perpendicular to its plane : Let I be the MI of the disc about a tangent perpendicular to its plane.

According to the theorem of parallel axis,

Question 80.
Assuming the expression for the moment of inertia of a thin uniform disc about a transverse axis through its centre, obtain an expression for its moment of inertia about any diameter. Hence, write the expression for the corresponding radius of gyration.
Answer:
Consider a thin uniform disc of mass M and radius R in the xy plane, as shown in below figure. Let I_x, I_y and I_z be the moments of inertia of the disc about the x, y and z axes respectively. But, I_x = I_y, since each

represents the moment of inertia (MI) of the disc about its diameter and, by symmetry, the MI of the disc about any diameter is the same.
As I_z is the MI of the disc about the z-axis through its centre and perpendicular to its plane,
I_z = \(\frac{1}{2}\)MR² … (1)

According to the theorem of perpendicular axes,

Question 81.
Given the moment of inertia of a thin uniform disc about its diameter to be \(\frac{1}{4}\)MR², where M and R are respectively the mass and radius of the disc, find its moment of inertia about an axis normal to the disc and passing through a point on its edge.
Answer:
Consider a thin uniform disc of mass M and radius R in the xy plane. Let Ix, ly and Iz be the moments of inertia of the disc about the x, y and z axes respectively.
Now, I_x = I_y
since each represents the moment of inertia (MI) of the disc about its diameter and, by symmetry, the MI of the disc about any diameter is the same.
∴ I_x = I_y = \(\frac{1}{4}\)MR² (Given)
According to the theorem of perpendicular axes,
I_z = I_x + I_y = 2(\(\frac{1}{4}\)MR²) = \(\frac{1}{2}\)MR²
Let I be the MI of the disc about a tangent normal to the disc and passing through a point on its edge (i.e., a tangent perpendicular to its plane). According to the theorem of parallel axis,
I = I_CM + Mh²
Here, I_CM = I_z = \(\frac{1}{2}\)MR² and h = R.
∴ I = \(\frac{1}{2}\)MR² + MR² = \(\frac{3}{2}\)MR²
which is the required expression.

Question 82.
Assuming the expression for the moment of inertia of a thin uniform disc about its diameter, show that the moment of inertia of the disc about a tangent in its plane is \(\frac{5}{4}\)MR². Write the expression for the corresponding radius of gyration.
Answer:
Let M be the mass and R be the radius of a thin uniform disc. Let I_CM be the moment of inertia (MI) of the disc about a diameter. Then,

Question 83.
State the expressions for the moment of inertia of a solid cylinder of uniform cross section about
(1) an axis through its centre and perpendicular to its length
(2) its own axis of symmetry.
OR
State the expressions for the MI of a solid cylinder about
(1) a transverse symmetry axis
(2) its cylindrical symmetry axis. Also deduce the expressions for the corresponding radii of gyration.
Answer:
Consider a solid cylinder of uniform density, length L, radius R and total mass M.

Notes :

1. For R « L, a solid cylinder can be approximated as a thin rod, and the expression for the MI about its transverse symmetry axis reduces to the corresponding expression for a thin rod, viz., ML²/12.
2. The MI of a solid cylinder about its cylindrical symmetry axis is the same as that of a disc about its transverse symmetry axis and having the same mass and radius.

Question 84.
Assuming the expression for the moment of inertia of a uniform solid cylinder about a transverse symmetry axis, obtain the expression for its moment of inertia about a transverse axis through its one end.
Answer:
Let M be the mass, L the length and R the radius of a uniform solid cylinder. Let I_CM be the moment of inertia (MI) of the cylinder about a transverse symmetry axis. Then,

Question 85.
State an expression for the moment of inertia of a solid sphere about its diameter. Write the expression for the corresponding radius of gyration.
Answer:
Consider a solid sphere of uniform density, radius R and mass M. An axis along its diameter is an axis of spherical symmetry through its centre of mass.

The MI of the solid sphere about its diameter is
I_CM = \(\frac{2}{5}\)MR²
The corresponding radius of gyration is

Question 86.
A uniform solid sphere of mass 15 kg has radius 0.1 m. What is its moment of inertia about a diameter?
Answer:
Moment of inertia of the sphere about a diameter
= \(\frac{2}{5}\)MR² = \(\frac{2}{5}\) × 15 × (0.1)² = 6 × 10^-2 kg.m²

Question 87.
Assuming the expression for the MI of a uniform solid sphere about its diameter, obtain the expression for its moment of inertia about a tangent.
Answer:
Let M be the mass of a uniform solid sphere of radius R. Let I_CM be its MI about any diameter.

Let I be its MI about a parallel axis, tangent to the sphere. Here, h = R = distance between the two axis.
By the theorem of parallel axis, I = I_CM + Mh²
= \(\frac{2}{5}\)MR² + MR² = \(\frac{7}{5}\)MR²

Question 88.
The moment of inertia of a uniform solid sphere about a diameter is 2 kg m². What is its moment of inertia about a tangent ?
Answer:
Moment of inertia of a solid sphere about its 2
diameter, I_CM = \(\frac{2}{5}\)MR².

Question 89.
The radius of gyration of a uniform solid sphere of radius R is \(\sqrt{\frac{2}{5}}\)R for rotation about its diameter. Show that its radius of gyration for rotation about a tangential axis of rotation is \(\sqrt{\frac{7}{5}}\)R.
Answer:
Let the mass of the uniform solid sphere of radius R be M. Let I_CM and k_d be its MI about any diameter and the corresponding radius of gyration, respectively. Then,

Let I and k_t be its MI about a parallel tangential axis and the corresponding radius of gyration, respectively. Here, h = R = distance between the two axis.
∴ I = \(M k_{\mathrm{t}}^{2}\)
By the theorem of parallel axis,
I = I_CM + Mh²

Question 90.
State the expression for the MI of a thin spherical shell (i.e., a thin-walled hollow sphere) about its diameter. Hence obtain the expression for its MI about a tangent.
Answer:
Consider a uniform, thin-walled hollow sphere radius R and mass M. An axis along its diameter is an axis of spherical symmetry through its centre of mass. The MI of the thin spherical shell about its diameter is
I_CM = \(\frac{2}{3} M R^{2}\)

Let I be its MI about a tangent parallel to the diameter. Here, h = R = distance between the two axes. Then, according to the theorem of parallel axis,

Question 91.
Find the ratio of the radius of gyration of a solid sphere about its diameter to the radius of gyration of a hollow sphere about its tangent, given that both the spheres have the same radius.
Answer:
The radius of gyration of a body about a given axis, k = \(\sqrt{I / M}\), where M and I are respectively the mass of the body and its moment of inertia (MI) about the axis.

For a solid sphere rotating about its diameter,

Question 92.
Calculate the moment of inertia by direct integration of a thin uniform rectangular plate of mass M, length l and breadth b about an axis passing through its centre and parallel to its breadth.
Answer:
Consider a thin uniform rectangular plate of mass M, length l and breadth b. The axis of rotation passes through its centre and is parallel to its breadth. We consider the origin of coordinates to be at the centre of the plate and orient the axes as shown in below figure. Since the plate is thin, we can take the mass as distributed entirely in the xy-plane. Then, the surface mass density is constant and equal to

A rectangular element of the plate, shown shaded, has mass dm, length b and breadth dy.
∴ dm = σdA = σ(b dy)

If the distance of each element from the rotation axis is given by the variable y, the moment of inertia of :
an element about the axis of rotation is
dI_x = y²dm
Since the rod extends from y = -1/2 to y = 1/2, the MI of the thin plate about the axis is

Notes:

(1) The MI of a thin rectangular plate about an axis passing through its centre and parallel to its length (i.e., about the y-axis) is I_y = \(\frac{1}{12}\)Mb². Then, by the theorem of perpendicular axes, the MI of a thin plate about its transverse symmetry axis (i.e., about the z-axis) is I_z = I_x + I_y = \(\frac{1}{12} M\left(l^{2}+b^{2}\right)\)

(2) Suppose, for a rectangular bar of sides l, b and w, we take the origin of coordinates at the centre of mass of the bar and the x, y and z axes parallel to the respective sides. Then, I_x = \(\frac{1}{12}\)M(b² + w²), I_y = \(\frac{1}{12}\)M(w² + l²) and I_z = \(\frac{1}{2} M\left(l^{2}+b^{2}\right)\)

Question 93.
State the MI of a thin rectangular plate-of mass M, length l and breadth b- about an axis passing through its centre and parallel to its length. Hence find its MI about a parallel axis along one edge.
Answer:
Consider a thin rectangular plate of mass M, length l and breadth b. The MI of the plate about an axis passing through its centre and parallel to its edge of length l is

For a parallel axis along its one edge, h = \(\frac{1}{2} b\).
Therefore, by the theorem of parallel axis, the MI about this axis is

Question 94.
State the MI of a thin rectangular plate-of mass M, length l and breadth b about its transverse axis passing through its centre. Hence find its MI about a parallel axis through the midpoint of edge of length b.
Answer:
Consider a thin rectangular plate of mass M, length l and breadth b. The MI of the plate about its transverse axis passing through its centre is
I_CM = M(l² + b²)
For a parallel axis through the midpoint of its breadth, h = \(\frac{1}{2} l\). Therefore, by the theorem of parallel axis, the MI about this axis is

Question 95.
A uniform solid right circular cone of base radius R has mass M. Prove that the moment of inertia of the cone about its central symmetry axis is \(\frac{3}{10} M R^{2}\).
Answer:
Consider a uniform solid right circular cone of mass M, base radius R and height h. The axis of rotation passes through its centre and the vertex, Its constant mass density is

We consider an elemental disc of mass dm, radius r and thickness dz. If the distance of each mass element from the axis is given by the variable z,

Question 96.
Solve the following :

Question 1.
Calculate the moment of inertia of a ring of mass 500 g and radius 0.5 m about an axis of rotation passing through
(i) its diameter
(ii) a tangent perpendicular to its plane.
Solution :
Data : M = 500 g 0.5 kg, R = 0.5 m

(i) The moment of inertia of the ring about its

(ii) The moment of inertia of the ring about a tangent perpendicular to its plane
= 2MR² = 2 × 0.5 × (0.5)² = 0.25 kg.m²

Question 2.
A thin uniform rod 1 m long has mass 1 kg. Find its moment of inertia and radius of gyration for rotation about a transverse axis through a point midway between its centre and one end.
Solution :
Data : M = 1 kg, L = 1 m
Let I_CM and I be the moments of inertia of the rod about a transverse axis through its centre, and a parallel axis midway between its centre and one end.

Question 3.
The moment of inertia of a disc about an axis through its centre and perpendicular to its plane is 10 kg.m². Find its MI about a diameter.
Solution :
Data : I_z = 10 kg.m²
If the disc lies in the xy plane with its centre at the origin then, according to the theorem of perpendicular axes,
I_x + I_y = I_z
Since, I_x = I_y, 2I_x = I_z
∴ Its MI about a diameter,
I_x = \(\frac{I_{z}}{2}\) = \(\frac{10}{2}\) = 5 kg.m²

Question 4.
A solid cylinder of uniform density and radius 2 cm has a mass of 50 g. If its length is 12 cm, calculate its moment of inertia about an axis passing through its centre and perpendicular to its length.
Solution :
Data : M = 50 g, R = 2 cm, L = 12 cm

Question 5.
A compound object is formed of a thin rod and a disc attached at the end of the rod. The rod is 0.5 m long and has mass 2 kg. The disc has mass of 1 kg and its radius is 20 cm. Find the moment of inertia of the compound object about an axis passing through the free end of the rod and perpendicular to its length.
Solution :
Data : L = 0.5 m, R = 0.2 m, M_rod = 2 kg, M_disk = 1 kg About a transverse axis through CM,

Question 6.
The radius of gyration of a body about an axis at 6 cm from its centre of mass is 10 cm. Find its radius of gyration about a parallel axis through its centre of mass.
Solution :
Let O be a point at 6 cm from the centre of mass of the body.
Let I = MI about an axis through O,
k = radius of gyration about the axis through O,
I_CM = MI about a parallel axis through the centre of mass of the body,
k_CM = radius of gyration about a parallel axis through the centre of mass,
M = mass of the body,
h = distance between the two axes.
Data : h = 6 cm, k = 10 cm
By the theorem of parallel axis,
I = I_CM + Mh²
Also, I = Mk² and I_CM = \(M k_{\mathrm{CM}}^{2}\)

The radius of gyration about a parallel axis through its centre of mass is 8 cm.

Question 7.
The radius of gyration of a disc about its transverse symmetry axis is 2 cm. Determine its radius of gyration about a diameter.
Solution :
Data : k_CM = 2 cm
Let M and R be the mass and radius of the disc. Let I_CM and k_CM be the MI and radius of gyration of the disc about its transverse symmetry axis. Let I and k be the MI and radius of gyration of the disc about its diameter. Then

Question 8.
Calculate the MI and rotational kinetic energy of a thin uniform rod of mass 10 g and length 60 cm when it rotates about a transverse axis through its centre at 90 rpm.
Solution :
Data : M = 10 g = 10^-2 kg, L = 60 cm = 0.6 m,
f = 90 rpm = 90/60 Hz = 1.5 Hz
The MI of the rod about a transverse axis through its centre is

Angular speed, ω = 2πf = 2 × 3.142 × 1.5 = 9.426 rad/s
Rotational KE = \(\frac{1}{2}\)Iω² = \(\frac{1}{2}\left(3 \times 10^{-4}\right)(9.426)^{2}\)
= 0.01333 J

Question 9.
A thin rod of uniform cross section is made up of two sections made of wood and steel. The wooden section has length 50 cm and mass 0.6 kg. The steel section has length 30 cm and mass 3 kg. Find the moment of inertia of the rod about a transverse axis passing through the junction of the two sections.
Solution:
Data : L₁ =0.5m, M₁ =0.6 kg, L₂ = 0.3m,
The moment of inertia of a thin rod about a transverse axis through its end is \(\frac{M L^{2}}{3}\).

Question 10.
The mass and the radius of the Moon are, respectively, about \(\frac{1}{81}\) time and about \(\frac{1}{3.7}\) time those of the Earth. Given that the rotational period of the Moon is 27.3 days, compare the rotational kinetic energy of the Earth with that of the Moon.
Solution :
Data : M_M = \(\frac{1}{81}\)M_E, R_M = \(\frac{1}{3.7}\)R_E, T_M = 27.3 days, T_E = 1 day

Let I_E and I_M be the moments of inertia of the Earth and the Moon about their respective axes of rotation, and ω_E and ω_M be their respective rotational angular speeds. Assuming the Earth and the Moon to be solid spheres of uniform densities,

Question 11.
A solid sphere of radius R, rotating with an angular velocity ω about its diameter, suddenly stops rotating and 75% of its KE is converted into heat. If c is the specific heat capacity of the material in SI units, show that the temperature of 3R²CO²
the sphere rises by \(\frac{3 R^{2} \omega^{2}}{20 c}\).
Answer:
The MI of a solid sphere about its diameters, I = \(\frac{2}{5}\)MR²
where M is its mass.
The rotational KE of the sphere,

If ∆θ is the rise in temperature,

Question 97.
Define the angular momentum of a particle.
Answer:
Definition : The angular momentum of a particle is defined as the moment of the linear momentum of the particle. If a particle of mass m has linear momentum \(\vec{p}(=m \vec{v})\)), then the angular momentum of this particle with respect to a point O is a vector quantity defined as \(\vec{l}=\vec{r} \times \vec{p}=m(\vec{r} \times \vec{v})\)), where \(\vec{r}\) is the position vector of the particle with respect to O.

It is the angular analogue of linear momentum.

[Note : As the particle moves relative to O in the direction of its momentum \(\vec{p}(=m \vec{v})\), position vector \(\vec{r}\) rotates around O. However, to have angular momentum about O, the particle does not itself have to rotate around O.]

Question 98.
State the dimensions and SI unit of angular momentum.
Answer:

1. Dimensions : [Angular momentum] = [M¹L²T^-1]
2. SI unit: The kilogram.metre²/second (kg.m²/s).

Question 99.
Express the kinetic energy of a rotating body in terms of its angular momentum.
Answer:
The kinetic energy of a body of moment of inertia I and rotating with a constant angular velocity ω is
E = \(\frac{1}{2} I \omega^{2}\)
The angular momentum of the body, L = Iω.
∴E = \(\frac{1}{2}(I \omega) \omega=\frac{1}{2} L \omega\)
This is the required relation.

Question 100.
Why do grinding wheels have large mass and moderate diameter?
Answer:
A grinding wheel, used for abrasive machining operations (e.g., sharpening), is typically in the form of a heavy disc of moderate diameter. A grinding machine needs to have a high frequency of revolution but the machining operations exert braking torques on its wheel.

Angular momentum is directly proportional to mass. Hence, heavier the wheel, the greater is its angular momentum and lesser is the decelerating effect of the braking torques. Also, angular acceleration is inversely proportional to the moment of inertia. Since the wheel is made heavy, its diameter is kept moderate so that a large angular acceleration and high angular velocity can be achieved with a motor of given power.

Question 101.
Solve the following :

Question 1.
The angular momentum of a body changes by 80 kg.m²/s when its angular velocity changes from 20 rad/s to 40 rad/s. Find the change in its kinetic energy of rotation.
Solution :
Data : ω₁ = 20 rad/s, ω₂ = 40 rad/s
If I is the MI of the body, its initial angular momentum is Iω₁, and final angular momentum is Iω₂.
Change in angular momentum
= Iω₂ – Iω₁(ω₂ – ω₁)
∴ 80 = I(40 – 20)
∴ I = 4 kg.m²

Question 2.
A wheel of moment of inertia 1 kg.m² is rotating at a speed of 40 rad/s. Due to the friction on the axis, the wheel comes to rest in 10 minutes. Calculate the angular momentum of the wheel, two minutes before it comes to rest.
Solution :
Data : I = 1 kg.m², ω₁ = 40 rad/s, ω₂ = 0 at
t = 10 minutes = 60 × 10 s = 600 s,
t’ = 8 minutes = 60 × 8 s = 480 s

This is the required angular momentum of the wheel.

Question 3.
A flywheel rotating about an axis through its centre and perpendicular to its plane loses 100 J of energy on slowing down from 60 rpm to 30 rpm. Find its moment of inertia about the given axis and the change in its angular momentum.
Solution :
Data : f₁ = 60 rpm = 60/60 rot/s = 1 rot/s, f₂ = 30 rpm = 30/60 rot/s = \(\frac{1}{2}\) rot/s, ∆E = – 100 J

This gives the MI of the flywheel about the given axis.

(ii) Angular momentum, L = Iω = I(2πf) 2πIf
The change in angular momentum, ∆L
= L₂ – L₁ = 2πI(f₂ – f₁)
= 2 × 3.142 × 6.753\(\left(\frac{1}{2}-1\right)\)
= -3.142 × 6.753= -21.22 kg.m²/s

Question 102.
A torque of 4 N-m acting on a body of mass 1 kg produces an angular acceleration of 2 rad/s². What is the moment of inertia of the body?
Answer:
The moment of inertia of the body.

Question 103.
Two identical rings are to be rotated about different axes of rotation as shown by applying torques so as to produce the same angular acceleration in both. How is it possible ?

Answer:
The MI of ring 1 about a transverse tangent is I₁ = 2MR²
The MI of ring 2 about its diameter is
I₂ = \(\frac{1}{2}\)MR²
Since, torque T = \(\tau=I \alpha\), to produce the same angular acceleration in both,

∴ It will be possible to produce the same angular acceleration in both the rings only if \(\tau_{1}=4 \tau_{2}\).

Question 104.
Two wheels have the same mass. First wheel is in the form of a solid disc of radius R while the second is a disc with inner radius r and outer radius R. Both are rotating with same angular velocity ω₀ about transverse axes through their centres. If the first wheel comes to rest in time t₁ while the second comes to rest in time t₂, are t₁ and t₂ different? Why?
Answer:
The moments of inertia of the two wheels about transverse axes through their centres are

Question 105.
Solve the following :

Question 1.
A torque of magnitude 400 N-m, acting on a body of mass 40 kg, produces an angular acceleration of 20 rad/s². Calculate the moment of inertia and radius of gyration of the body.
Solution :
Data : T = 400 N.m, M = 40 kg, α = 20 rad/s²

Question 2.
A body starts rotating from rest. Due to a couple of 20 N.m, it completes 60 revolutions in one minute. Find the moment of inertia of the body.
Solution :

Question 3.
A wheel of moment of inertia 2 kg-m2 rotates at 50 rpm about its transverse axis. Find the torque that can stop the wheel in one minute.
Solution :
Data : I = 2 kg.m², f₀ = 50 rpm = \(\frac{50}{60}\) = \(\frac{5}{6}\) rev/s, t = 60 s

Question 4.
A circular disc of moment of inertia 10 kg.m² is rotated about its transverse symmetry axis at a constant frequency of 60 rpm by an electric motor of power 31.42 watts. When the motor is switched off, how many rotations does it complete before coming to rest?
Solution :
Data : I = 10 kg.m², P = 31.42 watts,
f = 60 rpm = 60/60 Hz = 1 Hz
In rotational motion,
power = torque × angular velocity

This torque provided by the motor overcomes the torque of the frictional forces and maintains a constant frequency of rotation.

When the motor is switched off, the disc slows down due to the retarding torque of the frictional forces.

Question 5.
A flywheel of mass 4 kg and radius 10 cm, rotating with a uniform angular velocity of 5 rad/s, is subjected to a torque of 0.01 N.m for 10 seconds.
If the torque increases the speed of rotation, find
(i) the final angular velocity of the flywheel
(ii) the change in its angular velocity
(iii) the change in its angular momentum
(iv) the change in its kinetic energy.
Solution :
Data : M = 4 kg, R = 10 cm = 0.1 m, ω₁ = 5 rad/s, \(\tau\) = 0.01 N.m, t = 10 s

(i) The final angular velocity of the flywheel,
ω₂ = ω₁ + αt
= 5 + 0.5 × 10 = 10 rad/s

(ii) The change in its angular velocity
= ω₂ – ω₁ = 5 rad/s

(iii) The change in its angular momentum
= Iω₂ – Iω₁ = I (ω₂ – ω₁)
= 0.02 × 5 = 0.1 kg.m²/s

(iv) The change in its kinetic energy

Question 6.
A torque of 20 N.m sets a stationary circular disc into rotation about a transverse axis through its centre and acts for 2π seconds. If the disc has a mass 10 kg and radius 0.2 m, what is its frequency of rotation after 2π seconds ?
Solution :
Data : \(\tau\) = 20 N.m, t = 2π s, M = 10 kg, R = 0.1 m Let f₁ and f₂ be the initial and final frequencies of rotation of the disc, and ω₁ and ω₂ be its initial and final angular speeds. Since the disc was initially stationary, f₁ = ω₁, = 0 and ω₂ = 2πf₂.
The MI of the disc about the given axis is

Now, ω₂ = ω₁ + αt = 0 + α t
∴ 2πf₂ = αt
∴ f₂ = \(\frac{\alpha t}{2 \pi}\) = \(\frac{100(2 \pi)}{2 \pi}\) = 100 Hz

Question 7.
A rope is wound around a hollow cylinder of mass 3 kg and radius 40 cm. If the rope is pulled downwards with a force of 30 N, find
(i) the angular acceleration of the cylinder
(ii) the linear acceleration of the rope.
Solution :
Data : M = 3 kg, R = 0.4 m, F = 30 N
(i) The MI of a hollow cylinder about its cylinder axis, I = MR²

(ii) The linear acceleration of the rope = the tangential acceleration at = αR = 25 × 0.4 = 10 m/s²

Question 106.
State and prove the principle (or law) of conservation of angular momentum.
Answer:
Principle (or law) of conservation of angular momentum : The angular momentum of a body is conserved if the resultant external torque on the body is zero.
Proof : Consider a moving particle of mass m whose position vector with respect to the origin at any instant is \(\vec{r}\).
Then, at this instant, the linear velocity of this particle is \(\vec{v}=\frac{\overrightarrow{d r}}{d t}\), its linear momentum is \(\vec{p}=m \vec{v}\) and its angular momentum about an axis through the origin is \(\vec{l}=\vec{r} \times \vec{p}\).

Suppose its angular momentum \(\vec{l}\) changes with time due to a torque \(\vec{\tau}\) exerted on the particle.

∴ \(\vec{l}\) = constant, i.e., \(\vec{l}\) is conserved. This proves the principle (or law) of conservation of angular momentum.

Alternate Proof : Consider a rigid body rotating with angular acceleration \(\vec{\alpha}\) about the axis of rotation. If I is the moment of inertia of the body about the axis of rotation, \(\vec{\omega}\) the angular velocity of the body at time t and \(\vec{L}\) the corresponding angular momentum of the body, then

∴ \(\vec{L}\) = constant, i.e., \(\vec{L}\) is conserved. This proves the principle (or law) of conservation of angular momentum.

Question 107.
What happens when a ballet dancer stretches her arms while taking turns?
Answer:
When a ballet dancer stretches her arms while pirouetting, her moment of inertia increases, and consequently her angular speed decreases to conserve angular momentum.

Question 108.
If the Earth suddenly shrinks so as to reduce its volume, mass remaining unchanged, what will be the effect on the duration of the day?
Answer:
If the Earth suddenly shrinks, mass remaining constant, the moment of inertia of the Earth will decrease, and consequently the angular velocity of rotation ω about its axis will increase. Since period \(T \propto \frac{1}{\omega}\), the duration of the day T will decrease.

Question 109.
Two discs of moments of inertia I₁ and I₂ about their transverse symmetry axes, respectively rotating with angular velocities to ω₁ and ω₂, are brought into contact with their rotation axes coincident. Find the angular velocity of the composite disc.
Answer:
We assume that the initial angular momenta (\(\vec{L}_{1}\) and \(\vec{L}_{2}\)) of the discs are either in the same direction or in opposite directions. Then,
the total initial angular momentum = \(\vec{L}_{1}+\vec{L}_{2}=I_{1} \overrightarrow{\omega_{1}}+I_{2} \overrightarrow{\omega_{2}}\)

After they are coupled, the total moment of inertia, i.e., the moment of inertia of the composite disc is I = I₁ + I₂ and the common angular velocity is \(\vec{\omega}\). Assuming conservation of angular momentum,

Question 110.
A boy standing at the centre of a turntable with his arms outstretched is set into rotation with angular speed ω rev/min. When the boy folds his arms back, his moment of inertia reduces to \(\frac{2}{5}\)th its initial value. Find the ratio of his final kinetic energy of rotation to his initial kinetic energy.
Answer:
Data : I₂ = \(\frac{2}{5}\)I₁
L = Iω
Assuming the angular momentum \(\vec{L}\) is conserved, in magnitude,

This gives the required ratio.

Question 111.
Name the quantity that is conserved when

1. \(\vec{F}_{\text {external }}\) is zero
2. \(\vec{\tau}_{\text {external }}\) is zero.

Answer:

1. Total linear momentum is conserved when \(\vec{F}_{\text {external }}\) is Zer0
2. Angular momentum is conserved when \(\vec{\tau}_{\text {external }}\) is zero.

Question 112.
What is the rotational analogue of the equation \(\vec{F}_{\text {external }}\) = \(\frac{d \vec{p}}{d t}\)?
Answer:
\(\vec{F}_{\text {external }}\) = \(\frac{d \vec{L}}{d t}\)

Question 113.
Fly wheels used in automobiles and steam engines producing rotational motion have discs with a large moment of inertia. Explain why?
Answer:
A flywheel is used as

(i) a mechanical energy storage, the energy being stored in the form of rotational kinetic energy

(ii) a direction and speed stabilizer. A flywheel rotor is typically in the form
of a disc. Rotational kinetic energy, \(E_{\mathrm{rot}}=\frac{1}{2} I \omega^{2}\), where I is the moment of inertia and ω is the angular speed. That is, E_rot ∝ I. Therefore, higher the moment of inertia, the higher is the rotational kinetic energy that can be stored or recovered.

Also, angular momentum, \(\vec{L}=I \vec{\omega}\), i.e., \(|\vec{L}| \propto I\). A torque aligned with the symmetry axis of a flywheel can change its angular velocity and thereby its angular momentum. A flywheel with a large angular momentum will require a greater torque to change its angular velocity. Thus, a flywheel can be used to stabilize direction and magnitude of its angular velocity by undesired torques.

Question 114.
Solve the following :

Question 1.
A uniform horizontal disc is freely rotating about a vertical axis passing through its centre at the rate of 180 rpm. A blob of wax of mass 1.9 g falls on it and sticks to it at 25 cm from the axis. If the frequency of rotation is reduced by 60 rpm, calculate the moment of inertia of the disc.
Solution :
Data : f₁ = 180 rpm = 180/60 rot/s = 3 rot/s, f₂ = (180 – 60) rpm = 120/60 rot/s = 2 rot/s, m = 1.9 g = 1.9 × 10^-3 kg, r = 25 cm = 0.25 m
Let I₁ be the MI of the disc. Let I₂ be the MI of the disc and the blob.

Question 2.
A horizontal disc is rotating about a transverse axis through its centre at 100 rpm. A 20 gram blob of wax falls on the disc and sticks to it at 5 cm from its axis. The moment of inertia of the disc about its axis passing through its centre is 2 × 10^-4 kg.m². Calculate the new frequency of rotation of the disc.
Solution :
Data : f₁ = 100 rpm, m = 20 g = 20 × 10^-3 kg, r = 5 cm = 5 × 10^-2 m, I₁ = I_disc = 2 × 10^-4 kg.m²
The MI of the disc and blob of wax is
I₂ = I₁ + mr²

This is the new frequency of rotation.

Question 3.
A ballet dancer spins about a vertical axis at 2.5 π rad/s with his arms outstretched. With the arms folded, the MI about the same axis of rotation changes by 25%. Calculate the new speed of rotation in rpm.
Solution:
Let I₁, ω₁, and f₁, be the moment of inertia, angular velocity and frequency of rotation of the ballet dancer with arms outstretched, and I₂, ω₂ and f₂ be the corresponding quantities with arms folded.
Data : ω₁ = 2.5 π rad /s
Since moment of inertia with arms folded is less than that with arms outstretched,

Question 4.
Two wheels, each of moment of inertia 4 kg.m², rotate side by side at the rate of 120 rpm and 240 rpm in opposite directions. If both the wheels are coupled by a weightless shaft so that they now rotate with a common angular speed, find this new rate of rotation.
Solution :
Data : I = 4 kg.m², f₁ = 120 rpm, f₂ 240 rpm
Initially, the angular velocities of the two wheels \(\overrightarrow{\omega_{1}}\), and \(\overrightarrow{\omega_{2}}\)) and, therefore, their angular momenta (\(\vec{L}_{1}\) and \(\vec{L}_{2}\)) are in opposite directions.

The magnitude of the total initial angular momentum
= – L₁ + L₂ = -Iω₁ + Iω₂ (∵ I₁ = I₂ = I)
= 2πl(f₂ – f₁) … (1)

After coupling onto the same shaft, the total moment of inertia is 21. Let ω = 2πf be the common angular speed.

The magnitude of the total final angular momentum = 2I.ω = 4πl.f … (2)

From Eqs. (1) and (2), by the principle of conservation of angular momentum,
4πf = 2πl(f₂ – f₁)
∴ f = \(\frac{f_{2}-f_{1}}{2}\) = \(\frac{240-120}{2}\) = 60 rpm
This gives their new rate of rotation.

Question 5.
A homogeneous (uniform) rod XY of length L and mass M is pivoted at the centre C such that it can rotate freely in a vertical plane. Initially, the rod is horizontal. A blob of wax of the same mass M as that of the rod falls vertically with speed V and sticks to the rod midway between points C and Y. As a result, the rod rotates with angular speed ω. What will be the angular speed in terms of V and L?
Solution :
The initial angular momentum of the rod is zero.
The initial angular momentum of the falling blob of wax about the point C is (in magnitude)
= mass × speed × perpendicular distance between its direction of motion and point C
= MV.\(\frac{L}{4}\)
The total initial angular momentum of the rod and blob of wax = \(\frac{M V L}{4}\) … (1)

After the blob of wax sticks to the rod, and the system rotates with an angular speed ω about the horizontal axis through point C perpendicular to the plane of the figure, the total final angular momentum of the system about this axis

This gives the required angular speed.

Question 6.
A satellite moves around the Earth in an elliptical orbit such that at perigee (closest approach) it is two Earth radii above the Earth’s surface. At apogee (farthest position), it travels with one-fourth the speed it has at perigee. In terms of the Earth’s radius R, what is the maximum distance of the satellite from the Earth’s surface ?
Solution:
Let r_p and r_a be the distances of the satellite from the centre of the Earth at perigee and apogee, respectively. Let v_p and v_a be its linear (tangential) velocities at perigee and apogee.
Data : r_p = 2R + R = 3R, v_a = \(\frac{1}{4}\)v_p

Let L_p and L_a be the angular momenta of the satellite about the Earth’s centre. Because the gravitational force (\(\vec{F}\)) on the satellite due to the Earth is always radially towards the centre of the Earth, its direction is opposite to that of the position vector (\(\vec{r}\)) of the satellite relative to the centre of the Earth, so that the torque \(\vec{\tau}=\vec{r} \times \vec{F}=0\). Hence, the angular momentum of the satellite about the Earth’s centre is constant in time.

At apogee, the distance of the satellite from the Earth’s surface is 12R – R = 11R.

Question 7.
A torque of 100 N.m is applied to a body capable of rotating about a given axis. If the body starts from rest and acquires kinetic energy of 10000 J in 10 seconds, find
(i) its moment of inertia about the given axis
(ii) its angular momentum at the end of 10 seconds.
Solution :
Data : \(\tau\) = 100 N.m, ω_i = 0, E_i = 0, E_f = 10⁴J, t = 10 s

Since the body starts from rest, its initial angular momentum, L_i = 0.
The final angular momentum,
L_f = τ∆t = (100)(10) = 10³ kg.m²/s
The final rotational kinetic energy, E_f = \(\frac{1}{2} L_{\mathrm{f}} \omega_{\mathrm{f}}\)

Question 8.
Two identical metal beads, each of mass M but negligible width, can slide along a thin smooth uniform horizontal rod of mass M and length L. The rod is capable of rotating about a vertical axis passing through its centre. Initially, the beads are almost touching the axis of rotation and the rod is rotating at speed of 14 rad/s. Find the angular speed of the system when the beads have moved up to the ends of the rod. (Assume that no external torque acts on the system.)
Solution :
Data : ω₁ = 14 rad/s
The MI of the rod about a transverse axis through its CM,
I_rod = \(\frac{M L^{2}}{12}\)
Since the beads are almost particle-like, and initially touching the rotation axis, their MI about the vertical axis is taken to be zero.
When the beads move upto the ends of the rod, r = L/2, their MI about the vertical axis is

Question 115.
Discuss how pure rolling (i.e., rolling without slipping) on a plane surface is a combined translational and rotational motion.
Answer:
Rolling motion (without slipping) is an important case of combined translation and rotation. Consider a circularly symmetric rigid body, like a wheel or a disc, rolling on a plane surface with friction along a straight path.

The centre of mass of the wheel is at its geometric centre O. For purely translational motion (the wheel sliding smoothly along the surface without rotating at all), every point on the wheel has the same linear velocity \(\vec{v}_{\mathrm{CM}}\) = \(\vec{v}_{\mathrm{O}}\) as the centre O. For purely rotational motion (as if the horizontal rotation axis through O were stationary), every point on the wheel rotates about the axis with angular velocity \(\vec{\omega}\); in this case, every point on the rim has the same linear speed ωR.

We view the combined motion in the inertial frame of reference in which the surface is at rest. In this frame, since there is no slipping, the point of contact of the wheel with the surface is instantaneously stationary, v_A = 0, so that the wheel is turning about an instantaneous axis through the point of contact A. The instantaneous linear speed of point C (at the top) is V_C = ω(2R) – faster than any other point of the wheel.

Question 116.
Deduce an expression for the kinetic energy of a body rolling on a plane surface without slipping.
OR
Obtain an expression for the total kinetic energy of a rolling body in the form \(\frac{1}{2} M v^{2}\left[1+\frac{k^{2}}{R^{2}}\right]\)
OR
Derive an expression for the kinetic energy when a rigid body is rolling on a horizontal surface without slipping. Hence, find the kinetic energy of a solid sphere.
Answer:
Consider a symmetric rigid body, like a sphere or a wheel or a disc, rolling on a plane surface with friction along a straight path. Its centre of mass (CM) moves in a straight line and, if the frictional force on the body is large enough, the body rolls without slipping. Thus, the rolling motion of the body can be treated as translation of the CM and rotation about an axis through the CM. Hence, the kinetic energy of a rolling body is
E = E_tran + E_rot ….. (1)
where E_tran and E_rot are the kinetic energies associated with translation of the CM and rotation about an axis through the CM, respectively.

Let M and R be the mass and radius of the body. Let ω, k and I be the angular speed, radius of gyration and moment of inertia for rotation about an axis through its centre, and v be the translational speed of the centre of mass.

Equation (4) or (5) or (6) gives the required expression.

Question 117.
A uniform solid sphere of mass 10 kg rolls on a horizontal surface. If its linear speed is 2 m/s, what is its total kinetic energy?
Answer:
Total kinetic energy of the sphere
= \(\frac{7}{10}\)Mv² = \(\frac{7}{10}\) × 10 × (2)² = 28 J

Question 118.
A disc of mass 4 kg rolls on a horizontal surface. If its linear speed is 3 m/s, what is its total kinetic energy?
Answer:
Total kinetic energy of the disc
= \(\frac{3}{4}\)Mv² = \(\frac{3}{4}\) × 4 × (3)² = 27 J

Question 119.
Assuming the expression for the kinetic energy of a body rolling on a plane surface without slipping, deduce the expression for the total kinetic energy of rolling motion for
(i) a ring
(ii) a disk
(iii) a hollow sphere. Also, find the ratio of rotational kinetic energy to total kinetic energy for each body.
Answer:
For a body of mass M and radius of gyration k, rolling on a plane surface without slipping with speed v, its total KE and rotational KE are respectively

[Note : The moment of inertia of all the round bodies above can be expressed as I = βMR², where β is a pure number less than or equal to 1. β is equal to 1 for a ring or a thin-walled hollow cylinder, \(\frac{1}{2}\) for a disc or solid cylinder, \(\frac{2}{3}\) for a hollow sphere and \(\frac{2}{5}\) for a solid sphere.
All uniform rings or hollow cylinders of the same mass and moving with the same speed have the same total kinetic energy, even if their radii are different. All discs or solid cylinders of the same mass and moving with the same speed have the same total kinetic energy; all solid spheres of the same mass and moving with the same speed have the same total kinetic energy. Also, for the same mass and speed, bodies with small c have less total kinetic energy.

Question 120.
State the expression for the speed of a circularly symmetric body rolling without slipping down an inclined plane. Hence deduce the expressions for the speed of
(i) a ring
(ii) a solid cylinder
(iii) a hollow sphere
(iv) a solid sphere, having the same radii.
Answer:
Consider a circularly symmetric body, of mass M and radius of gyration k, starting from rest on an inclined plane and rolling down without slipping. Its speed after rolling down through a height h is

[Note : If the inclined plane is ‘smooth’, i.e., there is no friction, the bodies will slide along the plane without any rotation. They will then have only translational kinetic energy, undergo equal acceleration and all three would arrive at the bottom at the same time with the same speed.]

Question 121.
State with reason if the statement is true or false : A wheel moving down a perfectly frictionless inclined plane will undergo slipping (not rolling) motion.
Answer:
The statement is true.
Explanation : Rolling on a surface (horizontal or inclined) without slipping may be viewed as pure rotation about an horizontal axis through the point of contact, when viewed in the inertial frame of reference in which the surface is at rest. The point of contact of the wheel with the surface will be instantaneously at rest, resulting in a rolling motion, provided the wheel is able to ‘grip’ the surface, i.e., friction is necessary. With little or no friction, the wheel will slip at the point of contact. On an inclined plane, this will result in pure translation along the plane. On a horizontal surface, the wheel will simply rotate about its axis through the centre without translation.

Question 122.
A ring and a disc roll down an inclined plane through the same height. Compare their speeds at the bottom of the plane.
Answer:

Question 123.
State the expression for the acceleration of a circularly symmetric rigid body rolling without slipping down an inclined plane. Hence, deduce the acceleration of
(i) a ring
(ii) a solid cylinder
(iii) a hollow cylinder
(iv) a solid sphere, rolling without slipping down an inclined plane.
Answer:
A circularly symmetric rigid body, of radius R and radius of gyration k, on rolling down an inclined plane of inclination θ has an acceleration

Question 124.
A spherical shell rolls down a plane inclined at 30° to the horizontal. What is its acceleration ?
Answer:
The acceleration of the spherical shell,
a = \(\frac{3}{5} g \sin \theta\) = 0.6g sin 30° = 0.6g × \(\frac{1}{2}\) = 0.3g m

Question 125.
A spherical shell and a uniform solid sphere roll down the same inclined plane. Compare their accelerations.
Answer:
The ratio of the accelerations, in the usual notation,

Question 126.
A solid sphere, starting from rest, rolls down two different inclined planes from the same height but with different angles of inclination θ₁ > θ₂. On which plane will the sphere take longer time to roll down?
Answer:
Let L₁ and L₂ be the distances rolled down by the sphere along the corresponding inclines from the same height h.
∴ L₁ sin θ₁ = L₂ sin θ₂ = h

The sphere will take longer time to roll down from the same height on the plane with smaller inclination.

Question 127.
Two circular discs A and B, having the same mass, have four identical small circular discs placed on them, as shown in the diagram. They are simultaneously released from rest at the top of an inclined plane. If the discs roll down without slipping, which disc will reach the bottom first?

Answer:
The disc A has the smaller discs closer to the centre than disc B. Hence, the moment of inertia of disc A (I_A) is less than that of disc B (I_B).

[Suppose the larger discs have radius R, the smaller discs have mass m and radius r, and the centre of each smaller disc on disc A is at a distance x from the centre. Then, x = \(\sqrt{2} r\)r and, it can be shown that, I_B – I_A = 4m[R² – (x – r)²] > 0.]

Each composite disc is equivalent to a disc of the same radius R and mass M’ = M + 4m, where m is the mass of each smaller disc, but of different thicknesses.

Suppose, starting from rest, the composite discs roll down the same distance L along a plane inclined at an angle θ, their respective accelerations will be

i.e., the disc A will reach the bottom first.

Question 128.
Solve the following :

Question 1.
A lawn roller of mass 80 kg, radius 0.3 m and moment of inertia 3.6 kg.m², is drawn along a level surface at a constant speed of 1.8 m/s. Find
(i) the translational kinetic energy
(ii) the rotational kinetic energy
(iii) the total kinetic energy of the roller.
Answer:
Data : M = 80 kg, R = 0.3 m, I = 3.6 kg.m², v = 1.8 m/s
(i) The translational kinetic energy of the centre of mass of the roller,

Question 2.
A solid sphere of mass 1 kg rolls on a table with linear speed 2 m/s, find its total kinetic energy.
Solution :
Data : M = 1 kg, v = 2 m/s
The total kinetic energy of a rolling body,

Question 3.
A ring and a disc having the same mass roll on a horizontal surface without slipping with the same linear velocity. If the total KE of the ring is 8 J, what is the total KE of the disc?
Solution :
Data : M_ring = M_disc = M, v_ring = v_disc = v, E_ring = 8J
The total kinetic energies of rolling without slipping on a horizontal surface,
E_ring = Mv² and E_disc = \(\frac{3}{4} M v^{2}\)
since they have the same mass and linear velocity.
∴ E_disc = \(\frac{3}{4}\)E_ring = \(\frac{3}{4}\) × 8 = 6J

Question 4.
A solid cylinder, of mass 2 kg and radius 0.1 m, rolls down an inclined plane of height 3 m. Calculate its rotational energy when it reaches the foot of the plane.
Solution :
Data : M = 2 kg, R = 0.1 m, h = 3 m, g = 10 m/s²

Question 5.
A solid sphere rolls up a plane inclined at 45° to the horizontal. If the speed of its centre of mass at the bottom of the plane is 5 m/s, find how far the sphere travels up the plane.
Solution :
Data : v = 5 m/s, θ = 45°, g = 9.8 m/s²
The total energy of the sphere at the bottom of the plane is
E = \(\frac{7}{10} M v^{2}\)
where M is the mass of the sphere.
In rolling up the incline through a vertical height h, it travels a distance L along the plane. Then,

The sphere travels 2.526 m up the plane.

Question 129.
Choose the correct option:

Question 1.
The bulging of the Earth at the equator and flattening at the poles is due to
(A) centripetal force
(B) centrifugal force
(C) gravitational force
(D) electrostatic force.
Answer:
(B) centrifugal force

Question 2.
A body of mass 0.4 kg is revolved in a horizontal circle of radius 5 m. If it performs 120 rpm, the centripetal force acting on it is
(A) 4π² N
(B) 8π² N
(C) 16π² N
(D) 32π² N.
Answer:
(D) 32π² N.

Question 3.
Two particles with their masses in the ratio 2 : 3 perform uniform circular motion with orbital radii in the ratio 3 : 2. If the centripetal force acting on them is the same, the ratio of their speeds is
(A) 4 : 9
(B) 1 : 1
(C) 3 : 2
(D) 9 : 4.
Answer:
(C) 3 : 2

Question 4.
When a motorcyclist takes a circular turn on a level race track, the centripetal force is
(A) the resultant of the normal reaction and frictional force
(B) the horizontal component of the normal reaction
(C) the frictional force between the tyres and road
(D) the vertical component of the normal reaction.
Answer:
(C) the frictional force between the tyres and road

Question 5.
The maximum speed with which a car can be driven safely along a curved road of radius 17.32 m and banked at 30° with the horizontal is [g = 10 m/s²]
(A) 5 m/s
(B) 10 m/s
(C) 15 m/s
(D) 20 m/s.
Answer:
(B) 10 m/s

Question 6.
A track for a certain motor sport event is in the form of a circle and banked at an angle 6. For a car driven in a circle of radius r along the track at the optimum speed, the periodic time is

Answer:
(C) \(2 \pi \sqrt{\frac{r}{g \tan \theta}}\)

Question 7.
The period of a conical pendulum in terms of its length (l), semivertical angle (θ) and acceleration due to gravity (g), is

Answer:
(C) \(4 \pi \sqrt{\frac{l \cos \theta}{4 g}}\)

Question 8.
A conical pendulum of string length L and bob of mass m performs UCM along a circular path of radius r. The tension in the string is

Answer:
(A) \(\frac{m g L}{\sqrt{L^{2}-r^{2}}}\)

Question 9.
The centripetal acceleration of the bob of a conical pendulum is

Answer:
(D) \(\frac{r g}{L \cos \theta}\)

Question 10.
A small object tied at the end of a string is to be whirled in a vertical circle of radius r. If its speed at the lowest point is \(2 \sqrt{g r}\), then
(A) the string will be slack at the lowest point
(B) it will not reach the midway point
(C) its speed at the highest point will be \(\sqrt{g r}\)
(D) it will just reach the highest point with zero speed.
Answer:
(D) it will just reach the highest point with zero speed.

Question 11.
A small bob of mass m is tied to a string and revolved in a vertical circle of radius r. If its speed at the highest point is \(\sqrt{3 r g}\), the tension in the string at the lowest point is
(A) 5 mg
(B) 6 mg
(C) 7 mg
(D) 8 mg.
Answer:
(D) 8 mg.

Question 12.
A small object, tied at the end of a string of length r, is launched into a vertical circle with a speed \(2 \sqrt{g r}\) at the lowest point. Its speed when the string is horizontal is
(A) > \(3 \sqrt{g r}\)
(B) = \(3 \sqrt{g r}\)
(C) = \(2 \sqrt{g r}\)
(D) 0.
Answer:
(C) = \(2 \sqrt{g r}\)

Question 13.
Two bodies with moments of inertia I₁ and I₂ (I₁ > I₂) rotate with the same angular momentum. If E₁ and E₂ are their rotational kinetic energies, then
(A) E₂ > E₁
(B) E₂ = E₁
(C) E₂ < E₁
(D) E₂ ≤ E₁
Answer:
(A) E₂ > E₁

Question 14.
The radius of gyration k for a rigid body about a given rotation axis is given by

Answer:
(B) \(k^{2}=\frac{1}{M} \int r^{2} d m\)

Question 15.
Three point masses m, 2m and 3m are located at the three vertices of an equilateral triangle of side l. The moment of inertia of the system of particles about an axis perpendicular to their plane and equidistant from the vertices is
(A) 2ml²
(B) 3ml²
(C) \(2 \sqrt{3}\) ml²
(D) 6ml²
Answer:
(A) 2ml²

Question 16.
The moment of inertia of a thin uniform rod of mass M and length L, about an axis passing through a point midway between the centre and one end, and perpendicular to its length, is
(A) \(\frac{48}{7}\)ML²
(B) \(\frac{7}{48}\)ML²
(C) \(\frac{1}{48}\)ML²
(D) \(\frac{1}{16}\)ML²
Answer:
(B) \(\frac{7}{48}\)ML²

Question 17.
A thin uniform rod of mass M and length L has a small block of mass M attached at one end. The moment of inertia of the system about an axis through its CM and perpendicular to the length of the rod is
(A) \(\frac{13}{12}\) ML²
(B) \(\frac{1}{3}\) ML²
(C) \(\frac{5}{24}\) ML²
(D) \(\frac{7}{48}\) ML²
Answer:
(C) \(\frac{5}{24}\) ML²

Question 18.
A thin wire of length L and uniform linear mass density λ is bent into a circular ring. The MI of the ring about a tangential axis in its plane is

Answer:
(C) \(\frac{3 \lambda L^{3}}{8 \pi^{2}}\)

Question 19.
When a planet in its orbit changes its distance from the Sun, which of the following remains constant ?
(A) The moment of inertia of the planet about the Sun
(B) The gravitational force exerted by the Sun on the planet
(C) The planet’s speed
(D) The planet’s angular momentum about the Sun
Answer:
(D) The planet’s angular momentum about the Sun

Question 20.
If L is the angular momentum and I is the moment of inertia of a rotating body, then \(\frac{L^{2}}{2 I}\) represents its
(A) rotational PE
(B) total energy
(C) rotational KE
(D) translational KE.
Answer:
(C) rotational KE

Question 21.
A thin uniform rod of mass 3 kg and length 2 m rotates about an axis through its CM and perpendicular to its length. An external torque changes its frequency by 15 Hz in 10 s. The magnitude of the torque is
(A) 3.14 N.m
(B) 6.28 N.m
(C) 9.42 N.m
(D) 12.56 N.m.
Answer:
(C) 9.42 N.m

Question 22.
The flywheel of a motor has mass 300 kg and radius of gyration 1.5 m. The motor develops a constant torque of 2000 N.m and the flywheel starts from rest. The work done by the motor during the first 4 revolutions is
(A) 2 kJ
(B) 8 kJ
(C) 8n kJ
(D) 16π kJ.
Answer:
(D) 16π kJ.

Question 23.
Two uniform solid spheres, of the same mass but radii in the ratio R₁ : R₂ = 1 : 2, roll without slipping on a plane surface with the same total kinetic energy. The ratio ω₁ : ω₂ of their angular speed is
(A) 2 : 1
(B) \(\sqrt{2}\) : 1
(C) 1 : 1
(D) 1 : 2.
Answer:
(A) 2 : 1

Question 24.
A circularly symmetric body of radius R and radius of gyration k rolls without slipping along a flat surface. Then, the fraction of its total energy associated with rotation is [c = k²/R²]
(A) c
(B) \(\frac{c}{1+c}\)
(C) \(\frac{1}{c}\)
(D) \(\frac{1}{1+c}\)
Answer:
(B) \(\frac{c}{1+c}\)

Balbharti Maharashtra State Board 12th Biology Important Questions Chapter 6 Plant Water Relation Important Questions and Answers.

Maharashtra State Board 12th Biology Important Questions Chapter 6 Plant Water Relation

Multiple Choice Questions

Question 1.
What is the reason behind various properties of water?
(a) Physical state
(b) Hydrogen bonding
(c) Colour
(d) Fluidity
Answer:
(b) Hydrogen bonding

Question 2.
Which special type of tissue is present in epiphytic roots?
(a) Velamen
(b) Lenticel
(c) Aerenchyma
(d) Haustoria
Answer:
(a) Velamen

Question 3.
In which zone of root we come across root hairs?
(a) meristematic region
(b) zone of elongation
(c) zone of absorption
(d) maturation zone
Answer:
(c) zone of absorption

Question 4.
In double layered cell wall of root hair, outer layer is of ……………..
(a) cellulose
(b) pectin
(c) cutin
(d) suberin
Answer:
(b) pectin

Question 5.
What is rhizosphere?
(a) Root ball formed by growth of roots
(b) Covering of root tip
(c) Microenvironment around root
(d) region of root hairs
Answer:
(c) Microenvironment around root

Question 6.
A root hair is derived from …………….. cell.
(a) epidermal
(b) cortical
(c) endodermal
(d) pericycle
Answer:
(a) epiderinal

Question 7.
When we keep raisins in water they swell up, due to ……………..
(a) exosmosis
(b) plasmolysis
(c) imbibition
(d) diffusion
Answer:
(c) imbibition

Question 8.
This phenomenon is associated with exchange of gases ……………..
(a) osmosis
(b) diffusion
(c) imbibition
(d) plasmolysis
Answer:
(b) diffusion

Question 9.
What is correct expression for diffusion pressure deficit (D.PD.)?
(a) D.PD. = O.R – S.E
(b) D.PD. = T.E – O.E
(c) D.PD. = W.E – T.R
(d) D.PD. = O.R – T.R
Answer:
(d) D.PD. = O.P – T.P

Question 10.
What is true for a turgid cell?
(a) T.R is zero
(b) T.R = S.R
(c) D.P.D. is zero
(d) W.R = S.R
Answer:
(c) D.P.D. is zero

Question 11.
The particles which easily diffuse through cell membrane are ……………..
(a) lipid soluble
(b) water soluble
(c) hydrophilic
(d) lipophobic
Answer:
(a) lipid soluble

Question 12.
Which proteins help in facilitated diffusion process?
(a) cutin
(b) mucin
(c) aquaporins
(d) lipoproteins
Answer:
(c) aquaporins

Question 13.
D.PD. is now known as ……………..
(a) water potential
(b) solute potential
(c) pressure potential
(d) osmotic potential
Answer:
(a) water potential

Question 14.
Water always flows from ……………..
(a) more negative water potential to less negative water potential
(b) high water potential area to low water potential area
(c) low water potential area to high water potential area
(d) negative water potential area to area of zero water potential
Answer:
(b) high water potential area to low water potential area

Question 15.
Plasmolysed cell becoming turgid is process of ……………..
(a) replasmolysis
(b) incipient plasmolysis
(c) deplasmolysis
(d) exosmosis
Answer:
(c) deplasmolysis

Question 16.
In a fully turgid cell ……………..
(a) T.P = O.P
(b) T.P = S.P
(c) O.P = S.P
(d) T.RP = 0 (zero)
Answer:
(a) T.P = O.P

Question 17.
Casparian strip of endodermis has material ……………..
(a) pectin
(b) suberin
(c) cutin
(d) porin
Answer:
(b) suberin

Question 18.
Water from pericycle is forced into xylem due to ……………..
(a) aquaporin
(b) plasmodesmata
(c) root pressure
(d) ion-channels
Answer:
(c) root pressure

Question 19.
Water absorbed from root hair when passes through intercellular spaces and cell wall it is …………….. pathway.
(a) apoplast
(b) symplast
(c) transmembrane
(d) plasmodesmata
Answer:
(a) apoplast

Question 20.
In root system, secondary roots arise from ……………..
(a) cortical cells
(b) endodermis
(c) pericycle
(d) passage cell
Answer:
(c) pericycle

Question 21.
Active absorption of water occurs during ……………..
(a) early morning
(b) daytime
(c) bright sunlight
(d) night time
Answer:
(d) night-time

Question 22.
The value of root pressure is usually about ……………..
(a) +1 to +12 bars
(b) -1 to +1 bars
(c) +1 to +2 bars
(d) +1 to +21 bars
Answer:
(c) +1 to +2 bars

Question 23.
The ascent of sap in plants takes place through ……………..
(a) xylem
(b) phloem
(c) parenchyma
(d) endodermis
Answer:
(a) xylem

Question 24.
From the following, which water will show greatest water potential (ψ)
(a) pure water
(b) salt water
(c) sugar water
(d) salt + sugar + water
Answer:
(a) pure water

Question 25.
Due to entry of water in a cell, the pressure potential ……………..
(a) increases
(b) decreases
(c) remains unaffected
(d) becomes zero
Answer:
(a) increases

Question 26.
Which mineral element is not remobilized in plants?
(a) P
(b) K
(c) S
(d) Ca
Answer:
(d) Ca

Question 27.
Phloem sap analysis is done using isotope ……………..
(a) ¹⁶O
(b) ¹⁴C
(c) ¹⁵N
(d) ³⁵S
Answer:
(b) ¹⁴C

Question 28.
In plants, food is mainly translocated in form of ……………..
(a) starch
(b) glucose
(c) sucrose
(d) amino acids
Answer:
(c) sucrose

Question 29.
Transport of food through phloem is ……………..
(a) unidirectional
(b) bidirectional
(c) three dimensional
(d) absent
Answer:
(b) bidirectional

Question 30.
Guttation occurs through ……………..
(a) stomata
(b) lenticels
(c) hydathodes
(d) velamen
Answer:
(c) hydathodes

Question 31.
Amount of cuticular transpiration is about ……………..
(a) 0.1-1%
(b) 8 – 10%
(c) 90 – 93%
(d) 2 – 8%
Answer:
(b) 8 – 10%

Question 32.
Epistomatic leaf shows ……………..
(a) stomata on upper side
(b) stomata on lower side
(c) stomata on both surfaces
(d) absence of stomata
Answer:
(a) stomata on upper side

Question 33.
Dumbbell shaped guard cells are found in ……………..
(a) most dicots
(b) grasses
(c) gymnosperms
(d) desert plants
Answer:
(b) grasses

Question 34.
Stomatal transpiration occurs during night time in ……………..
(a) most dicots
(b) grasses
(c) gymnosperms
(d) desert plants
Answer:
(d) desert plants

Question 35.
Reservoir of K⁺ ions is ……………..
(a) guard cells
(b) epidermal cells
(c) subsidiary cells
(d) mesophyll
Answer:
(c) subsidiary cells

Question 36.
Guard cells are surrounded by ……………..
(a) epidermal hairs
(b) mesophyll cells
(c) accessory cells
(d) lenticels
Answer:
(c) accessory cells

Question 37.
When guard cells close stomata at night which acid prevents uptake of K⁺ and C^– ions?
(a) Abscissic acid
(b) Pyruvic acid
(c) Malic acid
(d) Acetic acid
Answer:
(a) Abscissic acid

Question 38.
Which type of injury is noticed in plants if there is excessive transpiration?
(a) Burning
(b) Chlorosis
(c) Necrosis
(d) Wilting
Answer:
(d) Wilting

Question 39.
Maximum transpiration occurs through ……………..
(a) cuticle
(b) lenticels
(c) stomata
(d) bark
Answer:
(c) stomata

Question 40.
What will be the condition of guard cells during night-time?
(a) Show increased turgor pressure
(b) Become flaccid
(c) Increase uptake of K⁺ and Cl^– ions
(d) Starch converted to sugar
Answer:
(b) Become flaccid

Question 41.
Cell A has water potential – 10 bars and cell B has – 5 bars, the movement of water will occur from ……………..
(a) A to B
(b) B to A
(c) No movement
(d) Either A to B or B to A
Answer:
(b) B to A

Question 42.
What is the correct symbol and unit of water potential?
(a) ψ and ha
(b) ω and ha
(c) ω and Pa
(d) ψ and Pa
Answer:
(d) ψ and Pa

Question 43.
When root system absorbs water, which is the first physical process concerned with this activity?
(a) Osmosis
(b) Imbibition
(c) Facilitated diffusion
(d) Diffusion
Answer:
(b) Imbibition

Match the columns

Question 1.

Column A (Scientist)	Column B (Theory)
(1) Munch	(a) Proton transport theory
(2) Bohem	(b) Pressure flow theory
(3) J. Pristley	(c) Capillary theory
(4) Levitt	(d) Root Pressure theory

Answer:

Column A (Scientist)	Column B (Theory)
(1) Munch	(b) Pressure flow theory
(2) Bohem	(c) Capillary theory
(3) J. Pristley	(d) Root Pressure theory
(4) Levitt	(a) Proton transport theory

Question 2.

Column A (Scientist)	Column B (Theory)
(1) Dixon and Joly	(a) Starch-sugar                inter conversion
(2) Steward	(b) Osmotic absorption theory
(3) Atkins and Pristley	(c) Non-osmotic absorption theory
(4) Kramer and Thimann	(d) Cohesion tension theory

Answer:

Column A (Scientist)	Column B (Theory)
(1) Dixon and Joly	(d) Cohesion tension theory
(2) Steward	(a) Starch-sugar inter conversion
(3) Atkins and Pristley	(b) Osmotic absorption theory
(4) Kramer and Thimann	(c) Non-osmotic absorption theory

Question 3.

Column A	Column B (New Terminology)
(1) D.P.D.	(a) Osmotic potential
(2) O.E	(b) Pressure potential
(3) T.P	(c) Water potential

Answer:

Column A	Column B (New Terminology)
(1) D.P.D.	(c) Water potential
(2) O.E	(a) Osmotic potential
(3) T.P	(b) Pressure potential

Classify the following to form Column B as per the category given in Column A

Question 1.
B, Co, Mn, P, N, S

Column A	Column B
Macro elements	——–, ———-, ——–
Micro elements	——–, ———-, ——–

Answer:

Column A	Column B
Macro elements	P, N, S
Micro elements	B, Co, Mn

Question 2.
Capillary water, Combined water, Hygroscopic water, Gravitational water.

Column A	Column B
(1) Water adsorbed on soil particles	—————
(2) Water penetrated deep in soil	————–
(3) Water present as hydrated oxides	————-
(4) Water present between soil particles	————-

Answer:

Column A	Column B
(1) Water adsorbed on soil particles	Hygroscopic water
(2) Water penetrated deep in soil	Gravitational water
(3) Water present as hydrated oxides	Combined water
(4) Water present between soil particles	Capillary water

Very short answer question

Question 1.
Why water acts as a thermal buffer?
Answer:
The water has high specific heat, high heat of vaporization and high heat of fusion hence it acts as a thermal buffer.

Question 2.
Why water can easily rise in capillaries?
Answer:
Water has high surface tension and high adhesive and cohesive force hence it can easily rise in capillaries.

Question 3.
Enlist the kind of water available in soil environment.
Answer:
Soil environment has gravitational water, hygroscopic water, combined water and capillary water.

Question 4.
Mention examples of imbibition process.
Answer:
Soaking of seeds, swelling up of raisins, kneading of flour and warping of wooden doors in rainy season.

Question 5.
What is the mechanism of imbibition?
Answer:
When imbibition takes place water molecules (imbibate) get tightly adsorbed on imbibant without formation of solution.

Question 6.
Why water enters plant cell by process of diffusion?
Answer:
Water present around cell wall has more diffusion pressure than inner cell sap hence water moves in the cell through freely permeable cell wall by diffusion.

Question 7.
What is isotonic condition in osmotic system?
Answer:
A condition where concentration of solution has neither gain nor loss of water in an osmotic system is isotonic.

Question 8.
What is effect on protoplasm when cell is plasmolysed ?
Answer:
When cell is plasmolysed, protoplast of cell shrinks and recedes from the cell wall thus gap is observed between cell wall and protoplast.

Question 9.
What is apoplast pathway?
Answer:
When water absorbed by root hair passes across the roots through cell wall and intercellular spaces of root cortex it is called apoplast pathway.

Question 10.
Which experiment is a proof for existence of root pressure?
Answer:
When a stem of potted plant is cut above the soil, xylem sap is seen oozing through cut end, that proves presence of root pressure.

Question 11.
Which analysis indicates that minerals are absorbed by plants?
Answer:
Analysis of plant ash contents is indication of absorbed minerals.

Question 12.
Which ions are readily remobilized in plants?
Answer:
Ions of phosphorus, sulphur and nitrogen are remobilized from older plants, parts (leaves) to younger parts.

Question 13.
What is radial and tangential translocation?
Answer:
When lateral translocation of food occurs in root or stem, transport from phloem to pith is radial translocation while that from phloem to cortex is tangential translocation.

Question 14.
What is loading of vein?
Answer:
In turgid cell, due to increased turgor pressure of photosynthetic cell, sugar is forced into the sieve tube of the vein, which is known as loading of vein.

Question 15.
What is unloading of vein?
Answer:
At the sink end, turgor pressure is lowered and hence turgor pressure gradient is developed from sieve-tube which translocates food passively along concentration gradient, this is vein unloading.

Question 16.
What is peculiarity of wall of guard cells?
Answer:
The inner wall of guard cell that faces opening is thick and inelastic while its outer wall or lateral wall is thin and elastic.

Question 17.
Give reaction of starch-sugar interconversion theory.
Answer:

Question 18.
How do land plants absorb water and mineral salts from the soil?
Answer:
Land plants absorb water and mineral salts from soil with the help of their roots.

Question 19.
In which form the water is lost from leaves to the atmosphere?
Answer:
The water is lost in the form of vapour from leaves to the atmosphere.

Question 20.
How does plants lift the water from soil up to canopy without any pump?
Answer:
Plants have vascular tissue system of xylem, mainly through vessels and tracheids. Water is conducted upwards due to pull created in this continuous channel of water.

Question 21.
What is a peculiarity of epiphytic plants like orchids?
Answer:
The epiphytic plants like orchids have epiphytic roots with special water vapour absorbing layer of velamen tissue that absorbs water vapour from air.

Question 22.
What are root hairs?
Answer:
Root hairs are the extensions of epiblema or epidermal cells in the region of absorption.

Question 23.
Why do the wooden doors become very hard to close and open in rainy season?
Answer:
During rainy season wooden doors swell up due to imbibition of water. The humidity or moisture content of air increases and wood which is hydrophilic takes up this moisture.

Question 24
How does the water come out through the surface of porous earthen pot?
Answer:
Earthen pot has tiny pores through which water diffuses out. Due to evaporation of this water from surface water kept inside becomes cool.

Question 25.
Describe external structure of root hair.
Answer:
A root hair is a cytoplasmic extension of epiblema cell which is colourless, unbranched, ephemeral, very delicate tubular structure of about 1 to 10 mm long.

Question 26.
What is gravitational water?
Answer:
Water present in the soil that percolates deep inside due to gravitational force is called gravitational water.

Question 27.
How can we describe osmotic movement of water related to energy?
Answer:
The osmotic movement of water is on the basis of free energy which is used to do work.

Question 28.
Which process is responsible for transport of minerals and to make them available to cells?
Answer:
The minerals absorbed by roots are transported upwards through sap and from veins by process of diffusion cells uptake them.

Question 29.
Give examples of vertical translocation of food in downward direction and upward direction.
Answer:
Food is translocated in downward direction from leaves (source) to root, while during germination of seed, bulbil, corm it is in upward direction.

Question 30.
What is translocation of food?
Answer:
The transport of food from one part of plant to the other part, i.e. source to sink is called translocation of food.

Question 31.
Which plant tissues are involved in transport of water, minerals and food?
Answer:
A complex plant tissue xylem, mainly with its tracheids and vessels is involved in transport of water and minerals while complex tissue phloem with sieve tubes and companion cells is involved in transport of food.

Question 32.
Which is a direct pathway of transport available in plants through root system?
Answer:
Secondary roots that originate from pericycle that is outer layer of vascular cylinder, bypass endodermis with Casparian strip allow direct apoplast pathway to enter xylem and phloem.

Question 33.
For which type of plants root pressure theory of ascent of sap is applicable?
Answer:
Root pressure theory is applicable to plants having height up to 10 to 20 metres.

Question 34.
What is hydrogen bond?
Answer:
Hydrogen bond results from the attractive force between a hydrogen atom covalently bonded to a very electronegative atom like O or N.

Question 35.
When you burn an incense stick in one corner of room, its fragrance spreads all over the room in a short time. How does it happen?
Answer:
The process of diffusion is responsible for spreading the fragrance of an incense stick in one corner of room.

Give definitions of the following

Question 1.
Facilitated diffusion
Answer:
The passive absorption of solutes when mediated by a carrier is called facilitated diffusion.

Question 2.
Osmotic pressure / Osmotic potential
Answer:
The pressure exerted due to osmosis is osmotic pressure now termed as osmotic potential.

Question 3.
Deplasmolysis
Answer:
When plasmolysed cell is placed in hypotonic solution, endo-osmosis occurs making cell turgid again then this is called deplasmolysis.

Question 4.
Translocation of food
Answer:
The movement of food from one part of plant to the other part is called translocation of food.

Name the following

Question 1.
Condition of cell wall and cell membrane : based on permeability.
Answer:
Cell wall freely permeable and Cell membrane semipermeable.

Question 2.
Weak solution having low osmotic concentration.
Answer:
Hypotonic

Question 3.
Strong solution having high osmotic concentration.
Answer:
Hypertonic

Question 4.
A suberised layer on endodermis.
Answer:
Casparian strip

Question 5.
One example of each epistomatic, hypostomatic and amphistomatic leaf.
Answer:

1. Epistomatic leaf – Lotus
2. Hypostomatic – Nerium.
3. Amphistomatic – Grass

Question 6.
Pathway for entry of water into xylem from endodermis.
Answer:
Symplast pathway

Question 7.
A material deposited on endodermis which forms barrier.
Answer:
Suberin

Question 8.
Water imbibed or adsorbed on soil particles.
Answer:
Hygroscopic water

Question 9.
Water potential was previously known as.
Answer:
D.ED. or Diffusion pressure deficit

Question 10.
Free energy per molecule in chemical system.
Answer:
Chemical potential

Question 11.
The substance synthesized in cell responsible for increasing osmotic concentration as per Munch theory.
Answer:
Glucose

Question 12.
A waxy substance present in layer on outer surface of epidermis.
Answer:
Cutin in layer cuticle

Question 13.
Anatomical structure through which guttation occurs.
Answer:
Hydathode

Question 14.
Type of leaves in hydrophytes based on distribution of stomata.
Answer:
Epistomatic

Question 15.
Type of leaves in xerophytes based on distribution of stomata.
Answer:
Hypostomatic

Question 16.
The form in which food is transported in plants and then stored in plants.
Answer:
Transported in the form of sugar, sucrose and stored as starch.

Question 17.
Chief vascular element concerned with transport of food in plant.
Answer:
Sieve tubes of phloem.

Give functions/significance of the following

Question 1.
Zone of absorption of root.
Answer:
This zone has unicellular root hairs which absorb water available in rhizosphere.

Question 2.
Diffusion.
Answer:

1. Necessary for absorption of water by root hairs
2. For absorption of minerals
3. Conduction of water against gravity
4. Exchange of gases
5. Transport and distribution of food.

Question 3.
Turgor pressure.
Answer:

1. Keeps cells and cell organelles stretched
2. Provides support
3. During growth essential for cell enlargement
4. Maintains shape of cell
5. Facilitates opening and closing of stomata

Question 4.
Osmosis.
Answer:

1. Absorption of water into root
2. Maintains turgidity of cell
3. Facilitates cell to cell movement of absorbed water
4. Resistance to drought or frost injury
5. Helps in drooping movement of leaflet.
   E.g. Touch me not plant

Question 5.
Root pressure.
Answer:
Hydrostatic pressure developed in living cells of root helps in forcing water from pericycle into xylem
Helps in upward conduction of water against gravity

Question 6.
Transpiration.
Answer:

1. Removal of excess of water
2. Helps in passive absorption of water and minerals
3. Helps in ascent of sap – transpiration pull
4. Maintains turgor of cells
5. Imparts cooling effect by reducing temperature 90% – 93% is stomatal transpiration and hence when stomata are open gaseous exchange takes place.

Question 7.
Guard cells.
Answer:

1. They contain few chloroplasts so capable of photosynthesis.
2. They can change their size hence opening and closing of stomatal aperture.

Distinguish between the following

Question 1.
Diffusion and Osmosis.
Answer:

Diffusion	Osmosis
1. Diffusion is the movement of ions, atoms or molecules of solute, liquids or gases.	1. Osmosis Is the special type of diffusion of water or solvent.
2. Diffusion involves the flow of diffusing particles in both the directions.	2. Osmosis involves the unidirectional flow of solvent molecules.
3. Diffusion does not occur through a semi- permeable membrance.	3. Osmosis occurs through a semi-permeable membrance.
4. Diffusion occurs from a place of higher concentration of diffusing particles to a place of lower concentration.	4. Osmosis occurs from a solution of lower concentration to a solution of higher concentration.

Question 2.
Active absorption and Passive absorption.
Answer:

Active absorption	Passive absorption
1. Active absorption is a physiological process.	1. Passive absorption is a physical process.
2. Active absorption takes place by the process of osmosis.	2. Passive absorption takes place by suction force.
3. It involves the expenditure of energy on the part of absorbing cells.	3. It does not involve the expenditure of energy on the part of absorbing cells.
4. Active absorption takes place against the concentration gradient.	4. Passive absorption takes place along the concentration gradient.

Question 3.
Cuticular transpiration and Stomatal transpiration.
Answer:

Cuticular transpiration	Stomatal transpiration
1. Cuticular traspiration takes place through the cuticle.	1. Stomatal traspiration takes place through the stomata.
2. Cuticular transpiration accounts for 8 to 10% of total loss of water from plants.	2. Stomatal transpiration accounts for 80 to 90% of total loss of water from plants.
3. Cuticular transpiration depends upon the thickness of the cuticle.	3. Stomatal traspiration depends upon the number and size of stomata.

Give reasons or explain the statements

Question 1.
Water is considered as ‘elixir of life’.
Answer:

1. Water plays an important role in living organisms.
2. About 90 – 95% water is present in cell which is functional and structural unit of living organisms.
3. It helps in maintaining turgidity and shape of cells and cell organelles.
4. Due to its various properties, it is medium of biochemical reactions, transporting medium and thermal buffer also.
5. Therefore it is absolutely necessary for life i.e. ‘elixir of life’.

Question 2.
Water is a best transporting medium.
Answer:

1. Water is in liquid state at room temperature.
2. It is best solvent for most of the solutes. Thus called universal solvent.
3. It is inert inorganic compound with neutral pH i.e. pH 7 when in pure form.
4. Hence it is best medium for dissolved minerals.

Question 3.
Water is significant molecule that connects physical world with biological processes.
Answer:

1. Water is an important constituent of cell. About 90 – 95% of protoplasm is water.
2. Water in liquid state is best solvent in which various minerals and food molecules are dissolved and transported.
3. Water acts as the thermal buffer has high specific heat.
4. Water molecules have high adhesive and cohesive forces of attraction.
5. It can rise in capillaries due to high surface tension and adhesive forces, e.g. Ascent of sap in plants.
6. Due to all these important factors it is a significant molecule connecting physical world with biological processes.

Question 4.
Absorption of water by roots from soil is with physical processes inhibition, diffusion and osmosis.
Answer:

1. Water is absorbed from rhizosphere with the help of unicellular root hairs.
2. Root hairs have plasma membrane and thin, double layer cell wall of pectin and cellulose.
3. During imbibition water molecules get tightly adsorbed to the wall of hydrophilic colloids.
4. Cell wall is freely permeable membrane hence through diffusion water passes into the cell.
5. Osmosis is a special kind of diffusion of solvent through a semipermeable membrane and as plasma membrane is semipermeable, water enters cell by osmotic mechanism.
6. Hence all these three physical processes occur sequentially when water is absorbed by roots.

Question 5.
Additional apoplastic pathway through secondary roots is beneficial to plants.
Answer:

1. Secondary roots develop from the pericycle which is inside endodermis.
2. Protoxylem is situated close to pericycle in root.
3. Endodermis have suberized layer Casparian strip which forces water to move in the symplast so that it can enter the vascular xylem.
4. Since secondary roots originate form pericycle, they bypass the Casparian strip.
5. Therefore, a direct pathway to xylem and phloem is available without moving into symplast.

Write shorts notes

Question 1.
Role of water or Biological importance of water.
Answer:

1. Water is absolutely necessary for life.
2. It is a major constituent of protoplasm.
3. If provides aqueous medium for various metabolic reactions that take place in plant.
4. It is raw material for photosynthesis.
5. It helps in maintaining the turgidity of cells.
6. It is excellent solvent for various organic materials.
7. It is transporting medium for dissolved minerals.
8. It is thermal buffer.

Question 2.
Properties of water.
Answer:

1. Water is a compound and it is in liquid state at room temperature.
2. It is an inert inorganic compound with neutral pH.
3. It has high specific heat, high heat of vapourization, high heat of fusion.
4. It has high surface tension.
5. Water molecule has good adhesive and cohesive forces of attraction.
6. The various properties of water are result of weak hydrogen bonding between the water molecules.

Question 3.
Lenticular transpiration.
Answer:

1. Small, raised structures, which are composed of loose cells with large intercellular spaces situated on bark are lenticels.
2. They are observed on bark of old stem, root and on woody pericarp of fruits.
3. Lenticular transpiration is of very small amount about 0.1% to 1% of total transpiration.
4. It is very slow process but occurs throughout the day.

Question 4.
Structure of stomatal apparatus.
Answer:

1. Stomata are minute openings mainly located in the epidermal surfaces of young stem and leaves.
2. It is composed of two guard cells and accessory cells which form the opening stomatal pore for transpiration.
3. Guard cells are kidney shaped in dicot plants and dumbbell shaped in grasses.
4. Guard cells have unevenly thickened wall, inner wall thick, inelastic while outer wall is thin and elastic.
5. Guard cells are nucleated cells with few chloroplasts and hence can perform photosynthesis.
6. Accessory cells are specialized cells that surround the guard cells and are reservoirs of K⁺ ions.

Question 5.
Apoplast pathway.
Answer:

1. The movement of water across the root cells occur by two pathways viz. apoplast and symplast.
2. Apoplast movement of water in plants occurs exclusively through cell walls and intercellular spaces in living cells of cortex.
3. Apoplast pathway is up to the endodermis as the suberized layer of Casparian strip obstructs the movement.
4. Additional apoplastic route giving direct contact to xylem and phloem is through secondary roots which originate at pericycle and bypass endodermis.
5. The cellulosic walls of root hair, cortical cells are hydrophilic and permeable to water.

Question 6.
Water potential.
Answer:

1. Chemical potential of water is called water potential.
2. The Greek letter (ψ) psi represents water potential.
3. The unit of measurement is in bars/pascals (Pa) or atmosphere.
4. Water potential of pure water is always zero.
5. Water potential of protoplasm is equal to D.PD. but it has a negative value.
6. Water always moves from less negative potential to more negative water potential.

Short answer questions

Question 1.
Enlist macronutrients and micronutrients required for plant growth.
Answer:

1. Some minerals which are required in large amounts for plant metabolism are macronutrients. E.g. C, H, O, E N, S, Mg, Ca, K. etc.
2. Some minerals which are required in small amounts for plant metabolism are micronutrients. E.g. Cu, Co, Mn, B, Zn, Cl, etc.

Question 2.
Explain about the factors that affect water absorption.
Answer:

1. Water is absorbed by unicellular root hairs from soil.
2. Presence of capillary water in soil is needed as this water from soil is absorbed by root hairs.
3. Soil temperature of 20-30°C favours water absorption.
4. Rate of absorption is decreased by high concentration of solutes in soil.
5. Soil should be properly aerated, poorly aerated soil shows poor absorption rate.
6. Increased transpiration accelerates rate of absorption of water.

Question 3.
Explain mechanism of sugar transport through phloem.
Answer:

1. The part of plant where food is synthesized is known as source and where it is utilized is sink.
2. Food is translocated through phloem tissue in the form of sucrose along the concentration gradient from source to sink.
3. Munch’s pressure flow theory or mass flow hypothesis is widely accepted theory for sugar transport.
4. Glucose is synthesized in photosynthetic cells hence endo-osmosis occurs due to increased osmotic concentration.
5. With this turgor pressure increases and sugar is forced in sieve tube of vein from photosynthetic cell – Vein loading.
6. At the sink, sugar is utilized and excess amount converted to starch, hence osmotic concentration is lowered and exosmosis takes place.
7. Turgor pressure is lowered hence turgor pressure gradient is set and food is translocated passively against concentration gradient – Vein unloading.

Question 4.
Explain the principles of cohesion tension theory and its limitations?
Answer:

1. Cohesion tension theory is widely accepted theory for translocation of water proposed by Dixon and Joly.
2. It is based on principles cohesion and adhesion of water molecules along with transpiration pull.
3. Strong attractive force between water molecules is cohesive force and the strong force of attraction between water molecules and wall of lumen of xylem vessels is called adhesive force.
4. Owing to these cohesive and adhesive forces a continuous column of water is maintained in xylem elements from root to aerial parts, tips of leaves.
5. Transpiration pull is developed in the xylem vessel in leaves.
6. This pull is transmitted downwards and due to suction pressure, water is pulled passively against gravity, i.e. ascent of sap.

Limitations of theory-

1. For activity of transpiration pull, water column should be maintained constantly and continuously. Owing to changes in temperature during day and night, gas bubbles may be formed in the water channel. This will break the continuity.
2. According to this theory, tracheids are more efficient than vessels because of their tapering end walls which support water column. Vessels are tubular structure with open ends.

Question 5.
What is the meaning of specific heat, heat of vaporization and heat of fusion?
Answer:

1. Specific heat : The specific heat is the amount of heat per unit mass required to raise the temperature by one degree. The specific heat of water is 1 calorie/gram degree C.
2. Heat of vapourization : It is the amount of energy that must be added to a liquid substance to transform a quantity of that substance to gas. It is also known as heat of evaporation.
3. Heat of fusion : It is amount of energy typically heat, provided to a specific quantity of the substance to change its state from solid to liquid at constant pressure.

Question 6.
What are adhesive and cohesive forces?
Answer:

1. Adhesive and cohesive forces are attractive forces between molecules of same substance.
2. Cohesive forces exist between molecules of the same type. E.g. between water-water molecule.
3. Adhesive forces exist between dissimilar molecules. E.g. water molecule and lignin deposited wall of xylem element.

Chart based/Table based questions

Question 1.
Complete the table based on types of solution.

Answer:

Question 2.
Complete the table.

Event	Physical process
1. Soaking of Seeds	—————-
2. Water entering guard cells	—————-
3. Exchange of gases	—————
4. Loss of water in liquid form	—————
5. Water coming out through earthen pot	—————
6. Loss of water in vapour form	—————
7. Absorption of solutes passively by carrier	—————
8. Spreading of fragrance of incense stick	—————

Answer:

Event	Physical process
1. Soaking of Seeds	Imbibition
2. Water entering guard cells	Osmosis
3. Exchange of gases	Diffusion
4. Loss of water in liquid form	Guttation
5. Water coming out through earthen pot	Diffusion
6. Loss of water in vapour form	Transpiration
7. Absorption of solutes passively by carrier	Facilitated Diffusion
8. Spreading of fragrance of incense stick	Diffusion

Diagram based questions

Question 1.
Zones of root
Answer:

Question 2.
Structure of root hair
Answer:

Question 3.
Diffusion of water
Answer:

Question 4.
Pathway for water uptake
Answer:

Question 5.
L. S. of sieve tube (Transport of food)
Answer:

Question 6.
Structure of stomata and Types of guard cells
Answer:

Long answer questions

Question 1.
Write a note on macronutrients and micronutrients required for plant growth.
Answer:

1. Plants absorb mincral nutrients from their surroundings.
2. For a proper growth of plants about 35 to 40 different elements are required.
3. Plants absorb these nutrients in ionic or dissolved form from soil with their root system e.g. Phosphorus as PO₄^–. Sulphur as SO₄^2- etc.
4. Based on their requirement in quantity. they are classified as major nutrients or macronutrients and those needed In small amounts are minor or micronutrients.
5. Macroclements are required in large amounts, as they play nutritive and structural roles e.g. C, H, O. R Mg. N, K. S and Ca. -Ca pectate cell wall component. Mg component of chlorophyll.
6. C. H, O are non-mineral major elements obtained from air and water e.g. CO₂ is source of carbon, Hydrogen from water.
7. Microelements arc required In traces as they mainly have catalytic role as co-factors or activators of enzymes.
8. Microelernents may be needed for certain activity in life cycle of plant e.g. B for pollen germination, Si has protective role during stress conditions and fungal attacks, Al enhances availability of phosphorus.
9. The Important micronutrients for plant growth are Mn. B. Cu, Zn, Cl.

Balbharti Maharashtra State Board Class 11 Psychology Important Questions Chapter 8 Memory Important Questions and Answers.

Maharashtra State Board 11th Psychology Important Questions Chapter 8 Memory

1A. Complete the following statements.

Question 1.
__________ is the retention of information over time for the purpose of future action.
(A) Learning
(B) Memory
(C) Attention
Answer:
(B) Memory

Question 2.
__________ plays an important role in storage of memory.
(A) Hippocampus
(B) Amygdala
(C) Nervous system
Answer:
(A) Hippocampus

Question 3.
The term __________ memory was coined by Miller, Galanter, and Pribram around 1960.
(A) sensory
(B) working
(C) long-term
Answer:
(B) working

Question 4.
Visuo-spatial sketch pad (VSSP) is a/an __________ system.
(A) passive
(B) active
(C) non-functional
Answer:
(A) passive

Question 5.
Autobiographical memory is a type of __________ memory.
(A) implicit
(B) procedural
(C) declarative
Answer:
(C) declarative

Question 6.
In __________ recall, the material is recalled in the exact order in which it was presented.
(A) serial
(B) free
(C) definite
Answer:
(A) serial

Question 7.
__________ is one of the major ways of measuring memory.
(A) Rote learning
(B) Perception
(C) Relearning
Answer:
(C) Relearning

Question 8.
__________ is the evidence for the organisation of long-term memory.
(A) Serial recall
(B) Tip of the tongue phenomenon
(C) Recognition
Answer:
(B) Tip of the tongue phenomenon

Question 9.
The pioneer of experiments on forgetting was __________
(A) Murdock
(B) Hermann Ebbinghaus
(C) Baddeley
Answer:
(B) Hermann Ebbinghaus

Question 10.
__________ interference means backward interference.
(A) Retroactive
(B) Proactive
(C) Passive
Answer:
(A) Retroactive

Question 11.
__________ is a Greek word meaning ‘of memory or related to memory.’
(A) Mnemonic
(B) Syllable
(C) LTM
Answer:
(A) Mnemonic

Question 12.
The final stage in POWER method is __________
(A) evaluate
(B) rethink
(C) relearn
Answer:
(B) rethink

1B. Match the following pairs.

Question 1.

A	B
i. LTM	a. Conscious
ii. Explicit memory	b. Less than one second
iii. Sensory memory	c. Life-time memory
iv. Procedural memory	d. Autobiographical events
	e. Unconscious

Answer:

A	B
i. LTM	c. Life-time memory
ii. Explicit memory	a. Conscious
iii. Sensory memory	b. Less than one second
iv. Procedural memory	e. Unconscious

1C. State whether the following statements are true or false. If false, correct them. If true, explain why.

Question 1.
There are four basic processes of memory.
Answer:
False
Reason: There are three basic processes of memory, viz. encoding/acquisition, storage, and retrieval.

Question 2.
Short-term memory is known by many other terms.
Answer:
True
Explanation: Short-term or working memory was formerly known as ‘short term store’. It is also known as primary memory, immediate memory, operant memory, and provisional memory.

Question 3.
Baddeley called LTM the working bench of memory.
Answer:
False
Reason: Baddeley called STM the working bench of memory because STM is the most important stage of memory which is used most of the time for problem-solving.

Question 4.
Very often forgetting is due to unconscious processes like repression.
Answer:
True
Explanation: We subconsciously push unwanted thoughts and memories into our unconsciousness. It is one of the causes of forgetting.

Question 5.
In recognition, a person has to retrieve information from LTM with no cues.
Answer:
False
Reason: In recognition, a person has to point out or recognize previously learned material that is presented to him in a different context.

Question 6.
There are five levels of motivated forgetting.
Answer:
False
Reason: There are two levels of motivated forgetting, viz. Repression and Thought suppression.

Question 7.
The Method of Loci provides information about how context can affect memory.
Answer:
False
Reason: ‘The encoding specificity of memory’ provides information about how context can affect memory.

Question 8.
There are many techniques for improving memory.
Answer:
True
Explanation: Some of the techniques of improving memory are keyword method, encoding specificity, method of loci, mnemonic devices, practice, and rehearsal, minimizing interference, and POWER method.

1D. Identify the odd item from the following.

Question 1.
Episodic memory, Semantic memory, Autobiographical memory, Implicit memory
Answer:
Implicit memory

2A. Explain the following concepts

Question 1.
Memory
Answer:
According to Tulving, ‘Memory is the means by which we draw on our past experiences in order to use that information in the present. Memory is the term given to the structure and processes involved in the storage and subsequent retrieval of information.

Question 2.
Central executive
Answer:
The central executive is a supervisor responsible for the coordination of the subsystems and the selection of reasoning and storage strategies in the working memory model given by Baddeley.

Question 3.
Episodic buffer
Answer:
The episodic buffer holds information that is not covered by all other slave systems in the working memory model given by Baddeley. It is a link between working memory and long-term memory.

Question 4.
Autobiographical memory
Answer:
Autobiographical memory refers to episodes recollected from an individual’s own life. It is a type of declarative memory.

Question 5.
Flashbulb memory
Answer:
Flashbulb memory is a highly detailed and exceptionally clear ‘snapshot’ of mostly a traumatic moment. It is a type of autobiographical memory. Flashbulb memories illustrate that exceptional memories are easily retrieved.

Question 6.
Relearning
Answer:
Relearning measures retention by measuring how much faster one learns a previously learned material after an interval of time, i.e. the same material is learned by the same subject based on the same learning criterion at two different occasions separated by time interval.

Question 7.
Forgetting
Answer:
Forgetting is the inability to remember the things which we want to remember at that moment. In other words, it is the failure to retrieve the material from our long-term memory which we have already stored.

2B. Compare and contrast.

Question 1.
Primacy effect and Recency effect
Answer:

• The primacy effect occurs when the subject is able to recall items that are presented at the starting point of the list while the recency effect occurs when the subject is able to recall the items which are presented at the end. Free recall is effective in studying both these effects.
• Both primary and recency effect was witnessed in Murdock’s experiment where subjects could prominently recall the first few and last few words from the list.
• The primacy effect is the tendency to remember the first piece of information that we encounter in a better manner than the information presented later on. Conversely, the recency effect is the tendency to remember the most recent information in a better way.

Question 2.
Retroactive interference and Proactive interference
Answer:

• Retroactive interference is the partial or complete forgetfulness of the previously learned material due to new memories that get mixed up with the older ones. On the other hand, proactive interference is the partial or complete forgetfulness of newly learned material due to the old material.
• e.g. You studied Psychology yesterday and you studied Sociology today. If you forget Psychology due to the study of Sociology, it is due to retroactive interference while if you forget Sociology due to the study of Psychology, it is due to proactive interference.

3. Answer the following questions in around 35-40 words each.

Question 1.
State the types of human memory.
Answer:
The following flow chart explains types of human memory:

Question 2.
Explain the stages of memory.
Answer:

• Environmental stimulus is received with the help of sense organs (sensory information store). This is where the memory is stored.
• When we pay attention to the sensations, they are transferred to STM.
• If information is rehearsed or appears frequently, then it is transferred to the LTM.
  
• After encountering any problem, we bring the information from LTM to STM so that it’s available for solving the problem.

Question 3.
What do you mean by magical number 7?
Answer:

• The magical number 7+/-2 provides evidence for a limited capacity of the STM.
• Most adults can store between five and nine items in the STM.
• This idea was put forward by George Miller in 1956 and hence called 7 as a magical number.

Question 4.
What is the Phonological Loop (PL)?
Answer:

• The Phonological loop is responsible for verbal information.
• It has two subsystems as follows:
  • The phonological/acoustic store is a passive component of the phonological loop. It holds on verbal information. If not rehearsed, this information is forgotten.
  • The articulatory loop involves rehearsing and refreshing the information, just like our inner voice.

Question 5.
Explain Murdock’s experiment.
Answer:

• Murdock performed experiments to check the recall.
• He asked his subjects to learn a list of words. Later, their recall was tested by a free recall method.
• Murdock found that the subjects could recall the first few (primacy effect) and the last few (recency effect) words prominently. But they got confused with the words in the middle part (serial position effect).

4. Short Notes.

Question 1.
visuospatial Sketch Pad (VSSP)
Answer:

• VSSP handles visual and spatial information. It is responsible for storing speech-based information.
• It has two components, viz. Phonological memory store and Articulatory Subvocal Rehearsal.
• Phonological memory stores can hold traces of acoustic or speech-based information.
• Articulatory Subvocal Rehearsal maintains material in short-term store. Prevention of articulatory rehearsal leads to rapid forgetting.

Question 2.
Recall
Answer:

• The recall is the retrieval of information from LTM with few or no cues. Recall can be in the written form or it can be oral.
• The recall involves remembering a fact, event, or object that is not currently physically present and requires the direct uncovering of information from memory.
• The recall is of two types, viz. free recall and serial recall.
• Free recall is a recollection of the items in the list without their serial order, e.g. we may listen to a lecture and later recall few important points irrespective of the order in which they were presented.
• In serial recall, the material is recalled in the exact order in which it was presented, e.g. when you solve a mathematical problem, you are doing steps one after the other; so, it is serial recall.
• The recall is greatly affected by emotions and motivation at the time of learning and retrieval.
• Also, memory for the free recall is always better than if the subjects are asked to recall in a serial order.

Question 3.
Hermann Ebbinghaus’s experiment
Answer:

• The pioneer of experiments on forgetting was Hermann Ebbinghaus.
• He created several lists of nonsense syllables and learned them. A nonsense syllable is a set of three alphabets where two on both the sides are consonants and the middle one is a vowel, e.g. NOM, GEX.
• He checked his own recall at various periods of time.
• He found out that he forgot 40% of whatever he had learned in the first 20 minutes.
• After one hour, he forgot 60% while after nine hours he forgot a total of 70% of what he had learned.
• After one day, he could recall only around 30% of the material he learned. After that, his recall was steady for a long period of time.
• This experiment proved that we forget most of the things we learn in a short span.

Balbharti Maharashtra State Board Class 11 Psychology Important Questions Chapter 7 Nervous System Important Questions and Answers.

Maharashtra State Board 11th Psychology Important Questions Chapter 7 Nervous System

1A. Complete the following statements with appropriate options.

Question 1.
__________ of the neuron absorbs the food and keeps the cell alive.
(A) Nucleus
(B) Axon
(C) Cell body
Answer:
(A) Nucleus

Question 2.
__________ plays a role mainly in cognition, reward, learning and memory.
(A) Acetylcholine
(B) Dopamine
(C) Serotonin
Answer:
(C) Serotonin

Question 3.
The brain plays an important role in __________ mental processes like thinking, reasoning, and emotions.
(A) higher-order
(B) lower order
(C) neutral
Answer:
(A) higher-order

Question 4.
Cerebellum consists of __________ parts.
(A) two
(B) three
(C) four
Answer:
(A) two

Question 5.
__________ is a vital centre of the brain.
(A) Medulla oblongata
(B) Pons
(C) Forebrain
Answer:
(A) Medulla oblongata

Question 6.
__________ is a bridge between forebrain and hindbrain.
(A) Pons
(B) Midbrain
(C) Cerebrum
Answer:
(B) Midbrain

Question 7.
Cerebrum is the __________ part of the brain.
(A) largest
(B) smallest
(C) insignificant
Answer:
(A) largest

Question 8.
Each hemisphere of the brain is divided into __________ lobes.
(A) four
(B) six
(C) two
Answer:
(A) four

Question 9.
__________ is called as relay station of the brain.
(A) Hippocampus
(B) Thalamus
(C) Amygdala
Answer:
(B) Thalamus

Question 10.
__________ is called as the pleasure centre of our body.
(A) Hypothalamus
(B) Occipital lobe
(C) Spinal cord
Answer:
(A) Hypothalamus

Question 11.
A protein in the brain called __________ is related to Alzheimer’s disease.
(A) beta-amyloid plaque
(B) thyroxin
(C) gonad
Answer:
(A) beta-amyloid plaque

Question 12.
The spinal cord is connected to the periphery through __________ pairs of spinal nerves.
(A) 25
(B) 13
(C) 31
Answer:
(C) 31

Question 13.
The chemical substances secreted by endocrine glands are called __________
(A) hormones
(B) neurons
(C) axons
Answer:
(A) hormones

Question 14.
Hyposecretion of thyroxin leads to cretinism among __________
(A) old people
(B) children
(C) adults
Answer:
(B) children

Question 15.
In the case of __________, a person loses his weight and experiences irritated mood, sleeplessness as well as sweaty palms.
(A) Myxedema
(B) Acromegaly
(C) Grave’s disease
Answer:
(C) Grave’s disease

Question 16.
__________ is secreted by the pancreas.
(A) Glycogen
(B) Cortisone
(C) Progesterone
Answers:
(A) Glycogen

1B. Match the following pairs.

Question 1.

A	B
i. Spinal cord	a. PNS
ii. Autonomic Nervous system	b. Telodendria
iii. Terminal Button	c. Cerebrum
iv. Reticular Formation	d. CNS
	e. The Alarm clock of the body

Answer:

A	B
i. Spinal cord	d. CNS
ii. Autonomic Nervous system	a. PNS
iii. Terminal Button	b. Telodendria
iv. Reticular Formation	e. The alarm clock of the body

1C. State whether the following statements are true or false.

Question 1.
The human nervous system is amongst all living creatures.
Answer:
True

Question 2.
The autonomic nervous system internal activity of the human body.
Answer:
True

Question 3.
Dendrite is a gap between two neurons.
Answer:
False

Question 4.
Glutamate is the chief inhibitory neurotransmitter.
Answer:
False

Question 5.
The brain consists of five major parts.
Answer:
False

Question 6.
The brain stem is divided into medulla oblongata and pons.
Answer:
True

Question 7.
Medulla oblongata receives messages from higher centers of the brain.
Answer:
True

Question 8.
The reticular activation system is a bridge between two hemispheres.
Answer:
False

Question 9.
Midbrain consists of the superior and inferior colliculus.
Answer:
True

Question 10.
Two hemispheres of the brain are connected by a bundle of fibers called the corpus callosum.
Answer:
True

Question 11.
If a person’s left side of the body is paralyzed, neurons from the left side of his body stop functioning.
Answer:
False

Question 12.
The temporal lobe is in the cortex, just above the ears.
Answer:
True

Question 13.
People who exercise regularly have a higher risk of developing Alzheimer’s disease.
Answer:
False

Question 14.
The human brain consumes 40% of the body’s total energy.
Answer:
False

Question 15.
The spinal cord extends from neck to waist.
Answer:
True

Question 16.
Exocrine glands are also called ductless glands.
Answer:
False

Question 17.
In myxedema, a person becomes very huge, lacks motivation, and complains about weakness.
Answer:
True

Question 18.
The adrenal gland is also known as the sex gland.
Answer:
False

1D. Identify which hormones with hyposecretion or hypersecretion would lead to the following conditions.

Question 1.
Grave’s disease
Answer:
Hypersecretion – Thyroxin

2A. Explain the following concepts.

Question 1.
Nervous system
Answer:
The nervous system is the complex network of neurons that carry signals from brain to body and body to brain. Our nervous system consists of two major parts, viz, the central nervous system and the peripheral nervous system.

Question 2.
Cerebral cortex
Answer:
The cerebral cortex is the grey coloured outside cover of the cerebrum. It controls higher-order mental processes such as attention, perception, learning, and memory.

Question 3.
Reflex action
Answer:
Reflex action is an involuntary and nearly instantaneous movement in response to stimulus, e.g. salivation, sneezing, knee jerk, blinking of eyes. These are quick and simple patterns of behaviour without the involvement of the brain.

Question 4.
Glands
Answer:
Glands are specialized groups of cells or organs that secrete chemical substances. There are many glands. All glands fall into two categories, viz. endocrine and exocrine.

3. Answer the following questions in 35-40 words.

Question 1.
Explain the classification of the somatic nervous system.
Answer:

• The somatic nervous system is divided into sensory and motor systems of the body.
• It consists of sensory nerves (afferent nerves) and motor nerves (efferent nerves). Sensory nerves send messages from the body to the brain and motor nerves send messages from the brain to the body.

Question 2.
Why do we get different reactions to every situation?
Answer:

• When a neural message passes from end buttons to the dendrite of another neuron, it has to cross the chemical gap between two neurons (synapse).
• As neurons are not directly connected to each other, we don’t have fixed reactions to every situation.

Question 3.
State any two functions of the brain.
Answer:

• The brain helps to adapt to the environment and tries to analyze, store and synthesize the information it receives.
• The brain plays a crucial role in every aspect of our lives like decision making, emotional experience, and social interactions.

Question 4.
Why are we supposed to wear helmets while riding a bike?
Answer:

• If someone meets with an accident while riding a bike, the person falls back on his head.
• Most of the time, his Medulla oblongata is damaged which will lead to instant death.
• Hence, we are supposed to wear helmets while riding a bike.

Question 5.
Explain the impact of hypersecretion of any four hormones.
Answer:

• Parathyroxin: An individual experiences a feeling of nausea, vomiting sensation. He also feels sleepy and relaxed.
• Cortin or cortisone: An individual experiences increased sexual drive. Females start looking like males.
• Adrenalin and noradrenaline: An individual experiences increased heartbeat, blood pressure, and breathing rate.
• Androgen and testosterone: An individual shows a tendency towards sexual behaviour. He feels very energetic and engages in aggressive behaviour.

Question 6.
Explain the impact of the hyposecretion of any three hormones.
Answer:

• Parathyroxin: An individual lacks motivation and energy. He experiences weakness, muscle cramps, and spasms.
• Cortin or cortisone: An individual feels very lazy, lacks sexual drive, and experiences loss of hunger and weight.
• Androgen and testosterone: Males do not have a desire for sex and their voice remains childlike.

4. Write short notes.

Question 1.
Nervous system
Answer:

• The nervous system is the complex network of neurons that carry signals from brain to body and body to brain.
• The human nervous system is the most complicated yet highly developed among all living creatures.
• Our nervous system consists of two major parts, viz, the central nervous system and the peripheral nervous system.
• The central nervous system consists of the brain and spinal cord while the peripheral nervous system consists of the somatic and autonomic nervous systems.

Question 2.
Brain and nutrition
Answer:

• Nutrition plays an important role in brain functioning. Nutritional deficiency may lead to neurological problems.
• Despite representing only 2% of the body’s total mass, the human brain consumes 20% of the body’s total energy due. to the increased metabolic needs of human beings.
• Nutrition plays a crucial role during developing years so to optimize the functions of the brain. It is also essential during old age to avoid the degeneration of cells.
• Nutrition enhances brain functioning. It prevents as well as helps in treating neurological disorders.
• As evolution took place, human life became more complicated and demanding. As a result, the need for nutrition by the brain kept on increasing.
• Today human brain is exposed to a high level of stress which results in oxidation, Any food which is high in antioxidants (almonds, dark chocolate, onions, berries, mangoes, seafood) helps to control the ill effects of oxidation.

Question 3.
Spinal Cord
Answer:

• The spinal cord is an important part of the central nervous system. It extends from neck to waist.
• Its main function is to send information from the brain to the body and from to the body to the brain.
• It controls reflex actions like salivation, knee jerk, blinking of eyes.
• The spinal cord is connected to the periphery through 31 pairs of spinal nerves.
• Each spinal nerve is joined to the spinal cord through two routes: the dorsal and ventral routes.
• If dorsal routes are injured, we will not have sensations while if ventral routes are injured, we will not be able to move our body and control reflex actions.

5. Answer in 150-200 words.

Question 1.
Write a note on neurons.
Answer:

• Neurons are specialized networks of cells that transmit messages from the brain to the body and from the body to the brain. They are the basic units of the nervous system.
• A neuron consists of dendrites, axon, cell body, and terminal button or telocentric.
• The cell body is the body of neurons. The nucleus of the neuron absorbs the food and keeps the cell alive.
• The neural message comes in through the dendrites. These are branch-like structures.
• The neural message goes out from the axon of the neuron.
• At the end of the axon, there are end buttons. It is a bulb-like structure containing chemicals known as neurotransmitters.
• Neurotransmitters are chemical messengers. The neural message jumps across the synapse in order to reach the dendrite of another neuron.
• When the neural message is passing through, it excites or inhibits the neurotransmitter in it. This chemical reaction decides our reaction to various situations in life.

Question 2.
Explain any five pillars of better brain functioning.
Answer:

• Physical and mental exercise: Exercise improves blood flow and memory. It also stimulates chemical changes in the brain that improve learning, mood, and thinking.
• Tackling medical problems: Hypertension, diabetes, obesity, depression, head trauma, higher cholesterol, and smoking increase the risk of dementia. One can control and reduce this risk by going for regular health check-ups and taking medication if required.
• Sleep and relaxation: Sleep energizes the brain, improves mood and immune system by clearing wastage and toxins from the body. Practicing meditation and managing stress will help to control the age-related decline in brain health.
• Mental fitness: It improves the brain’s functioning and promotes new brain cell growth. This helps to decrease the chances of developing dementia. A person can keep his brain stimulated by solving puzzles, watching stimulating movies, or learning something new.
• Social interaction: It is good for brain health to spend time with others, participate in stimulating conversation, and stay connected with family and friends. Studies have shown that those who interact more show less decline in their memory.

Balbharti Maharashtra State Board Class 11 Psychology Important Questions Chapter 6 Stress Important Questions and Answers.

Maharashtra State Board 11th Psychology Important Questions Chapter 6 Stress

1A. Complete the following statements.

Question 1.
_____________ stress is referred to as a physical or psychological reaction that may lead to illness.
(A) Medical
(B) Emotional
(C) Psychological
Answer:
(A) Medical

Question 2.
_____________ is the base of four Kleshas.
(A) Avidya
(B) Dvesha
(C) Abhinivesha
Answer:
(A) Avidya

Question 3.
In Sanskrit, mental health is explained as _____________
(A) swasthya
(B) avidya
(C) arogya
Answer:
(A) swasthya

Question 4.
According to the _____________ prefix, ‘EU’ means good.
(A) Greek
(B) Latin
(C) Italian
Answer:
(A) Greek

Question 5.
_____________ is known as good stress.
(A) Hypo stress
(B) Distress
(C) Eustress
Answer:
(C) Eustress

Question 6.
_____________ increases the heart rate, elevates blood pressure and boosts energy supplies.
(A) Adrenaline
(B) Dopamine
(C) Cortisol
Answer:
(A) Adrenaline

Question 7.
Hans Selye’s General Adaptation Syndrome Model consists of _____________ stages.
(A) four
(B) five
(C) three
Answer:
(C) three

Question 8.
Conflict is a _____________ word which means ‘striking two things at the same time’.
(A) Latin
(B) Greek
(C) French
Answer:
(A) Latin

Question 9.
Lewin talked about _____________
(A) valance
(B) psychoanalysis
(C) conflicts
Answer:
(A) valance

Question 10.
In _____________ focused coping strategy, stress is reduced by resolving the conflict through work on the task at hand.
(A) emotion
(B) problem
(C) thought
Answer:
(B) problem

Question 11.
At times, a lack of capacity to achieve the goal urges individuals to readjust their goals. This is known as _____________
(A) withdrawal
(B) compromise
(C) attack
Answer:
(B) compromise

Question 12.
_____________ theory of Psychoanalysis is the base of defence mechanisms.
(A) Freud’s
(B) Selye’s
(C) Webster’s
Answer:
(A) Freud’s

Question 13.
_____________ operates on two energies.
(A) Ego
(B) ID
(C) Superego
Answer:
(B) ID

Question 14.
_____________ is an indirect way to deal with stress.
(A) Psychoanalysis
(B) Problem-focused coping
(C) Defence mechanism
Answer:
(C) Defence mechanism

Question 15.
In _____________, the material can be recalled up to a certain extent.
(A) suppression
(B) repression
(C) sublimation
Answer:
(A) suppression

1B. Match the following pairs.

Question 1.

A	B
i. Avidya	a. Ego
ii. Asmita	b. Repulsion
iii. Raga	c. Ignorance
iv. Dvesha	d. Lust for life
v. Abhinivesha	e. Attraction

Answer:

A	B
i. Avidya	c. Ignorance
ii. Asmita	a. Ego
iii. Raga	e. Attraction
iv. Dvesha	b. Repulsion
v. Abhinivesha	d. Lust for life

1C. State whether the following statements are true or false. If false, correct them. If true, explain why?

Question 1.
Different people deal with stress in different ways.
Answer:
True
Explanation: Different people deal with stress in different ways depending upon their genetic predisposition and environmental conditions.

Question 2.
Dopamine and oxytocin hormones are associated with negative feelings.
Answer:
False
Reason: Dopamine and oxytocin hormones are associated with positive feelings.

Question 3.
Depression is an example of chronic distress.
Answer:
True
Explanation: Depression is an example of chronic distress as it causes constant changes in moods for a long period of time. A depressed person experiences recurrent negative stress.

Question 4.
Hans Selye asserted that stress is always harmful.
Answer:
False
Reason: Hans Selye asserted that the stress of creative and successful work is beneficial whereas that of failure and humiliation is harmful.

Question 5.
External stressors result in peace of mind.
Answer:
False
Reason: External stressors result in frustration, anger, and disappointment.

Question 6.
The value of the goal differs from person to person.
Answer:
True
Explanation: The value of the goal is subjective. A goal may be attractive or unattractive based on whether an individual wants to achieve it or avoid it.

Question 7.
In avoidance-avoidance conflict, an individual is repelled by both goals.
Answer:
True
Explanation: Avoidance-Avoidance conflict involves two unattractive goals with negative values.

1D. Identify the odd item from the following.

Question 1.
Avidya, Arogya, Asmita, Abhinivesha
Answer:
Arogya

Question 2.
Fatigue, Burn out, Depression, Irritability, Anxiety
Answer:
Irritability

Question 3.
Noise, crowding, Strict parents, Weak economic condition, Hunger
Answer:
Hunger

1E. Identify the conflict of motive that is experienced by the person in the following situation.

Question 1.
Ajit likes two cars but he has enough money to buy only one of them.
Answer:
Approach – Approach conflict

Question 2.
Nishant must do his mathematics homework which he dislikes or get a beating from his parents.
Answer:
Avoidance – Avoidance conflict

Question 3.
Sartaj has to choose between two girls for marriage. One is good-looking but boring. The other one is fun but short.
Answer:
Double Approach – Avoidance conflict

Question 4.
Ami would love to buy a new house but it will create a burden on the family’s financial budget.
Answer:
Approach – Avoidance conflict

Question 5.
Shreya has to decide between two appealing destinations for her vacation.
Answer:
Approach – Approach conflict

Question 6.
Rita either has to be late for work or break traffic rules by driving during the red light.
Answer:
Avoidance – Avoidance conflict

Question 7.
Nisha has a choice between two jobs. One is far away but pays well. The other one is close to her house but has no room for advancement.
Answer:
Double Approach – Avoidance conflict

1F. Identify the defense mechanism used in each of the following examples.

Question 1.
Alisha, who was sexually abused as a child, cannot remember the abuse at all.
Answer:
Repression

Question 2.
Ritesh dresses and acts like Spiderman, his favourite superhero.
Answer:
Identification

Question 3.
Suresh hates his neighbour but believes that his neighbour hates him.
Answer:
Projection

Question 4.
Rajesh, who is very aggressive, becomes a football player.
Answer:
Sublimation

Question 5.
Sanjana gets reprimanded by her boss and goes home and fights with her husband.
Answer:
Displacement

Question 6.
Sanket who is cut off from a sports team fantasizes about winning the Olympics.
Answer:
Daydreaming

Question 7.
Shruti is attracted to her sister’s husband but denies this and believes that her sister’s husband is attracted to her.
Answer:
Projection

Question 8.
After being rejected by a prestigious university, Harshil explains that he is glad because he would be happier at a smaller, less competitive college.
Answer:
Rationalization

Question 9.
Neha really admires Priya, the most popular girl in school and tries to copy her behaviour and dressing style.
Answer:
Identification

Question 10.
Three years after being hospitalized, Sonali can remember only vague details about the event.
Answer:
Repression

Question 11.
Angered by her neighbour’s hateful comment, Ekta spanks her daughter for accidentally spilling milk.
Answer:
Displacement

2A. Explain the following concepts.

Question 1.
Stress
Answer:
The word stress is derived from the Latin word ‘stringi’ which means ‘to be drawn tight’. Stress refers to the discomfort experienced by an individual in demanding situations. It arises when an individual is able to mobilize lesser resources than the situation demands.

Question 2.
Leisure
Answer:
Leisure is quality time spent away from work, domestic duties, studies, after a heavily exhausting period. It has relaxing and recreational qualities. We have choice and freedom in our leisure time.

Question 3.
Cortisol
Answer:
Cortisol is the primary stress hormone. It increases sugar (glucose) in the bloodstream, enhancing the brain’s use of glucose and increases the availability of substances that repair tissues.

Question 4.
Stressors
Answer:
Stressors are environmental conditions, external stimuli, or events that cause stress to an organism. There are two types of stressors, viz. internal stressors and external stressors.

Question 5.
Frustration
Answer:
Frustration is a common emotional response related to anger and disappointment. When an individual is highly motivated to achieve something and when his goal-directed behaviour is blocked by an obstacle, it results in frustration.

Question 6.
Id
Answer:
Id is the most primitive storehouse of our biological energy. Id has psychic energy. It operates on the pleasure principle and demands immediate gratification of desires.

Question 7.
Defense mechanisms
Answer:
Defense mechanisms are an indirect way to combat stress. These are the unconscious strategies used to protect the ego from shattering due to unacceptable and harsh reality. It is a stop-gap arrangement that provides some time for the person to come to terms with reality. It is a face-saving device.

2B. Compare and contrast with examples.

Question 1.
Acute distress and Chronic distress
Answer:

• Acute distress is an intense, short-term negative stress while chronic distress is a long-lasting, recurrent negative distress.
• Acute distress occurs when there is a sudden change in routine or when we experience panic or threat, e.g. traffic jam, accident. Chronic distress is the worst type of stress-causing constant changes in routine for a long period of time. e.g. illness of a family member, death of a spouse.

3. Answer the following questions in around 35-40 words each.

Question 1.
What are the effects of hypo stress?
Answer:
Hypo stress is an insufficient amount of stress which is caused when a person has nothing to do at all. Its effects are as follows:

• It leads to boredom.
• It causes feelings of restlessness.
• People become demotivated and unenthusiastic.

Question 2.
What are the ill effects of distress?
Answer:
Distress is a negative type of stress. Its ill effects are as follows:

• Our body is flooded with emergency response hormones such as adrenaline and cortisol.
• It can cause physical conditions like headaches, digestive issues, and sleep disturbances.

Question 3.
Identify the possible reactions to Approach-Avoidance conflict.
Answer:
The three possible reactions to Approach-Avoidance conflict are:

• One may give importance to the positive value.
• One may be very cautious about the negative value.
• One may leave the goal altogether to avoid the situation.

Question 4.
Explain the impact of excessive use of defense mechanisms.
Answer:

• Excessive use of defense mechanisms leads to a habit of escaping from reality and indulgence in falsehood about one’s own self.
• However, reality chases, and sooner or later one has to face it.
• At such a point, the ego is no longer protected and it may lead to symptoms of mental disorders.

4. Write short notes in 35-40 words each.

Question 1.
Anxiety
Answer:

• Anxiety is a state where a person may feel that something is wrong and will experience symptoms like palpitation, rapid heart rate, sweaty palms, and dry throat.
• Two types of anxiety in Freud’s theory are:
  • Neurotic anxiety: Here, id and ego are in conflict with each other
  • Moral anxiety: Here, the superego and ego are in conflict with each other

For your understanding

• Neurotic anxiety comes from the unconscious fear that the basic impulses of the id will take control of the person, leading to eventual punishment from expressing the id’s desires.
• Moral anxiety comes from the superego. It appears in the form of fear of violating moral codes or values, leading to feelings of guilt and shame.

Question 2.
Defense mechanisms
Answer:

• Defense mechanisms are an indirect way to combat stress.
• They are unconscious strategies used to protect the ego from breaking due to unacceptable/harsh reality. It functions as a shock absorber.
• It should be used moderately as its excessive use leads to a habit of escaping from reality.
• Some commonly used defense mechanisms are projection, displacement, and daydreaming.

6. Answer the following questions in 150-200 words each.

Question 1.
Elaborate on the General Adaptation Syndrome model proposed by Hans Selye.
Answer:

• Hans Selye, the father of stress research, introduced the General Adaptation Syndrome (GAS) model in 1936 showing the effects of stress on the human body.
• He asserted that stress is a major cause of disease since chronic distress causes long term chemical changes.
• The GAS model may be defined as the manifestation of stress in the whole body. It consists of three stages:

a. Alarm stage: It is the first reaction to stress. The organism recognizes that there is a danger and prepares to deal with the threat by a ‘fight or flight response. This natural reaction provides energy to the body to deal with stressful situations.

b. Resistance stage: After the initial shock, the body begins to repair itself. In this phase, it remains on high alert for a while. If one overcomes the stress, the body continues to repair itself until the hormone level, heart rate, and blood pressure come to normal. Signs of this stage include irritability, frustration, poor concentration.

c. Exhaustion stage: If stress is not resolved in the resistance stage, a person enters the exhaustion stage. Here, the body’s ability to cope up becomes less. The individual may collapse quickly and the body’s immune system, as well as the ability to resist stress, diminishes. Signs of the exhaustion stage include fatigue, burnout, depression, and anxiety.

Question 2.
Explain aspects of Freud’s psychoanalysis theory.
Answer:
According to Freud’s theory of psychoanalysis, our personality is controlled by three aspects. They are:

• Id: It operates on the pleasure principle and demands immediate gratification of desires. It operates on primary process thinking where logical rules are not applied. It operates on two energies: Libido (sexual energy) and Thanatos (destructive energy).
• Ego: It operates on the reality principle and has better problem-solving abilities as compared to Id. Ego indulges in secondary process thinking and it knows how and when to satisfy desires.
• Superego: It internalizes the moral values of society (do’s and don’ts) and also includes the ‘rights’ and ‘wrongs’ we unknowingly learn from our role models. It helps us to control impulses coming from the Id and makes our behaviour less selfish and more virtuous. According to this theory, our reactions to situations depend upon the interaction of these three systems.

Question 3.
Explain the different types of defense mechanisms.
Answer:
Some of the defense mechanisms described by Dr. Sigmund Freud are as follows:

• Repression: It is an unconscious mechanism employed by the ego to keep disturbing or threatening thoughts outside our conscious awareness. These are the thoughts that would result in feelings of guilt from the superego.
• Displacement: It is the redirection of an impulse onto a less powerful target. A person cannot retaliate against the actual source of anger and so, redirects his anger on a less threatening object.
• Projection: It involves the individual attributing his own thoughts, feelings, and motives to another person.
• Sublimation: It is one of the most adaptive defense mechanisms as it can transform negative anxiety into positive energy. A person uses sublimation to redirect his motivation into more acceptable and productive tasks.
• Identification: Flere, by adopting another person’s mannerisms, language, patterns, etc., a person tries to imitate his character traits and starts behaving like another person.
• Daydreaming: When life appears to be distressing, people often use fantasy as a way of escaping reality. This is called daydreaming.
• Rationalization: It occurs when a person attempts to explain or create excuses for his failure. In doing so, an individual is able to avoid accepting the true cause or reason for his failure.

Some other important defense mechanisms are:

• Denial: It is an outright refusal to admit or recognize that something has occurred or is currently occurring. e.g. alcoholics often deny that their behaviour is problematic.
• Compensation: It means people overachieve in one area to compensate for failures in another. e.g. a student who fails in studies may compensate by becoming a champion in athletics.
• Intellectualization: It works to reduce anxiety by thinking about events in a cold and clinical way. e.g. a person diagnosed with a terminal illness might focus on learning everything about the disease in order to avoid distress and remain distant from the reality of the situation.

Balbharti Maharashtra State Board Class 11 Psychology Important Questions Chapter 5 Healthy Me – Normal Me Important Questions and Answers.

Maharashtra State Board 11th Psychology Important Questions Chapter 5 Healthy Me – Normal Me

1A. Complete the following statements.

Question 1.
A ‘well-adjusted individual’ would indicate a __________ person.
(A) normal
(B) thoughtful
(C) healthy
(D) obedient
Answer:
(A) normal

Question 2.
Getting betrayed but not allowing it to hurt you for long time shows __________
(A) realistic perception of the world
(B) openness to new experiences
(C) high intellectual ability
(D) high self-esteem
Answer:
(A) realistic perception of the world

Question 3.
__________ intelligence is the ability to understand one’s own and others’ emotions.
(A) Emotional
(B) Social
(C) Cultural
(D) Intellectual
Answer:
(A) Emotional

Question 4.
There are __________ components of EI.
(A) 3
(B) 4
(C) 5
(D) 6
Answer:
(C) 5

Question 5.
__________ is your attitude towards yourself.
(A) Self-esteem
(B) Humanistic perspective
(C) Self-awareness
(D) Emotional intelligence
Answer:
(A) Self-esteem

Question 6.
A scale to measure self-esteem was developed by __________
(A) John Mayer
(B) Peter Salovey
(C) Morris Rosenberg
(D) Daniel Goleman
Answer:
(C) Morris Rosenberg

Question 7.
Sometimes traumatic effects continue for a longer period and a person can get __________
(A) burn out
(B) churn out
(C) fag out
(D) dire out
Answer:
(A) burn out

Question 8.
Pinky is 32 years old and still needs her mother to make her hair plaits every day. This is __________ behaviour.
(A) abnormal
(B) normal
(C) well-adjusted
(D) healthy
Answer:
(A) abnormal

Question 9.
Having poor energy levels and thus not being able to perform expected responsibilities on any given day is a sign of __________
(A) low self-esteem
(B) impaired functioning
(C) low confidence
(D) poor emotional intelligence
Answer:
(B) impaired functioning

Question 10.
Childhood memories are linked to __________ perspective.
(A) evolutionary
(B) psychoanalytic
(C) biological
(D) cognitive
Answer:
(B) psychoanalytic

1B. State whether the following statements are true or false and justify your answer with reason.

Question 1.
Today EQ is considered equally important as IQ.
Answer:
True
Explanation: Emotional intelligence is as important as intellectual abilities since it enables a person to live a balanced and happy life.

Question 2.
Being respectful, trusting, and supporting others are components of self-esteem.
Answer:
False
Reason: All these are components of maintaining a healthy relationship with others.

Question 3.
Adolescents face many challenges.
Answer:
True
Explanation: Adolescents face many challenges such as gender identity issues, sexual orientation issues, bullying, etc.

Question 4.
Morris Rosenberg developed the EI model.
Answer:
False
Reason: John D. Mayer co-developed the model of emotional intelligence with Peter Salovey.

1C. Identify the odd item from the following.

Question 1.
Respect, Fairness, Trust, Threatening behaviour
Answer:
Threatening behaviour

Question 2.
Identity crisis, Bullying, Inferiority complex, Genetic problems
Answer:
Genetic problems

1D. Complete the following table.

Question 1.

1. Michel Beldoch	_________________
2. _______________	Self-control
3. Empathy	_________________
4. Self-esteem	_________________
5. _______________	Diathesis
6. Androgynous	_________________
7. _______________	10th October

Answer:

1. Michel Beldoch	Term ‘Emotional Intelligence’
2. Self-regulation	Self-control
3. Empathy	Putting yourself in other’s shoes
4. Self-esteem	Sense of self-worth
5. Predisposition to disorder	Diathesis
6. Androgynous	Display of masculine and feminine traits
7. World Mental Health Day	10th October

2A. Explain the following concepts.

Question 1.
Emotional intelligence
Answer:
Emotional intelligence is the ability to perceive one’s own and other’s emotions, to discriminate among them, and to use that information to guide one’s thinking and action. It includes the ability to control emotions and handle crucial situations in an appropriate way. Individuals with high emotional intelligence are well-adjusted individuals.

Question 2.
Self-regulation
Answer:
Self-regulation refers to controlling the expression of emotions. It is the ability to express oneself appropriately at the right place and the right time. Emotionally intelligent and well-adjusted individuals are able to control their emotions.

Question 3.
Social skill
Answer:
Social skill is the ability to interact well with others. Some important social skills are active listening, verbal communication skills, nonverbal communication skills, leadership, and persuasiveness. Being helpful, respecting other’s opinions, accepting criticism, being positive, etc. also demonstrate good social skills. These skills enable a person to develop healthy relations with others.

Question 4.
Self-esteem
Answer:
Self-esteem refers to the sense of self-worth and personal value. According to Rosenberg, it is your attitude towards yourself. Well-adjusted individuals have high self-esteem.

Question 5.
Cognitive Psychology
Answer:
Cognitive Psychology is an area of psychology that focuses on mental processes such as memory, thinking, problem-solving, language, and decision-making.

Question 6.
Gender identity
Answer:
Gender identity is a perception of one’s own gender which may or may not be corresponding to their birth sex.

3. Write short notes in 50-60 words each.

Question 1.
Components of emotional intelligence
Answer:

• Self-awareness: The ability to be aware of own emotions, wants, motivations, actions, strengths, and weaknesses.
• Self-regulation: Ability to control emotions and restrain inappropriate actions.
• Self-motivation: Ability to push oneself towards a goal that one has set, without any external reward or punishment.
• Social skills: Ability to comfortably and co-operatively interact and communicate with others.
• Empathy: Ability to put oneself in other’s shoes and understand their pain, loss, grief, or distress.

Question 2.
Diathesis model
Answer:

• The diathesis model explains that those who are already genetically predisposed towards a particular disorder, are more likely to show abnormal behaviour if exposed to environmental stress.
• The disorder is a result of both biological factors (nature) and life experiences (nurture).
• Stressful environmental experiences could be childhood abuse, family conflict, divorce, or death.
• The model states that the stronger the diathesis, the lesser is the less stress required to trigger abnormal behaviour.
• Hence, a similar situation leads to different reactions by different people.

Question 3.
Biopsychosocial model
Answer:

• According to this model, abnormal behaviour results from biological, psychological, and socio-cultural factors.
• Biological factors include age, genetics, physical health.
• Psychological factors include mental and emotional health, beliefs, and expectations.
• Sociocultural factors include interpersonal relations and socioeconomic factors.
• If a person who has a biological predisposition towards abnormality, gets to live in a protective and nurturing environment, there are fewer chances of developing abnormal behaviour.
• Conversely, if he is placed in an environment detrimental to his mental and social well-being, he will show abnormal behaviour.

4. Answer the following questions in 150-200 words.

Question 1.
Explain the needs of adolescents.
Answer:
The ten needs of adolescents can be explained as follows:

• I need to understand myself and be myself (self-awareness and independence)
• I need to have fun, enjoy life, and fill my mind and time with good things, (enjoyment and constructive use of resources)
• I need to know that I am important I am called to be a hero, (power and self-esteem)
• I need to love and be loved just as I am. (need to feel wanted)
• I need to have friends and be a friend, (sense of belonging)
• I need to be a part of something with my friends, (sense of belonging)
• I need to have positive role models for my life, (having someone to look up to)
• I need to understand the meaning of my sexuality. (understanding sexual orientation)
• I need to understand the world around me. (awareness of world)
• I need to have a new relationship with Almighty: deep, real, captivating, and foundation of truth. (strong relation with almighty).

Balbharti Maharashtra State Board Class 11 Psychology Important Questions Chapter 4 Human Development Important Questions and Answers.

Maharashtra State Board 11th Psychology Important Questions Chapter 4 Human Development

1A. Complete the following statements.

Question 1.
The term ‘____________’ means a progressive series of changes that occur as a result of maturation and experience.
(A) Human Development
(B) Personal Growth
(C) Maturity
Answer:
(A) Human Development

Question 2.
____________ refers to the physical changes like increase in size and weight as the age advances.
(A) Maturation
(B) Growth
(C) Development
Answer:
(B) Growth

Question 3.
____________ psychologists study the characteristics and patterns of developmental stages.
(A) Clinical
(B) Developmental
(C) Industrial
Answer:
(B) Developmental

Question 4.
The duration of prenatal period is ____________ to 290 days.
(A) 251
(B) 238
(C) 283
Answer:
(B) 238

Question 5.
The last stage in prenatal period is called ____________ stage.
(A) germinal
(B) fetal
(C) embryonic
Answer:
(B) fetal

Question 6.
The ____________ sleeps for around 18 to 20 hours.
(A) neonate
(B) embryo
(C) foetus
Answer:
(A) neonate

Question 7.
____________ is also called preschool age or age of curiosity.
(A) Early childhood
(B) Infancy
(C) Late childhood
Answer:
(A) Early childhood

Question 8.
Parents call early childhood as ____________ age.
(A) toy
(B) play
(B) pre-gang
Answer:
(A) toy

Question 9.
Educators regard ____________ as a critical period in the achievement drive.
(A) late childhood
(B) early childhood
(C) adulthood
Answer:
(A) late childhood

Question 10.
The rate of adolescent suicide is higher for ____________
(A) girls
(B) boys
(C) transgenders
Answer:
(B) boys

Question 11.
World suicide prevention day is observed on ____________ every year.
(A) 10th September
(B) 8th October
(C) 11th December
Answer:
(A) 10th September

Question 12.
Late adulthood is the time of ‘____________’.
(A) Empty Nest
(B) High achievement
(C) Low curiosity
Answer:
(A) Empty Nest

Question 13.
____________ is associated with forced leisure.
(A) Late adulthood
(B) Old age
(C) Early adulthood
Answer:
(B) Old age

Question 14.
____________ life begins in old age.
(A) Retirement
(B) Relaxation
(C) Depressed
Answer:
(A) Retirement

1B. Match the following pairs.

Question 1.

A	B
1. Prenatal stage	a. Birth to two weeks
2. Neonatal stage	b. 60 years till death
3. Early childhood	c. Six years to 10-12 years
4. Late childhood	d. 40 years to 60 years
5. Adolescence	e. Conception to birth
6. Early adulthood	f. 12-14 years to 20-21 years
7. Late adulthood	g. Two years to six years
8. Old age	h. 21 years to 40 years

Answers:

A	B
1. Prenatal stage	e. Conception to birth
2. Neonatal stage	a. Birth to two weeks
3. Early childhood	g. Two years to six years
4. Late childhood	c. Six years to 10-12 years
5. Adolescence	f. 12-14 years to 20-21 years
6. Early adulthood	h. 21 years to 40 years
7. Late adulthood	d. 40 years to 60 years
8. Old age	b. 60 years till death

1C. State whether the following statements are true or false.

Question 1.
The life of an individual is real and significant just as the geographical age of the earth.
Answer:
True

Question 2.
Growth is a part of development.
Answer:
True

Question 3.
All developmental changes are genetic by nature.
Answer:
False

Question 4.
Growth, maturation, and development are parallel concepts.
Answer:
True

Question 5.
Maturation is dependent on the environment as well as training.
Answer:
False

Question 6.
Infants develop control of the head and face movements within the first two months after birth.
Answer:
True

Question 7.
By the end of the embryonic stage, the zygote gets attached to the wall of the uterus.
Answer:
False

Question 8.
During infancy, rapid physical and motor development takes place.
Answer:
True

Question 9.
Child experiences stranger anxiety in late childhood.
Answer:
False

Question 10.
James Marcia’s approach discusses identity development in adulthood.
Answer:
False

Question 11.
World suicide prevention day is observed since 2003.
Answer:
True

Question 12.
Erikson asserts that people in late adulthood get experiences and society expects them to be more constructive.
Answer:
True

Question 13.
Elderly people have a minority-group status.
Answer:
True

Question 14.
Most stereotypes about old people are favourable to them.
Answer:
False

2. Explain the following concepts.

Question 1.
Write about internal factors affecting human development.
Answer:

• Internal factors affecting human development are basically related to heredity characteristics or genes.
• Some of the internal factors are Predisposition to certain diseases, immunity, over or under secretion of hormones.
• Internal factors determine the inherent physical and mental characteristics of an individual and thereby, have a crucial impact on the development.

Question 2.
Write about external factors affecting human development.
Answer:

• External factors affecting human development are basically related to nature or the environment.
• Some of the external factors are Parental attitudes and expectations, peer group and
  interpersonal relations, mass media, and overall social environment.
• External factors shape the thoughts, attitudes, and beliefs of an individual and thereby, have a significant effect on development.

3. Answer the following questions in around 35-40 words each.

Question 1.
Explain the cephalocaudal principle of development.
Answer:

• The cephalocaudal principle describes the direction of growth and development.
• It states that the development proceeds from the head to toe.
• According to this principle, the child gains control of the head first, then the arms, and then the legs.

Question 2.
Explain the proximodistal principle of development.
Answer:

• The proximodistal principle describes the direction of development.
• It states that the development proceeds from the center of the body to outward.
• This means that the spinal cord develops before outer body parts.
• The child’s arms develop before the hands and the hands and feet develop before the fingers and toes.

Question 3.
State any four changes in infancy.
Answer:

• During infancy, rapid physical and motor development takes place.
• Within two months, the child can turn his head.
• A child can sit and walk with support by nine months.
• The child starts walking independently by around 12 months of age.

Question 4.
Explain any four changes in early childhood.
Answer:

• The child develops control over his muscles.
• The child becomes physically independent.
• The physical territory of the child increases and he automatically learns about social behaviour.
• The child asks a number of questions to others.

Question 5.
Explain any three changes in late childhood.
Answer:

• The fundamental skills of reading, writing, and calculations develop at this age.
• Hand-eye coordination develops along with micro-skills.
• Even cognitive abilities like thinking and reasoning begin to develop.

Question 6.
Explain any two characteristics of late adulthood.
Answer:

• Late adulthood is a time of achievement. Erikson says that at this age people get experiences and society expects them to be more constructive.
• Late adulthood is a time of evaluation where people evaluate themselves by their achievements and previous aspirations.

4. Write short notes.

Question 1.
Characteristics of infancy
Answer:
Infancy ranges from two weeks after birth to two years. The characteristics of infancy are as follows:

• It is a foundation age because many behavioural patterns, attitudes, and emotions develop during this age.
• There is rapid growth and many changes during this stage. These changes are qualitative as well as quantitative.
• Infancy is an age of independence due to control of body movements. It enables an infant to sit, stand and walk, and manipulate objects around him.
• Due to developed interests and abilities, infancy is the age of increased individuality.
• It is the beginning of socialization since the infant goes from being asocial to social.

Question 2.
Adolescence
Answer:

• The age between late childhood and youth is called adolescence. This age ranges between 12-13 years to 19-20 years.
• During this age, rapid physical development takes place. This stage begins with puberty.
• Height and weight of adolescent increases. Menarche in girls and nocturnal emission in boys occur due to the maturation of sex organs.
• Secondary sex characteristics develop during this stage, e.g. breast development among girls, growth of mustache, and pubic hair among boys.
• Also, the search for identity and independence develops among adolescents. Their thoughts are more logical, abstract, and idealistic.
• Adolescents like to spend more time with their friends.
• Adolescents face a number of problems like identity crisis, addiction, and depression.

Question 3.
Old age
Answer:
Old age ranges from 60 years to death. Old people have to adjust to their environment. In some cases, the death of a spouse leads to loneliness, The characteristics of old age are as follows:

• Old age is a period of decline in physical and mental capacities. Individuals in this stage also face certain health issues.
• There are individual differences in the impact of aging.
• Old age is judged by different criteria, e.g. society tends to judge age in terms of physical appearance and activities.
• There are many stereotypes about old people.
• Most stereotypes are unfavourable.
• Elderly people have a minority-group status.
• Aging requires role changes.
• Adjustment is poor during old age.
• The desire to be young is seen in old age.

5. Answer the following questions in 150-200 words.

Question 1.
Explain three prenatal stages.
Answer:
A period between conception till birth is called a prenatal period. During this period, major developmental changes take place in a very rapid manner. This development goes through three stages as follows:

1. Germinal stage: It ranges from conception to two weeks. Within few hours after conception, the zygote starts a journey down the fallopian tube to the uterus; where it begins the process of cell duplication.
In this process, the zygote divides itself into two cells and then goes on duplicating itself. By the end of this period, the zygote gets attached to the wall of the uterus.

2. Embryonic stage: It starts from the third week after conception and continues till the ninth week. It is a time when the mass of cells (embryo) becomes distinct from a human. This stage plays a crucial role in the development of the brain. Almost all internal and external organs develop by the end of this period.

3. Fetal stage: It begins during the ninth week and lasts until birth. This period is marked by more important changes in the brain. The body parts and structures established in the embryonic stage continue to develop during this stage.

Question 2.
Explain the characteristics of adolescence.
Answer:
The age between late childhood and youth is called adolescence. This age ranges between 12-13 years to 19-20 years. The characteristics of adolescence are as follows:

• Adolescence is an important period that has an immediate effect on the attitude and behavior of an adolescent.
• Adolescence is a transitional period, i.e. it is a bridge between childhood and adulthood.
• Adolescence is a period of physical changes.
• Adolescence is an age of challenges. During childhood, the majority of problems are solved by parents and teachers but an adolescent wants to be independent.
• Adolescence is a time for searching for one’s own identity.
• Adolescence is a time of unrealistic ideas. They have a tendency to look at life through rose-tinted glasses and engage in daydreaming. As a result, they face problems like lack of concentration.
• Adolescence is a threshold of adulthood.

Question 3.
Explain Marcia’s approach to identity development.
Answer:
James Marcia’s approach to identity development during adolescence is as follows:

• Identity foreclosure: Here, adolescents just accept others’ decisions about what is best for them. e.g. a doctor’s son becomes a doctor. These adolescents are happy and self-satisfied. They tend to be authoritarian and have a need for self-approval.
• Identity diffusion: Here, adolescents neither explore nor commit to the alternatives. They are socially withdrawn. These adolescents appear carefree but their lack of commitment impairs their ability to form close relationships.
• Moratorium: Here, adolescents explore some alternatives but make no commitments. They experience high anxiety and psychological conflict. They are lively and appealing and seek intimacy with others
• Identity achievement: Here, adolescents explore and search about ‘who they are and ‘what they do’. Teens who have reached this stage tend to be psychologically healthier, higher in achievement, motivation, and moral reasoning.
• Some adolescents shift among all the above-mentioned categories but for most of them identity gels in late teens and early twenties.