Maharashtra Board 12th Commerce Maths Solutions Chapter 8 Differential Equation and Applications Ex 8.5

Balbharati Maharashtra State Board Std 12 Commerce Statistics Part 1 Digest Pdf Chapter 8 Differential Equation and Applications Ex 8.5 Questions and Answers.

Maharashtra State Board 12th Commerce Maths Solutions Chapter 8 Differential Equation and Applications Ex 8.5

Solve the following differential equations.

Question 1.
$\frac{d y}{d x}+y=e^{-x}$
Solution:
$\frac{d y}{d x}+y=e^{-x}$ …….(1)
This is the linear differential equation of the form

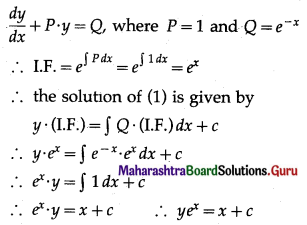
This is the general solution.

Question 2.
$\frac{d y}{d x}$ + y = 3
Solution:
$\frac{d y}{d x}$ + y = 3
This is the linear differential equation of the form

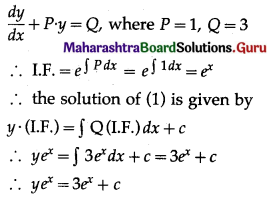
This is the general solution.

Question 3.
x$\frac{d y}{d x}$ + 2y = x² . log x.
Solution:
x$\frac{d y}{d x}$ + 2y = x² . log x
∴ $\frac{d y}{d x}+\left(\frac{2}{x}\right) \cdot y=x \cdot \log x$ …….(1)
This is the linear differential equation of the form


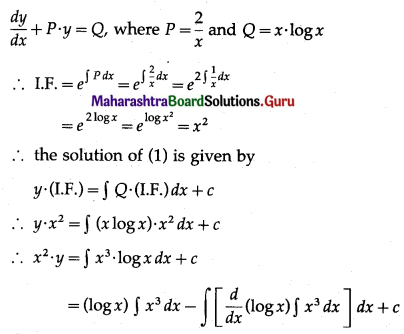
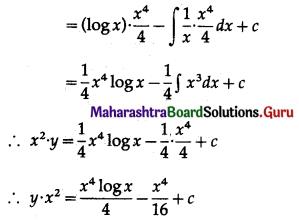
This is the general solution.

Question 4.
(x + y)$\frac{d y}{d x}$ = 1
Solution:
(x + y) $\frac{d y}{d x}$ = 1
∴ $\frac{d x}{d y}$ = x + y
∴ $\frac{d x}{d y}$ – x = y
∴ $\frac{d x}{d y}$ + (-1) x = y ……(1)
This is the linear differential equation of the form

This is the general solution.

Question 5.
y dx + (x – y²) dy = 0
Solution:
y dx + (x – y²) dy = 0
∴ y dx = -(x – y²) dy
∴ $\frac{d x}{d y}=-\frac{\left(x-y^{2}\right)}{y}=-\frac{x}{y}+y$
∴ $\frac{d x}{d y}+\left(\frac{1}{y}\right) \cdot x=y$ ……(1)
This is the linear differential equation of the form

This is the general solution.

Question 6.
$\frac{d y}{d x}$ + 2xy = x
Solution:
$\frac{d y}{d x}$ + 2xy = x ………(1)
This is the linear differential equation of the form

This is the general solution.

Question 7.
(x + a) $\frac{d y}{d x}$ = -y + a
Solution:
(x + a) $\frac{d y}{d x}$ + y = a
∴ $\frac{d y}{d x}+\left(\frac{1}{x+a}\right) y=\frac{a}{x+a}$ ……..(1)
This is the linear differential equation of the form

This is the general solution.

Question 8.
dy + (2y) dx = 8 dx
Solution:
dy + (2y) dx = 8 dx
∴ $\frac{d y}{d x}$ + 2y = 8 …….(1)
This is the linear differential equation of the form

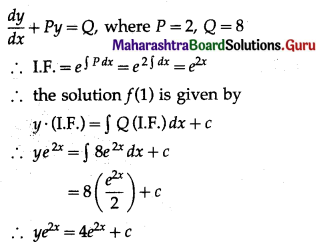
This is the general solution.