Balbharti Maharashtra State Board Class 10 Maths Solutions covers the Practice Set 2.1 Geometry 10th Class Maths Part 2 Answers Solutions Chapter 2 Pythagoras Theorem.
10th Standard Maths 2 Practice Set 2.1 Chapter 2 Pythagoras Theorem Textbook Answers Maharashtra Board
Class 10 Maths Part 2 Practice Set 2.1 Chapter 2 Pythagoras Theorem Questions With Answers Maharashtra Board
Question 1.
Identify, with reason, which of the following are Pythagorean triplets.
i. (3,5,4)
ii. (4,9,12)
iii. (5,12,13)
iv. (24,70,74)
v. (10,24,27)
vi. (11,60,61)
Solution:
i. Here, 52 = 25
32 + 42 = 9 + 16 = 25
∴ 52 = 32 + 42
The square of the largest number is equal to the sum of the squares of the other two numbers.
∴ (3,5,4) is a Pythagorean triplet.
ii. Here, 122 = 144
42 + 92= 16 + 81 =97
∴ 122 ≠ 42 + 92
The square of the largest number is not equal to the sum of the squares of the other two numbers.
∴ (4,9,12) is not a Pythagorean triplet.
iii. Here, 132 = 169
52 + 122 = 25 + 144 = 169
∴ 132 = 52 + 122
The square of the largest number is equal to the sum of the squares of the other two numbers.
∴ (5,12,13) is a Pythagorean triplet.
iv. Here, 742 = 5476
242 + 702 = 576 + 4900 = 5476
∴ 742 = 242 + 702
The square of the largest number is equal to the sum of the squares of the other two numbers.
∴ (24, 70,74) is a Pythagorean triplet.
v. Here, 272 = 729
102 + 242 = 100 + 576 = 676
∴ 272 ≠ 102 + 242
The square of the largest number is not equal to the sum of the squares of the other two numbers.
∴ (10,24,27) is not a Pythagorean triplet.
vi. Here, 612 = 3721
112 + 602 = 121 + 3600 = 3721
∴ 612 = 112 + 602
The square of the largest number is equal to the sum of the squares of the other two numbers.
∴ (11,60,61) is a Pythagorean triplet.
Question 2.
In the adjoining figure, ∠MNP = 90°, seg NQ ⊥ seg MP,MQ = 9, QP = 4, find NQ.
Solution:
In ∆MNP, ∠MNP = 90° and [Given]
seg NQ ⊥ seg MP
NQ2 = MQ × QP [Theorem of geometric mean]
∴ NQ = \(\sqrt { MQ\times QP }\) [Taking square root of both sides]
= \(\sqrt { 9\times 4 } \)
= 3 × 2
∴NQ = 6 units
Question 3.
In the adjoining figure, ∠QPR = 90°, seg PM ⊥ seg QR and Q – M – R, PM = 10, QM = 8, find QR.
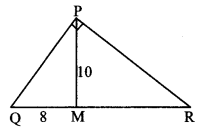
Solution:
In ∆PQR, ∠QPR = 90° and [Given]
seg PM ⊥ seg QR
∴ PM2 = OM × MR [Theorem of geometric mean]
∴ 102 = 8 × MR
∴ MR = \(\frac { 100 }{ 8 } \)
= 12.5
Now, QR = QM + MR [Q – M – R]
= 8 + 12.5
∴ QR = 20.5 units
Question 4.
See adjoining figure. Find RP and PS using the information given in ∆PSR.
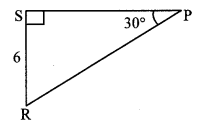
Solution:
In ∆PSR, ∠S = 90°, ∠P = 30° [Given]
∴ ∠R = 60° [Remaining angle of a triangle]
∴ ∆PSR is a 30° – 60° – 90° triangle.
RS = \(\frac { 1 }{ 2 } \) RP [Side opposite to 30°]
∴6 = \(\frac { 1 }{ 2 } \) RP
∴ RP = 6 × 2 = 12 units
Also, PS = \(\frac{\sqrt{3}}{2}\) RP [Side opposite to 60°]
= \(\frac{\sqrt{3}}{2}\) × 12
= \(6 \sqrt{3}\) units
∴ RP = 12 units, PS = 6 \(\sqrt { 3 }\) units
Question 5.
For finding AB and BC with the help of information given in the adjoining figure, complete the following activity.
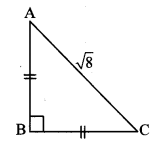
Solution:
AB = BC [Given]
∴ ∠BAC = ∠BCA [Isosceles triangle theorem]
Let ∠BAC = ∠BCA = x (i)
In ∆ABC, ∠A + ∠B + ∠C = 180° [Sum of the measures of the angles of a triangle is 180°]
∴ x + 90° + x = 180° [From (i)]
∴ 2x = 90°
∴ x = \(\frac { 90° }{ 2 } \) [From (i)]
∴ x = 45°
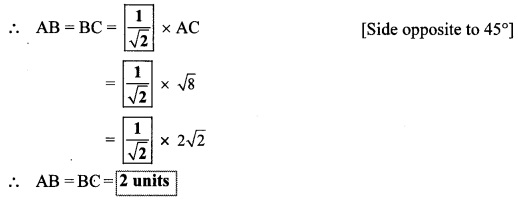
Question 6.
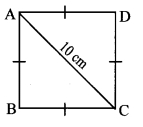
Find the side and perimeter of a square whose diagonal is 10 cm.
Solution:
Let ꠸ABCD be the given square.
l(diagonal AC) = 10 cm
Let the side of the square be ‘x’ cm.
In ∆ABC,
∠B = 90° [Angle of a square]
∴ AC2 = AB2 + BC2 [Pythagoras theorem]
∴ 102 = x2 + x2
∴ 100 = 2x2
∴ x2 = \(\frac { 100 }{ 2 } \)
∴x2 = 50
∴ x = \(\sqrt { 50 }\) [Taking square root of both sides]
= \(=\sqrt{25 \times 2}=5 \sqrt{2}\)
∴side of square is 5\(\sqrt { 2 }\) cm.
= 4 × 5 \(\sqrt { 2 }\)
∴ Perimeter of square = 20 \(\sqrt { 2 }\) cm
Question 7.
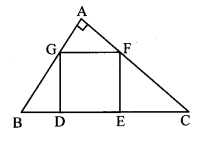
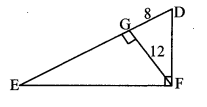
In the adjoining figure, ∠DFE = 90°, FG ⊥ ED. If GD = 8, FG = 12, find
i. EG
ii. FD, and
iii. EF
Solution:
i. In ∆DEF, ∠DFE = 90° and FG ⊥ ED [Given]
∴ FG2 = GD × EG [Theorem of geometric mean]
∴ 122 = 8 × EG .
∴ EG = \(\frac { 144 }{ 8 } \)
∴ EG = 18 units
ii. In ∆FGD, ∠FGD = 90° [Given]
∴ FD2 = FG2 + GD2 [Pythagoras theorem]
= 122 + 82 = 144 + 64
= 208
∴ FD = \(\sqrt { 208 }\) [Taking square root of both sides]
∴ FD = 4 \(\sqrt { 13 }\) units
iii. In ∆EGF, ∠EGF = 90° [Given]
∴ EF2 = EG2 + FG2 [Pythagoras theorem]
= 182 + 122 = 324 + 144
= 468
∴ EF = \(\sqrt { 468 }\) [Taking square root of both sides]
∴ EF = 6 \(\sqrt { 13 }\) units
Question 8.
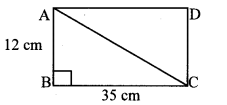
Find the diagonal of a rectangle whose length is 35 cm and breadth is 12 cm.
Solution:
Let ꠸ABCD be the given rectangle.
AB = 12 cm, BC 35 cm
In ∆ABC, ∠B = 90° [Angle of a rectangle]
∴ AC2 = AB2 + BC2 [Pythagoras theorem]
= 122 + 352
= 144 + 1225
= 1369
∴ AC = \(\sqrt { 1369 }\) [Taking square root of both sides]
= 37 cm
∴ The diagonal of the rectangle is 37 cm.
Question 9.
In the adjoining figure, M is the midpoint of QR. ∠PRQ = 90°.
Prove that, PQ2 = 4 PM2 – 3 PR2.

Solution:
Proof:
In ∆PQR, ∠PRQ = 90° [Given]
PQ2 = PR2 + QR2 (i) [Pythagoras theorem]
RM = \(\frac { 1 }{ 2 } \) QR [M is the midpoint of QR]
∴ 2RM = QR (ii)
∴ PQ2 = PR2 + (2RM)2 [From (i) and (ii)]
∴ PQ2 = PR2 + 4RM2 (iii)
Now, in ∆PRM, ∠PRM = 90° [Given]
∴ PM2 = PR2 + RM2 [Pythagoras theorem]
∴ RM2 = PM2 – PR2 (iv)
∴ PQ2 = PR2 + 4 (PM2 – PR2) [From (iii) and (iv)]
∴ PQ2 = PR2 + 4 PM2 – 4 PR2
∴ PQ2 = 4 PM2 – 3 PR2
Question 10.
Walls of two buildings on either side of a street are parallel to each other. A ladder 5.8 m long is placed on the street such that its top just reaches the window of a building at the height of 4 m. On turning the ladder over to the other side of the street, its top touches the window of the other building at a height 4.2 m. Find the width of the street.
Solution:
Let AC and CE represent the ladder of length 5.8 m, and A and E represent windows of the buildings on the opposite sides of the street. BD is the width of the street.
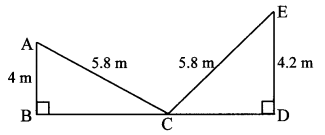
AB = 4 m and ED = 4.2 m
In ∆ABC, ∠B = 90° [Given]
AC2 = AB2 + BC2 [Pythagoras theorem]
∴ 5.82 = 42 + BC2
∴ 5.82 – 42 = BC2
∴ (5.8 – 4) (5.8 + 4) = BC2
∴ 1.8 × 9.8 = BC2

CE2 = CD2 + DE2 [Pythagoras theorem]
∴ 5.82 = CD2 + 4.22
∴ 5.82 – 4.22 = CD2
∴ (5.8 – 4.2) (5.8 + 4.2) = CD2
∴ 1.6 × 10 = CD2
∴ CD2 = 16
∴ CD = 4m (ii) [Taking square root of both sides]
Now, BD = BC + CD [B – C – D]
= 4.2 + 4 [From (i) and (ii)]
= 8.2 m
∴ The width of the street is 8.2 metres.
Question 1.
Verify that (3,4,5), (5,12,13), (8,15,17), (24,25,7) are Pythagorean triplets. (Textbook pg. no. 30)
Solution:
i. Here, 52 = 25
32 + 42 = 9 + 16 = 25
∴ 52 = 32 + 42
The square of the largest number is equal to the sum of the squares of the other two numbers.
∴ 3,4,5 is a Pythagorean triplet.
ii. Here, 132 = 169
52 + 122 = 25 + 144 = 169
∴ 132 = 52 + 122
The square of the largest number is equal to the sum of the squares of the other two numbers.
∴ 5,12,13 is a Pythagorean triplet.
iii. Here, 172 = 289
82 + 152 = 64 + 225 = 289
∴ 172 = 82 + 152
The square of the largest number is equal to the sum of the squares of the other two numbers.
∴ 8,15,17 is a Pythagorean triplet.
iv. Here, 252 = 625
72 + 242 = 49 + 576 = 625
∴ 252 = 72 + 242
The square of the largest number is equal to the sum of the squares of the other two numbers.
∴ 24,25, 7 is a Pythagorean triplet.
Question 2.
Assign different values to a and b and obtain 5 Pythagorean triplets. (Textbook pg. no. 31)
Solution:
i. Let a = 2, b = 1
a2 + b2 = 22 + 12 = 4 + 1 = 5
a2 – b2 = 22 – 12 = 4 – 1 = 3
2ab = 2 × 2 × 1 = 4
∴ (5, 3, 4) is a Pythagorean triplet.
ii. Let a = 4,b = 3
a2 + b2 = 42 + 32 = 16 + 9 = 25
a2 – b2 = 42 – 32 = 16 – 9 = 7
2ab = 2 × 4 × 3 = 24
∴ (25, 7, 24) is a Pythagorean triplet.
iii. Let a = 5, b = 2
a2 + b2 = 52 + 22 = 25 + 4 = 29
a2 – b2 = 52 – 22 = 25 – 4 = 21
2ab = 2 × 5 × 2 = 20
∴ (29, 21, 20) is a Pythagorean triplet.
iv. Let a = 4,b = 1
a2 + b2 = 42 + 12 = 16 + 1 = 17
a2 – b2 = 42 – 12 = 16 – 1 = 15
2ab = 2 × 4 × 1 = 8
∴ (17, 15, 8) is a Pythagorean triplet.
v. Let a = 9, b = 7
a2 + b2 = 92 + 72 = 81 + 49 = 130
a2 – b2 = 92 – 72 = 81 – 49 = 32
2ab = 2 × 9 × 7 = 126
∴ (130,32,126) is a Pythagorean triplet.
Note: Numbers in Pythagorean triplet can be written in any order.
Class 10 Maths Digest