Important Questions

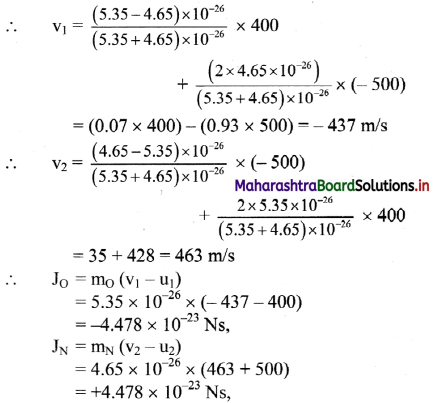
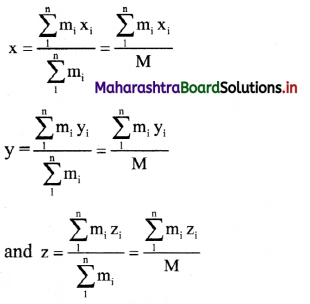
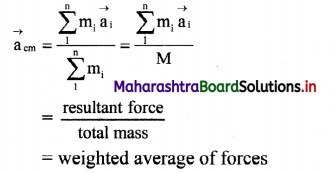
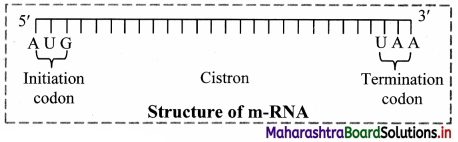
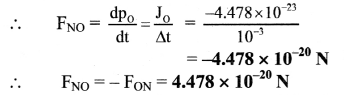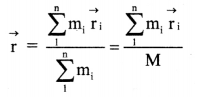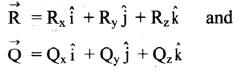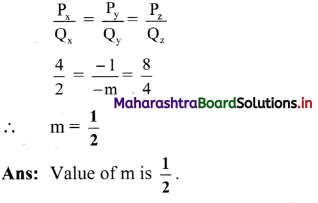Balbharti Maharashtra State Board 12th Chemistry Important Questions Chapter 5 Electrochemistry Important Questions and Answers.

Maharashtra State Board 12th Chemistry Important Questions Chapter 5 Electrochemistry

Question 1.
What is electrochemistry ?
Answer:
Electrochemistry : It is the branch of physical chemistry which involves the study of the inter-relation between chemical changes and electrical energy and also concerned with the electrical properties of electrolytic solutions such as resistance and conductance.

Question 2.
What is electric conduction?
Answer:
The transfer of electric charge or electrons from one point to another is called electric conduction which results in an electric current.

Question 3.
What are the electric conductors?
Answer:
The substances that allow the flow of electricity or electric charge transfer through them are called the electric conductors.

Question 4.
What is a flow of electricity or a transfer of electric charge?
Answer:
The flow of electricity or a transfer of electric charge through a conductor involves the transfer of electrons from one point to the other point. This takes place under the influence of applied electric potential.

Question 5.
What are the types of electric conductors? On what basis are they classified ?
Answer:
The electric conductors are classified according to the mechanism of the transfer of electrons or charge. There are two types of conductors as follows :

(i) Electrons (or metallic) conductors : The electric conductors through which the conduction of electricity takes place by a direct flow of electrons under the influence of applied potential are called electronic conductors.

In this case, there is no transfer of matter like atoms or ions. For example, solid and molten metals such as Al, Cu, etc.

(ii) Electrolytic conductors : The conductors in which the conduction of electricity takes place by the migration of positive ions (cations) and negative ions (anions) of the electrolyte are called electrolytic conductors. In this, the conduction involves the transfer of matter and it is accompanied with chemical changes. For example, solutions of electrolytes (strong and weak), molten salts.

Question 6.
Distinguish between electronic and electrolytic conductors.
Answer:
Electronic conductors:

1. The flow of electricity takes place by direct flow of electrons through the conductor.
2. The conduction does not involve the transfer of a matter.
3. No chemical change is involved during conduction.
4. The resistance of the conductor increases and conductivity decreases with the increase in temperature.
5. The conductance of metallic conductors is very high.
6. Examples are solid or molten metals, such as Al, Cu, etc.

Electrolytic conductors:

1. The electron transfer takes place by the migration of ions (cations and anions) of the electrolyte.
2. The conduction involves the transfer of a matter.
3. Chemical changes are always involved during the passage of an electric current.
4. The resistance decreases and the conductivity increases with the increase in temperature.
5. The conductance of the electrolytes is comparatively low.
6. Examples are aqueous solutions of acids, bases or salts.

Question 7.
What information is provided by measurement of conductivities of solutions?
Answer:

• The conducting and nonconducting properties of solutions can be identified by the measurement of their conductivities.
• The substances like sucrose and urea which do not dissociate in aqueous solutions have same conductivity as that of water. Hence they are nonelectrolytes.
• The substances like KCl, CH₃COOH, NaOH, etc. dissociate in their aqueous solutions and their conductivities are higher than water. Hence they are electrolytes.
• On the basis of high or low electrical conductivity, the electrolytes can be classified as strong and weak electrolytes. The solutions of strong electrolytes have high conductivities while solutions of weak electrolytes have lower conductivities.

Question 8.
What is Ohm’s law?
Answer:
Ohm’s law : According to Ohm’s law, the electrical resistance R of a conductor is equal to the electric potential difference, V divided by the electric current, I.
R = \(\frac{V}{I}\) ohm

Question 9.
What are SI units of
(a) electrical resistance
(b) potential and
(c) electric current?
Answer:
(a) The SI unit of electrical resistance is Ohm denoted by Ω (omega).
(b) The SI unit of potential is volt denoted by V.
(c) The SI unit of electric current is ampere denoted by A.

Question 10.
How is electrical conductance of a solution denoted ? What are its units ?
Answer:
The electrical conductance of a solution is denoted by G and it is the reciprocal of resistance, R.
G = \(\frac{1}{R}\)
The unit of G is siemens denoted by S or Ω^-1.
Hence we can write, S = Ω^-1 = AV^-1 = CV^-1S^-1 where A is ampere and C is coulomb.

Question 11.
What is electrical conductance? What are its units ?
Answer:
The reciprocal of the electrical resistance of a solution is called the conductance. It is represented by G.
∴ Conductance (G) = \(\frac{1}{\text { Resistance }}=\frac{1}{\mathrm{R}}\)
The conductance has units of reciprocal of ohm (Ω^-1, ohm^-1 or mho). In SI units, conductance has units as Siemens, (S). (1 S = 1 Ω^-1 = 1 ohm^-1 = 1 mho = AV^-1 = CV^-1 S, where C represents electric charge in coulomb, and A represents current strength)

Question 12.
What is specific conductance or conductivity?
Answer:
The reciprocal of specific resistance or resistivity is called specific conductance or conductivity.
If ρ is the resistivity then,
conductivity = \(\frac{1}{\text { resistivity }}=\frac{1}{\rho}\)
Conductivity is denoted by κ (kappa), where κ = \(\frac{1}{\rho}\)
It is the conductance of a conductor that is 1 m in length and 1 m² in cross section area in SI units. (In C.G.S. units, it is the resistance of a conductor that is 1 cm in length and 1 cm² in cross section area.) It is the conductance of a conductor of volume 1 m³ (or in C.G.S. units, the volume of 1 cm³).

Question 13.
What are the units of specific conductance or conductivity?
Answer:
If ρ is a resistivity and κ is conductivity or specific conductance, then

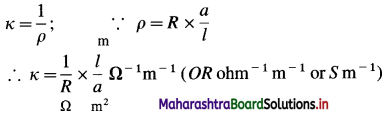
(where S is Siemens)
(In C.G.S. system, the units of κ are Ω^-1 cm^-1 or S cm^-1 which are commonly used.)

Question 14.
Define molar conductivity. What is the significance of it ?
Answer:
Molar conductivity: It is defined as a conductance of a volume of the solution containing ions from one mole of an electrolyte when placed between two parallel plate electrodes 1 cm apart and of large area, sufficient to accommodate the whole solution between them, at constant temperature. It is denoted by ∧_m.

Thus, the significance of molar conductivity is the conductance due to ions from one mole of an electrolyte.

Question 15.
Obtain a relation between conductivity (κ) and molar conductivity (∧_m).
Answer:
Conductivity or specific conductance (κ) is the conductance of 1 cm³ of the solution in C.G.S. units, while molar conductivity is the conductance of a solution containing one mole of an electrolyte. Consider C molar solution, i.e., C moles of an electrolyte present in 1 litre or 1000 cm³ of the solution.
∴ C moles of an electrolyte are present in 1000 cm³ solution.
∴ 1 mole of an electrolyte is present in \(\frac{1000}{\mathrm{C}}\) cm
solution.
Now,
∴ Conductance of 1 cm³ of this solution is κ,
∴ Conductance of \(\frac{1000}{\mathrm{C}}\) cm³ of the solution is \(\frac{\kappa \times 1000}{C}\)
This represents molar conductivity, ∧_m.
∴ ∧_m = \(\frac{\kappa \times 1000}{C}\) cm² mol^-1 (in C.G.S units)
[In case of SI units :
Consider a solution in which C moles of an electrolyte are present in 1 m³ of solution.
Conductivity κ is the conductance of 1 m³ of solution.
∵ C moles of an electrolyte are present in 1 m³ solution.
∴ 1 mol of an electrolyte is present in \(\frac{1}{C}\) solution.
∵ Conductance of 1 m³ of this solution is κ.
∴ Conductance of \(\frac{1}{C}\) m³ of the solution is \(\frac{\kappa}{\mathrm{C}}\)
This represents molar conductivity, ∧_m.
∴ ∧_m = \(\frac{\kappa}{\mathrm{C}}\)Ω^-1 m² mol^-1 (In SI units).]

Question 16.
What are the units of molar conductivity, ∧_m?
Answer:
In SI units: Conductivity κ is expressed in Ω^-1m^-1 (or S m^-1) and concentration of the solution is expressed in mol m^-3.

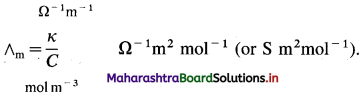
In C.G.S. units : Conductivity is expressed in Ω^-1 cm^-1 (or S cm^-1) and concentration of the solution is expressed in mol L^-1 or moles in 1000 cm³ of the solution.

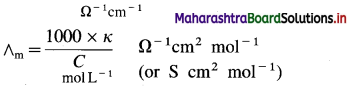
Question 17.
Explain the variation of molar conductivity with concentration for strong and weak electrolytes.
OR
How is the molar conductivity of strong electrolytes at zero concentration determined by graphical method? Why is this method not useful for weak electrolytes?
Answer:
(i) As the dilution of an electrolytic solution increases, the dissociation of the electrolyte increases, hence the total number of ions increases, therefore, the molar conductivity increases.

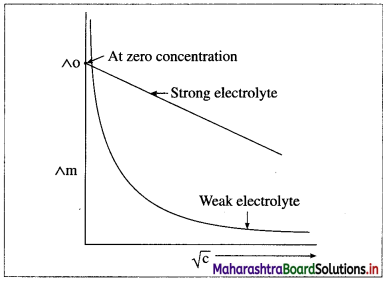
Fig. 5.5 : Variation of molar conductivity with \(\sqrt{\mathbf{c}}\)
(ii) The increase in molar conductivity with increase in dilution or decrease in concentration is different for strong and weak electrolytes.
(iii) On dilution, the molar conductivity of strong electrolytes increases rapidly and approaches to a maximum limiting value at infinite dilution or zero concentration and represented as ∧ ∞ or ∧₀ or ∧⁰_m. In case of weak electrolytes which dissociate less as compared to strong electrolytes, the molar conductivity is low and increases slowly in high concentration region, but increases rapidly at low concentration or high dilution. This is because the extent of dissociation increases with dilution rapidly.
(v) ∧₀ values for strong electrolytes can be obtained by extrapolating the linear graph to zero concentration (or infinite dilution). However ∧₀ for the weak electrolytes cannot be obtained by this method, since the graph increases exponentially at very high dilution and does not intersect ∧_m axis at zero concentration.

Question 18.
Why has the molar conductance of an electrolyte the maximum value at infinite dilution ?
Answer:

• As the dilution of an electrolytic solution increases or concentration decreases, the dissociation of an electrolyte increases.
• At infinite dilution, the dissociation of an electrolyte is complete (100% dissociation). Hence all the ions from one mole of an electrolyte are available to carry electricity.

Therefore the molar conductance at infinite dilute (∧₀) for a given electrolyte has the highest or limiting value. It is always constant for the given electrolyte at constant temperature.

Question 19.
State Kohlrausch’s law.
OR
State and explain Kohlrausch’s law of independent migration of ions.
Answer:
(A) Statement of Kohlrausch’s law : This states that at infinite dilution of the solution, each ion of an electrolyte migrates independently of its co-ions and contributes independently to the total molar conductivity of the electrolyte, irrespective of the nature of other ions present in the solution.

(B) Explanation : Both the ions, cation and anion of the electrolyte make a definite contribution to the molar conductivity of the electrolyte at infinite dilution or zero concentration (∧₀).
If \(\lambda_{+}^{0}\) and \(\lambda_{-}^{0}\) are the molar ionic conductivities of cation and anion respectively at infinite dilution, then
∧₀ = \(\lambda_{+}^{0}\) + \(\lambda_{-}^{0}\)
This is known as Kohlrausch’s law of independent migration of ions.
For an electrolyte, B_x A_y giving x number of cations and y number of anions,
∧₀ = x\(\lambda_{+}^{0}\) + y\(\lambda_{-}^{0}\)

(C) Applications of Kohlrausch’s law :
(1) With this law, the molar conductivity of a strong electrolyte at zero concentration can be determined. For example,
\(\wedge_{0(\mathrm{KCl})}=\lambda_{\mathrm{K}^{+}}^{0}-\lambda_{\mathrm{Cl}^{-}}^{0}\)
(2) ∧₀ values of weak electrolyte with those of strong electrolytes can be obtained. For example,

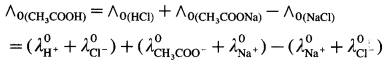
Question 20.
State Kohlrausch’s law and write mathematical expression of molar conductivity of the given solution at infinite dilution.
Answer:
Statement of Kohlrausch’s law : This states that at infinite dilution of the solution, each ion of an electrolyte migrates independently of its co-ions and contributes independently to the total molar conductivity of the electrolyte, irrespective of the nature of other ions present in the solution.

This law of independent migration of ions is represented as
∧₀ = \(\lambda_{+}^{0}\) + \(\lambda_{-}^{0}\).
where ∧₀ is the molar conductivity of the electrolyte at infinite dilution or zero concentration while \(\lambda_{+}^{0}\) and \(\lambda_{-}^{0}\) are the molar ionic conductivities of cation and anion respectively at infinite dilution.

Question 21.
Explain the determination of molar conductivity of a weak electrolyte at infinite dilution or zero concentration using Kohlrausch’s law.
Answer:
Molar conductivity of a weak electrolyte at infinite dilution or zero concentration cannot be measured experimentally.
Consider the molar conductivity (∧₀) of a weak acid, CH₃COOH at zero concentration. By Kohlrausch s law, ∧_0CH3COOH = λ⁰_CH3COOH^– + λ⁰ _H⁺ where λ⁰ _CH3COO^– and λ⁰ _H⁺ are the molar ionic conductivities of CH₃COO^– and H⁺ ions respectively.

If ∧_0CH3COONa, ∧_0HCl and ∧_0NaCl are the molar conductivities of CH₃COONa, HCl and NaCl respectively at zero concentration, then by
Kohlrausch’s law,

Hence, from ∧₀ values of strong electrolytes, ∧₀ of a weak electrolyte CH₃COOH, at infinite dilution can be calculated.

Question 22.
How is the degree of dissociation related to the molar conductance of the electrolytic solution ?
Answer:
(i) At zero concentration or at infinite dilution, the molar conductivity has a maximum value denoted by ∧₀.
(ii) This is due to complete dissociation of the weak electrolyte making all the ions available from one mole of the electrolyte to carry electricity at zero concentration.
(iii) If α is the degree of dissociation, then

This suggests that at zero concentration or infinite dilution, the electrolyte is completely (100%) dissociated.

Question 23.
Write the relation between molar conductivity and molar ionic conductivities for the following electrolytes :
(a) KBr, (b) Na₂SO₄, (c) AlCl₃.
Answer:
(a) If ∧₀ is molar conductivity of an electrolyte at infinite dilution and \(\lambda_{+}^{0}\) and \(\lambda_{-}^{0}\) are molar ionic conductivities then,

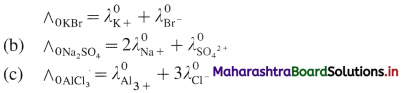
Question 24.
How is molar conductivity of an electrolytic solution measured ?
Answer:
The resistance of an electrolytic solution is measured by using a conductivity cell and Wheatstone bridge.

Fig. 5.6 : Measurement of conductance

The measurement of molar conductivity of a solution involves two steps as follows :
Step I : Determination of cell constant of the conductivity cell :
KCl solution (0.01 M) whose conductivity is accurately known (κ = 0.00141 Ω^-1 cm^-1) is taken in a beaker and the conductivity cell is dipped. The two electrodes of the cell are connected to one arm while the variable known resistance (R) is placed in another arm of Wheatstone bridge.

A current detector D’ which is a head phone or a magic eye is used. J is the sliding jockey (contact) that slides on the arm AB which is a wire of uniform cross section. A source of A.C. power (alternating power) is used to avoid electrolysis of the solution.

By sliding the jockey on wire AB, a balance point (null point) is obtained at C. Let AC and BC be the lengths of wire.
If R_solution is the resistance of KCl solution and R_x is the known resistance then by Wheatstone’s bridge principle,
\(\frac{R_{\text {solution }}}{\mathrm{BC}}=\frac{R_{x}}{\mathrm{AC}}\)
∴ R_solution = \(\mathrm{BC} \times \frac{R_{x}}{\mathrm{AC}}\)
Then the cell constant ‘b’ of the conductivity cell is obtained by, b = κ_KCl × R_solution.

Step II : Determination of conductivity of the given solution :
KCl solution is replaced by the given electrolytic solution and its resistance (R_s) is measured by Wheatstone bridge method by similar manner by obtaining a null point at D.
The conductivity (κ) of the given solution is, cell constant b
κ = \(\frac{\text { cell constant }}{R_{\mathrm{s}}}=\frac{b}{R_{\mathrm{s}}}\)

Step III: Calculation of molar conductivity :
The molar conductivity (∧_m) is given by,

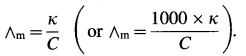
Since the concentration of the solution is known, ∧_m can be calculated.

Solved Examples 5.3

Question 25.
Solve the following :

(1) The resistance of a solution is 2.5 × 10³ ohm. Find the conductance of the solution.
Solution :
Given : Resistance of solution = R = 2.5 × 10³ Ω
Conductance of solution = G = ?
G = \(\frac{1}{R}\)
= \(\frac{1}{2.5 \times 10^{3}}\) ohm^-1 (Ω^-1 or S)
= 4 × 10^-3 Ω^-1 (or S)
Ans. Conductance = G = 4 × 10^-3 Ω^-1

(2) A conductivity cell has two electrodes 20 mm apart and of cross section area 1.8 cm². Find the cell constant.
Solution :
Given: Distance between two electrodes = l
= 20 mm
= 2 cm
Cross section area = a = 1.8 cm
Cell constant = b = ?
b = \(\frac{l}{a}=\frac{2}{1.8}\) = 1.111 cm^-1
Ans. Cell constant = 1.111 cm^-1

(3) The conductivity of 0.02 M AgNO₃ at 25 °C is 2.428 × 10^-3 Ω^-1 cm^-1. What is its molar conductivity ?
Solution :
Given : Concentration of solution = C = 0.02 M AgNO₃
Temperature = T = 273 + 25 = 298 K
Conductivity = κ = 2.428 × 10^-3 Ω^-1 cm^-1 (or S cm^-1)
Molar conductivity = ∧_m = ?
∧_m = \(\frac{\kappa \times 1000}{C}\)
= \(\frac{2.428 \times 10^{-3} \times 1000}{0.02}\)
= 121.4 Ω^-1 cm² mol^-1 (or 121.4 S cm² mol^-1)
Ans. Molar conductivity = ∧_m
= 121.4 Ω^-1 cm² mol^-1

(4) 0.05 M NaOH solution offered a resistance of 31.6 in a conductivity cell at 298 K. If the cell constant of the cell is 0.367 cm^-1, calculate the molar conductivity of NaOH solution.
Solution :
Given : Concentration = C = 0.05 M NaOH
Resistance = R = 31.6 Ω
Cell constant = b = 0.367 cm^-1

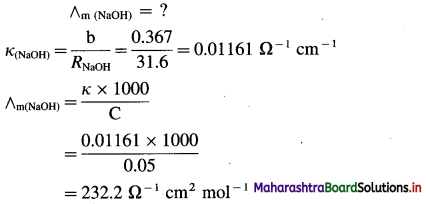
Ans. Molar conductivity = ∧_m = 232.2 Ω^-1 cm² mol^-1

(5) A conductivity cell filled with 0.1 M KCl gives at 25 °C a resistance of 85.5 ohms. The conductivity of 0.1 M KCl at 25° is 0.01286 ohm^-1 cm^-1. The same cell filled with 0.005 M HCl gives a resistance of 529 ohms. What is the molar conductivity of HCl solution at 25 °C ?
Solution :
Given : Resistance of KCl solution = R_KCl = 85.5 Ω
Conductivity of KCl solution = κ_KCl
= 0.01286 ohm^-1 cm^-1
Concentration = C = 0.005 M HCl
Resistance of HCl solution = R_soln = 529 ohms
Molar conductivity of HCl = ∧_m(HCl) = ?

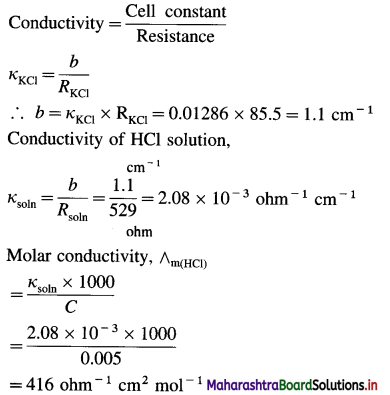
Ans. Molar conductivity of HCl solution = ∧_m(HCl)
= 416 ohm^-1 cm² mol^-1

(6) The molar conductivity of 0.05 M BaCl₂ solution at 25 °C is 223 Ω^-1 cm² mol^-1. What is its conductivity?
Solution :
Given : Molar conductivity = ∧_m
= 223 Ω^-1 cm² mol^-1
Concentration = C = 0.05 M BaCl₂
Conductivity = κ = ?

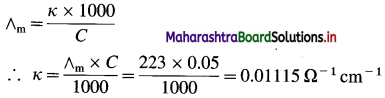
Ans. Conductivity = κ = 0.01115 Ω^-1 cm^-1

(7) Conductivity of a solution is 6.23 × 10^-5 Ω^-1 cm^-1 and its resistance is 13710 Ω. If the electrodes are 0.7 cm apart, calculate the cross-sectional area of electrode.
Solution :
Given : κ = 6.23 × 10^-5 Ω^-1 cm^-1
R = 13710 Ω
l = 0.7 cm
a = ?

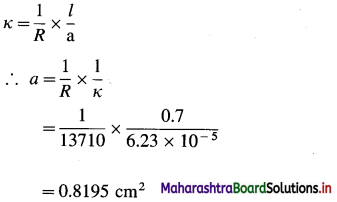
Ans. Cross sectional area of electrode = 0.8195 cm²

(8) A conductivity cell filled with 0.01 M KCl gives at 25 °C the resistance of 604 ohms. The conductivity of KCl at 25 °C is 0.00141 Ω^-1 cm^-1. The same cell filled with 0.001 M AgNO₃ gives a resistance of 6529 ohms. Calculate the molar conductivity of 0.001 M AgNO₃ solution at 25 °C.
Solution :
Given : Resistance of KCl solution = R_KCl
= 604 ohm (Ω)
Conductivity of KCl solution = κ_KCl
= 0.00141 Ω^-1 cm^-1
Concentration = C = 0.001 M AgNO₃
Resistance of solution = R_sol = 6529 ohm (Ω)
Molar conductivity = ∧_m = ?

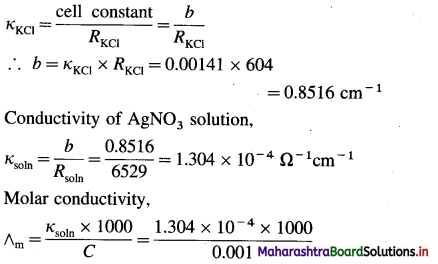
cell constant b
= 130.4 Ω^-1 cm² mol^-1
Ans. Molar conductivity of AgNO₃ solution = ∧_m
= 130.4 Ω^-1 cm² mol^-1

(9) Resistance and conductivity of a cell containing 0.001 M KCl solution at 298 K are 1500 Ω and 1.46 × 10^-4 S.cm^-1 respectively. What is cell constant.
Solution :
Given : Resistance of KCl solution = 1500 Ω, conductivity of KCl solution = κ = 1.46 × 10^-4 S.cm^-1, Cell constant = b = ?
Cell constant = Conductivity (k) × Resistance
= 1.46 × 10^-4 × 1500
= 0.219 cm^-1
Ans. Cell constant = 0.219 cm^-1

(10) A conductivity cell filled with 0.02 M H₂SO₄ gives at 25 °C resistance of 122 ohms. If the molar conductivity of 0.02 H₂SO₄ is 618 Ω^-1 cm² mol^-1, what is the cell constant?
Solution :
Given : Concentration = C = 0.02 M H₂SO₄
Resistance of H₂SO₄ solution = R_soln = 122 Ω
Molar conductivity = ∧_m = 618 Ω^-1 cm² mol^-1
Cell constant = b = ?

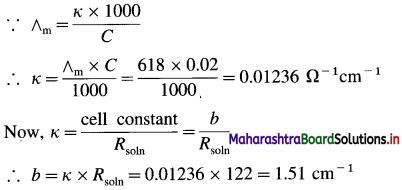
Ans. Cell constant = b = 1.51 cm^-1

(11) A conductivity cell filled with 0.02 M AgNO₃ gives at 25 °C resistance of 947 ohms. If the cell constant is 2.3 cm^-1, what is the molar conductivity of 0.02 M AgNO₃ at 25 °C?
Solution :
Given : Concentration = C = 0.02 M AgNO₃
Resistance of solution = R_soln = 947 Ω
Cell constant = b = 2.3 cm^-1
Molar conductivity = ∧_m = ?
Conductivity of soln = κ

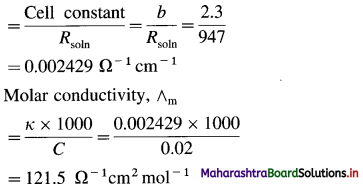
Ans. Molar conductivity = ∧_m
= 121.5 Ω^-1 m² mol^-1

(12) Resistance of conductivity cell filled with 0.1 M KCl solution is 100 ohms. If the resistance of the same cell when filled with 0.02 M KCl solution is 520 ohms, calculate the conductivity and molar conductivity of 0.02 M KCl solution. [Given : Conductivity of 0.1 M KCl solution is 1.29 Sm^-1.]
Solution:
Given : Resistance of 0.1 M KCl solution = R₁ = 100 Ω
Resistance of 0.02 M KCl solution = R₂ = 520 Ω
Conductivity of 0.02 M KCl solution = κ₂ = ?
Molar conductivity of 0.02 M KCl solution = ∧_m = ?
Conductivity of 0.1 M KCl solution = κ₁
= 1.29 S m^-1
Cell constant = b = κ₁ × R₁ = 1.29 × 100
= 129 m^-1
= 1.29 cm^-1

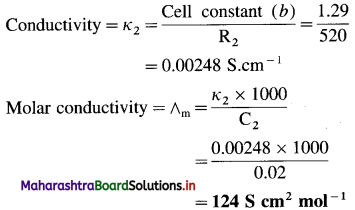
(13) The molar conductivities at zero concentration (or at infinite dilution) of CH₃COONa, HCl and NaCl in Ω^-1 cm² mol^-1 are 90.8,426.2 and 126.4 respectively. Calculate the molar conductivity of CH₃COOH at infinite dilution.
Solution :

(14) The molar conductivities at zero concentrations of NH₄Cl, NaOH and NaCl are respectively 149.7Ω^-1 cm² mol^-1, 248.1 Ω^-1 cm² mol^-1 and 126.5 Ω^-1 cm² mol^-1. What is the molar conductivity of NH₄OH at zero concentration ?
Solution :

(15) What is the molar conductivity of AgI at zero concentration if the ∧₀ values of NaI, AgNO₃ and NaNO₃ are respectively 126.9 Ω^-1 cm² mol^-1, 133.4 Ω^-1 cm² mol^-1 and 121.5 Ω^-1 cm² mol^-1 ?
Solution :

Adding equations (i) and (ii) and subtracting equation (iii) we get equation I.

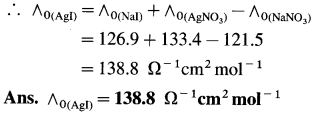
(16) Molar conductivity of KCl at infinite dilution is 150.3 S cm² mol^-1. If the molar conductivity of K⁺ is 73.4, calculate that of Cl^–.
Solution :
Given : Molar conductivity at infinite dilution
= ∧_(KCl) = 150.3 S cm² mol^-1
Molar conductivity of K⁺
= \(\lambda_{\mathrm{K}^{+}}^{0}\) = 73.4 S cm² mol^-1
Molar conductivity of Cl^– = \(\lambda_{\mathrm{Cl}^{-}}^{0}\) = ?
By Kohlrausch’s law,

(17) Molar conductivities at infinite dilution of Mg²⁺ and Br^– are 105.8 Ω^-1 cm² mol^-1 and 78.2 Ω^-1 cm² mol^-1 respectively. Calculate molar conductivity at zero concentration of MgBr₂.
Solution :
Given : \(\lambda_{\mathrm{Mg}^{2+}}^{0}\) = 105.8 Ω^-1 cm² mol^-1
\(\lambda_{\mathrm{Br}^{-}}^{0}\) = 78.2 Ω^-1 cm² mol^-1
\(\wedge_{0\left(\mathrm{MgBr}_{2}\right)}\) = ?
By Kohlrausch’s law,
\(\wedge_{0\left(\mathrm{MgBr}_{2}\right)}\) = \(\lambda_{\mathrm{Mg}^{2+}}^{0}\) + 2\(\lambda_{\mathrm{Br}^{-}}^{0}\)
= 105.8 + 2 × 78.2 = 105.8 + 156.4
= 262.2 Ω^-1 cm² mol^-1
Ans. Molar conductivity of MgBr₂ at zero concentration = \(\wedge_{0\left(\mathrm{MgBr}_{2}\right)}\) = 262.2 Ω^-1 cm² mol^-1

(18) The molar conductivity of 0.1 M CH₃COOH at 25 °C is 15.9 Ω^-1 cm² mol^-1. If the molar conductivities of CH3COO^– and H⁺ ions in Ω^-1 cm² mol^-1 at zero concentration are 40.8 and 349.6 respectively, calculate degree of dissociation of 0.1 M CH₃COOH.
Solution :
Given : Concentration = C = 0.1 M CH₃COOH
Molar conductivity = ∧_m = 15.9 Ω^-1 cm² mol^-1
\(\lambda_{\mathrm{CH}_{3} \mathrm{COO}^{-}}^{0}\) = 40.8 Ω-1 cm² mol^-1;
\(\lambda_{\mathrm{H}^{+}}^{0}\) = 349.6 Ω^-1 cm² mol^-1
Degree of dissociation = α = ?
By Kohlrausch’s law,
\(\wedge_{0\left(\mathrm{CH}_{3} \mathrm{COOH}\right)}=\lambda_{\mathrm{CH}_{3} \mathrm{COO}^{-}}^{0}+\lambda_{\mathrm{H}^{+}}^{0}\)
= 40.8 + 349.6
= 390.4 Ω^-1 cm² mol^-1
α = ∧_m/∧0
= \(\frac{15.9}{390.4}\) = 0.0407
Ans. The degree of dissociation of CH₃COOH = 0.0407

(19) The dissociation constant of a weak monoacidic base is 1.2 × 10^-5 at 25 °C. The molar conductivity of the base at zero concentration is 354.8 Ω^-1 cm² mol^-1 at 25°C. Calculate the percentage dissociation and molar conductivity of the weak base at 0.1 M concentration.
Solution :
Given : Dissociation constant of the base = K_b = 1.2 × 10^-5
Concentration = C = 0.1 M
∧₀ = 354.8 Ω^-1 cm² mol^-1
Percentage dissociation = ?
∧_m = ?
K_a = \(\frac{\mathrm{C} \alpha^{2}}{1-\alpha}\); For a week electrolyte, α is small,
∴ K_a = cα²;
∴ α = \(\sqrt{\frac{\mathrm{K}_{\mathrm{a}}}{\mathrm{C}}}=\left(\frac{1.2 \times 10^{-5}}{0.1}\right)^{\frac{1}{2}}\) = 1.0954 × 10^-2
∴ Percentage dissociation = α × 100
= 1.0954 × 10-2 × 100 = 1.0954%
Now, α = \(\frac{\wedge_{\mathrm{m}}}{\wedge_{0}}\)
∴ ∧_m = α × ∧₀ = 1.954 × 10^-2 × 354.8
= 6.932 Ω^-1 cm² mol^-1
Ans. Percentage dissociation = 1.0954
Molar conductivity = ∧_m
= 6.932 Ω^-1 cm² mol^-1

Question 26.
What is an electrochemical cell? What does it consist of?
Answer:
Electrochemical cell : It consists of two electronic conductors such as metal plates dipping into an electrolytic or ionic conductor which is an aqueous electrolytic solution or a pure liquid of a molten electrolyte.

Question 27.
What are electrochemical reactions ?
Answer:

1. Electrochemical reactions : The chemical reactions occurring in electrochemical cells which involve transfer of electrons from one species to other are called electrochemical reactions. They are redox reactions.
2. These reactions are made of two half reactions namely oxidation at one electrode (anode) and reduction at another electrode (cathode) of the electrochemical cell.
3. The net reaction is the sum of the above two half reactions.

Question 28.
Define electrode.
Answer:
Electrode : The arrangement consisting of a metal rod dipping in an aqueous solution or molten electrolyte containing ions and conduct electric current due to oxidation or reduction half reactions occurring on its surface is called an electrode.

The electrodes which take part in the reactions are called active electrodes while those which do not take part in the reactions are called inert electrodes.

Question 29.
Define : (a) Anode (b) Cathode.
Answer:
(a) Anode : An electrode of an electrochemical cell, at which oxidation half reaction occurs due to the loss of electrons from some species is called an anode.
(b) Cathode : An electrode of an electrochemical cell at which reduction half reaction occurs due to gain of electrons by some species is called a cathode.

Question 30.
What are the types of electrochemical cells ?
Answer:
There are two types of electrochemical cells as follows :

1. Electrolytic cells
2. Voltaic or galvanic cells.

Question 31.
Define : (1) Electrolytic cell (2) Voltaic or galvanic cell.
Answer:
(1) Electrolytic cell : An electrochemical cell in which a non-spontaneous chemical reaction is forced to occur by passing direct electric current into the solution from the external source and where electrical energy is converted into chemical energy is called an electrolytic cell. E.g. voltameter, electrolytic cell for deposition of a metal.

(2) Voltaic or galvanic cell : An electrochemical cell in which a spontaneous chemical reaction occurs producing electricity and where a chemical energy is converted into an electrical energy is called voltaic cell or galvanic cell. E.g. Daniell cell, dry cell, lead storage battery, fuel cells, etc.

Question 32.
Define electrolysis.
Answer:
Electrolysis : The process of a non-spontaneous chemical decomposition of an electrolyte by the passage of an electric current through its aqueous solution or fused mass and in which electrical energy is converted into chemical energy is called electrolysis. E.g. Electrolysis of fused NaCl.

Question 33.
Describe electrolysis of aqueous NaCl.
Answer:
(1) Construction of an electrolytic cell : It consists of a vessel containing aqueous solution of NaCl. Two inert electrodes (graphite electrodes) are dipped in it and connected to an external source of electricity like battery. The electrode connected to the negative terminal is a cathode and that connected to a positive terminal is an anode.

(2) Working of the cell :
(A) NaCl_(aq) and H₂O_(l) dissociate as follows :

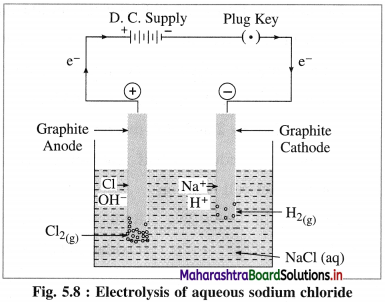
(3) Reactions in electrolytic cell :
(i) Reduction half reaction at cathode : There are Na⁺ and H⁺ ions but since H⁺ are more reducible than Na⁺, they undergo reduction liberating hydrogen and Na⁺ are left in the solution.
2H₂O_(l) + 2e^– → H_2(g) + \(2 \mathrm{OH}_{(\mathrm{aq})}^{-}\) (reduction)
E⁰ = -0.83 V
(ii) Oxidation half reaction at anode : At anode there are Cl^– and OH^–. But Cl^– ions are preferably oxidised due to less decomposition potential.

Net cell reaction : Since two electrons are gained at cathode and two electrons are released at anode for each redox step, the electrical neutrality is maintained. Hence we can write,

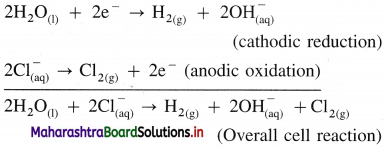
Since Na⁺ and OH^– are left in the solution, they form NaOH_(aq).

(4) Results of electrolysis :

• H₂ gas is liberated at cathode.
• Cl₂ gas is liberated at anode.
• NaOH is formed in the solution and it reacts basic.

Question 34.
Define and explain the following electrical units : (1) Coulomb (2) Ampere (3) Volt (4) Joule (5) Ohm.
Answer:
(1) Coulomb : It is a quantity of electricity obtained when one ampere current flows for one second.
It is the unit of quantity of electricity.
Q = I × t Coulomb (C)
where Q is the charge or quantity of electricity in coulombs.

(2) Ampere : It is a strength of an electric current obtained when one coulomb of electricity is passed through a circuit for one second.
∴ I = Q/t

(3) Volt : It is the potential difference between two points of an electric conductor required to send a current of one amphere through a resistance of one ohm.
∴ V = I × R
where V is the potential difference in volts and R is the resistance of a conductor in ohms.

(4) Joule : It is the electrical work or energy produced when one coulomb of electricity is passed through a
potential difference of one volt.
∴ Electrical work = Q × V J
where Q is electrical charge in coulombs and V is the potential difference.

(5) Ohm : It is the resistance of an electrical conductor across which when potential difference of 1 volt is applied, a current of one ampere is obtained. It has units, Ω or per siemens.

Question 35.
Explain quantitative aspects of electrolysis.
Answer:
(1) Calculation of quantity of electricity : If an electric current of strength I A is passed through the cell for t seconds, then quantity of electricity (Q) obtained is given by,
Q = I × t C (Coulomb)

(2) Calculation of moles of electrons passed : The charge carried by one mole of electrons is referred to as one faraday (F). If total charge passed is Q C, then moles of electrons passed = \(\frac{Q(\mathrm{C})}{F\left(\mathrm{C} / \mathrm{mol} \mathrm{e}^{-}\right)}\)

(3) Calculation of moles of product formed : Consider one mole of ions, \(\mathbf{M}_{(\mathrm{aq})}^{n^{+}}\) which will require n moles of electrons for reduction.
\(\mathbf{M}_{(\mathrm{aq})}^{n^{+}}\) + ne^– → M (Reduction half reaction)

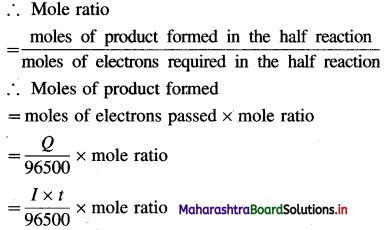
(4) Calculation of mass of product : Mass, W of product formed is given by,
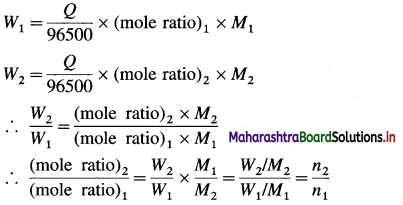
W = moles of product × molar mass of product (M)

When two electrolytic cells containing different electrolytes are connected in series so that same quantity of electricity is passed through them, then the masses W₁ and W₂ of products produced are given by,

Question 36.
Define Faraday.
Answer:
Faraday : It is defined as the quantity of the electric charge carried by one mole of electrons.
It has value, 1F = 96500 C/mol

Question 37.
Obtain a charge on one electron from Faraday’s value.
Answer:

• One Faraday is the electric charge on one mole of electrons (6.022 × 10²³ electrons).
• 1 Faraday = 96500 (per mol of electrons).
• Hence the charge on one electron is, change on one electron = \(\frac{96500}{6.022 \times 10^{23}}\)
  = 1.602 × 10^-9 C.

Solved Examples 5.4-5.5

Question 38.
Solve the following :

(1) An electric current of 100 mA is passed through an electrolyte for 2 hours, 20 minutes and 20 seconds. Find the quantity of electricity passed.
Solution :
Given : Electric current = I = 100 mA
= 100 × 10^-3 A
= 0.1 A
Time = t = 2 hrs + 20 min + 20 s
= 2 × 60 × 60 + 20 × 60 + 20
= 8420 s
The quantity of electricity = Q = ?
Q = I × t
= 0.1 × 8420
= 842 C
Ans. Quantity of electricity passed, Q = 842 C

(2) An electric current of 500 mA is passed for 1 hour and 30 minutes. Calculate the
(i) Quantity of electricity (or charge)
(ii) Number of Faradays of electricity
(iii) Number of electrons passed (Charge on 1 electron = 1.602 × 10^-19 C)
Solution :
Given : Electric current = I = 500 mA
= 500 × 10^-3 A = 0.5 A
Time = t = 1 hr + 30 min
= 1 × 60 × 60 + 30 × 60
= 5400 s
(i) The quantity of electricity = Q = ?
(ii) Number of Faradays of electricity = ?
(iii) Number of electrons passed = ?

(i) Q = I × t = 0.5(A) × 5400(s) = 2700 C

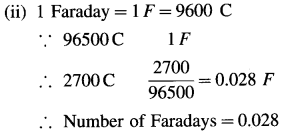
(iii) 1F is the electric charge on 6.022 × 10²³ electrons.
∴ 0.028F is the charge on,
0.028 × 6.022 × 10²³ = 1.686 × 10²² electrons
∴ Number of electrons passed = 1.686 × 10²²
Ans. (i) The quantity of electricity = Q = 2700 C
(ii) Number of Faradays of electricity = 0.028 F
(iii) Number of electrons passed = 1.686 × 10²²

(3) How much electricity in terms of Faraday is required to produce :
(a) 20 g of Ca from molten CaCl₂
(b) 40 g of Al from molten Al₂O₃
(Given : Molar mass of Calcium and Aluminium are 40 g mol^-1 and 27 g mol^-1 respectively.)
Solution :

(4) For the following conversions,
calculate
(i) number of moles of electrons
(ii) number of Faradays
(iii) Amount of electricity :
(A) 0.1 mol conversion of Zn²⁺ to Zn.
(B) 0.08 mol conversion of \(\mathbf{M n O}_{4}^{2-}\) to Mn²⁺
(C) 1.1 mol conversion of \(\mathrm{Cr}_{2} \mathbf{O}_{7}^{2-}\) to Cr³⁺.
Solution :
(i) Number of moles of electrons = ?
(ii) Number of Faradays = ?
(iii) Amount of electricity = Q = ?
(A) Number of moles of Zn²⁺ =0.1 mol
Zn²⁺ + 2e^– → Zn
(i) ∵ 1 mol Zn²⁺ requires 2 mol electrons
0.1 mol Zn²⁺ will require
∴ 0.1 × 2 = 0.2 mol electrons
(ii) ∵ 1 mol electrons = 1 Faraday
∴ 0.2 mol electrons = 0.2 × 1
= 0.2 Faradays
(iii) ∵ 1 Faraday = 96500 C
∴ 0.2 Faraday = 96500 × 0.2 = 48250 C
Amount of electricity required =48250C

(B) Number of moles of \(\mathrm{MnO}_{4}^{-}\) = 0.08 mol
\(\mathrm{MnO}_{4}^{-}+5 \mathrm{e}^{-} \longrightarrow \mathrm{Mn}^{2+}\)
(i) ∵ 1 mol \(\mathrm{MnO}_{4}^{-}\) requires 5 mol electrons
∴ 0.08 mol \(\mathrm{MnO}_{4}^{-}\) will require
5 × 0.08 = 0.4 mol electrons
(ii) Number of Faradays = 0.4 × 1 = 0.4
(iii) Amount of electricity = Q = 0.4 × 96500
= 38600 C

(C) Number of moles of \(\mathrm{Cr}_{2} \mathrm{O}_{7}^{2-}\) = 1.1 mol

(i) ∵ 1 mol \(\mathrm{Cr}_{2} \mathrm{O}_{7}^{2-}\) requires 6 mol electrons
∴ 1.1 mol \(\mathrm{Cr}_{2} \mathrm{O}_{7}^{2-}\) will require
6 × 1.1 = 6.6 mol electrons
(ii) Number of Faradays = 1 × 6.6 = 6.6
(iii) Amount of electricity = 6.6 × 96500
= 6.369 × 10⁵ C
Ans.
(A) (i) Number of moles of electrons = 0.2 mol
(ii) Number of Faradays = 0.2
(iii) Amount of electricity = 48250 C

(B) (i) Number of moles of electrons = 0.4 mol
(ii) Number of Faradays = 0.4
(iii) Amount of electricity = 38600 C

(C) (i) Number of moles of electrons = 6.6 mol
(ii) Number of Faradays = 6.6
(iii) Amount of electricity = 6.369 × 10⁵ C

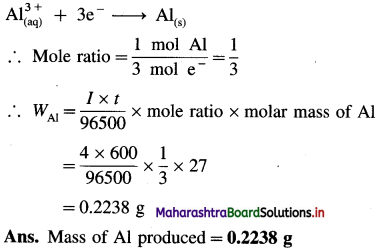
(5) What mass of aluminium is produced at the cathode during the passage of 4 ampere current through Al₂(SO₄)₃ solution for 100 minutes? Molar mass of aluminium is 27 g mol^-1.
Solution :
Given : I = 4 A; t = 100 × 60 = 600 s
F = 96500 C mol^-1, M = 27 g mol^-1, W_Al = ?

(6) How long will it take to produce 2.415 g Ag metal from its salt solution by passing a current of 3 amperes? How many moles of electrons are required ? Molar mass of Ag is 107.9 gmol^-1.
Solution :
Given : Electric current = I = 3A
Mass of Ag produced = 2.415 g
Molar mass of Ag = Atomic mass of Ag
= 107.9 gmol^-1
Time = t = ? Number of moles of electrons = ?
Reduction half reaction at cathode :

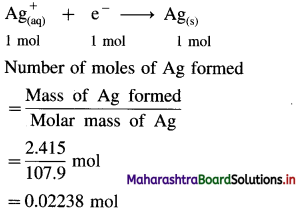
From the reaction,
∵ 1 mole of Ag requires 1 mole of electrons
∴ 0.02238 mole of Ag will require,
0.02238 mol electrons
∵ 1 mole of electrons carries a charge of 96500 C,
∴ 0.02238 mole of electrons will carry a charge, 0.02238 × 96500 = 2160 C
∴ Quantity of electricity passed = Q = 2160 C
Let I be the current strength and t be time of electrolysis. Then,
∵ Q = I × t
∴ t = \(\frac{Q}{I}=\frac{2160}{3}\) = 720 s = \(\frac{720}{60}\) min = 12 min.
Ans. Time of electrolysis = 12 min
Moles of electrons = 0.02238 mol

(7) What current strength in ampere will be required to produce 2.369 × 10^-3 kg of Cu from CuSO4 solution in one hour? How many moles of electrons are required? Molar mass of copper is 63.5 gmol^-1.
Solution :
Given : Mass of Cu produced = 2.369 × 10^-3 kg
= 2.369 g
Time = t = 1 hr = 1 × 60 × 60 = 3600 s
Molar mass of Cu = 63.5 g mol^-1
Strength of current = I = ?
1 Faraday = 96500 C = 1 mol electrons
1 mol Cu = Molar mass of Cu = 63.5 g
Reduction half reaction :

Moles of Cu deposited = \(\frac{2.369}{63.5}\) = 0.0373 mol Cu
From the reaction,
∵ 1 mol of Cu requires 2 mol electrons
∴ 0.0373 mol Cu will require 2 × 0.0373
= 0.0746 mol electrons
Now,
∵ 1 mol electrons = 96500 C
∴ 0.0746 mol electrons = 96500 × 0.0746 = 7199 C
∴ Quantity of electricity required = Q = 7199 C
∴ Q = I × t
∴ Current, I = \(\frac{Q}{t}=\frac{7199}{3600}\) = 2A
Ans. Current strength = I = 2A
Moles of electrons required = 0.0746 mol

(8) A current of 6 amperes is passed through AlCl₃ solution for 15 minutes using Pt electrodes, when 0.504 g Al is produced. What is the molar mass of Al ?
Solution :
Given : Electric current = I = 6 A
Time = t = 15 min = 15 × 60 s = 900 s
Mass of Al produced = 0.504 g
Molar mass of Al = ?
Reduction half reaction,
\(\mathrm{Al}_{(\mathrm{aq})}^{3+}+3 \mathrm{e}^{-} \longrightarrow \mathrm{Al}_{(\mathrm{aq})}\)
Quantity of electricity passed = Q = I × t
= 6 × 900 = 5400 C
Number of moles of electrons = \(\frac{Q}{F}=\frac{5400}{96500}\)
= 0.05596 mol
From half reaction,
∵ 3 moles of electrons deposit 1 mole Al
∴ 0.05596 moles of electrons will deposit,
\(\frac{0.05596}{3}\) = 0.01865 mol Al
Now,
∵ 0.01865 mole Al weighs 0.504 g
∴ 1 mole Al will weigh, \(\frac{0.504}{0.01865}\) = 27 g
Hence molar mass of Al is 27 g mol^-1
Ans. Molar mass of Al = 27 g mo^-1

(9) How many moles of electrons are required for the reduction of (i) 3 moles of Zn²⁺ to Zn,
(ii) 1 mol of Cr³⁺ to Cr ?
How many Faradays of electricity will be required in each case ?
Solution :
(i) Given : For reduction of 3 mol Zn²⁺ to Zn;
Number of moles of electrons required = ?
Reduction half reaction,
Zn²⁺ + 2e^– → Zn
∵ 1 mole of Zn²⁺ requires 2 moles of electrons
∴ 3 moles of Zn²⁺ will require,
∵ 3 × 2 = 6 moles of electrons
∴ 1 mole of electrons = 1 F 6 moles of electrons = 6 F

(ii) Given : Reduction of 1 mol of Cr³⁺ to Cr :
Reduction half reaction,
Cr³⁺ + 3e^– → Cr
Hence 1 mole of Cr³⁺ will require 3 moles of electrons
∵ 1 mole of electrons = 1
∴ 3 moles of electrons = 3 F
Ans. (i) 6 mol electrons and 6 Faradays.
(ii) 3 mol electrons and 3 Faradays.

(10) In an electrolysis of AgNO₃ solution, 0.7 g of Ag is deposited after a certain period of time. Calculate the quantity of electricity required in coulomb. (Molar mass of Ag is 107.9 g mol^-1.)
Solution :
Given : Mass of Ag deposited = 0.7 g
Molar mass of Ag = 107.9 g mol^-1
Quantity of electricity = Q = ?
Reduction half reaction is,
Ag⁺ + e^– → Ag
1 mole of Ag = 107.9 g Ag requires 1 mole of electrons
∴ 0.7 g Ag will require, \(\frac{0.7}{107.9}\) = 6.49 × 10^-3 mole of electrons
∵ 1 mole of electrons carry 96500 C charge
∴ 6.49 × 10^-3 mole of electrons will carry, 96500 × 6.49 × 10^-3 = 626 C
Ans. Quantity of electricity required = 626 C,

(11) Calculate the amounts of Na and Chlorine gas produced during the electrolysis of fused NaCl by the passage of 1 ampere current for 25 minutes. Molar masses of Na and Chlorine gas are 23 g mol^-1 and 71 g mol^-1 respectively.
Solution :
Given : Electric current = I = 1 ampere
Time = t = 25 minutes = 25 × 60 s = 1500 s
Molar mass of Na = 23 g mol^-1
Molar mass of Cl₂ = 71 g mol^-1
Mass of Na produced = ?, Mass of Cl₂ produced = ?
Reactions during electrolysis :

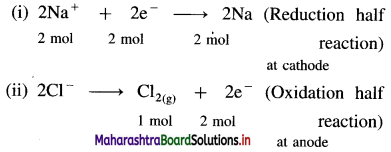
Quantity of electricity = Q = I × t = 1 × 1500
= 1500 C
Number of moles of electrons passed
= \(\frac{Q}{F}=\frac{1500}{96500}\) = 0.01554
From half reaction (i),
∵ 2 moles of electrons deposit 2 moles of Na
∴ 0.01554 moles of electrons will deposit, \(\frac{0.01554 \times 2}{2}\) = 0.01554 mol Na
Mass of Na = Moles of Na × Molar mass of Na
= 0.01554 × 23 = 0.3572 g Na
From half reaction (ii)
∵ 2 moles of electrons produce 1 mole Cl₂
∴ 0.01554 moles of electrons will produce,
\(\frac{0.01554 \times 1}{2}\) = 7.77 × 10^-3 × 71
∴ Mass of Cl₂ gas = Moles of Cl₂ × Molar mass
= 7.77 × 10^-3 × 71
= 0.5518 g
Ans. Mass of Na deposited = 0.3572 g
Mass of Cl2 liberated = 0.5518 g

(12) Calculate the mass of Mg and the volume of Chlorine gas at NTP produced during the electrolysis of molten MgCl₂ by the passage of 2 amperes of current for 1 hour. Molar masses of Mg and Cl2 are 24 g mol-1 and 71 g mol-1 respectively.
Solution :
Given : Electric current = I = 2A
Time = t = 1 hr = 1 × 60 × 60 s = 3600 s
Molar mass of Mg = 23 g mol-1
Molar mass of Cl₂ = 71 g mol-1
Mass of Mg produced = ?
Volume of Cl₂ at NTP produced = ?
Reactions during electrolysis :
(i) Mg²⁺ + 2e^– → Mg (Reduction half reaction)
(ii) 2Cl^– → Cl_2(g) + 2e^– (Oxidation half reaction)
Quantity of electricity passed = Q = I × t
= 2 × 3600 = 7200 C
∵ 1 Faraday = 1 mol electrons
∴ Number of moles of electrons passed
= \(\frac{Q}{F}=\frac{7200}{96500}\) = 0.07461 mol
From half reaction (i),
∵ 2 moles of electrons deposit 1 mole of Mg
∴ 0.07461 moles of electrons will deposit, \(\frac{0.07461 \times 1}{2}\) = 0.037305 mol Mg
Mass of Mg = Moles of Mg × Molar mass of Mg
= 0.037305 × 24 = 0.8953 g Mg
From half reaction (ii),
∵ 2 moles of electrons produce 1 mol Cl₂ gas
∴ 0.07461 moles of electrons will produce,
\(\frac{0.07461}{2}\) = 0.037305 mol Cl₂
∵ 1 mole of Cl₂ occupies 22.4 dm at NTP
∴ 0.037305 mole of Cl₂ will occupy,
22.4 × 0.037305 = 0.8356 dm3
∴ Volume of Cl₂ gas produced
= 0.8356 dm³
= 0.8356 × 10³ cm³
= 835.6 cm³
Ans. Mass of Mg produced = 0.8953 g
Volume of Cl_2(g) at NTP produced = 835.6 cm³

(13) How many Faradays would be required to plate out one mole of free metal from the following cations?
(a) Mg²⁺ (b) Cr³⁺ (c) Pb²⁺ (d) Cu⁺
Solution :
(a) Reduction half reaction :

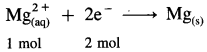
∵ 1 mol electrons = 1 Faraday
Since to deposite 1 mol Mg, two moles of electrons are required,
∴ To plate one mole Mg, 2 Faradays of electricity will be required.

(b) Reduction half reaction :
\(\mathrm{Cr}_{(\mathrm{aq})}^{3+}+3 \mathrm{e}^{-} \longrightarrow \mathrm{Cr}_{(\mathrm{s})}\)
∴ 1 mol Cr will require 3 mol electrons, hence 3 Faradays of electricity are required.

(c) Reduction half reaction :
\(\mathrm{Pb}_{(\mathrm{aq})}^{2+}+2 \mathrm{e}^{-} \longrightarrow \mathrm{Pb}_{(\mathrm{s})}\)
∴ 1 mol Pb will require 2 mol electrons, hence 2 Faradays are required.

(d) Reduction half reaction :
\(\mathrm{Cu}_{(\mathrm{aq})}^{+}+\mathrm{e}^{-} \longrightarrow \mathrm{Cu}_{(\mathrm{s})}\)
∴ 1 mol Cu will require 1 mol electrons hence one Faraday of electricity is required.

(14) In a certain electrolysis experiment, 0.561 g of Zn is deposited in one cell containing ZnSO₄ solution. Calculate the mass of Cu deposited in another cell containing CuSO₄ solution in series with ZnSO₄ cell. Molar masses of Zn and Cu are 65.4 g mol^-1 and 63.5 g mol^-1 respectively.
Solution :
Given : Mass of Zn deposited = W_Zn = 0.561 g
Molar mass of Zn = 65.4 g mol^-1
Molar mass of Cu = 63.5 g mol^-1
Mass of Cu deposited = ?
Number of moles of Zn deposited
= \(\frac{\text { Mass of Zn deposited }}{\text { Molar mass of } \mathrm{Zn}}=\frac{0.561}{65.4}\)
= 8.578 × 10^-3 mol Zn
Reactions of electronics:
(i) Zn⁺⁺ + 2e^– → Zn (Half reaction in ZnSO₄ cell)
(ii) Cu⁺⁺ + 2e^– → Cu (Half reaction in CuSO₄ cell)
Mole ratio of Zn

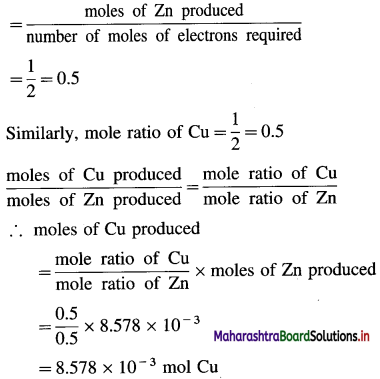
∴ Mass of Cu produced
= moles of Cu × molar mass of Cu
= 8.578 × 10^-3 × 63.5
= 0.5447 g Cu
Ans. Mass of Cu deposited = 0.5447 g

(15) Two electrolytic cells, one containing AlCl₃ solution and the other containing ZnSO₄ solution are connected in series. The same quantity of electricity is passed through the cells. Calculate the amount of Zn deposited in ZnSO₄ cell if 1.2 g of Al are deposited in AlCl₃ cell. The molar masses of Al and Zn are 27 g mol^-1 and 65.4 g mol^-1 respectively.
Solution :
Given : Mass of Al deposited = 1.2 g
Molar mass of Al = 27 g mol^-1
Molar mass of Zn = 65.4 g mol^-1
Mass of zinc deposited = ω_Zn = ?
Reduction reactions in electrolysis :

Number of moles of Al deposited = \(\frac{1.2}{27}\)
= 0.04444 mol.
From reaction (i),
∵ 1 mol Al requires 3 mol electrons
∴ 0.04444 mol Al requires 3 × 0.04444
= 0.1333 mol electrons
Hence 0.1333 moles of electrons are passed through both the cells in the series.
From reaction (ii),
∵ 2 moles of electrons deposit 1 mol Zn
∴ 0.1333 moles of electrons will deposit, \(\frac{0.1333}{2}\) = 0.06665 mol Zn
Mass of Zn deposited = 0.06665 × 65.4 = 4.36 g
Ans. Mass of Zn deposited = 4.36 g

(16) How much quantity of electricity in coulomb is required to deposit 1.346 × 10^-3 kg of Ag in 3.5 minutes from AgNO₃ solution ?
(Given : Molar mass of Ag is 108 × 10^-3 kg mol^-1)
Solution :
Given : Mass of Ag deposited = 1.346 × 10^-3 kg
Molar mass of Ag = 108 × 10^-3 kg mol^-1
Time = t = 3.5 × 60 s

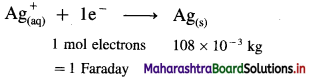
∵ 108 × 10^-3 kg Ag requires 1 Faraday
1.346 × 10^-3 kg Ag will require,
\(\frac{1.346 \times 10^{-3}}{108 \times 10^{-3}}\) = 0.01246 F
∵ If F = 96500 C
∴ 0.01246 F = 96500 × 0.01246 = 1202 C
Ans. Amount of electricity required = 1202 C

(17) How many electrons will have a total charge of 1 Coulomb ?
Solution :
Given : Charge = 1 Coulomb
Number of electrons = ?
1 Faraday = 96500 C per mol electrons
∵ 96500 C electric charge is present on 1 mol electrons
∴ 1C charge is present on \(\frac{1}{96500}\) mol electrons
∴ Number of electrons = \(\frac{1}{96500}\) × 6.022 × 10²³
= 6.24 × 10¹⁸ electrons
Ans. 1 Coulomb charge is present on 6.24 × 10¹⁸ electrons.

(18) A constant electric current flows for 4 hours through two electrolytic cells connected in series. One contains AgNO₃solution and second contains CuCl₂ solution. During this time, 4 grams of Ag are deposited in the first cell.
(a) How many grams of Cu are deposited in the second cell?
(b) What is the current flowing in amperes? (Atomic mass : Cu = 63.5 gmol^-1; Ag = 107.9 gmol^-1)
Solution :
Given : Mass of Ag deposited = 4 g
Molar mass of Cu = 63.5 g mol^-1
Molar mass of Ag = 107.9 g mol^-1
Time = t = 4 hrs = 4 × 60 × 60 = 14400 s
Mass of Cu deposited = W_Cu = ?
Current = I = ?
(a) Number of moles of Ag deposited
\(=\frac{\text { Mass of } \mathrm{Ag}}{\text { Molar mass of } \mathrm{Ag}}=\frac{4}{107.9}\)
= 0.03707 mol of Ag
Reactions of electrolysis :
(i) Ag⁺ + e^– → Ag (Half reaction in AgNO₃ cell)
(ii) Cu²⁺ + 2e^– → Cu (Half reaction in CuCl₂ cell)
Mole ratio of Ag

∴ Mass of Cu produced = 0.01854 × 63.5 = 1.177 g

(b) From the reaction,
∵ 1 mol Ag⁺ requires 1 mol electrons
∴ 0.03707 mol Ag will require 0.03707 mol electrons
∵ 1 mol electrons = 1 Faraday
∴ 0.03707 mol electrons = 0.03707 Faraday
∵ 1 Faraday = 96500 C
∴ 0.03707 Faraday
= 0.03707 × 96500 = 3577 C
∴ Quantity of electricity = Q = 3577 C.
Q = I × t
∴ I = \(\frac{\mathrm{Q}}{t}=\frac{3577}{14400}\) = 0.25 A
Ans. (a) Mass of Cu deposited = 1.177 g
(b) Current passed = 0.25 A

(19) The passage of 0.95 A current for 40 minutes deposited 0.7493 g Cu from CuSO₄ solution. Calculate the molar mass of Cu.
Solution :
Given : Electric current = I = 0.95 A
Time = f = 40 min = 40 × 60 = 2400 s
Mass of Cu deposited = 0.7493 g
Molar mass of Cu = ?
Reduction half reaction,
\(\mathrm{Cu}_{(\mathrm{aq})}^{2+}+2 \mathrm{e}^{-} \longrightarrow \mathrm{Cu}_{(\mathrm{s})}\)
Quantity of electricity = Q = I × t
= 0.95 × 2400
= 2280 C
Number of moles of electrons = \(\frac{2280}{96500}\)
= 0.02362 mol
∵ 2 mol electrons deposit 1 mol Cu
∴ 0.02362 mol electrons will deposit,
\(\frac{0.02362}{2}\) = 0.01181 mol Cu
Now,
0.01181 mol Cu weighs 0.7493 g
∴ 1 mol of Cu weigh, \(\frac{0.7493 \times 1}{0.01181}\) = 63.44 g
Hence molar mass of Cu 63.44 g mol^-1
Ans. Molar mass of Cu = 63.44 g mol^-1

(20) A quantity of 0.3 g of Cu was deposited from CuSO₄ solution by passing 4A through the solution for 3.8 min. Calculate the value of Faraday constant. (Atomic mass of Cu = 63.5 g mol^-1)
Solution :
Given : Mass of Cu deposited = 0.3 g
Electric current = I = 4A
Time = t = 3.8 min = 3.8 × 60 = 228 s
Value of Faraday = ?
Quantity of electricity passed = Q = I × t
= 4 × 228 = 912 C
Reduction half reaction,
\(\mathrm{Cu}_{(\mathrm{aq})}^{2+}+2 \mathrm{e}^{-} \longrightarrow \mathrm{Cu}_{(\mathrm{s})}\)
Number of moles of Cu deposited 0.3
= \(\frac{0.3}{63.5}\) = 0.004724 mol
From reduction half reaction,
1 mol Cu ≡ 2 mol electrons
∴ 0.004724 mol Cu = 2 × 0.004724
= 0.009448 mol electrons
Now
∵ 0.009448 mol electrons = 912 C
∴ 1 mol electrons = \(\frac{912}{0.009448}\) = 96528 C
∵ 1 Faraday charge is equal to charge on 1 mol electrons
∴ 1 Faraday = 96528 C
Ans. 1 Faraday = 96528 C

(21) In the electrolysis of water, one of the half reactions is
2H⁺_(aq) + 2e^– → H_2(g)
Calculate the volume of H₂ gas collected at 25 °C and 1 atm pressure by passing 2A for 1h through the solution. R = 0.08205 L atm K^-1 mol^-1.
Solution :
Given : Reduction half reaction :

Temperature = T = 273 + 25 = 298 K
Pressure = P = 1 atm
Electric current = I = 2A
Time = t = 1 hr = 1 × 60 × 60 = 3600 s
R = 0.08205 L atm K-1 mol-1
Volume of H₂ = V_H2 = ?
Quantity of electricity passed = Q = I × t
= 2 × 3600 = 7200 C
Number of moles of electrons = \(\frac{Q}{F}\)
\(\frac{7200}{96500}\) = 0.0746 mol
From the reaction,
∵ 2 mol electrons produces 1 mol H₂ gas
∴ 0.0746 mol electrons will produce \(\frac{0.0746}{2}\)
= 0.0373 mol H₂.
pV_H2 = nRT

= 0.912 L
Ans. Volume H₂ gas = 0.912 L

(22) Calculate the current strength and number of moles of electrons required to produce 2.369 × 10^-3 kg of Cu from CuSO₄ solution in one hour. (Molar mass of Cu is 63.5 g/mol)
Solution :
Given : Mass of Cu deposited = 2.369 × 10^-3 kg;
t = 1 hr = 3600 s
Molar mass of Cu = 63.5 g mol^-1
I = ?; Number of moles of electrons = ?

∵ For 63.5 × 10^-3 kg Cu Q = 2 × 96500 C
∴ For 2.369 × 10^-3 kg Cu

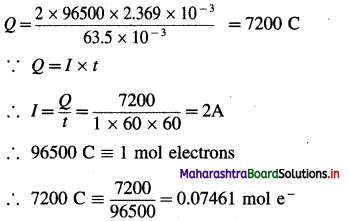
Ans. I = 2A; Number of moles of electrons = 0.07461

Question 39.
Define : Galvanic cell or voltaic cell.
Answer:
Galvanic or voltaic cell : An electrochemical cell which is used to produce electrical energy by a spontaneous chemical reaction inside it is called an electrochemical cell. In this chemical energy is converted into electrical energy.
Example : Daniell cell.

Question 40.
Define : Half cell or Electrode.
Answer:
Half cell or Electrode : It is a metal electrode dipped in the electrolytic solution and capable of establishing oxidation reduction equilibrium with one of the ions of electrolyte solution and develop electrode potential. E.g. Zn in ZnSO₄ solution.

Question 41.
What are the functions of a salt bridge ?
Answer:
The functions of a salt bridge are :

1. It maintains the electrical contact between the two electrode solutions of the half cells.
2. It prevents the mixing of electrode solutions.
3. It maintains the electrical neutrality in both the solutions of two half cells by a flow of ions.
4. It eliminates the liquid junction potential.

Question 42.
What are the conventions used to write galvanic cell or cell diagram (cell formula) ?
Answer:
A galvanic cell or voltaic cell is represented by a short notation or diagram which includes electrodes, aqueous solutions of ions and other species that may or may not involve in the cell reaction.
The following conventions are used to represent the cell or write the cell notation :
(1) The metal electrodes or the inert electrodes like platinum are placed at the ends of the cell formula.
(2) The galvanic cell consists of two half cells or electrodes. The electrode on the extreme left hand side is anode where oxidation takes place and it carries negative (-) charge while extreme right hand electrode is cathode where reduction takes place and it carries positive (+) charge.
(3) The gases or insoluble substances are placed in the interior positions adjacent to the metal electrode.
(4) A single vertical line is written between two phases like solid electrode and aqueous solution containing ions.
(5) A double vertical line is drawn between two solutions of two electrodes which indicates a salt bridge connecting them electrically.
(6) The concentration of solutions or ions or pressures of gases are written in brackets along with the substances in the cell.
(7) Different ions in the same solution are separated by a comma.

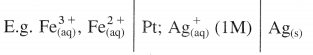
(8) Examples of electrochemical cells :
(i) Daniel cell is represented as,

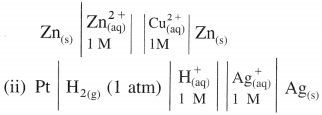
Question 43.
How to write cell reaction for a galvanic cell ?
Answer:
(1) A galvanic cell consists of two half cells or electrodes.
(2) Write oxidation half reaction for left hand electrode which is an anode and reduction half reaction for right hand electrode which is a cathode.
(3) Balance the number of electrons in the oxidation and reduction reactions.
(4) By adding both the reactions, overall cell reaction is obtained.
(5) For example, consider following cell :

Question 44.
Why is anode in a galvanic cell considered to be negative?
Answer:

1. According to IUPAC conventions, the electrode of a galvanic cell where de-electronation or oxidation takes place releasing electrons is called anode. Zn_(s) → \(\mathrm{Zn}_{(\mathrm{aq})}^{2+}\) + 2e^–
2. The electrons released due to oxidation reaction are accumulated on the metal electrode surface charging it negatively.

Hence anode in the galvanic cell is considered to be negative.

Question 45.
Why is cathode in a galvanic cell considered to be positive electrode?
Answer:
(1) According to IUPAC conventions, the electrode of the galvanic cell where electronation or reduction takes place is called cathode. In this, the electrons from the metal electrode are removed by cations required for their reduction.
\(\mathrm{Cu}_{(\mathrm{aq})}^{2+}\) + 2e^– → Cu_(s)

(2) Since the electrons are lost, the metal electrode acquires a positive charge.
Hence cathode in the galvanic cell is considered to be positive.

Question 46.
Give the cell reactions in the case of the following cells :
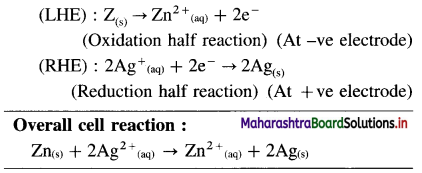
(1)

Answer:

(2)

Answer:

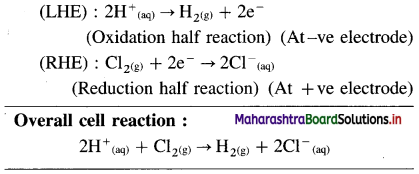
(3) Pt, H_2(g) | H⁺_(aq) || Cl^–_(aq) | Cl_2(g), Pt
Answer:

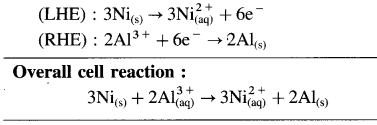
(4) Ni_(s)|Ni²⁺ (1 M) || Al³⁺ (1 M) | Al_(s)
Answer:

Question 47.
Represent the half cells or electrodes for the following reactions :

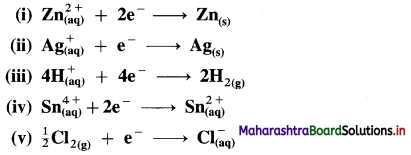
Answer:

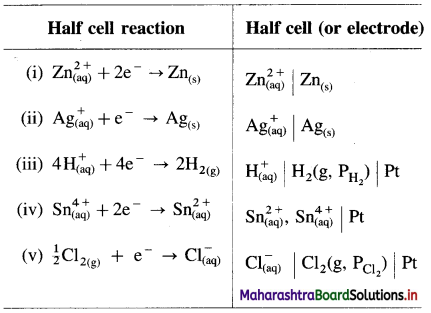
Question 48.
Formulate a cell from the following electrode reactions :
(a) Cl_2(g) + 2e^– → 2Cl^–_(aq)
(b) 2I^–_(aq) → I_2(s) + 2e^–
Answer:
(a) Cl_2(g) + 2e^– → 2Cl^–_(aq) (Reduction half reaction)
(b) 2I^–_(aq) → I_2(s) + 2e^– (Oxidation half reaction)
The galvanic cell is,
Pt |I_2(s)|I^–_(aq) (1 M) | Cl^–_(aq) (1 M) | Cl₂(g, P_Cl2)|Pt

Question 49.
Formulate a cell for each of the following reactions :
(a) \(\mathrm{Sn}_{\text {(aq) }}^{2+}\) + 2AgCl_(s) → \(\mathrm{Sn}_{(\mathrm{aq})}^{4+}\) + 2Ag(s) + \(2 \mathrm{Cl}_{(\mathrm{aq})}^{-}\)
(b) Mg_(s) + Br_2(l) → \(\mathrm{Mg}_{(\mathrm{aq})}^{2+}+2 \mathrm{Br}_{(\mathrm{aq})}^{-}\)
Answer:
(a) \(\mathrm{Sn}_{\text {(aq) }}^{2+}\) + 2AgCl_(s) → \(\mathrm{Sn}_{(\mathrm{aq})}^{4+}\) + 2Ag(s) + \(2 \mathrm{Cl}_{(\mathrm{aq})}^{-}\)
The overall reaction takes place into two steps :
(i) \(\mathrm{Sn}_{\text {(aq) }}^{2+}\) → Sn⁴⁺ + 2e^– (Oxidation half reaction)
(ii) 2AgCl_(s) + 2e^– → 2Ag_(s) + \(2 \mathrm{Cl}_{(\mathrm{aq})}^{-}\) (Reduction half reaction)
Hence the cell is,

(b) Mg_(s) + Br_2(l) → \(\mathrm{Mg}_{(\mathrm{aq})}^{2+}+2 \mathrm{Br}_{(\mathrm{aq})}^{-}\)
The overall reaction takes place into two steps :

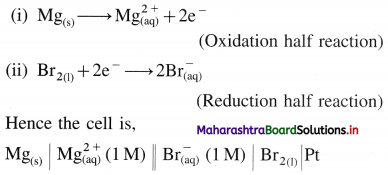
Question 50.
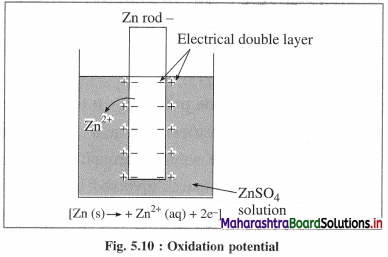
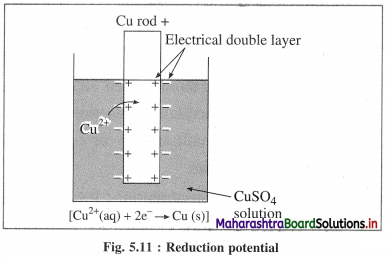
What is electrode potential?
Answer:
(1) Electrode potential : It is defined as the difference of electrical potential established due to electrode half reaction between metal electrode and the solution around it at equilibrium at constant temperature.

(2) Explanation : When a metal is immersed into a solution containing its ions there arises oxidation (or reduction) reaction involving a release of electrons (or gain of electrons). This gives rise to the formation of an electrical double layer, consisting of a charged metal surface and an ionic layer. The potential across this double layer i.e., between metal and the solution is an electrode potential.

Question 51.
Define :
(1) Oxidation potential,
(2) Reduction potential.
Answer:
(1) Oxidation potential : It is defined as the difference of electrical potential between metal electrode and the solution around it at equilibrium developed due to oxidation reaction at anode and at constant temperature.

(2) Reduction potential : It is defined as the difference of electrical potential between metal electrode and the solution around it at equilibrium developed due to reduction reaction at cathode and at constant temperature.

Question 52.
What is a standard state of a substance ?
Answer:
The standard state of a substance is that state in which the substance has unit activity or concentration at 25 °C. i.e., For solution having concentration 1 molar, gas at 1 atm, pure liquids or solids are said to be in their standard states.

Question 53.
Define the following terms :
(1) Standard electrode potential
(2) Standard oxidation potential
(3) Standard reduction potential.
Answer:
(1) Standard electrode potential : It is defined as the difference of electrical potential between metal electrode and the solution around it equilibrium when all the substances involved in the electrode reaction are in their standard states of unit activity or concentration at constant temperature.

(2) Standard oxidation potential : It is defined as the difference of electrical potential between metal electrode and the solution around it at equilibrium due to oxidation reaction, when all the substances involved in the oxidation reaction are in their standard states of unit activity or concentration at constant temperature.

(3) Standard reduction potential : It is defined as the difference of electrical potential between metal electrode and the solution around it at equilibrium due to reduction reaction, when all the substances involved in the reduction reaction are in their standard states of unit activity or concentration at constant temperature.

Question 54.
What is the standard potential of an electrode according to IUPAC convention?
Answer:
Standard reduction potential : According to IUPAC convention, the standard potential of an electrode due to reduction reaction at 298 K is taken as the standard reduction potential. In this active mass of the substance has unit value.

Question 55.
What is cell potential or emf of a cell ?
Answer:
Cell potential or emf of a cell : It is defined as the potential difference between two electrodes, responsible for an external flow of electrons from the left hand electrode at higher potential (anode), to the right hand electrode at lower potential (cathode), when connected to form an electrochemical or galvanic cell.

Since there is oxidation reaction at left hand electrode (LHE) or anode and reduction reaction at right hand electrode (RHE) or cathode, emf of the galvanic, Ecell, is given by
E_cell = (E_oxi)_anode + (E_red)_cathode
Since by IUPAC conventions, generally reduction potentials are used, hence, for the given cell,
(∵ E_oxi = -E_red)
∴ E_cell = (E_red)_cathode – (E_red)_anode
Similarly, standard emf of the cell, E⁰_cell is given by
E⁰_cell = (E⁰_red)_cathode – (E⁰_red)_anode

Question 56.
Explain dependence of cell potential on concentration.
OR
Explain Nernst equation for cell potential.
Answer:
Consider following general reaction taking place in the galvanic cell.
aA + bB → cC + dD
The cell voltage is given by,

where,
T → temperature
R → Gas constant
F → Faraday
n → Number of electrons in the redox cell reaction.

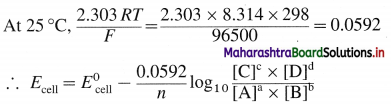
This is Nernst equation for cell potential. It is used to calculate cell potential and electrode potentials.

Question 57.
State (or write) Nernst equation for the electrode potential and explain the terms involved.
Answer:
The Nernst equation for the single electrode reduction potential for a given ionic concentration in the solution in the case, \(M_{(a q)}^{n+}\) + ne^– → M_(s) is given by

\(\mathrm{E}_{\mathrm{M}^{\mathrm{n}+} / \mathrm{M}}\) is the single electrode potential,
\(E_{\mathrm{M}^{n+} / \mathrm{M}}^{0}\) is the standard reduction electrode potential,
R is the gas constant = 8.314 JK^-1 mol^-1
T is the absolute temperature,
n is the number of electrons involved in the reaction,
F is Faraday (96500 C)
[Mn⁺] is the molar concentration of ions.

Question 58.
Obtain Nernst equation for the following cell :

Answer:
Electrode reactions and a cell reaction for the given cell are,

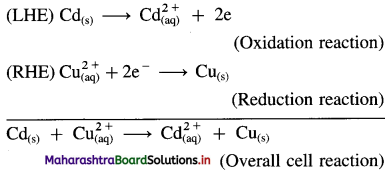
Here, n = 2
By Nernst equation, the cell potential is given by,

Question 59.
Obtain Nernst equation for the electrode potential for the electrode, \(\mathrm{Zn}_{(\mathrm{aq})}^{2+} \mid \mathrm{Zn}_{(\mathrm{s})}\).
Answer:
For the electrode, \(\mathrm{Zn}_{(\mathrm{aq})}^{2+} \mid \mathrm{Zn}_{(\mathrm{s})}\),
the reduction reaction is,
\(\mathrm{Zn}_{(\mathrm{aq})}^{2+}+2 \mathrm{e}^{-} \longrightarrow \mathrm{Zn}_{(\mathrm{s})}\) ∴ n = 2
By Nernst equation, the reduction electrode potential is given by,

where E⁰_zn²⁺/zn is the standard electrode potential of zinc electrode.

Question 60.
Obtain a relation between cell potential and Gibbs energy for the cell reaction.
Answer:
Consider a galvanic cell which involves n number of electrons in the overall cell reaction. Since one mole of electrons involve the electric charge equal to one Faraday (F) which is equal to 96500 C, the total charge involved in the reaction is,
Electric charge = n × F
If Ecell is the cell potential, then Electrical work = n × F × E_cell
According to thermodynamics, electric work is equal to decrease in Gibbs energy, -ΔG, we can write,
Electric work = n × F × E_cell = -ΔG
∴ ΔG = -nFE_cell
Under standard conditions, we can write
∴ ΔG⁰ = -nF\(E_{\text {cell }}^{0}\)
where \(E_{\text {cell }}^{0}\) is the standard cell potential and ΔG⁰ is the standard Gibbs free energy change.

Question 61.
Write Nernst Equation for the following reactions :
(a) Cr_(s) + 3Fe³⁺_(aq) → Cr³⁺_(aq) + 3Fe²⁺_(aq)
(b) Al³⁺_(aq) + 3e^– → Al_(s)
Answer:
(a) Cr_(s) + 3Fe³⁺_(aq) → Cr³⁺_(aq) + 3Fe²⁺_(aq)
The cell formulation is,
Cr_(s)|Cr³⁺_(aq) || Fe³⁺_(aq), Fe²⁺_(aq)| Pt
Hence cell potential is,

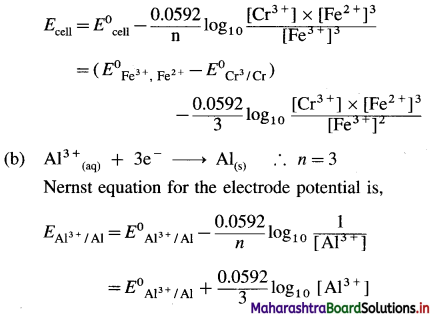
Question 62.
A single electrode potential can’t be measured but the cell potential can be measured. Explain.
Answer:
(1)


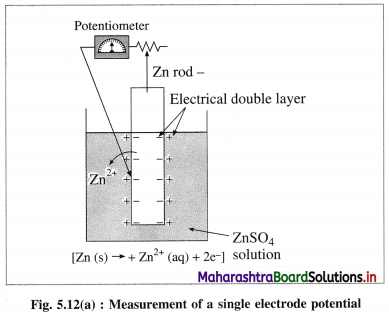
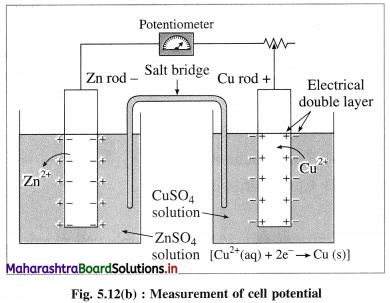
According to Nemst theory, electrode potential is the potential difference between the metal and ionic layer around it at equilibrium, i.e. the potential across the electric double layer.

(2) For measuring the single electrode potential, one part of the double layer, that is metallic layer can be connected to the potentiometer but not the ionic layer. Hence, single electrode potential can’t be measured experimentally.

(3) When an electrochemical cell is developed by combining two half cells or electrodes, they can be connected to the potentiometer and the potential difference or cell potential can be measured.
E_cell = E₂ – E₁
where E₁ and E₂ are reduction potentials of two electrodes.

(4) If one of the electrode potentials is known or arbitrarily assumed and E_cell is measured by potentiometer, then potential of another electrode can be obtained. Therefore it is necessary to choose a reference electrode with arbitrarily fixed potential and measure the potentials of other electrodes.

(5) Therefore Standard Hydrogen Electrode (SHE) is selected assuming arbitrary potential 0.0 volt. Hence potentials of all other electrodes are referred to as hydrogen scale potentials.

Question 63.
Describe the construction and working of the standard hydrogen electrode (S.H.E.). Give its advantages and disadvantages.
OR
What is the standard hydrogen electrode
OR
Primary reference electrode? Write the construction and working of it.
Answer:
A single electrode potential cannot be measured, but the cell potential can be measured experimentally. Hence, it is necessary to have a reference electrode. S.H.E. is a primary reference electrode.
(1) Construction :
(1) The standard hydrogen electrode (S.H.E.) consists of a glass tube at the end of which a piece of platinised platinum foil is attached as shown in Fig. 5.14. Around this plate there is an outer jacket of glass which has a side inlet through which pure and dry hydrogen gas is bubbled at one atmosphere pressure. The inner tube is filled with a little mercury and a copper wire is dipped into it. This provides an electrical contact with the platinum foil. The outer jacket ends into a broad opening.

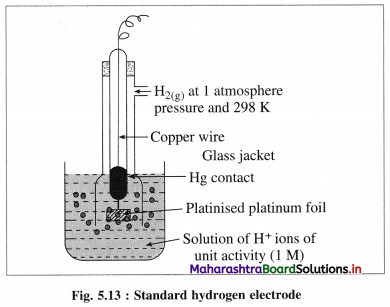
(2) The whole assembly is kept immersed in a solution containing hydrogen ions (H⁺) of unit activity.
(3) This electrode is arbitrarily assigned zero potential.
(4) The platinised platinum foil is used to provide an electrical contact for the electrode. This permits rapid establishment of the equilibrium between the hydrogen gas adsorbed by the metal and the hydrogen ions in solution.

(2) Representation of S.H.E. :
H⁺ (1 M) | H₂ (g, 1 atm) | Pt

(3) Working :
Reduction : H⁺_(aq) + e^– ⇌ \(\frac {1}{2}\)H_2(g) E⁰ = 0.00 V
H₂ gas in contact with H⁺_(aq) ions attains an equilibrium establishing a potential.

(4) Applications of SHE : A reversible galvanic cell with the experimental (indicator) electrode, Zn²⁺ (1M) | Zn_(s) and SHE can be developed as follows :

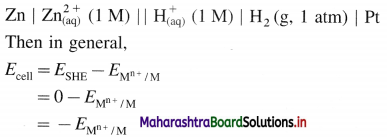
Thus the potential can be directly obtained.

(5) Disadvantages (Drawbacks or Difficulties) :

• It is difficult to construct and handle SHE.
• Pure and dry H₂ gas cannot be obtained.
• Pressure of H₂ gas cannot be maintained exactly at 1 atmosphere.
• The active mass or concentration of H⁺ from HCl cannot be maintained exactly unity.

Question 64.
How is the potential of hydrogen electrode obtained?
Answer:
Hydrogen gas electrode is represented as,
H⁺_(aq) | H₂ (g, P_H2) | Pt
Electrode reduction reaction is,
2H⁺_(aq) + 2e^– → H_2(g)
By Nernst equation, the reduction potential is,

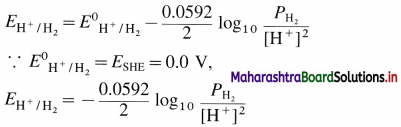
If H₂ gas is passed at 1 atm, then P_H2 = 1 atm

Question 65.
Draw the diagram for the determination of standard electrode potential with SHE.
Answer:
Consider the following cell :
Zn | Zn²⁺_(aq) || HCl | H₂(g, 1atm) | Pt

Question 66.
A voltaic cell consisting of Fe²⁺_(aq)|Fe_(s) and Bi³⁺_(aq) | Bi_(s) electrodes is constructed. When the circuit is closed, mass of Fe electrode decreases and that of Bi electrode increases.
(a) Write cell formula, (b) Which electrode is cathode and which electrode is anode ? (c) Write electrode reactions and overall cell reaction.
Answer:
(a) Since the mass of Fe electrode decreases, it undergoes oxidation and it is an anode or an oxidation electrode while as the mass of Bi electrode increases, there is a reduction of Bi³⁺ to Bi and it is cathode or a reduction electrode. Hence the cell formula is,
\(\mathrm{Fe}_{(\mathrm{s})}\left|\mathrm{Fe}_{\mathrm{(aq})}^{2+}(1 \mathrm{M}) \| \mathrm{Bi}_{(\mathrm{aq})}^{3+}(1 \mathrm{M})\right| \mathrm{Bi}\)

(b) The left hand electrode is an anode and right hand electrode is a cathode.

(c) Reactions :

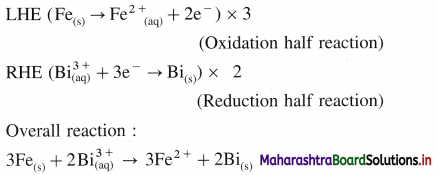
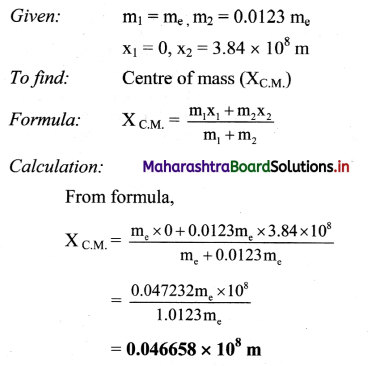
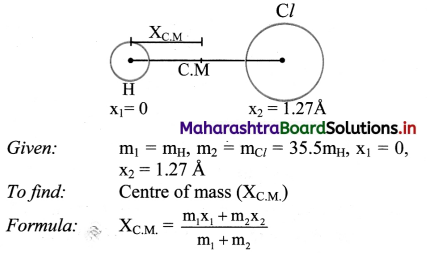
Solved Examples 5.7 – 5.9

Question 67.
Solve the following :

(1) Write the reaction and calculate the potential of the half cell,
\(\mathbf{Z n}_{(\mathbf{a q})}^{2+}\) (0.2M) | Zn. (E⁰_Zn²⁺/Zn = – 0.76 V).
Solution :
Given : E⁰_Zn²⁺/Zn = -0.76 V
Concentration of Zn²⁺ = [Zn²⁺] = 0.2 M
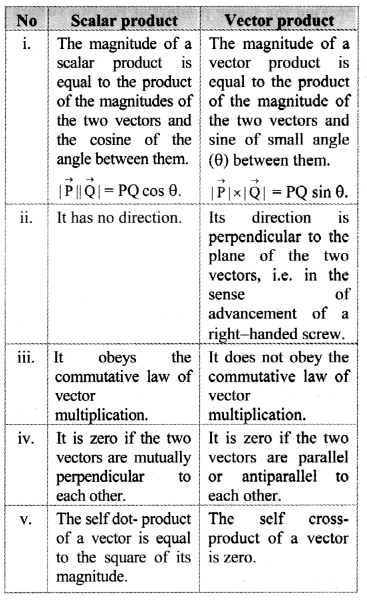
E_Zn²⁺/Zn = ?
Reduction reaction for the half cell,

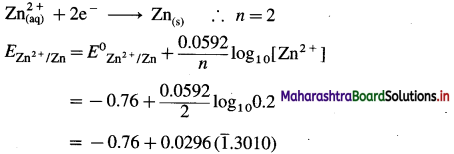
= – 0.76 + 0.0296 (-0.6990)
= -0.76 – 0.02069
= -0.78069 V
Ans. E⁰_Zn²⁺/Zn = -0.78069 V

(2) Write a reaction and calculate the potential of the electrode, \(\mathrm{Cl}_{(\mathrm{aq})}^{-}\) (0.05 M) | Cl₂ (g, 1 atm) | Pt E⁰_Cl2/Cl^– = 1.36 V.
Solution :
Given : Reduction reaction :

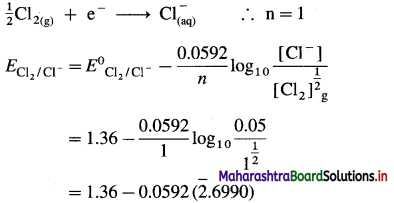
= 1.36 – 0.0592 (- 2 + 0.6990)
= 1.36 – 0.0592 (-1.3010)
= 1.36 + 0.077
= 1.437 V
Ans. Potential of the electrode = 1.437 V

(3) Calculate the potential of the electrode,
pH = 4.5 | H₂ (g, 1 atm) |Pt.
Solution :
Given : pH = 4.5

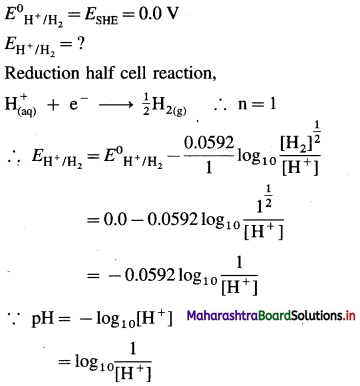
∴ E_H⁺/H2 = -0.0592 pH
= -0.0592 × 4.5
= -0.2664 V
Ans. E_H⁺/H2 = -0.2664 V

(4) If the standard cell potential of Daniell cell is 1.1 V, calculate standard free energy change for the cell reaction.
Solution :
Given : Daniell cell :

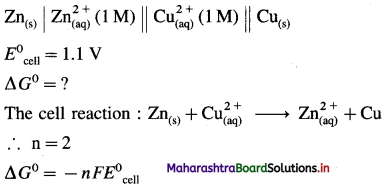
= – 2 × 96500 × 1.1
= -212300 J
= -212.3 kJ
Ans. Standard free energy change = ΔG⁰
= -212.3 kJ

(5) Write balanced equations for the half reactions and calculate the reduction potentials at 25 °C for the following half cells :
(a) Cl^– (1.2 M) | Cl₂(g, 3.6 atm) E⁰ = 1.36 V
(b) Fe²⁺ (2 M) | Fe_(s) E⁰ = – 0.44 V
Solution :
(a) Given : Half cell,
\(\mathrm{Cl}_{(\mathrm{aq})}^{-}\) (1.2 M) | Cl₂(g, 3.6 atm)|Pt
E⁰_Cl2/Cl^– = 1.36 V
The reduction reaction:

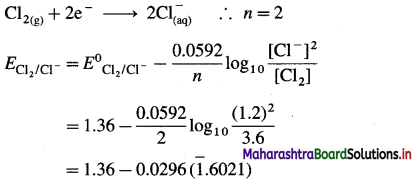
= 1.36 – 0.0296 (-0.3979)
= 1.36 + 0.01178
= 1.37178
≅ 1.372 V

(b) Given: Half cell, \(\mathrm{Fe}_{(\mathrm{aq})}^{2+}\) (2M) |Fe_(s)
E⁰ _Fe²⁺/Fe = -0.44 V
The reduction reaction:

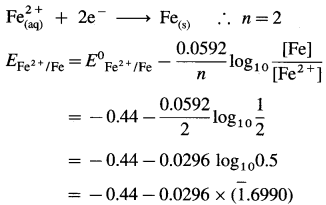
= – 0.44 – 0.0296 × (- 0.3010)
= -0.44 + 0.00891
= -0.43109 V
Ans. (a) Half reaction : Cl_2(g) + 2e^– → \(2 \mathrm{Cl}_{\text {(aq) }}^{-}\)
E_Cell = 1.372 V
(b) \(\mathrm{Fe}_{(\mathrm{aq})}^{2+}\) + 2e^– → Fe_(s)
E_Cell = -0.43109 V.

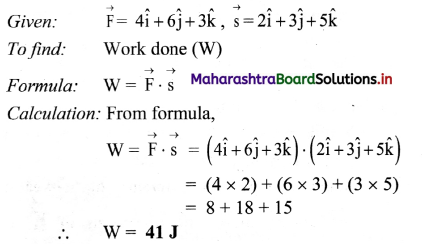
(6) Using Nernst equation, calculate the potentials for the following half reactions :

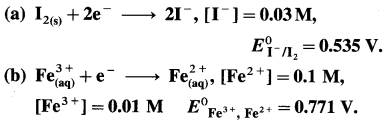
Solution :
(a) Given :

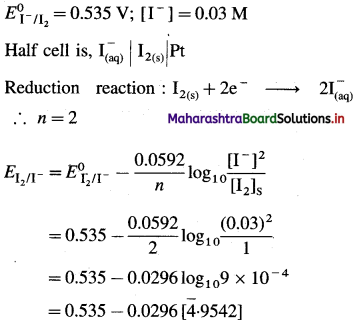
= 0.535 – 0.0296 [ – 4 + 0.9542]
= 0.535 – 0.0296 [-3.0458]
= 0.535 + 0.0902
= 0.6252 V

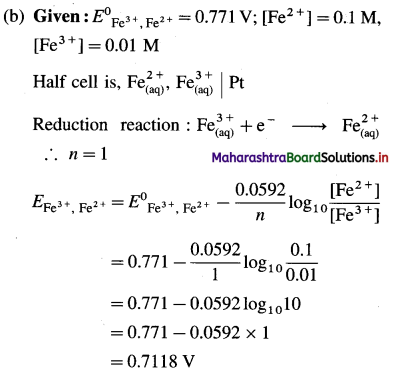
Ans. (a) Potential of the half cell = 0.6252 V
(b) Potential of the half cell = 0.7118 V.

(7) Write the cell reaction and calculate the standard potential of the cell,
Ni_(s) | Ni²⁺(1 M) || Cl^–(1M) | Cl₂ (g, 1 atm) | Pt
E⁰_Cl2 = 1.36 V and E⁰Ni = – 0.25 V.
Solution :

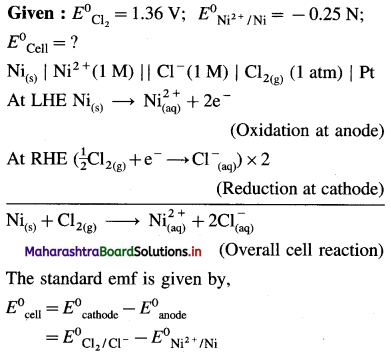
= 1.36 – (-0.25)
= 1.36+ 0.25 = 1.61V
Ans. Cell reaction : Ni_(s) + Cl_2(g) → \(\mathrm{Ni}_{(\mathrm{aq})}^{2+}+2 \mathrm{Cl}_{(\mathrm{aq})}^{-}\)
E⁰_Cell = 1.61 V

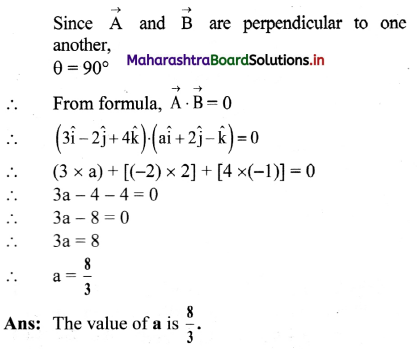
(8) Write the cell reaction and calculate cell potential and standard free energy change for a cell reaction in the following cell :
\(\mathbf{A l}_{(\mathrm{s})}\left|\mathbf{A l}_{(\mathbf{a q})}^{3+}(1 \mathbf{M}) \| \mathbf{C d}_{(\mathbf{a q})}^{2+}(1 \mathrm{M})\right| \mathbf{C} d\)
E⁰_Al³⁺/Al = -1-66 V and E⁰_cd²⁺/cd = -0.403 V
Solution :

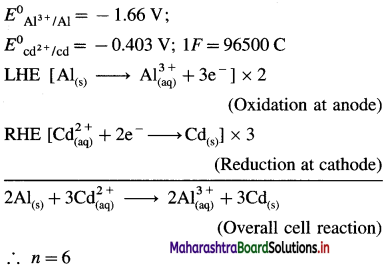
Since concentrations of ions are 1 M each, it is a standard cell, hence the cell potential is E⁰_Cell.

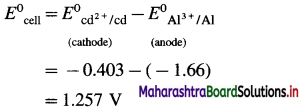
Standard free energy change ΔG⁰ is given by,
ΔG⁰= – nFE⁰_Cell
= -6 × 96500 × 1.257
= – 727800 J
= -727.8 kJ
Ans. Cell reaction :

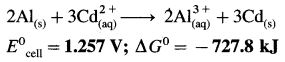
(9) Write the cell reaction and calculate cell potential and the standard free energy change for the cell reaction in the following cell :
Pt | H₂ (g, 1 atm) | \(\mathbf{H}_{(\mathrm{aq})}^{+}\)(1M) || \(\mathrm{Cu}_{\text {(aq) }}^{2+}\) (1M) | Cu_(s).
Mention anode and cathode and direction of flow of electrons in the external circuit. (E⁰_Cu²⁺/Cu = 0.337 V)
Solution :
Given : E⁰_Cu²⁺/Cu = 0.337 V;
E⁰_H⁺/H2 = E⁰_SHE = 0.0V
Pt | H₂(g, 1 atm) | \(\mathbf{H}_{(\mathrm{aq})}^{+}\) (1M) || \(\mathrm{Cu}_{\text {(aq) }}^{2+}\) (1M) | Cu_(s)

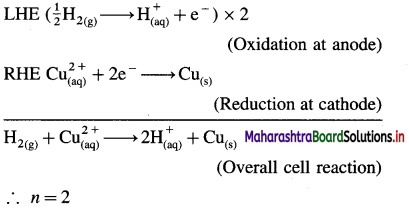
Anode : Hydrogen gas electrode (LHE)
Cathode : Copper electrode (RHE)
E⁰_Cell = E⁰_Cu²⁺/Cu – E⁰_H⁺/H2
= 0.337 – (0.0)
= 0.337 V
ΔG⁰ = – nFE⁰ = -2 × 96500 × 0.337
= – 65040J
= – 65.04 kJ
Electrons in the external circuit will flow from (LHE) hydrogen gas electrode to (RHE) copper electrode.
Ans. Cell reaction : H_2(g) + \(\mathrm{Cu}_{(\mathrm{aq})}^{2+}\) → 2 \(\mathrm{H}_{(\mathrm{aq})}^{+}\) + Cu_(s)
Cell potential = E⁰_Cell = 0.337 V
ΔG⁰ = -65.04 kJ

(10) Calculate the reduction potential of the electrode, Zn²⁺ (0.02 M) | Zn_(s). E⁰_Zn⁺⁺/Zn = – 0.76 V.
Solution :
Given :E⁰_red = E⁰_Zn⁺⁺/Zn = -0.76 V;
Concentration of \(\mathrm{Zn}_{(\mathrm{aq})}^{2+}\) = [Zn²⁺] = 0.02 M
The reduction reaction for the electrode,
\(\mathrm{Zn}_{(\mathrm{aq})}^{2+}\) +2e^– → Zn_(s); ∴ n = 2
The reduction potential is given by,

= – 0.76 + 0.0296 (- 1.6990)
= -0.76 – 0.0296 × 1.6990
= -0.76 – 0.0503
= -0.8103 V
Ans. E_red = E_Zn²⁺/Zn = -0.8103 V

(11) Calculate the potential of the following cell at 25 °C :
Zn | Zn²⁺(0.6 M) ||H⁺(1.2 M) | H₂ (g, 1 atm) | Pt
E°Zn2+/Zn = -0.763 V
Solution :
Given : E⁰_Zn²⁺/Zn = -0.763 V;
Concentrations : [Zn²⁺] = 0.6 M; [H⁺] = 1.2 M
[H₂]_g = 1 atm
Cell potential = E_cell = ?

= 0.763 – 0.0296 × (- 0.3801)
= 0.763 + 0.01125
= 0.77425 V
Ans. Cell potential = E⁰_cell = 0.77425 V

(12) The following redox reaction occurs in a galvanic cell.
2Al_(s) + 3Fe²⁺(1 M) → 2Al³⁺(1 M)+ 3Fe_(s)
(a) Write the cell notation.
(b) Identify anode and cathode
(c) Calculate E⁰_cell if E⁰_anode = – 1.66 V and E⁰_cathode = – 0 44 V
(d) Calculate ΔG⁰ for the reaction.
Solution :

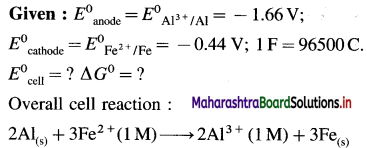
(a) In the cell reaction, Al is oxidised from zero to 3+ while Fe³⁺ is reduced from 3+ to zero. Hence the cell notation is,

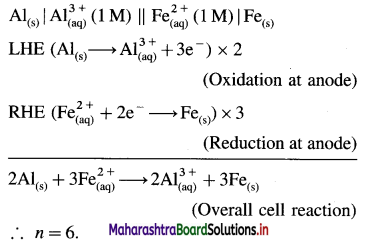
(b) Anode : Al electrode at LHE
Cathode : Fe electrode at RHE

(d) The standard free energy change ΔG⁰ is given by,
ΔG⁰ = – nFE⁰_cell
= – 6 × 96500 × 1.22
= – 70640 J
= – 706.4 kJ
Ans. (a) Cell notation :
\(\mathrm{Al}_{(\mathrm{s})}\left|\mathrm{Al}_{(\mathrm{aq})}^{3+}(1 \mathrm{M}) \| \mathrm{Fe}_{(\mathrm{aq})}^{2+}(1 \mathrm{M})\right| \mathrm{Fe}_{(\mathrm{s})}\)
(b) Anode : Al; Cathode : Fe
(c) E⁰_cell = 1.22 V
(d) ΔG⁰ = – 706.4 kJ

(13) Construct a cell consisting of \(\mathbf{N i}_{(\mathrm{aq})}^{2+}\) | Ni(s) half cell and H⁺ | H_2(g) | Pt half cell.
(a) Write the cell reaction
(b) Calculate emf of the cell if [Ni²⁺] = 0.1M,
P_H2 = 1 atm [H⁺] = 0.05 M and
E⁰_Ni = – 0.257 V.
Solution :


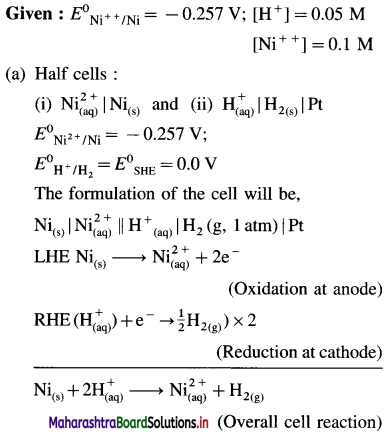
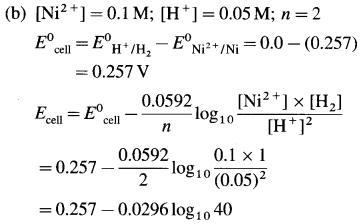
= 0.257 – 0.0296 × 1.6020
= 0.257 – 0.04742
= 0.20958
≅ 0.2096 V
Ans.

(14) Calculate the cell potential of the following cell at 25°C,

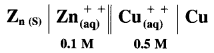
Standard reduction potentials (SRP) of Zn and Cu are -0.76 V and 0.334 V respectively.
Solution:
Given:

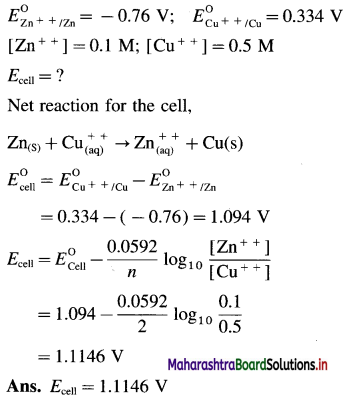
(15) Set up the cell consisting of \(\mathbf{H}_{\text {(aq) }}^{+} \mid \mathbf{H}_{2(\mathrm{~g})}\) and \(\mathbf{P b}_{(\mathbf{a q})}^{2+}\) | Pb_(s) electrodes. Calculate the emf at 25 °C of the cell if [Pb²⁺] = 0.1 M,
[H⁺] = 0.5 M and hydrogen gas is at 2 atm pressure. E⁰_pb²⁺/pb = – 0.126 V.
Solution :
Given : Half cells :

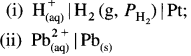
Concentrations : [H⁺] = 0.5 M; [Pb²⁺] = 0.1M;
[H₂]_g = P_H2 = 2 atm; E⁰_H⁺/H2 = E_SHE = 0.0 V;
E⁰_pb²⁺/pb = -0.126 V
Since E⁰_pb²⁺/pb (reduction) < E⁰_H⁺/H2 the Pb electrode is anode and hydrogen gas electrode is cathode.
The cell formulation :


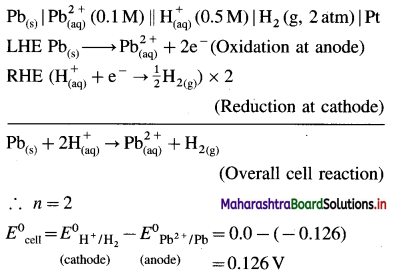
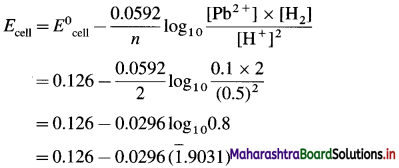
= 0.126 – 0.0296 (- 0.0969)
= 0.126 + 0.002868
= 0.128868
≅ 0.1289 V
Ans. E_cell = 0.1289 V

(16) Consider a galvanic cell that uses the half reactions,
2H⁺_(aq) + 2e^– → H_(g)
Mg²⁺_(aq) + 2e^– → Mg_(s)
Write balanced equation for the cell reaction. Calculate E⁰_cell, E_cell and ΔG⁰ if concentrations are 1M each and P_H2 = 10 atm
E⁰_Mg²⁺/Mg = -2.37 V.
Solution :



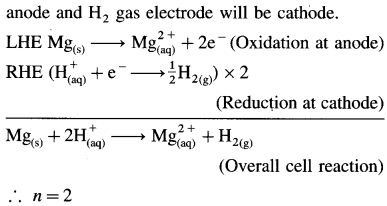
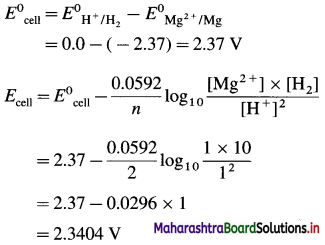
The standard free energy change ΔG⁰ is given by
ΔG⁰= – nFE⁰_cell
= – 2 × 96500 × 2.37
= -457400 J
= – 457.4 kJ
Ans. E⁰_cell = 2.37 V; E_cell = 2.3404 V;
ΔG⁰ = -457.4 kJ

(17) Calculate E_cell and ΔG for the following at 28 °C : Mg_(s) + Sn²⁺ (0.04M) → Mg²⁺ (0.06M) + Sn_(s)
E⁰_cell = 2.23 V
Is the reaction spontaneous ?
Solution:
Given:
Mg_(s) + Sn²⁺ (0.04 M) → Mg²⁺ (0.06 M) + Sn
[Sn²⁺] = 0.4 M
[Mg²⁺] = 0.06 M
E⁰_cell = 2.23V
E_cell = ?
ΔG = ?

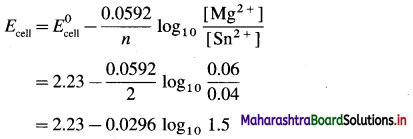
2.23 – 0.0296 × 0.1761
= 2.23 – 0.005213
= 2.224V
ΔG = – nFE
= – 2 × 96500 × 2.224
= – 4.292 × 105 J
= -429.2 kJ
Since ΔG is negative, the electrochemical reaction is spontaneous.

(18) The standard potentials for Sn²⁺/Sn and Fe²⁺/Fe half reactions are -0.136 V and -0.440 V respectively. At what relative concentrations of Sn²⁺ and Fe²⁺ will these have the same reduction potentials?
Solution :



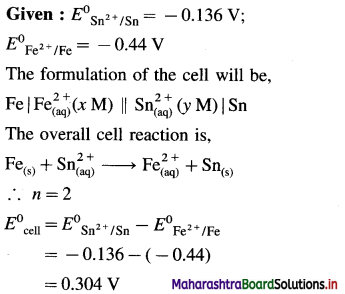
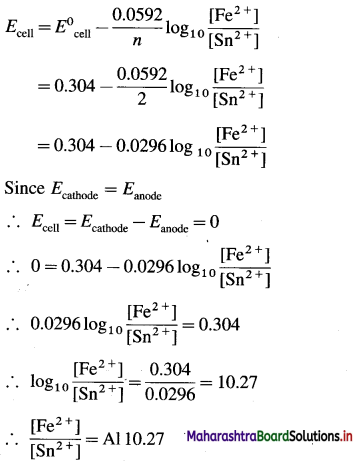
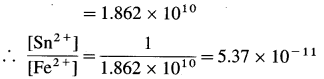
Hence when relative concentrations of Sn²⁺ and Fe²⁺ i.e., [Sn²⁺]/[Fe²⁺] = 5.37 × 10^-11, both the electrodes will have same potential.

(19) Write the cell reaction and calculate the emf of the cell at 25 °C.
Cr_(s) | Cr³⁺(0.0065 M) || Co²⁺(0.012 M) | Co_(s)
E⁰_Co = – 0.280 V, E⁰_Cr = – 0.74 V
What is ΔG for the cell reaction ?
Solution :
Given :

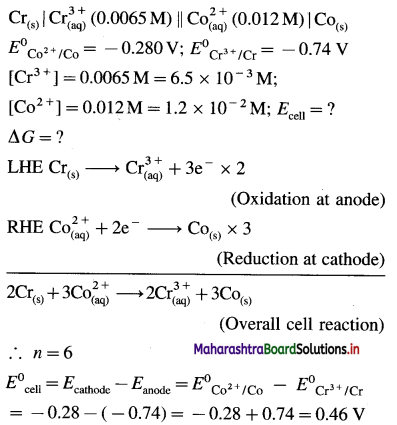
By Nernst equation,

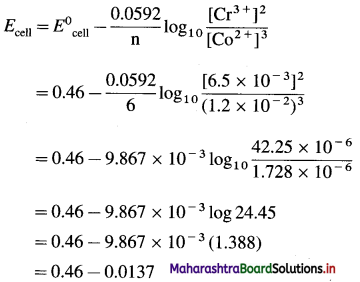
Ecell = 0.4463 V
ΔG = -nFE_Cell
= – 6 × 96500 × 0.4463
= – 258407 J
= – 258.4 kJ
Ans. Cell reaction :
\(2 \mathrm{Cr}_{(\mathrm{s})}+3 \mathrm{Co}_{(\mathrm{aq})}^{2+} \rightarrow 2 \mathrm{Cr}_{(\mathrm{aq})}^{3+}+3 \mathrm{Co}_{(\mathrm{s})}\)
E_Cell = 0.4463 V; ΔG = – 258.4 kJ.

(20) Calculate E⁰_Cell, ΔG⁰ and equilibrium constant for the reaction 2Cu⁺ → Cu²⁺ + Cu.
E⁰_Cu⁺/Cu = 0.52 V and E⁰_Cu²⁺,Cu+ = 0.16 V.
Solution :
Given : Cell reaction : 2Cu⁺_(aq) → Cu²⁺_(aq) +Cu_(s)
E⁰_Cu⁺/Cu = 0.52V; E⁰_Cu²⁺,Cu+ = 0.16 V
1F = 96500 C
E⁰_Cell = ? ΔG⁰ = ? K=?
(i) The formulation of the cell :

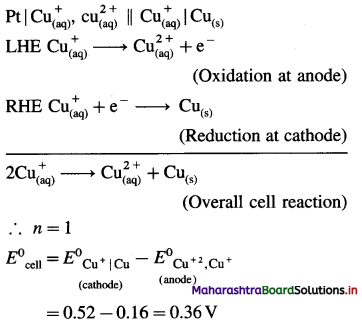
(ii) ΔG⁰ = – nFE⁰_Cell = – 1 × 96500 × 0.36
= – 34740 J
= – 34.74 kJ
(iii) Electrochemical redox reactions are considered as reversible reactions. If K is the equilibrium constant for the electrochemical redox reaction, then

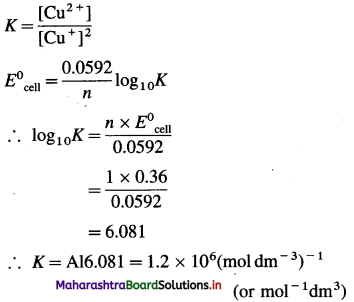
Ans. E⁰_Cell = 0.36 V; ΔG⁰ = – 34.74 kJ; Equilibrium constant = K= 1.2 × 10⁶ mol^-1 dm³.

(21) Calculate the equilibrium constant for the redox reaction at 25 °C.
Sr_(s) + Mg²⁺ → Sr²⁺_(aq) + Mg_(s),
that occurs in a galvanic cell. Write the cell formula.
E⁰_Mg = – 2.37 V and E⁰_Sr = – 2.89 V.
Solution :
Given :
Cell reaction : Sr_(s) + Mg²⁺ → Sr²⁺_(aq) + Mg_(s)
E⁰ _Mg²⁺/Mg = -2.37 V; E⁰ _Sr²⁺/Sr = -2.89 V
Equilibrium constant K = ?
The formation of the cell :

Ans. Equilibrium constant = K = 3.698 × 10¹⁷

(22) The equilibrium constant for the following reaction at 25 °C is 2.9 × 10⁹. Calculate standard voltage of the cell.
Cl_2(g) + 2Br^–_(aq) ⇌ Br_2(l) + 2Cl^–_(aq)
Solution :
Given : Cell reaction : Cl_2(g) + 2Br^–_(aq) ⇌ Br_2(l) + 2Cl^–_(aq)
Equilibrium constant = K = 2.9 × 10⁹ atm^-1
Standard voltage of the cell = E⁰_Cell = ?
The formulation of the cell :

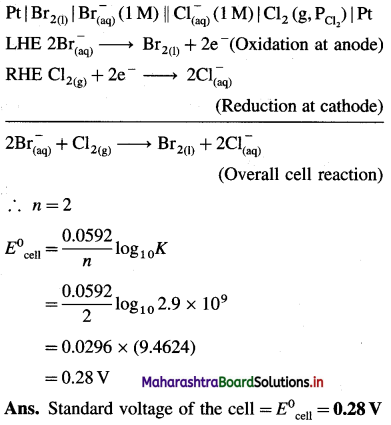
(23) Write the cell representation and calculate equilibrium constant for the following redox reaction :
Ni_(s) + 2 Ag⁺_(aq) (1M) → Ni²⁺_(aq) (1 M) + 2Ag_(s)
at 25 °C
E⁰_Ni = – 0.25 V and E⁰_Ag = 0.799 V
Solution :
Given : E⁰_Ni²⁺/Ni = – 0.25 V; E⁰_Ag⁺/Ag = 0.799 V
Equilibrium constant = K = ?

(24) Calculate the cell potential of the following galvanic cell :
Pt|H₂ (g, 1 atm)|\(\mathbf{H}_{\text {(aq) }}^{+} \mathbf{p H}\) = 3.51||Calomel electrode
E_cal = 0.242 V at 25 °C.
Solution :

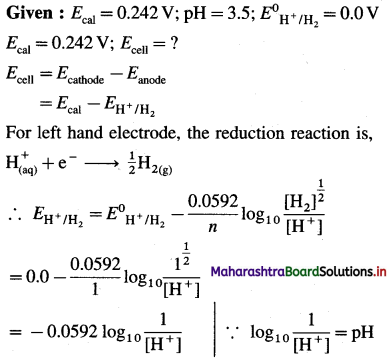
= -0.0592 × pH
= -0.0592 × 3.5
= -0.2072 V
∴ E_cell = E_cal – E_H⁺/H2
= 0.242 – (-0.2072)
= 0.242 + 0.2072
= 0.4492 V
Ans. E_cell = 0.4492 V

Question 68.
How are the voltaic cells classified ?
Answer:
The voltaic cells are classified as primary and secondary voltaic cells.
(1) Primary voltaic cells : These are the voltaic cells in which the electrical energy or cell potentials are developed within the cells due to oxidation and reduction reactions at the reversible electrodes.

The chemicals and electrode materials consumed during the discharging can be regenerated by passing the current in opposite direction from the external source of electricity i.e., these cells can be recharged. For example, Daniell cell. There are the examples where the primary cells can’t be recharged. E.g. Dry cell.

(2) Secondary voltaic cells :
(i) These are the voltaic cells in which the electrical energy or cell potentials are not developed within the cell but electrical energy can be stored or cell potentials can be regenerated by passing electricity from the external source of electricity. Since the electrical energy obtained is second hand, these cells are called secondary cells or accumulators or storage cells.

(ii) These cells can be recharged by passing electric current in opposite direction from the external source of higher emf. Therefore the secondary cells are reversible cells. For example, lead accumulator (lead storage battery).

Question 69.
Explain the construction and working of a dry cell (or Leclanche’s cell).
OR
Write a note on dry cell.
Answer:
(A) Principle :

• Leclanche’s cell is a primary voltaic cell.
• It doesn’t contain mobile liquid electrolyte but contains moist viscose aqeuous paste of the electrolytes.
• It is an irreversible voltaic cell which can’t be recharged.

(B) Construction :
(i) It consists of a small zinc vessel which serves as an anode (negative electrode).

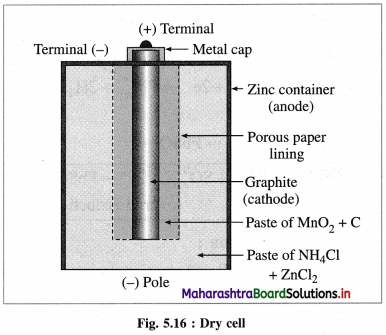
(ii) The zinc vessel contains a porous paper bag containing an inert graphite (C) electrode which serves as cathode, immersed in a paste of MnO₂ and carbon black. This paper bag divides the dry cell into two compartments, namely anode and cathode compartments.
(iii) The rest of the cell is filled with a moist paste of NH₄Cl and ZnCl₂ which acts as an electrolyte for zinc anode.
(iv) The graphite rod is fitted with a metal cap and the cell is sealed to prevent the drying of moist paste by evaporation.

(C) The dry cell can be represented as,
^– Zn|ZnCl_2(aq), NH₂Cl_(aq), MnO_2(s)|C⁺.

(D) Reactions in the dry cell :
(i) Oxidation at zinc anode :
Zn_(s) → \(\mathrm{Zn}_{\text {(aq) }}^{2+}\) + 2e^– (oxidation half reaction)
(ii) Reduction at graphite (C) cathode :
The electrons released in the oxidation reaction at anode, flow to cathode through external circuit.
Hydrogen in NH₄ ion is reduced to molecular hydrogen which reduces MnO₂ to Mn₂O₃.

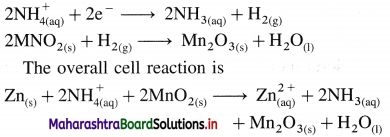
(iii) Zn²⁺ react with NH₃ and form a complex.
\(\mathrm{Zn}_{(\mathrm{aq})}^{2+}+4 \mathrm{NH}_{3(\text { aq) }} \longrightarrow\left[\mathrm{Zn}\left(\mathrm{NH}_{3}\right)_{4}\right]^{2+}{ }_{(\mathrm{aq})}\)
Since Zn²⁺ ions are removed, the overall cell reaction can’t be reversed.

(E) Uses of dry cell :

• Dry cell is used as a source of electric power in radios, flashlights, torches, clocks, etc.
• Since they are available in small size and portable, they can be used conveniently.

Question 70.
Describe the construction and working of lead accumulator (lead storage cell).
OR
Draw a neat labelled diagram of the lead accumulator. Explain the reactions involved in discharging and charging this cell. Represent this cell using cell conventions.
Answer:
(A) Principle :
(1) The lead accumulator is a secondary electrochemical cell since electrical energy and emf are not developed within the cell but it is previously stored by passing an electric current. Hence it is also called lead accumulator or lead storage battery.
(2) It is reversible since the electrochemical reaction can be reversed by passing an electric current in opposite direction and consumed reactants can be regenerated.
(3) Hence battery can be charged after it is discharged.

(B) Construction : In a lead accumulator, the negative terminal (anode) is made up of lead sheets packed with spongy lead, while the positive terminal (cathode) is made up of lead grids packed with PbO₂.

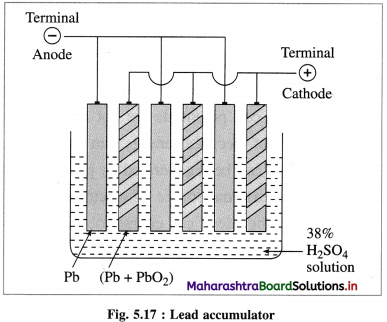
Sulphuric acid of about 38% strength (%w/w) or specific gravity 1.28 or 4.963 molar is the electrolyte in which the lead sheets and lead grids are dipped. The positive terminal and negative terminal are alternatively arranged in the electrolyte and are separately interconnected.

(C) Representation of lead accumulator :

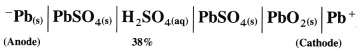
(D) Working of a lead accumulator :
(1) Discharging : When the electric current is withdrawn from lead accumulator, the following reactions take place :
Oxidation at the – ve electrode or anode :

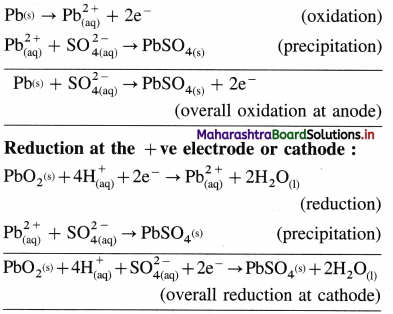
(2) Net cell reaction :
(i) Thus, the overall cell reaction during discharging is

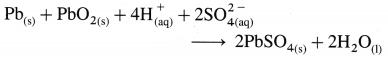
OR
Pb_(s) + PbO_2(s) + 2H₂SO_4(aq) → 2PbSO_4(s) + 2H₂O_(l)
The cell potential or emf of the cell depends upon the concentration of sulphuric acid. During the operation, the acid is consumed and its concentration decreases and specific gravity decreases from 1.28 to 1.17. As a result, the emf of the cell decreases. The emf of a fully charged cell is about 2.0 V.

(ii) Recharging of the cell : When the discharged battery is connected to external electric source and a higher external potential is applied the cell reaction gets reversed generating H₂SO₄.
Reduction at the – ve electrode or cathode :
PbSO_4(s) + 2e^– → Pb(s) + \(\mathrm{SO}_{4(a q)}^{2-}\)
Oxidation at the + ve electrode or anode :
PbSO_4(s) + 2H_2(l)O → PbO_2(s) + 4H⁺_(aq) + \(\mathrm{SO}_{4(a q)}^{2-}\) + 2e^–
The net reaction during charging is
2PbSO_4(s) + 2H₂O_(l) → Pb_(s) + PbO_2(s) + 4H⁺_(aq) + 2\(\mathrm{SO}_{4(a q)}^{2-}\)
OR
2PbSO_4(s) + 2H₂O → Pb_(s) + PbO_2(s) + 2H₂SO_4(aq)
The emf of the accumulator depends only on the concentration of H₂SO₄.

(E) Applications :

1. It is used as a source of d.c. electric supply.
2. It is used in automobile in ignition circuits and lighting the head lights by connecting 6 batteries giving 12V potential.
3. It is also used in invertors.

Question 71.
In lead accumulator which electrode is coated with PbO₂ ? Anode or cathode ?
Answer:
In lead accumulator, cathode is coated with PbO₂.

Question 72.
Write net charging and discharging reactions for lead storage battery.
Answer:
For lead storage battery :
Net charging reaction :
2PbSO_4(s) + 2H₂O_(l) → Pb_(s) + PbO_2(s) + 2H₂SO_4(aq)
Net discharging reaction :
Pb_(s) + PbO_2(s) + 2H₂SO_4(aq) → 2PbSO_4(s) + 2H₂O_(l)

Question 73.
Write a note on Nickel-Cadmium (NICAD) cell.
Answer:
(1) Nickel-Cadminum (NICAD) cell is a secondary dry cell.
(2) It is rechargable, hence it is a reversible cell.
(3) It consists of a cadmium electrode in contact with an alkali and acts as anode while nickel (IV) oxide, NiO₂ in contact with an alkali acts as cathode. The alkali used is moist paste of KOH.
(4) Reactions in the cell :
(i) Oxidation at cadmium anode :
Cd_(s) + 2OH^–_(aq) → Cd(OH)_2(s) + 2e^–
(ii) Reduction at Ni02(s) cathode :
NiO_2(s) + 2H₂O_(l) + 2e^– → Ni(OH)_2(s) + 2OH^–_(aq)
The overall cell reaction is the combination of above two reactions.
Cd_(s) + NiO_2(s) + 2H₂O_(l) → Cd(OH)_2(s) + Ni(OH)_2(s)
(5) Since the net cell reaction doesn’t involve any electrolytes but solids, the voltage is independent of the concentration of alkali electrolyte.
(6) The cell potential is about 1.4 V.
(7) This cell has longer life than other dry cells.

Question 74.
Write a note on mercury battery.
Answer:
(1) Mercury battery is a rechargeable secondary dry cell.
(2) It consists of zinc anode amalgamated with mercury.
(3) The cathode consists of a paste of Hg and carbon.
(4) The electrolyte is a paste of KOH and ZnO in a strong alkaline medium.
(5) Reactions:

(6) The overall reaction involves only solid substances and electrolytic composition remains unchanged.
(7) Therefore mercury battery provides constant voltage (1.35 V) over a long period.
(8) It is superior to Leclanche’s cell in durability.
(9) Uses : It is used in hearing aids, electric watches, pacemakers, etc.

Question 75.
Describe the construction and working of hydrogen-oxygen (H₂-O₂) fuel cell.
Answer:
(A) Principle :
(i) The functioning of the fuel cell is based on the combustion reaction like,
2H_2(g) + O_2(g) → 2H₂O_(g) is exothermic redox reaction and hence it can be used to produce electricity.
(ii) The reactants of this fuel cell can be continuously supplied from outside, hence this can be used to supply electrical energy for a very long period.

(B) Construction :
(i) In fuel cell the anode and cathode are porous electrodes with suitable catalyst like finely divided platinum.

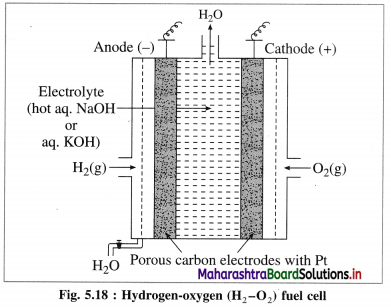
(ii) The electrolyte used is hot aqueous KOH solution in which porous anode and cathode carbon rods are immersed.
(iii) H₂ is continuously bubbled through anode while O₂ gas is bubbled through cathode.

(C) Working (cell reactions) :
(i) Oxidation at anode : At anode, hydrogen gas is oxidised to H₂O.
2H_2(g) + 4OH^–_(aq) → 4H₂O_(l) + 4e^– (oxidation half reaction)
(ii) Reduction at cathode : The electrons released at anode travel to cathode through external circuit and reduce oxygen gas to OH-.
O_2(g) + 2H₂O_(l) + 4e^– → 4OH^–_(aq) (reduction half reaction)
(iii) Net cell reaction: Addition of both the above reactions at anode and cathode gives a net cell reaction.
2H_2(g) + O_2(g) → 2H₂O_(l) (overall cell reaction)

(D) Representation of the cell :

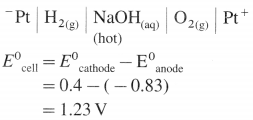
The overall cell reaction is an exothermic combustion reaction. However in this, H₂ and O₂ gases do not react directly but react through electrode reactions. Hence the chemical energy released in the formation of O-H bonds in H₂O, is directly converted into electrical energy.

(E) Advantages :

1. The fuel cell operates continuously as long as H₂ and O₂ gases are supplied to the electrodes.
2. The cell reactions do not cause any pollution.
3. The efficiency of this galvanic cell is the highest about 70% as compared to ordinary galvanic cells.

(F) Drawbacks of H₂-O₂ fuel cell :

1. The cell requires expensive electrodes like Pt, Pd.
2. In practice, voltage is less than 1.23 volt due to spontaneous reactions at the electrodes.
3. H₂ gas is expensive and hazardous.

(G) Applications :

1. It was successfully used in spacecraft.
2. It has potential applications in automobiles, power generators for domestic and industrial uses.

Question 76.
What are the applications of the fuel cells?
Answer:

1. Fuel cells have been used in the space programme providing electrical energy for a long duration.
2. The fuel cells have been used in automobiles on experimental basis.
3. In case of H₂-O₂ fuel cell, used in spacecraft, the water produced is used for drinking for astronauts.
4. The fuel cells using methanol as a fuel for combustion are used in electronic products such as cell phones and laptop computers.
5. The fuel cells have many potential applications as power generators for domestic and industrial uses.

Question 77.
In what way fuel cell differs from ordinary galvanic cells ?
Answer:

1. Fuel cell is a modified galvanic cell in which the thermal energy of combustion reactions is directly converted into electrical energy.
2. In the fuel cell, the reactants are not placed within the cell like ordinary galvanic cells, but they are continuously supplied to the electrodes from outside reservoir.
3. They cannot be recharged unlike ordinary galvanic cell.

Question 78.
Define electrochemical series or electromotive series.
Answer:
Electrochemical series (Electromotive series) : It is defined as the arrangement in a series of electrodes of elements (metal or non-metal in contact with their ions) with the electrode half reactions in the decreasing order of their standard reduction potentials.

Question 79.
Explain electrochemical series or electromotive series.
Answer:
The conventions used in the construction of electrochemical series (or electromotive series) are as follows :

• The (reduction) electrodes or half cells of the elements are written on the left hand side of the series and they are arranged in the decreasing order of their standard reduction potentials (E°red).
• Reduction half reactions are written for each half cell in such a way that the species with higher oxidation state and electrons are on left hand side while reduced species with lower oxidation state are on right hand side.
• The standard reduction potential of standard hydrogen electrode is 0.00 V, i.e., E⁰_H⁺/H2 = 0.0 V. The electrodes and half cell reactions with positive E⁰_red values are located above hydrogen and those with negative E⁰_red values below hydrogen. Above hydrogen, positive E⁰_red values increase, while below hydrogen negative E⁰ values increase.
• The positive E⁰_red values indicate the tendency for reduction and the negative E⁰_red values indicate the tendency for oxidation.
• The elements, whose electrodes are at the top of the series having high positive values for E⁰_red are good oxidising agents.
• The elements, whose electrodes are at the bottom of the series having high negative values for E⁰_red are good reducing agents.

Question 80.
What are the applications of electrochemical series (or electromotive series) ?
Answer:
The applications of electrochemical series (or electromotive series) are as follows :
(1) Relative strength of oxidising agents in terms of E⁰_red values : The E⁰_red value is a measure of the tendency of the species to be reduced i.e., to accept electrons and act as an oxidising agent. The species mentioned on left hand side of the half reactions are oxidising agents.

The substances in the upper positions in the series and hence in the upper left side of the half reactions have large positive E⁰_red values hence are stronger oxidising agents. For example, F₂, Ce⁴⁺, Au³⁺, etc. As we move down the series, the oxidising power decreases. Hence from the position of the elements in the electrochemical series, oxidising agents can be selected.

(2) Relative strength of reducing agents in terms of E⁰_red values : The lower E⁰_red value means lower tendency to accept electrons but higher tendency to lose electrons. The tendency for reverse reaction or oxidation increases as E⁰_red becomes more negative and we move towards the lower side of the series. For example, Li, K, Al, etc. are good reducing agents.

(3) Identifying the spontaneous direction of reaction : From the standard reduction potentials, E⁰_red, the spontaneity of a redox reaction can be determined. The difference between E⁰_red values for any two electrodes represents cell potential E⁰_cell, constituted by them.

If E°cell is positive then the reaction is spontaneous while if E⁰_cell is negative the reaction is non-spontaneous. For example, E⁰_Mg²⁺/Mg and E⁰_Ag⁺/Ag have values -2.37 V and 0.8 V respectively. Then Mg will be a better reducing agent than Ag. Therefore Mg can reduce Ag⁺ to Ag.

The corresponding reactions will be:

Therefore above reaction in the forward direction will be spontaneous while in the reverse direction will be non-spontaneous since for it E⁰_cell = -3.17V.

(4) Calculation of standard cell potential E⁰_cell : From the electrochemical series, the standard cell potential, E⁰_cell from the E⁰_red values for the half reactions given can be calculated.
For example,

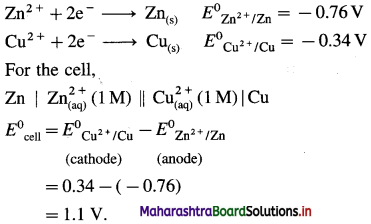
Question 81.
Write any four applications of electrochemical series.
Answer:
The applications of electrochemical series are as follows :

1. Predicting relative strength of oxidising agents.
2. Predicting relative strength of reducting agents.
3. Identifying the spontaneous direction of a reaction.
4. To calculate the standard cell potential E°cell.

Multiple Choice Questions

Question 82.
Select and write the most appropriate answer from the given alternatives for each subquestion :

1. The cell constant of a conductivity cell is given by
(a) l × a
(b) \(\frac{a}{l}\)
(c) \(\frac{1}{l \times a}\)
(d) \(\frac{l}{a}\)
Answer:
(d) \(\frac{l}{a}\)

2. A conductivity cell has two platinum electrodes of area 1.2 cm² and 0.92 cm apart. Hence the cell constant is
(a) 1.104 cm^-1
(b) 1.304 cm^-1
(c) 0.906 cm^-1
(d) 0.767 cm^-1
Answer:
(d) 0.767 cm^-1

3. The conductivity of 0.02 M KI solution is 4.37 × 10^-4 Ω^-1 cm^-1. Hence its molar conductivity is
(a) 8.74 × 10^-6 Ω^-1 cm² mol^-1
(b) 21.85 Ω^-1 cm² mol^-1
(c) 4.58 × 10^-4 Ω^-1 cm² mol^-1
(d) 136.5 Ω^-1 cm² mol^-1
Answer:
(b) 21.85 Ω^-1 cm2 mol^-1

4. The specific conductance of 0.02 M HCl is 8.2 × 10^-3 Ω^-1 cm^-1. Hence its molar conductivity is
(a) 164 Ω^-1 cm² mol^-1
(b) 6.1 × 10³Ω^-1 cm² mol^-1
(c) 239.6 S cm² mol^-1
(d) 410 S cm² mol^-1
Answer:
(d) 410 S cm² mol^-1

5. Molar conductivity of an electrolyte is given by,

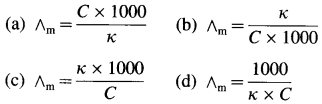
Answer:
(c) \(\wedge_{\mathrm{m}}=\frac{\kappa \times 1000}{\mathrm{C}}\)

6. The units of molar conductivity are
(a) Ω cm^-2 mol^-1
(b) Ω^-1 cm² mol^-1
(c) Ω^-1 cm^-1 mol^-1
(d) Ω^– cm^-1 mol^-2
Answer:
(b) Ω^-1 cm² mol^-1

7. If conductivity is expressed in Ω^-1 m^-1 and concentration of the electrolytic solution in mol m^-3 then, the molar conductance is given by

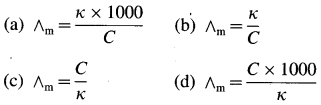
Answer:
(b) \(\wedge_{\mathrm{m}}=\frac{\kappa}{C}\)

8. Kohlrausch’s law is represented as

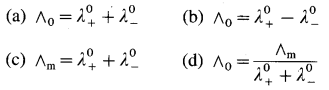
Answer:
(a) \(\wedge_{0}=\lambda_{+}^{0}+\lambda_{-}^{0}\)

9. The degree of dissociation of a weak electrolyte is given by

Answer:
(c) α = \(\frac{\wedge_{\mathrm{m}}}{\wedge_{0}}\)

10. ∧₀ for CH₃COOH is 390.7 Ω^-1 cm² mol^-1. If ∧₀ for CH₃COOK, and HBr in Ω^-1 cm² mol^-1 are 115 and 430.4 respectively, then ∧0 for KBr is
(a) 74.6 Ω^-1 cm² mol^-1
(b) 180.6 Ω^-1 cm² mol^-1
(c) 154.7 Ω^-1 cm² mol^-1
(d) 706.1 Ω^-1 cm² mol^-1
Answer:
(c) 154.7 Ω^-1 cm² mol^-1

11. The molar conductivity of cation and anion of salt BA are 180 and 220 mhos respectively. The molar conductivity of salt BA at infinite dilution is
(a) 90 mhos · cm² · mol^-1
(b) 110 mhos · cm² · mol^-1
(c) 200 mhos · cm² · mol^-1
(d) 400 mhos · cm² · mol^-1
Answer:
(d) 400 mhos · cm² · mol^-1

12. If ∧_m and ∧₀ are the molar conductivities of a weak electrolyte at concentration C and at zero concentration, then the dissociation constant Ka is given by

Answer:
(b) K_a = \(\frac{\wedge_{\mathrm{m}}^{2} \times \mathrm{C}}{\Lambda_{0}\left(\wedge_{0}-\wedge_{\mathrm{m}}\right)}\)

13. What is the ratio of volumes of H₂ and O₂ liberated during electrolysis of acidified water ?
(a) 1 : 2
(b) 2 : 1
(c) 1 : 8
(d) 8 : 1
Answer:
(b) 2 : 1

14. What weight of copper will be deposited by passing 2 Faradays of electricity through a cupric salt? (atomic mass = 63.5)
(a) 63.5 g
(b) 31.75 g
(c) 127 g
(d) 12.7 g
Answer:
(a) 63.5 g

15. The S.I. unit of cell constant for conductivity cell is
(a) m^-1
(b) S·m^-2
(c) cm^-2
(d) S·dm²·mol^-1
Answer:
(a) m^-1

16. The charge of how many coulomb is required to deposit 1.0 g of sodium metal (molar mass 23.0 g mol^-1) from sodium ions is
(a) 2098
(b) 96500
(c) 193000
(d) 4196
Answer:
(d) 4196

17. The amount of electricity equal to 0.05 F is
(a) 48250 C
(b) 3776 C
(c) 4825 C
(d) 4285 C
Answer:
(c) 4825 C

18. The number of electrons that have a total charge of 965 coulombs is
(a) 6.022 × 10²³
(b) 6.022 × 10²²
(c) 6.022 × 10²¹
(d) 3.011 × 10²³
Answer:
(c) 6.022 × 10²¹

19. When 0.2 Faraday of electricity is passed through an electrolytic solution, the number of electrons involved are
(a) 96500
(b) 1.603 × 10^-19
(c) 1.2046 × 10²³
(d) 12 × 10⁶
Answer:
(c) 1.2046 × 10²³

20. When a charge of 0.5 Faraday is passed through AlCl₃ solution, the amount of aluminium deposited at the cathode is (Atomic weight of Al = 27)
(a) 4.5
(b) 18
(c) 27
(d) 2.7
Answer:
(a) 4.5

21. The quantity of electricity required to deposit 54 g of silver from silver nitrate solution is
(a) 0.5 Coulomb
(b) 0.5 Ampere
(c) 0.5 Faraday
(d) 0.5 Volt
Answer:
(c) 0.5 Faraday

22. Passage of 5400 C of electricity through an electrolyte deposited 5.954 × 10^-3 kg of the metal with atomic mass 106.4. The charge on the metal ion is
(a) + 1
(b) + 2
(c) + 3
(d) + 4
Answer:
(a) + 1

23. On calculating the strength of current in amperes if a charge of 840 C (coulomb) passes through an electrolyte in 7 minutes, it will be
(a) 1
(b) 2
(c) 3
(d) 4
Answer:
(b) 2

24. On passing 1.5 F charge, the number of moles of aluminium deposited at cathode are [Molar mass of Al = 27 gram mol^-3]
(a) 1.0
(b) 13.5
(c) 0.50
(d) 0.75
Answer:
(c) 0.50

25. Number of faradays of electricity required to liberate 12 g of hydrogen is
(a) 1
(b) 8
(c) 12
(d) 16
Answer:
(c) 12

26. Daniell cell is
(a) Secondary cell
(b) Irreversible cell
(c) primary irreversible cell
(d) primary reversible cell
Answer:
(d) primary reversible cell

27. In the representation of galvanic cell, the ions in the same phase are separated by a
(a) single vertical line
(b) comma
(c) double vertical lines
(d) semicolon
Answer:
(b) comma

28. In the Daniell cell, reduction occurs at the
(a) anode
(b) zinc rod
(c) negative electrode
(d) positive electrode
Answer:
(d) positive electrode

29. The standard hydrogen electrode is represented as
(a) \(\mathrm{H}_{(\mathrm{aq})}^{+}\)|H₂(g, 1 atm) | Pt
(b) \(\mathrm{H}_{(\mathrm{aq})}^{+}\) 1M | H₂(g, 1 atm) | Pt
(c) \(\mathrm{H}_{(\mathrm{aq})}^{+}\) 1M|H_2(g)|Pt
(d) \(\mathrm{H}_{(\mathrm{aq})}^{+}\) 0.1M|H₂(g, 1 atm) | Pt
Answer:
(b) \(\mathrm{H}_{(\mathrm{aq})}^{+}\) 1M | H₂(g, 1 atm) | Pt

30. The essential condition to set a standard hydrogen electrode is
(a) 298 K
(b) pure and dry H₂ gas at 1 atm
(c) solution containing H⁺ at unit activity
(d) all of these
Answer:
(d) all of these

31. In hydrogen-oxygen fuel cell, the carbon rods are immersed in hot aqueous solution of
(a) KCl
(b) KOH
(c) H₂SO₄
(d) NH₄Cl
Answer:
(b) KOH

32. The emf of cell is 1.3 volt. The positive electrode has potential of 0.5 volt. The potential of negative electrode is
(a) 0.8 V
(b) -0.8 V
(c) 1.8 V
(d) – 1.8 V
Answer:
(b) -0.8 V

33. The electrode potential of a silver electrode dipped in 0.1 M AgNO₃ solution at 298 K is (E⁰_red of Ag = 0.80 volt)
(a) 0.0741 V
(b) 0.0591 V
(c) 0.741 V
(d) 0.859 V
Answer:
(c) 0.741 V

34. Which of the following species gains electrons more easily ?
(a) Na⁺
(b) H⁺
(c) Mg⁺
(d) Hg⁺
Answer:
(b) H⁺

35. In Nernst equation the constant 0.0592 at 298 K represents the value of

Answer:
(d) \(\frac{2.303 R T}{F}\)

36. The concept of electrode potential is explained on the basis of
(a) Arrhenius’ theory
(b) Ostwald’s theory
(c) Nemst’s theory
(d) Faraday’s law
Answer:
(c) Nemst’s theory

37. The standard reduction potentials of metals A and B are x and y respectively. If x > y, the standard emf of the cell containing these electrodes would be
(a) 2x – y
(b) y – x
(c) x – y
(d) x + y
Answer:
(c) x – y

38. The emf of the cell,

(E⁰_red = 0.34 V)
(a) -1.34
(b) 0.34 V
(c) -0.34 V
(d) 1.34
Answer:
(b) 0.34 V

39. The Electromotive Force of the following Cell Cu|Cu⁺⁺ (1 M)||A⁺g (1 M)|Ag is …………….. if E⁰_cu⁺⁺ = 0.33 V and E⁰ _Ag⁺⁺/Ag = 0.79 V
(a) 0.46 V
(b) – 0.46 V
(c) 1.12 V
(d) – 112 V
Answer:
(a) 0.46 V

40. The standard cell potential of the following cell is 0.463 V Cu|Cu⁺⁺ (1 M)||Ag⁺ (1 M)|Ag. If E⁰_Ag = 0.8 V, what is the standard potential of Cu electrode ?
(a) 1.137 V
(b) 0.337 V
(c) 0.463 V
(d) – 0.463 V
Answer:
(b) 0.337 V

41. The metal which cannot displace hydrogen from dil. H₂SO₄ solution is
(a) Zn
(b) Al
(c) Fe
(d) Ag
Answer:
(d) Ag

42. In the Lead storage battery during discharging
(a) pH of the electrolyte increases
(b) pH decreases
(c) pH remain unchanged
(d) pH increases or decreases depends on the extent of discharging
Answer:
(a) pH of the electrolyte increases

43. During the discharging of a lead storage battery,
(a) H₂SO₄ is consumed
(b) PbSO₄ is consumed
(c) Pb²⁺ ions are formed
(d) Pb is formed
Answer:
(a) H₂SO₄ is consumed

44. In lead accumulator, anode and cathode are
(a) (Pb + PbO₂), Pb
(b) Pb, PbO₂
(b) PbO₂, Pb
(d) Pb, (Pb + PbO₂)
Answer:
(d) Pb, (Pb + PbO₂)

45. The efficiency of the hydrogen-oxygen fuel cell is about
(a) 20%
(b) 40%
(c) 70%
(d) 90%
Answer:
(c) 70%

46. The strongest oxidizing agent among the species In³⁺ (E⁰ = – 1.34 V), Au³⁺ (E⁰ = 1.4 V), Hg²⁺ (E⁰ = 0.86 V), Cr³⁺ (E⁰ = – 0.74 V) is
(a) Cr³⁺
(b) Au³⁺
(c) Hg²⁺
(d) In³⁺
Answer:
(b) Au³⁺

47. The reaction, \(2 \mathrm{Br}_{(\mathrm{aq})}^{-}+\mathrm{Sn}_{(\mathrm{aq})}^{2+} \longrightarrow \mathrm{Br}_{2(\mathrm{l})}+\mathrm{Sn}_{(\mathrm{s})}\)
with the standard potentials, E⁰_Sn = -0.114 V, E⁰_Br2 = + 1.09 V, is
(a) spontaneous in reverse direction
(b) spontaneous in forward direction
(c) at equilibrium
(d) non-spontaneous in reverse direction
Answer:
(a) spontaneous in reverse direction

48. The cell potential of the following cell is
(E⁰_Al³⁺/Al = – 1.66 V)

(a) 1.66 V
(b) -1.66 V
(c) 0.5533 V
(d) 2.14 V
Answer:
(a) 1.66 V

49. The standard reduction potentials of Sn, Hg and Cr are – 1.36 V, 0.854 V and – 0.746 V respectively. The increasing order of oxidising power of the given elements is
(a) Sn < Hg < Cr
(b) Hg < Cr < Sn
(c) Sn < Cr < Hg
(d) Cr < Hg < Sn
Answer:
(c) Sn < Cr < Hg

50. If standard reduction potentials for Pb, K, Zn and Cu are -0.126 V, -2.925 V, -0.763 V and 0.337 V, the decreasing order of reducing power is
(a) Zn > Pb > K > Cu
(b) Cu > Pb > Zn > K
(c) K > Zn > Pb > Cu
(d) K > Pb > Cu > Zn
Answer:
(c) K > Zn > Pb > Cu+

Balbharti Maharashtra State Board 11th Physics Important Questions Chapter 5 Gravitation Important Questions and Answers.

Maharashtra State Board 11th Physics Important Questions Chapter 5 Gravitation

Question 1.
Mention some main characteristics of gravitational force.
Answer:
Characteristics of gravitational force:

1. Every massive object in the universe experiences gravitational force.
2. It is the force of mutual attraction between any two objects by virtue of their masses.
3. It is always an attractive force with infinite range.
4. It does not depend upon the intervening medium.
5. It is much weaker than other fundamental forces. Gravitational force is 10 times weaker than strong nuclear force.

Question 2.
State and explain Kepler’s law of orbits.
Answer:
Statement:
All planets move in elliptical orbits around the Sun with the Sun at one of the foci of the ellipse.
Explanation:

1. The figure M shows the orbit of a around the planet P Sun.
   
2. Here, S and S’ are the foci of the ellipse and the Sun is situated at S.
3. P is the closest point along the orbit from S and is called perihelion.
4. A is the farthest point from S and is called aphelion.
5. PA is the major axis whose length is 2a. PO and AO are the semimajor axes with lengths ‘a’ each.
   MN is the minor axis whose length is 2b. MO and ON are the semiminor axes with lengths ‘b’ each.

Question 3.
State and prove Kepler’s law of equal areas.
Answer:
Statement:

The line that joins a planet and the Sun sweeps equal areas in equal intervals of time.
Explanation:
i)Kepler observed that planets move faster when they are nearer to the Sun while they move slower when they are farther from the Sun.

ii) Suppose the Sun is at the origin. The position of planet is denoted by \(\vec{r}\) and its momentum is denoted by \(\vec{p}\) (component ⊥ \(\vec{r}\)).

vi) For central force the angular momentum is conserved. Hence, from equations (4) and (5),
\(\frac{\overrightarrow{\Delta \mathrm{A}}}{\Delta \mathrm{t}}=\frac{\overrightarrow{\mathrm{L}}}{2 \mathrm{~m}}\) = constant
This proves the law of areas.

Question 4.
What is a central force?
Answer:
A central force on an object is a force which is always directed along the line joining the position of object and a fired point usually taken to the origin of the coordinate system.

Question 5.
State and explain Kepler’s law of periods.
Answer:
Statement:
The square of the time period of revolution of a planet around the Sun is proportional to the cube of the semimajor axis of the ellipse traced by the planet.
Explanation:
If r is length of semi major axis then, this law states that.
T² × r³ or \(\frac{\mathrm{T}^{2}}{\mathrm{r}^{3}}\) = constant

Solved Examples

Question 6.
What would be the average duration of year if the distance between the Sun and the Earth becomes
i) thrice the present distance.
ii) twice the present distance.
Solution:
i) Consider r₁ be the present distance between the Earth and Sun
We know, T₁ = 365 days.
When the distance is made thrice, r₂ = 3r₁
According to Kepler’s law of period,

i) The duration of the year would be 1897 days when distance is made thrice.
ii) The duration of the year would be 1032 days when distance is made twice.

Question 7.
What would have been the duration of the year if the distance between the Earth and the Sun were half the present distance?
Solution:
Given: r₂ = \(\frac{\mathrm{r}_{1}}{2}\)
∴ \(\frac{r_{2}}{r_{1}}=\frac{1}{2}\)
To find. Time period (T₂)
Formula: \(\left(\frac{T_{2}}{T_{1}}\right)^{2}=\left(\frac{r_{2}}{r_{1}}\right)^{3}\)
Calculation: We know. T₁ = 365 days
From formula,

= 365 × 0.3536
= 129.1
T₂ = 129.1 days
The duration of the year would be 129.1 days.

Question 8.
Calculate the period of revolution of Jupiter around the Sun. The ratio of the radius of Jupiter’s orbit to that of the Earth’s orbit is 5.
(Period of revolution of the Earth is 1 year.)
Solution:
Given: \(\frac{\mathrm{r}_{\mathrm{J}}}{\mathrm{r}_{\mathrm{E}}}=\frac{5}{\mathrm{l}}\), T_E = 1 year
To find: Period of revolution of Jupiter (T_J)
Formula: T² ∝ r³
Calculation: From formula,
\(\left(\frac{T_{J}}{T_{E}}\right)^{2}=\left(\frac{r_{J}}{r_{E}}\right)^{3}\)
∴ T_J = 5^3/2
= 5 x \(\sqrt {5}\)
= 11.18 years.
Period of revolution of Jupiter around the Sun is 11.18 years.

Question 9.
A Saturn sear is 29.5 times the Earth’s year. How far is the Saturn from the Sun if the Earth is 1.50 × 10⁸ km away from the Sun?
Solution:
Given: T_S = 29.5 T_E,
r_E = 1.50 × 10⁸ km
To find: Distance of Saturn form the Sun (r_S)
Formula: T² ∝ r³
Calculation: From formula,

∴ r_S = 14.32 × 10⁸ km
Saturn is 14.32 × 10⁸ km away from the Sun.

Question 10.
The distances of two planets from the Sun are 10¹³ m and 10¹² m respectively. Find the ratio of time periods of the two planets.
Solution:
Given: r₁ = 10¹³ m, r₂ = 10¹² m

Question 11.
Heavy and light objects are released from same height near the Earth’s surface. What can we conclude about their acceleration?
Answer:
Heavy and light objects, when released from the same height, fall towards the Earth at the same speed., i.e., they have the same acceleration.

Question 12.
Explain how Newton concluded that gravitational force F ∝ = \(\frac{\mathrm{Mm}}{\mathrm{r}^{2}}\)
Answer:
Before generalising and stating universal law of gravitation, Newton first studied the motion of moon around the Earth.

1. The known facts about the moon were,
   • the time period of revolution of moon around the Earth (T) = 27.3 days.
   • distance between the Earth and the moon (r) = 3.85 × 10⁵ km.
   • the moon revolves around the Earth in almost circular orbit with constant angular velocity ω.
2. Thus, the centripetal force experienced by moon (directed towards the centre of the Earth) is given by,
   F = mrω² …………… (1)
   Where, m = mass of the moon
3. From Newton’s laws of motion,
   F = ma
   ∴ a = rω² ……………… (2)
4. 
5. This is the acceleration of the moon directed towards the centre of the Earth.
6. This acceleration is much smaller than the acceleration felt by bodies near the surface of the Earth (while falling on Earth).
7. The value of acceleration due to Earth’s gravity at the surface is 9.8 m/s².
   
8. Newton therefore concluded that the acceleration of an object towards the Earth is inversely proportional to the square of distance of object from the centre of the Earth.
   ∴ a ∝ \(\frac{1}{r^{2}}\)
   x. As, F = ma
   Therefore, the force exerted by the Earth on an object of mass m at a distance r from it is
   F ∝ \(\frac{\mathrm{m}}{\mathrm{r}^{2}}\)
   Similarly, an object also exerts a force on the Earth which is
   F_E ∝ \(\frac{\mathrm{M}}{\mathrm{r}^{2}}\)
   Where M is the mass of the Earth. .
9. According to Newton’s third law of motion, F = F_E. Thus, F is also proportional to the mass of the Earth. From these observations, Newton concluded that the gravitational force between the Earth and an object of mass m is F ∝ \(\frac{\mathrm{Mm}}{\mathrm{r}^{2}}\)

Question 13.
Discuss the vector form of gravitational force between two masses with the help of diagram.
Answer:

1. Consider two point masses m₁ and m₂ having position vectors \(\overrightarrow{\mathrm{r}_{1}}\) and \(\overrightarrow{\mathrm{r}_{2}}\) from origin O respectively as shown in figure.
   
2. The position vector of mass m₂ with respect to m₁ is given by, \(\vec{r}_{2}-\vec{r}_{1}=\vec{r}_{21}\)
3. Similarly, position vector of mass m₁ with respect to m₂ is, \(\overrightarrow{\mathrm{r}}_{1}-\overrightarrow{\mathrm{r}_{2}}=\overrightarrow{\mathrm{r}_{12}}\)
4. Let \(\left|\overrightarrow{\mathrm{r}_{12}}\right|=\left|\overrightarrow{\mathrm{r}_{21}}\right|\) Then, the force acting on mass m₂ due to mass m₁ will be given as,
   \(\overrightarrow{\mathrm{F}_{21}}=\mathrm{G} \frac{\mathrm{m}_{1} \mathrm{~m}_{2}}{\mathrm{r}^{2}}\left(-\hat{\mathrm{r}}_{21}\right)\)
   where, \(\hat{\mathbf{r}}_{21}\) is the unit vector from m₁ to m₂.
   The force \(\overrightarrow{\mathrm{F}_{21}}\) is directed from m₂ to m₁.
5. Similarly, force experienced by m₁ due to m₂ is given as, \(\overrightarrow{\mathrm{F}}_{12}=\mathrm{G} \frac{\mathrm{m}_{1} \mathrm{~m}_{2}}{\mathrm{r}^{2}}\left(-\hat{\mathrm{r}}_{12}\right)\)
   ∴ \(\overrightarrow{\mathrm{F}}_{12}=-\overrightarrow{\mathrm{F}}_{21}\)
   [Note: As \(\hat{\mathbf{r}}_{21}\) is defined as unit vector from m₁ to m₂, conceptually force \(\overrightarrow{\mathrm{F}}_{21}\) is directed from m₂ lo m₁.]

Question 14.
Why Is the law of gravitation known as universal law of gravitation?
Answer:
The law of gravitation is applicable to all material objects in the universe. Hence it is known as the universal law of gravitation.

Question 15.
Give formula for the gravitational force due to a collection of masses and represent it diagrammatically.
Answer:

For a collection of point masses, the force on any one of them is the vector sum of the gravitational forces exerted by all the other point masses.
For n particles, force on i^th mass \(\overrightarrow{\mathrm{F}}_{\mathrm{i}}=\sum_{\mathrm{j}=1 \atop \mathrm{j} \neq \mathrm{i}}^{n} \overrightarrow{\mathrm{F}}_{\mathrm{ij}}\)
where, \(\vec{F}_{\mathrm{ij}}\) is the force on i^th particle due to j^th particle.

Question 16.
Discuss qualitative idea for the gravitational force of attraction due to a hollow, thin spherical shell of uniform density on a point mass situated inside it.
Answer:

1. Let us consider the case when the point mass A, is at the centre of the hollow thin shell.
2. In this case as every point on the shell is equidistant from A, all points exert force of equal magnitude on A but the directions of these forces are different.
3. Consider the forces on A due to two diametrically opposite points on the shell.
4. The forces on A due to them will be of equal magnitude but will be in opposite directions and will cancel each other.
5. Thus, forces due to all pairs of points diametrically opposite to each other will cancel and there will be no net force on A due to the shell.
6. When the point object is situated elsewhere inside the shell, the situation is not symmetric. However, gravitational force varies directly with mass and inversely with square of the distance.
7. When the point object is situated elsewhere inside the shell, the situation is not symmetric. However, gravitational force varies directly with mass and inversely with square of the distance.
8. Thus, some part of the shell may be closer to point A, but its mass is less. Remaining part will then have larger mass but its centre of mass is away from A.
9. In this way, mathematically it can be shown that the net gravitational force on A is still zero, so long as it is inside the shell.
10. Hence, the gravitational force at any point inside any hollow closed object of any shape is zero.

Question 17.
Discuss qualitative idea for the gravitational force of attraction between a hollow spherical shell or solid sphere of uniform density and a point mass situated outside the shell / sphere.
Answer:

• Gravitational force caused by different regions of shell can be resolved into components along the line joining the point mass to the centre and along a direction perpendicular to this line.
• The components perpendicular to this line cancel each other and the resultant force remains along the line joining the point to the centre.
• Mathematical calculations show that, this resultant force on this point equals the force that would get exerted by the shell whose entire mass is situated at its centre.

Solved Problems

Question 18.
The gravitational force between two bodies is 1 N. If distance between them is doubled, what will be the gravitational force between them?
Solution:
Let m₁ and m₂ be masses of the given two bodies. If they are r distance apart initially, then the force between them will be,

The force between two bodies reduces to 0.25 N.

Question 19.
Calculate the force of attraction between two metal spheres each of mass 90 kg, if the distance between their centres is 40 cm. (G = 6.67 × 10^-11 N m²/kg²)
Solution:
Given: m₁ = 90 kg, m₂ = 90 kg,
r = 40 cm = 40 × 10^-2 m.
G = 6.67 × 10^-11 N m²/kg²
To find: Force of attraction (F)
Formula: F = \(\frac{\mathrm{Gm}_{1} \mathrm{~m}_{2}}{\mathrm{r}^{2}}\)
Calculation.
From formula,
F = \(\frac{6.67 \times 10^{-11} \times 90 \times 90}{\left(40 \times 10^{-2}\right)^{2}}\)
= \(\frac{6.67 \times 8100}{1600} \times 10^{-7}\)
∴ F = 3.377 × 10^-6 N
The force of attraction between the two metal spheres is 3.377 × 10^-6 N.

Question 20.
Three particles A, B, and C each having mass m are kept along a straight line with AB = BC = 1. A fourth particle D is kept on the perpendicular bisector of AC at a distance ¡ from B. Determine the gravitational force on D.
Solution:

From figure,
distance of particle D, from particles A and C is.
ADCD = \(\sqrt{\mathrm{AB}^{2}+\mathrm{BD}^{2}}\)
= \(\sqrt{(l)^{2}+(l)^{2}}=l \sqrt{2}\)
Gravitational force on particle D is the vector sum of forces due to particles A, B, and C. Gravitational force due to A,
F_A = \(\frac{\mathrm{Gmm}}{(l \sqrt{2})^{2}}=\frac{\mathrm{Gm}^{2}}{2 l^{2}}\) = along \(\overrightarrow{\mathrm{DA}}\)
This force can he resolved into horizontal and vertical components using rectangular unit vectors as shown in figure.
From figure,


Negative sign indicates net force is acting along DB.
The net force acting on particle D is \(\frac{\mathbf{G m}^{2}}{l^{2}}\left(\frac{1}{\sqrt{2}}+1\right)\) directed along \(\overrightarrow{\mathbf{D B}}\).

[Note: When force, in given case is resolved into its components, its horizontal component contains cos argument while vertical component contains sine argument.]

Question 21.
Three balls of masses 5 kg each are kept at points P(1, 2, 0) Q(2, 3, 0) and R(2, 2, 0). Find the gravitational force exerted on the ball at point R.
Solution:
The net force acting on ball placed at R will be vector sum of forces acting due to balls at P and Q.

= 2.358 × 10^-9 N
The net force acting on the ball at point R will be 2.358 × 10^-9 N.

Question 22.
For what purpose Cavendish balance is used?
Answer:
Cavendish balance is used to find the magnitude of the gravitational constant G.

Question 23.
Describe the construction of Cavendish balance with the help of neat labelled diagram.
Answer:

1. The Cavendish balance consists of a light rigid rod. It is supported at the centre by a fine vertical metallic fibre about 100 cm long.
2. Two small spheres, s₁ and s₂ of lead having equal mass m and diameter about 5 cm are mounted at the ends of the rod and a small mirror M is fastened to the metallic fibre as shown in figure.
   
3. The mirror can be used to reflect a beam of light onto a scale and thereby measure the angel through which the wire will be twisted.
4. Two large lead spheres L₁ and L₂ of equal mass M and diameter of about 20 cm are kept close to the small spheres on opposite side as shown in figure.

Question 24.
Describe the working of the experiment performed to measure the value of gravitational constant.
Answer:

1. In the experiment performed to find the magnitude of gravitational constant (G), Cavendish balance is used.
2. The large spheres in the balance attract the nearby smaller spheres by equal and opposite force \(\overrightarrow{\mathrm{F}}\). Hence, a torque is generated without exerting any net force on the bar.
3. Due to the torque the bar turns and the suspension wire gets twisted till the restoring torque due to the elastic property of the wire becomes equal to the gravitational torque.
4. If r is the initial distance of separation between the centres of the large and the neighbouring small sphere, then the magnitude of the force between them is, F = G\(\frac{\mathrm{mM}}{\mathrm{r}^{2}}\)
5. If length of the rod is L, then the magnitude of the torque arising out of these forces is
   τ = FL = G\(\frac{\mathrm{mM}}{\mathrm{r}^{2}}\)L
6. At equilibrium, it is equal and opposite to the restoring torque.
   ∴ G\(\frac{\mathrm{mM}}{\mathrm{r}^{2}}\)L = Kθ
   Where, K is the restoring torque per unit angle and θ is the angle of twist.
7. By knowing the values of torque τ₁ it and corresponding angle of twist a, the restoring torque per unit twist can be determined as K = τ₁/α.
8. Thus, in actual experiment measuring θ and knowing values of τ, m, M and r, the value of G can be calculated from equation (2).

Question 25.
Derive the expression for the acceleration due to gravity on the surface of the Earth.
Answer:

1. The Earth is an extended object and can be assumed to be a uniform sphere.
2. If the mass of the Earth is M and that of any point object is m, the distance of the point object from the centre of the Earth is r then the force of attraction between them is given by,
   F = \(\text { G } \frac{M m}{r^{2}}\) …. (1)
3. If the point object is not acted upon by any other force, it will be accelerated towards the centre of the Earth under the action of this force. Its acceleration can be calculated by using Newton’s second law,
   F = ma … (2)
4. From equations (1) and (2),
   ma = \(\frac{\mathrm{GMm}}{\mathrm{r}^{2}}\)
   ∴ Acceleration due to the gravity of the Earth
   \(=\frac{\mathrm{GMm}}{\mathrm{r}^{2}} \times \frac{1}{\mathrm{~m}}=\frac{\mathrm{GM}}{\mathrm{r}^{2}}\)
   This is denoted by g.
5. If the object is close to the surface of the Earth, r ≈ R, then,
   g_{Earth’s surface} = \(\frac{\mathrm{GM}}{\mathrm{R}^{2}}\)

Question 26.
Explain why the Earth doesn’t appear to move even though the object of mass m (m << M) kept on the Earth exerts equal and opposite gravitational force on it.
Answer:

1. An object of mass m (much smaller than the mass of the Earth) is attracted towards the Earth and falls on it.
2. At the same time, the Earth is also attracted by the equal and opposite force towards the mass m.
3. However, its acceleration towards m will be,
   
4. As m << M, a_Earth << g and is nearly zero. As, a result, practically only the mass m moves towards the Earth and the Earth doesn’t appear to move.

Solved Examples

Question 27.
Calculate mass of the Earth from given data, Acceleration due to gravity g = 9.81 m/s², Radius of the Earth R_E = 6.37 × 10⁶ m, G = 6.67 × 10^-11 N m²/kg²
Solution:
Given: g = 9.81 m/s², R_E = 6.37 × 10⁶ m,
G = 6.67 × 10^-11 N m²/kg²
To find: Mass of the Earth (M_E)
Formula: g = \(\frac{\mathrm{GM}_{\mathrm{E}}}{\mathrm{R}_{\mathrm{E}}^{2}}\)
Calculation: From formula,

= antilog {0.9912 + 0.8041 + 0.8041 – 0.8241) × 10²³
= antilog {1.7753} × 10²³
= 59.61 × 10²³
= 5.961 × 10²⁴ kg
Mass of the Earth is 5.961 × 10²⁴ kg.

Question 28.
Calculate the acceleration due to gravity at the surface of the Earth from the given data. (Mass of the Earth = 6 × 10²⁴ kg, Radius of the Earth = 6.4 × 10⁶ m, G = 6.67 × 10^-11 N m²/kg²)
Solution:
Given. M = 6 × 10²⁴ kg, R = 6.4 × 10⁶ m, G = 6.67 × 10^-11 N m²/kg²
Tofind. Acceleration due to gravity (g)
Formula: g = \(\frac{\mathrm{GM}}{\mathrm{R}^{2}}\)
Calculation: From formula,

The acceleration due to gravity at the surface of the Earth is 9.77 m/s².

Question 29.
Calculate the acceleration due to gravity on the surface of moon if mass of the moon is 1/80 times that of the Earth and diameter of the moon is 1/4 times that of the Earth (g = 9.8 m/s²)
Solution:
Given: M_m = \(\frac{\mathrm{M}_{\mathrm{E}}}{80}\), R_m = \(\frac{\mathrm{R}_{\mathrm{E}}}{4}\), g = 9.8 m/s²
To find: Acceleration due to gravity on the surface of moon (g_m)
Formula: g = \(\frac{\mathrm{GM}}{\mathrm{R}^{2}}\)
Calculation: For moon, from formula,

Acceleration due to gravity on surface of the planet is 0.245 m/s².

Question 30.
Find the acceleration due to gravity on a planet that is 10 times as massive as the Earth and with radius 20 times of the radius of the Earth (g = 9.8 m/s²).
Solution:
Given: M_P = 10 × Mass of the Earth = 10 M_E,
R_P = 20 × radius of the Earth = 20 R_E, g = 9.8 m/s²
To find: Acceleration due to gravity on surface of the planet (g_P)
Formula: g = \(\frac{\mathrm{GM}}{\mathrm{R}^{2}}\)
Calculation: From formula,

Acceleration due to gravity on surface of the planet is 0.245 m/s².

Question 31.
Acceleration due to gravity on the Earth is g. A planet has mass and radius half that of the Earth. How much will be percentage change in the acceleration due to gravity on the planet?
Solution:

The percentage change in acceleration due to gravity between the planet and the Earth will be 100%.

Question 32.
Explain the graph showing variation of acceleration due to gravity with altitude and depth.
Answer:
The value of acceleration due to gravity is calculated to be maximum at the surface of the Earth. The value goes on decreasing with
i) increase in depth below the Earth’s surface. [varies linearly with (R – d) = r]

ii) increase in height above the Earth’s surface. [varies inversely with (R + h)² = r²].

Graph of g, as a function of r, the distance from the centre of the Earth, is plotted as shown in figure.
For r< R,
g_d = g\(\left(1-\frac{\mathrm{d}}{\mathrm{R}}\right)\)
if r = R – d,
g(r) = g\(\left(\frac{r}{R}\right) \Rightarrow g(r) \propto r\)
Hence, the graph shows a straight line passing through origin and having slope \(\frac{\mathrm{g}}{\mathrm{R}}\).

which is represented in the graph.

Question 33.
Why does the weight of a body of a finite mass m is zero at the centre of the Earth?
Answer:
Acceleration at depth d due to gravity is given
by,
g_d = g\(\left(1-\frac{\mathrm{d}}{\mathrm{R}}\right)\)
As, at centre of the Earth d = R ⇒ g_d = 0.
Hence, the weight of a body of a finite mass m is zero at the centre of the Earth.

Question 34.
Discuss the variation of acceleration due to gravity at poles and equator due to latitude of the Earth.
Answer:

1. Effective acceleration due to gravity at P is given as,
   g’ = g – Rω²cos²θ.
2. As the value of θ increases, cos θ decreases. Therefore g’ will increase as we move away from equator towards any pole due to the rotation of the Earth.
3. At equator θ = 0°
   ∴ cos θ = 1
   ∴ g’_e = g – Rω²
   The effective acceleration due to gravity (g’_e) is minimum at equator, as here it is reduced by Rω²
4. At poles θ = 90° cos θ = 0
   ∴ g’_p = g – Rω² cos θ
   = g – 0
   = g
   There is no reduction in acceleration due to gravity at poles, due to the rotation of the Earth as the poles are lying on the axis of rotation and do not revolve.

Question 35.
If g = 9.8 m/s² on the surface of the Earth, find its value at h = \(\frac{\mathbf{R}}{\mathbf{2}}\) from the surface of the Earth.
Solution:
Given: g = 9.8 m/s², h = \(\frac{\mathrm{R}}{2}\)
To find: Acceleration due to gravity (g_h)
Formula: \(\frac{\mathrm{g}_{\mathrm{h}}}{\mathrm{g}}\) = \(\frac{\mathrm{R}^{2}}{(\mathrm{R}+\mathrm{h})^{2}}\)
Calculation:
From formula,

At h = \(\frac{\mathrm{R}}{2}\) from the surface of the Earth, the value of g is 4.35 m/s².

Question 36.
At what distance above the surface of Earth the acceleration due to gravity decreases by 10% of its value at the surface? (Radius of Earth = 6400 km)
Solution:
Given: g_h = 90% of g i.e., \(\frac{g_{h}}{g}\) = 0.9,
R = 6400 km = 6.4 × 10⁶ m
To find: Distance above the surface of the Earth (h)

Question 37.
Find the altitude at which the acceleration due to gravity is 25% of that at the surface of the Earth.
(Radius of the Earth = 6400 km)
Solution:
Given: g_h = 25% of g = \(\frac{25}{100} \times \mathrm{g}=\frac{\mathrm{g}}{4}\), R = 6400 km = 6.4 × 10⁶ m
To find: Height (h)
Formula: g_h = g\(\left(\frac{R}{R+h}\right)^{2}\)
Calculation: From formula,
\(\frac{\mathrm{g}}{4}\) = g\(\left(\frac{R}{R+h}\right)^{2}\)
(R + h)² = 4R²
R + h = 2R
∴ h = 2R – R
∴ h = R
∴ h = 6400 km

Question 38.
A hole is drilled half way to the centre of the Earth. A body is dropped into the hole. How much will it weigh at the bottom of the hole if the weight of the body on the Earth’s surface is 350 N?
Solution:
Given: W = mg = 350 N, d = \(\frac{\mathrm{R}}{2}\)
To find: Weight at certain depth (W_d)
Formula: g_d = \(\mathrm{g}\left[1-\frac{\mathrm{d}}{\mathrm{R}}\right]\)
Calculation: Since W_d = mg_d,
from formula,

Question 39.
Assuming the Earth to be a homogeneous sphere, determine the density of the Earth from following data. (g = 9.8 m/s², G = 6.673 × 10^-11 N m²/kg², R = 6400 km)
Solution:
Given g = 9.8 m/s²,
G = 6.673 × 10^-11 N m²/kg², R = 6400 km = 6.4 × 10⁶ m
To find: Density (ρ)
Formula: g = \(\frac{4}{3} \pi \mathrm{R} \rho \mathrm{G}\)
Calculation: From formula,

Question 40.
If the Earth were a perfect sphere of radius 6.4 × 10⁶ m rotating about its axis with the period of one day (8.64 × 10⁴ s), what is the difference in acceleration due to gravity from poles to equator?
Solution:
Given: R = 6.4 × 10⁶ m, T = 8.64 × 10⁴ s
To find: Difference in acceleration due to gravity (g_P – g_E)
Formula: g’ = g – Rω² cos²θ
Calculation: Since ω = \(\frac{2 \pi}{\mathrm{T}}\)
∴ ω = \(\frac{2 \times 3.14}{8.64 \times 10^{4}}\) = \(\frac{6.28}{8.64 \times 10^{4}}\)
= 0.7268 × 10^-4
ω = 7.268 × 10^-5 rad/s
At poles, θ = 90°
From formula,
g_P = g – Rω²cos² (90°)
= g – 0 ….(∵ cos 90° = 0)
∴ g_P = g …. (i)
At equator, θ = 0°,
∴ g_E = g – Rω²cos² 0°
g_E = g – Rω² …. (ii)
Subtracting equation (ii) from equation (i), we have,
g_P – g_E = g – (g – Rω²)
∴ g_P – g_E = Rω²
= 6.4 × 10⁶ × (7.268 × 10^-5)²
= 6.4 × 10⁶ × 52.82 × 10^-10
= 338 × 10^-4
∴ g_P – g_E = 3.38 × 10^-2 m/s²

Question 41.
The Earth is rotating with angular velocity ω about its own axis. R is the radius of the Earth. If Rω² = 0.03386 m/s², calculate the weight of a body of mass 100 gram at latitude 25°. (g = 9.8 m/s²)
Solution:
Given: Rω² = 0.03386 m/s², θ = 25°,
m = 0.1 kg, g = 9.8 m/s²
To find: Weight (W)

Formulae:
i) g’ = g – Rω² cos² θ
ii) W = mg

Calculation:
From formula (i),
g’ = 9.8 – [0.03386 – cos² (25°)]
∴ g’ = 9.8 – [0.03386 × (0.9063)²]
∴ g’ = 9.8 – 0.02781
∴ g’ = 9.772 m/s²
From formula (ii),
W = 0.1 × 9.772
∴ W = 0.9772 N

Question 42.
If the angular speed of the Earth is 7.26 × 10^-5 rad/s and radius of the Earth is 6,400 km, calculate the change in weight of 1 kg of mass taken from equator to pole.
Solution:
Given: R = 6.4 × 10⁶ m, ω = 7.26 × 10^-5 rad/s
To find: Change in weight (∆W)
Formulae:
i) ∆g = g_p – g_eq = Rω²
ii) ∆W = m∆g

Calculation: From formula (i) and (ii),
∆W = m(Rω²)
= 1 × 6.4 × 10⁶ × (7.26 × 10^-5)²
= 3373 × 10^-5 N

Question 43.
Define potential energy.
Answer:
Potential energy is the work done against conservative force (or forces) in achieving a certain position or configuration of a given system.

Question 44.
Explain with examples the universal law which states that “Every system always configures itself in order to have minimum potential energy or every system tries to minimize its potential energy”.
Answer:
Example 1:

1. A spring in its natural state, possesses minimum potential energy. Whenever we stretch it or compress it, we perform work against the conservative force.
2. Due to this work, the relative distances between the particles of the system change i.e., configuration changes and potential energy of the spring increases.
3. The spring finally regains its original configuration of minimum potential energy on removal of the applied force.
   This explains that the spring always try to rearrange itself in order to attain minimum potential energy.

Example 2:

1. When an object is lying on the surface of the Earth, the system of that object and the Earth has minimum potential energy.
2. This is the gravitational potential energy of the system as these two are bound by the gravitational force. While lifting the object to some height, we do work against the conservative gravitational force.
3. In its new position, the object is at rest due to balanced forces. However, now, the object has a capacity to acquire kinetic energy, when allowed to fall.
4. This increase in the capacity is the potential energy gained by the system. The object falls on the Earth to achieve the configuration of minimum potential energy on dropping it from the new position.

Question 45.
Obtain an expression for change in gravitational potential energy of any object displaced from one point to another.
Answer:
i) Work done against gravitational force in displacing an object through a small displacement, stored in the system in the form of increased potential energy of the system.
∴ dU = –\(\overrightarrow{\mathrm{F}}_{\mathrm{g}} \cdot \overrightarrow{\mathrm{dr}}\)
Negative sign appears because dU is the work done against the gravitational force \(\overrightarrow{\mathrm{F}_{\mathrm{g}}}\).

ii) For displacement of the object from an initial position \(\overrightarrow{\mathrm{r}_{\mathrm{i}}}\) to the final position \(\overrightarrow{\mathrm{r}_{\mathrm{f}}}\), the change in potential energy ∆U, can be obtained by integrating dU.

 

iii) Gravitational force of the Earth, \(\overrightarrow{\mathrm{F}}_{\mathrm{g}}\) = –\(\frac{\mathrm{GMm}}{\mathrm{r}^{2}} \hat{\mathrm{r}}\) where \(\hat{r}\) is the unit vector in the direction of \(\overrightarrow{\mathrm{r}}\).
Negative sign appears here because \(\overrightarrow{\mathrm{F}_{\mathrm{g}}}\) is directed towards centre of the Earth and opposite to \(\overrightarrow{\mathrm{r}}\).

iv) For Earth and mass system,

Hence, change in potential energy corresponds to the work done against conservative forces.

Question 46.
Using expression for change in potential energy, show that gravitational potential energy of the system of object of mass m and the Earth with separation of r is, –\(\frac{\text { GMm }}{\text { r }}\)
Answer:

1. Change in P.E. for a system of Earth and mass is given by,
   ∆U = GMm\(\left(\frac{1}{r_{i}}-\frac{1}{r_{f}}\right)\)
2. For gravitational force, point of zero potential energy is taken to be at r = ∞.
3. Hence, U(r_i) = 0 at r_i = ∞
   Final point r_f is the point where the potential energy of the system is to be determined.
4. At r_f = r
   
   This is gravitational potential energy of the system of object of mass m and Earth of mass M having separation r (between their centres of mass).

Question 47.
Derive the formula for increase in gravitational potential energy of a Earth – mass system when the mass is lifted to a height h provided h << R.
Answer:

1. If the object is on the surface of Earth, r = R
   U₁ = –\(\frac{\mathrm{GMm}}{\mathrm{R}}\)
   If the object is lifted to height h above the surface of Earth, the potential energy becomes _ GMm 12 ~~ R+h
   U₂ = –\(\frac{G M m}{R+h}\)
2. Increase in the potential energy is given by
   
3. If g is acceleration due to gravity on the surface of Earth. GM = gR²
   ∴ ∆U = mgh\(\left(\frac{R}{R+h}\right)\) … (1)
4. Equation (1) gives the work to be done to raise an object of mass rn to a height h, above the surface of the Earth.
5. If h << R, we can use R + h ≈ R.
   ∴ ∆U = mgh
   Thus, mgh is increase in the gravitational potential energy of the Earth – mass system if an object of mass m is lifted to a height h, provided h << R.

Question 48.
Write a short note on gravitational potential.
Answer:
The gravitational potential energy of the system of Earth and any mass m at a distance r from the centre of the Earth is given by,

The factor –\(\frac{\mathrm{GM}}{\mathrm{r}}\) = (V_E)_r is defined as the
gravitational potential of Earth at distance r from its centre.

As the potential depends only upon mass of the Earth and location of the object, it is same for any mass m bound to the Earth.

Question 49.
Explain the relation between the gravitational potential energy and the gravitational potential.
Answer:

1. In terms of potential, we can write the potential energy of the Earth-mass system as, Gravitational potential energy (U) = Gravitational potential (V_r) × mass (m)
2. Thus, gravitational potential is gravitational potential energy per unit mass.
   ∴ V_r = \(\frac{\mathrm{U}}{\mathrm{m}}\)
3. For any conservative force field, the concept of potential can be defined on similar lines.
4. Gravitational potential difference between any two points in gravitational field can be written as,
   V₂ – V₁ = \(\frac{U_{2}-U_{1}}{m}\)
   = \(\frac{\mathrm{dW}}{\mathrm{m}}\)
   This is the work done (or change in potential energy) per unit mass.
5. Therefore, in general, for a system of any two masses m₁ and m₂, separated by distance r, we can write,
   U = –\(\frac{\mathrm{G} \mathrm{m}_{1} \mathrm{~m}_{2}}{\mathrm{r}}\)
   = (V₁)m₂
   = (V₂)m₁
   Here V₁ and V₂ are gravitational potentials at r due to m₁ and m₂ respectively.

Solved Exmaples

Question 50.
What will be the change in potential energy of a body of mass m when it is raised from height R_E above the Earth’s surface to 5/2 R_E above the Earth’s surface? R_E and M_E are the radius and mass of the Earth respectively.
Solution:
Change in potential energy (P.E.) of a body of mass m is given by,
∆U = GM_Em\(\left(\frac{1}{r_{i}}-\frac{1}{r_{f}}\right)\)
Here, r_i = R_E + R_E = 2 R_E

[Note: Answer calculated above is in accordance with textual methods of calculation.]

Question 51.
What will be the change in potential energy of a body of mass m when it is placed on the surface of the Earth from height R above the Earth’s surface?
Solution:
Change in potential energy (P.E.) of a body of mass m is given by,
∆U = GM_Em\(\frac{1}{r_{i}}-\frac{1}{r_{r}}\)
Here, r_i = R + R = 2R
Similarly, r_f = R
∴ ∆U = GM_Em \(\left[\frac{1}{2 R}-\frac{1}{R}\right]\) = GM_Em\(\left(-\frac{1}{2 R}\right)\)
∴ ∆U = –\(\frac{\mathbf{G} \mathbf{M} \mathbf{m}}{\mathbf{2 R}}\)
Negative sign indicates that potential energy is decreasing.

Question 52.
Determine the gravitational potential of a body of mass 80 kg whose gravitational potential energy is 5 × 10⁹ J on the surface of the Earth.
Solution:
Given: m = 80 kg, U = 5 × 10⁹ J
To find: Gravitational potential (V)
Formula: V = \(\frac{\mathrm{U}}{\mathrm{m}}\)
Calculation: From formula,
V = \(\frac{5 \times 10^{9}}{80}=\frac{25}{4}\) × 10⁷
= 6.25 × 10⁷ J kg^-1
Potential of the body at the surface of the Earth is 6.25 × 10⁷ J kg^-1.

Question 53.
Calculate the escape velocity of a body from the surface of the Earth.
(Average density of Earth = 5.5 × 10³ kg/m³, G = 6.67 × 10^-11 N m²/kg², radius of Earth R = 6.4 × 10⁶ m)
Solution:
Given: ρ = 5.5 × 10³ kg/m³, R = 6.4 × 10⁶ m,
G = 6.67 × 10^-11 N m²/kg²
To find: Escape velocity (v_e)

= 2 × 6.4 × 10⁶ × 8.759 × 10^-4
∴ v_e = 11.21 × 10³ m/s = 11.21 km/s
The escape velocity of a body is 11.21 km/s.

Question 54.
What is a satellite?
Answer:
An object which revolves in an orbit around a planet is called as satellite.
Example:

• Moon is a natural satellite of the Earth.
• INSAT is an artificial satellite of the Earth.

Question 55.
Write a short note on Polar satellites.
Answer:

1. Polar Satellites are placed in lower polar orbits.
2. They are at low altitude 500 km to 800 km.
3. Period of revolution of polar satellite is nearly 85 minutes, so it can orbit the Earth 16 time per day.
4. They go around the poles of the Earth in a north-south direction while the Earth rotates in an east-west direction about its own axis.
5. The polar satellites have cameras fixed on them. The camera can view small stipes of the Earth in one orbit. In entire day the whole Earth can be viewed strip by strip.
6. Polar region and equatorial regions close to it can be viewed by these satellites.
7. Polar satellites are used for weather forecasting and meteorological purpose. They are also used for astronomical observations and study of Solar radiations.

Question 56.
Derive the expression for the critical velocity of a satellite revolving close to the surface of the Earth in terms of acceleration due to gravity.
Answer:

1. When the satellite is revolving close to the surface of the Earth, the height is very small as compared to the radius of the Earth.
2. Hence the height can be neglected and radius of the orbit is nearly equal to R, i.e., R + h ≈ R
3. The critical speed of the satellite then becomes,
   v_c = \(\sqrt{\frac{G M}{R}}\)
4. G is related to acceleration due to gravity by the relation,
   g = \(\frac{G M}{R^{2}}\)
   ∴ GM = gR²
5. Thus, critical speed in terms of acceleration due to gravity, neglecting the air resistance, can be obtained as,
   v_c = \(\sqrt{\frac{\mathrm{gR}^{2}}{\mathrm{R}}}=\sqrt{\mathrm{gR}}\)

Question 57.
From an inertial frame of reference, explain the apparent weight for a person standing in a lift having zero acceleration.
Answer:

1. A passenger inside a lift experiences only two forces:
   • Gravitational force mg directed vertically downwards and
   • normal reaction force N directed vertically upwards, exerted by the floor of the lift.
2. As these forces are oppositely directed, the net force in the downward direction will be F = ma – N.
3. Though the weight of a passenger is the gravitational force acting upon it, the person experiences his weight only due to the normal reaction force N exerted by the floor.
4. A lift has zero acceleration when the lift is at rest or is moving upwards or downwards with constant velocity.
5. Thus, a net force acting on the passenger inside the lift will be,
   F = 0 = mg – N
   ∴ mg = N
   Hence, in this case the passenger feels his normal weight mg.

Question 58.
What happens to the apparent weight of the person inside the lift moving with net upward acceleration?
Answer:

1. The lift is said to be moving with net upward acceleration in two possible conditions:
   • when the lift just starts moving upwards or
   • is about to stop at a lower floor during its downward motion.
2. As the net acceleration is upwards, the upward force must be greater.
   ∴ F = ma = N – mg
   ∴ N = mg + ma
   ∴ N > mg
3. Thus, for a passenger inside this lift, his apparent weight is more than his actual weight when the lift was not accelerated.

Question 59.
Why does a passenger feel lighter when the lift is about to stop at a higher floor during its upward motion?
Answer:

1. When the lift is about to stop at a higher floor during its upward motion it has a net downward acceleration.
2. As the net acceleration is downwards, the downward force must be greater.
   ∴ F = ma = mg – N
   ∴ N = mg – ma
   i.e., N < mg
   Hence, a passenger feels lighter when the lift is about to stop at a higher floor during its upward motion.

Question 60.
When does a weighing machine will record zero for a passenger in a lift?
Answer:
If the cables of the lift are cut, the downward acceleration of the lift, a_d = g. In this case, we get,
N = mg – ma_d = 0
Thus, there will not be any feeling of weight and the weighing machine will record zero.

Question 61.
Define time period of a satellite.
Obtain an expression for the period of a satellite in a circular orbit round the Earth. Show that the square of the period of revolution of a satellite is directly proportional to the cube of the orbital radius.
Answer:
Definition:
The time taken by a satellite to complete one revolution around the Earth is called its time period.
Expression for time period:
i) Consider, m = mass of satellite, h = altitude of satellite. Thus, r = R + h = radius of orbit of the satellite.
ii) In one revolution, distance traced by satellite is equal to circumference of its circular orbit.
iii) If T is the time period of satellite, then

Since π², G and M are constant,
∴ T² ∝ r³
Hence, square of the period of revolution of a satellite is directly proportional to the cube of the radius of its orbit.

vi) Taking square roots on both the sides of equation (4), we get,
T = 2π\(\sqrt{\frac{\mathrm{r}^{3}}{\mathrm{GM}}}\)
T = 2π\(\sqrt{\frac{(R+h)^{3}}{G M}}\)
This is the required expression for period of satellite orbiting around the Earth in circular path.

Question 62.
For an orbiting satellite very close to surface of the Earth, show that T = 2π \(\sqrt{\frac{\mathrm{R}}{\mathrm{g}}}\).
Answer:

1. Time period of an orbiting satellite at certain height is given by, T = 2π \(\sqrt{\frac{(R+h)^{3}}{G M}}\)
2. If satellite is orbiting very close to the Earth’s surface, then h ≈ 0
   

Solved Examples

Question 63.
Show that the critical velocity of a body revolving in a circular orbit very close to the surface of a planet of radius R and
mean density ρ is 2R\(\sqrt{\frac{G \pi \rho}{3}}\).
Solution:
Since the body is revolving very close to the surface of a planet,
∴ h << R
R = radius of planet
ρ = mean density of planet
Critical velocity of a body very close to Earth is given by,

Question 64.
Find the orbital speed of the satellite w hen satellite is revolving round the Earth in circular orbit at a distance 9 × 10⁶ m from its centre. (Given: Mass of Earth = 6 × 10²⁴ kg, G = 6.67 × 10^-11 N m²/kg²)
Solution:
Given: r = 9 × 10⁶ m, M = 6 × 10²⁴kg,
G = 6.67 × 10^-11 N m²/kg²
To find: Orbital speed (v_c)
Formula: v_c = \(\sqrt{\frac{\mathrm{GM}}{\mathrm{r}}}\)
Calculation: From formula,

∴ v_c = 6.668 × 10³ m/s
The orbital speed of the satellite is 6.668 × 103 m/s.

Question 65.
Taking radius of the Earth as 6400 km and g at the Earth’s surface as 9.8 m/s², calculate the speed of revolution of a satellite orbiting close to the Earth’s surface.
Solution:
Given: R = 6400 km = 6.4 × 10⁶ m, g = 9.8 m/s²
To find: Critical velocity (v_c)
Formula: v_c = \(\sqrt{\mathrm{gR}}\)
Calculation: From formula,
v_c = \(\sqrt{9.8 \times 6.4 \times 10^{6}}\)
= \(\sqrt{98 \times 64 \times 10^{4}}\)
= 7\(\sqrt{2}\) × 8 ×10²
= 7.92 × 10³ m/s
The speed of revolution of the satellite orbiting close to the Earth’s surface is 7.92 × 10³ m/s.

Question 66.
The critical velocity of a satellite revolving around the Earth is 10 km/s at a height where g_h = 8 m/s ². Calculate the height of the satellite from the surface of the Earth. (R = 6.4 × 10⁶ m)
Solution:
Given: v_c = 10 km/s = 10 × 10³ m/s,
g_h = 8 m/s³, R = 6.4 × 10⁶ m
To find: Height of the satellite (h)
Formula: v_c = \(\sqrt{g_{\mathrm{h}}(R+h)}\)
Calculation: From formula,
10 × 10³ = \(\sqrt{8 \times(\mathrm{R}+\mathrm{h})}\)
Squaring both the sides, we get,
100 × 10⁶ = 8(R + h)
∴ 8(R + h) = 100 × 10⁶
∴ R + h = \(\frac{100}{8}\) × 10⁶
∴ h = 12.5 × 10⁶ – R
= 12.5 × 10⁶ – 6.4 × 10⁶
= 6.1 × 10⁶m
∴ h = 6100 km
The height of the satellite from the surface of the Earth is 6100 km.

Question 67.
An artificial satellite revolves around a planet in circular orbit close to its surface. Obtain the formula for period of the satellite in terms of density p and radius R of planet.
Solution:
Time period of a satellite revolving around the planet at certain height is given by,

Question 68.
Calculate the period of revolution of a polar satellite orbiting close to the surface of the Earth. Given R = 6400 km, g = 9.8 m/s².
Solution:
Given: For satellite close to Earth surface,
R + h ≈ R
R = 6400 km = 6.4 × 10⁶ m, g = 9.8 m/s²
To find: Time period of satellite (T)
Formula: T = 2π \(\sqrt{\frac{R}{g}}\)
Calculation: From Formula,
T = 2 × 3.14 × \(\sqrt{\frac{6.4 \times 10^{6}}{9.8}}\)
= 6.28 × 8.081 × 10²
= 5.075 × 10³ sec
≈ 85 min
The time period of satellite very close to the Earth’s surface is nearly 85 minute.

Question 69.
A satellite orbits around the Earth at a height equal to R of the Earth. Find its period. (R = 6.4 × 10⁶ m, g = 9.8 m/s²)
Solution:
Given: h = R = 6.4 × 10⁶m, g = 9.8 m/s²
To find: Time period (T)

The time period of the satellite is 1.435 × 10⁴ s.

Question 70.
Calculate the height of the communication satellite. (Given: G = 6.67 × 10^-11 N m²/kg², M = 6 × 10²⁴ kg, R = 6400 km)
Solution:
For communication satellite, T = 24 × 60 × 60 s,
Given: M = 6 × 10²⁴ kg,
G = 6.67 × 10^-11 N m²/kg²,
R = 6400 km = 6.4 × 10⁶m
To find: Height (h)

The height of the communication satellite is 35910 km.

Question 71.
How will you ‘weigh the Sun’, that is estimate its mass? The mean orbital radius of the Earth around the Sun is 1.5 × 10⁸ km.
Solution:
Given: r = 1.5 × 10⁸ × 10³m,
T = 365 days = 365 × 24 × 60 × 60 s
To find: Mass (M)
Formula: T = 2π \(\sqrt{\frac{r^{3}}{G M}}\)
Calculation:
From formula,
M = \(\frac{4 \pi^{2} r^{3}}{G T^{2}}\)
= \(\frac{4 \times(3.14)^{2}\left(1.5 \times 10^{11}\right)^{3}}{\left(6.67 \times 10^{-11}\right)(365 \times 24 \times 60 \times 60)^{2}}\)
∴ M = 2.01 × 10³⁰kg
The mass of the Sun is 2.01 × 10³⁰ kg.
[Trick: To ‘weigh the Sun’, i.e., estimate its mass, one needs to know the period of one of its planets and the radius of the planetary orbit.]

Question 72.
Calculate the B.E. of a satellite of mass 2000 kg moving in an orbit very close to the surface of the Earth. (G = 6.67 × 10^-11 N m²/kg²)
Solution:
Given: m = 2 × 10³ kg, R = 6.4 × 10⁶ m,
R = 6.4 × 10⁶ m, M = 6 × 10²⁴kg,
G = 6.67 × 10^-11 N m²/kg²,
M = 6 × 10²⁴ kg
To find: Binding Energy (B.E.)
Formula: For satellite very close to Earth,
B.E. = \(\frac{1}{2} \times \frac{\mathrm{GMm}}{\mathrm{R}}\)
Calculation: From formula,
B.E. = \(\frac{1}{2} \times \frac{6.67 \times 10^{-11} \times 6 \times 10^{24} \times 2 \times 10^{3}}{6.4 \times 10^{6}}\)
∴ B.E. = 6.25 × 10¹⁰ joule
The binding energy of the satellite is 6.25 × 10¹⁰ joule.

Question 73.
Find the binding energy of a body of mass 50 kg at rest on the surface of the Earth. (Given: G = 6.67 × 10^-11 N m²/kg², R = 6400 km, M = 6 × 10²⁴ kg)
Solution:
Given: G = 6.67 × 10^-11 N m²/kg²,
R = 6400 km = 6.4 × 10⁶m,
M = 6 × 10²⁴ kg, m = 50 kg
To find: Binding energy (B.E.)
Formula: B.E. = \(\frac{\text { GMm }}{\mathrm{R}}\)
Calculation: From formula,
B.E. = \(\frac{6.67 \times 10^{-11} \times 6 \times 10^{24} \times 50}{6.4 \times 10^{6}}\)
= \(\frac{2001}{6.4}\) × 10⁷
∴ B.E. = 3.127 × 10⁹ J
The binding energy of the body 3.127 × 10⁹ J.

Question 74.
Find the total energy and binding energy of an artificial satellite of mass 1000 kg orbiting at height of 1600 km above the Earth’s surface.
(Given: G = 6.67 × 10^-11 N m²/kg², R = 6400 km, M = 6 × 10²⁴ kg)
Solution:
Given: h = 1600 km = 1.6 × 10⁶ m,
G = 6.67 × 10^-11 N m²/kg²,
R = 6400 km = 6.4 × 10⁶m,
m = 1000 kg, M = 6 × 10²⁴kg
To find:
i) Total Energy (T.E.)
ii) Binding Energy (B.E.)
Formulae: i. T.E. = –\(\frac{\mathrm{GMm}}{2(\mathrm{R}+\mathrm{h})}\)
ii. B.E. = -T.E.

Calculation: From formula (i),
T.E = – \(\frac{6.67 \times 10^{-11} \times 6 \times 10^{24} \times 1000}{2(6.4+1.6) \times 10^{6}}\)
= –\(\frac{40020 \times 10^{7}}{2 \times 8}\)
∴ T.E. = -2.501 × 10¹⁰J
From formula (ii),
B.E. = 2.501 × 10¹⁰ J
i) The total energy of the artificial satellite is -2.501 × 10¹⁰ J.
ii) The binding energy of the artificial satellite is 2.501 × 10¹⁰ J.

Question 75.
Determine the binding energy of satellite of mass 1000 kg revolving in a circular orbit around the Earth when it is close to the surface of Earth. Hence find kinetic energy and potential energy of the satellite. (Mass of Earth = 6 × 10²⁴ kg, radius of Earth = 6400 km; gravitational constant G = 6.67 × 10^-11 N m²/kg²)
Solution:
Given: m = 1000 kg, M = 6 × 10²⁴ kg,
R = 6400 km, G = 6.67 × 10^-11 N m²/kg²
To find:
i) Binding Energy (B.E.)
ii)Kinetic Energy (K.E.)
iii) Potential Energy (P.E.)

Formulae: For satellite very close to Earth,
i) B.E. = \(\frac{1}{2} \times \frac{\mathrm{GMm}}{\mathrm{R}}\)
ii) K.E.= B.E.
iii) P.E. = -2 K.E.

Calculation: From formula (i),
B.E. = \(\frac{6.67 \times 10^{-11} \times 6 \times 10^{24} \times 1000}{2 \times 6.4 \times 10^{6}}\)
∴ B.E. = 3.1265 × 10¹⁰ J
From formula (ii),
K.E. = 3.1265 × 10¹⁰
∴ K.E. = 3.1265 × 10¹⁰ J
From formula (iii),
P.E. = -2(3.1265 × 10¹⁰)
∴ P.E. = -6.2530 × l0¹⁰J
i) The binding energy of the satellite is 3.1265 × 10¹⁰ J.
ii) The kinetic energy of the satellite is 3.1265 × 10¹⁰ J.
iii) The potential energy of the satellite is -6.2530 × 10¹⁰J.

Apply Your Knowledge

Question 76.
How are Kepler’s law of periods and Newton’s law of gravitation related?
Answer:
Consider a planet of mass m revolving around the Sun of mass M in a circular orbit.
Let,
r = radius of the circular orbit of the planet.
T = Time period of revolution of planet around the Sun.
ω = angular velocity of planet.
F = Centripetal force exerted by the Sun on the planet.
Centripetal force is given by,
F = mrω²;
But ω = \(\frac{2 \pi}{T}\)
∴ F = mr (\(\frac{2 \pi}{\mathrm{T}}\))²
∴ F = \(\frac{4 \pi^{2} \mathrm{mr}}{\mathrm{T}^{2}}\) …(i)
According to Kepler’s third law,
T² ∝ r³
T² = Kr³ ……….. (where, K = constant) (ii)
Substituting equation (ii) in equation (i),
F = \(\frac{4 \pi^{2} \mathrm{mr}}{\mathrm{Kr}^{3}}\)
∴ F = \(\frac{4 \pi^{2}}{\mathrm{~K}} \frac{\mathrm{m}}{\mathrm{r}^{2}}\)
∴ F ∝ \(\frac{\mathrm{m}}{\mathrm{r}^{2}}\) ….(∵ \(\frac{4 \pi^{2}}{\mathrm{~K}}\) is a constant quantity)
Since, the gravitational attraction between the Sun and the planet is mutual, force exerted by the planet on the Sun will be proportional to the mass M of the Sun.
∴ F ∝ \(\frac{\mathrm{Mm}}{\mathrm{r}^{2}}\)
∴ F = \(\frac{\mathrm{GMm}}{\mathrm{r}^{2}}\)
The above equation represents Newton’s law of gravitation. In this manner, Newton’s law of gravitation is derived from Kepler’s law of periods.

Question 77.
Represent graphically the variation of total energy, kinetic energy and potential energy of a satellite with its distance from the centre of the Earth.
Answer:
For a satellite,
Potential energy (U) = \(\)
Kinetic energy (K) = \(\) and
Total energy (E) = \(\), where, r = R + h
Also, U and E remain negative whereas K remains positive.
Hence, the graph will be:

Multiple Choice Questions

Question 1.
Kepler’s law of equal areas is an outcome of
(A) conservation of energy
(B) conservation of linear momentum
(C) conservation of angular momentum
(D) conservation of mass
Answer:
(C) conservation of angular momentum

Question 2.
Amongst given statements, choose the correct statement.
(I) Kepler derived the laws of planetary motion.
(II) Newton provided the reason behind the laws of planetary motion.
(A) (I) is correct.
(B) (II) is correct.
(C) Both (I) and (II) are correct.
(D) Neither (I) nor (II) is correct.
Answer:
(B) (II) is correct.

Question 3.
The figure shows the motion of a planet satellite in terms of mean density of Earth. around the Sun in an elliptical orbit with Sun at the focus. The shaded areas A and B are also shown in the figure which can be assumed to be equal. If t₁ and t₂ represent the time for the planet to move from a to b and d to c respectively, then

(A) t₁ < t₂
(B) t₁ >t₂
(C) t₁ = t₂
(D) t₁ ≤ t₂
Answer:
(C) t₁ = t₂

Question 4.
A planet is revolving around a star in a circular orbit of radius R with a period T. If the gravitational force between the planet and the star is proportional to R^-3/2, then
(A) T^{2 ∝} R^5/2
(B) T² ∝ R^-7/2
(C) T² ∝ R^3/2
(D) T² ∝ R⁴
Answer:
(A) T² ∝ R^5/2

Question 5.
Time period of revolution of a satellite around a planet of radius R is T. Period of revolution around another planet whose radius is 3R is
(A) T
(B) 9T
(C) 3T
(D) 3\(\sqrt{3}\) T
Answer:
(D) 3\(\sqrt{3}\) T

Question 6.
Newton’s law of gravitation is called universal law because
(A) force is always attractive.
(B) it is applicable to lighter and heavier bodies.
(C) it is applicable at all times,
(D) it is applicable at all places of universe for all distances between all particles.
Answer:
(D) it is applicable at all places of universe for all distances between all particles.

Question 7.
If the mass of a body is M on the surface of the Earth, the mass of the same body on the surface of the moon is
M
(A) 6M
(B) \(\frac{M}{6}\)
(C) M
(D) Zero
Answer:
(C) M

Question 8.
Which of the following statements about the gravitational constant is true?
(A) It has no units.
(B) It has same value in all systems of units.
(C) It is a force.
(D) It does not depend upon the nature of medium in which the bodies lie.
Answer:
(D) It does not depend upon the nature of medium in which the bodies lie.

Question 9.
The gravitational force between two bodies is ______
(A) attractive at large distance only
(B) attractive at small distance only
(C) repulsive at small distance only
(D) attractive at all distances large or small
Answer:
(D) attractive at all distances large or small

Question 10.
Mass of a particle at the centre of the Earth is
(A) infinite.
(B) zero.
(C) same as at other places.
(D) greater than at the poles.
Answer:
(C) same as at other places.

Question 11.
Which of the following is not a property of gravitational force?
(A) It is an attractive force.
(B) It acts along the line joining the two bodies.
(C) The forces exerted by two bodies on each other form an action-reaction pair.
(D) It has a very finite range of action.
Answer:
(D) It has a very finite range of action.

Question 12.
If the distance between Sun and Earth is made two third times of the present value, then gravitational force between them will become
(A) \(\frac{4}{9}\)times
(B) \(\frac{2}{3}\)times
(C) \(\frac{1}{3}\)times
(D) \(\frac{9}{4}\) times
Answer:
(D) \(\frac{9}{4}\) times

Question 13.
The gravitational constant G is equal to 6.67 × 10^-11 N m²/kg² in vacuum. Its value in a dense matter of density 10¹⁰ g/cm³ will be
(A) 6.67 × 10^-1 N m²/kg²
(B) 6.67 × 10^-11 N m²/kg²
(C) 6.67 × 10^-10 N m²/kg²
(D) 6.67 × 10^-21 N m²/kg²
Answer:
(B) 6.67 × 10^-11 N m²/kg²

Question 14.
Acceleration due to gravity above the Earth’s surface at a height equal to the radius of the Earth is ______
(A) 2.5 m/s²
(B) 5 m/s²
(C) 9.8 m/s²
(D) 10 m/s²
Answer:
(A) 2.5 m/s²

Question 15.
If R is the radius of the Earth and g is the acceleration due to gravity on the Earth’s surface, the mean density of the Earth is

Answer:
(D) \(\frac{3 \mathrm{~g}}{4 \pi \mathrm{RG}}\)

Question 16.
Variation of acceleration due to gravity (g) with distance x from the centre of the Earth is best represented by (R → Radius of the Earth)

Answer:
(D)

Question 17.
Which of the following statements is not correct for the decrease in the value of acceleration due to gravity?
(A) As we go down from the surtce of the Earth towards its centre.
(B) As we go up from the surface of the Earth.
(C) As we go from equator to the poles on the surface on the Earth.
(D) As the rotational velocity of the Earth is increased.
Answer:
(C) As we go from equator to the poles on the surface on the Earth.

Question 18.
Calculate angular velocity of Earth so that acceleration due to gravity at 60° latitude becomes zero. (Radius of Earth = 6400 km, gravitational acceleration at poles = 10 m/s², cos60° = 0.5)
(A) 7.8 × 10^-2 rad/s
(B) 0.5 × 10^-3 radis
(C) 1 × 10^-3 radis
(D) 2.5 × 10^-3 rad/s
Answer:
(D) 2.5 × 10^-3 rad/s

Question 19.
The gravitational potential energy per unit mass at a point gives ________ at that point.
(A) gravitational field
(B) gravitational potential
(C) gravitational potential energy
(D) gravitational force
Answer:
(B) gravitational potential

Question 20.
A satellite is orbiting around a planet at a constant height in a circular orbit. If the mass of the planet is reduced to half, the satellite would
(A) fall on the planet.
(B) go to an orbit of smaller radius.
(C) go to an orbit of higher radius,
(D) escape from the planet.
Answer:
(D) escape from the planet.

Question 21.
How does the escape velocity of a particle depend on its mass?
(A) m²
(B) m
(C) m⁰
(D) m^-1
Answer:
(C) m⁰

Question 22.
Escape velocity on a planet is ve. If radius of the planet remains same and mass becomes 4 times, the escape velocity becomes
(A) 4v_e
(B) 2v_e
(C) v_e
(D) 0.5 v_e
Answer:
(B) 2v_e

Question 23.
If the escape velocity of a body on Earth is 11.2 km/s, the escape velocity of the body thrown at an angle 45° with the horizontal will be
(A) 11.2 km/s
(B) 22.4 km/s
(C) \(\frac{11.2}{\sqrt{2}}\)km/s
(D) 11.2 \(\sqrt{2}\) km/s
Answer:
(A) 11.2 km/s

Question 24.
Potential energy of a body in the gravitational field of planet is zero. The body must be
(A) at centre of planet.
(B) on the surface of planet.
(C) at infinity.
(D) at distance equal to radius of Earth.
Answer:
(C) at infinity.

Question 25.
If gravitational force of Earth disappears, what will happen to the satellite revolving round the Earth?
(A) Satellite will come back to Earth.
(B) Satellite will continue to revolve.
(C) Satellite will escape in tangential path.
(D) Satellite will start falling towards centre.
Answer:
(C) Satellite will escape in tangential path.

Question 26.
If v_e and v_o represent the escape velocity and orbital velocity of a satellite corresponding to a circular orbit of radius R respectively, then
(A) v_e = v_o
(B) \(\sqrt{2}\)v_o = v_e
(C) v_e = \(\frac{1}{\sqrt{2}}\)v_o
(D) v_e and v_o are not related
Answer:
(B) \(\sqrt{2}\)v_o = v_e

Question 27.
If the kinetic energy of a satellite is 2 × 10⁴ J, then its potential energy will be
(A) – 2 × 10⁴ J
(B) 4 × 10⁴ J
(C) -4 × 10⁴ J
(D) -10⁴J
Answer:
(C) -4 × 10⁴ J

Competitive Corner

Question 1.
A body weighs 200 N on the surface of the Earth. How much will it weigh half way down to the centre of the Earth?
(A) 250 N
(B) 100 N
(C) 150 N
(D) 200 N
Answer:
(B) 100 N
Hint:
Acceleration due to gravity at depth d,
g_d = g (1 – \(\frac{\mathrm{d}}{\mathrm{R}}\))
= g(1 – \(\frac{1}{2}\)) …(∵ d = \(\frac{1}{2}\))
∴ g_d = \(\frac{\mathrm{g}}{2}\)
Weight of the body at depth d = R/2,
W_d = mg_d = m × g/2 = \(\frac{1}{2}\) × 200
∴ W_{d = 100 N}

Question 2.
The work done to raise a mass m from the surface of the Earth to a height h, which is equal to the radius of the Earth, is:
(A) \(\frac{1}{2}\) mgR
(B) \(\frac{3}{2}\) mgR
(C) mgR
(D) 2mgR
Answer:
(A) \(\frac{1}{2}\) mgR
Hint:
Initial potential energy on Earth’s surface,
U_i = \(\frac{-\mathrm{GMm}}{\mathrm{R}}\)
Final potential energy at height h = R
U_f = \(\frac{-\mathrm{GMm}}{2 \mathrm{R}}\)
Work done, W = U_f – U_i

∴ W = \(\frac{1}{2}\) mgR

Question 3.
The time period of a geostationary satellite is 24 h, at a height 6R_E (R_E is radius of Earth) from surface of Earth. The time period of another satellite whose height is 2.5 R_E from surface will be,
(A) \(\frac{12}{2.5}\)h
(B) 6\(\sqrt{2}\) h
(C) 12\(\sqrt{2}\) h
(D) \(\frac{24}{2.5}\)h
Answer:
(B) 6\(\sqrt{2}\) h
Hint:
By Kepler’s third law,

Question 4.
Assuming that the gravitational potential energy of an object at infinity is zero, the change in potential energy (final – initial) of an object of mass m, when taken to a height h from the surface of Earth (of radius R), is given by,
(A) \(\frac{\text { GMm }}{R+h}\)
(B) – \(\frac{\text { GMm }}{R+h}\)
(C) \(\frac{\text { GMmh }}{R(R+h)}\)
(D) mgh
Answer:
(C) \(\frac{\text { GMmh }}{R(R+h)}\)
Hint:
Potential energy of object of mass m on the surface of Earth,
P.E = \(\frac{-\mathrm{GMm}}{\mathrm{R}}\)
Potential energy of object of mass m at a height h from the surface of the Earth,
P.E.’ = \(\frac{-\mathrm{GMm}}{\mathrm{R}+\mathrm{h}}\)
∴ Change in potential energy
= P.E.’ – P.E.

Question 5.
A body mass ‘m’ is dropped from height \(\), from Earth’s surface, where ‘R’ is the radius of Earth. Its speed when it will hit the Earth’s surface is (v_e = escape velocity from Earth’s surface)

Answer:
(B) \(\frac{\mathbf{v}_{\mathrm{e}}}{\sqrt{3}}\)
Hint:

Question 6.
According to Kepler’s Law, the areal velocity of the radius vector drawn from the Sun to any planet always
(A) decreases.
(B) first increases and then decreases.
(C) remains constant.
(D) increases.
Answer:
(C) remains constant.

Question 7.
A body is thrown from the surface of the Earth with velocity ‘u’ m/s. The maximum height in m above the surface of the Earth upto which it will reach is (R = radius of Earth, g = acceleration due to gravity)

Answer:
(A) \(\frac{u^{2} R}{2 g R-u^{2}}\)
Hint:
(T.E.) on surface = (T.E.) at height ‘h’
∴ (K.E.)₁ + (P.E.)₁ = (K.E.)₂ + (P.E.)₂

Question 8.
A satellite is revolving in a circular orbit at a height ‘h’ above the surface of the Earth of radius ‘R’. The speed of the satellite in its orbit is one-fourth the escape velocity from the surface of the Earth. The relation between ‘h’ and ‘R’ is
(A) h = 2R
(B) h = 3R
(C) h = 5R
(D) h = 7R
Answer:
(D) h = 7R
Hint:

Question 9.
Two astronauts are floating in gravitational free space after having lost contact with their spaceship. The two will:
(A) keep floating at the same distance between them.
(B) move towards each other.
(C) move away from each other.
(D) will become stationary.
Answer:
(B) move towards each other.

Balbharti Maharashtra State Board 11th Biology Important Questions Chapter 7 Cell Division Important Questions and Answers.

Maharashtra State Board 11th Biology Important Questions Chapter 7 Cell Division

Question 1.
Why interphase is known as preparatory phase.
Answer:
1. During interphase, the cell is metabolically very active.
2. In this phase, a cell grows to its maximum size, chromosomal material (DNA and histone proteins) duplicates and the cell prepares itself for next mitotic division. Hence, inteiphase is known as preparatory phase.

Question 2.
Name the following.
1. In which phase the amount of DNA per cell doubles?
2. Which types of RNA are synthesized during first growth phase?
Answer:
1. S-phase.
2. m-RNA, t-RNA and r-RNA

Question 3.
Match the Column I (Phases of Cell cycle) with Column II (Approximate time for completion).

Column I	Column II
1. G: Phase	(a) 1-3 Hours
2. Gi Phase	(b) 2-5 Hours
3. M Phase	(c) 6-8 Hours
4. S Phase	(d) 8 Hours

Answer:

Column I	Column II
1. G: Phase	(b) 2-5 Hours
2. Gi Phase	(d) 8 Hours
3. M Phase	(a) 1-3 Hours
4. S Phase	(c) 6-8 Hours

Question 4.
What is cell division? Mention the types of cell division.
Answer:
The division of cells into two (or more) daughter cells with same (or different) genetic material is called cell division. There are three types of cell divisions:
1. Amitosis:
a. It is the simplest form of cell division. The nucleus elongates and a constriction appears along its length.
b. This constriction deepens and divides nucleus into two daughter nuclei followed by division of cytoplasm resulting in formation of two daughter cells.
c. This type of division is observed in unicellular organisms, abnormal cells, old cells and in foetal membrane cells.

2. Mitosis:
a. In this type of cell division, the cell divides and forms two similar daughter cells which are identical to the parent cell.
b. It is completed in two steps as karyokinesis and cytokinesis.

3. Meiosis:
a. In this type of cell division, the number of chromosomes is reduced to half. Hence, this type of cell division is also called reductional division.
b. Meiosis produces four haploid daughter cells from a diploid parent cell.

Question 5.
With the help of suitable diagrams, explain karyokinesis in brief.
Answer:
Karyokinesis is the nuclear division which is divided into prophase, metaphase, anaphase and telophase.
1. Prophase:
a. In this phase, condensation of chromatin material, migration of centrioles, appearance of mitotic apparatus and disappearance of nuclear membrane takes place.
b. Due to condensation, each chromosome with its sister chromatids connected by centromere is clearly visible under light microscope.
c. The nucleolus starts to disappear.
d. Centrosome start moving towards the opposite poles of the cell.
e. Mitotic apparatus is almost completely formed.

2. Metaphase:
a. Chromosomes are completely condensed and appear short.
b. Centromere and sister chromatids become very prominent.
c. All the chromosomes are arranged at equatorial plane of cell. This is called metaphase plate.
d. Mitotic spindle is fully formed in this phase.
e. Centromere of each chromosome divides horizontally into two, each being associated with a chromatid. [Note: The centromeres divide at the beginning of anaphase so that the two chromatids of each chromosome become separated from each other.
Source: Cell Division, Donald B. McMillan, Richard J. Harris, in An Atlas of Comparative Vertebrate Histology, 2018.]

3. Anaphase:
a. In this phase, chromatids of each chromosome separate and form two chromosomes called daughter chromosomes.
b. The chromosomes which are formed are pulled away in opposite direction by spindle apparatus.
c. Anaphase ends when each set of chromosomes reach at opposite poles of the cell.

4. Telophase:
a. This is the final stage of karyokinesis.
b. The chromosomes with their centromeres begin to uncoil at the poles.
c. The chromosomes lengthen and lose their individuality.
d. The nucleolus reappears and the nuclear membrane appear around the chromosomes.
e. Spindle fibres breakdown and get absorbed in the cytoplasm. Thus, two daughter nuclei are formed.

These are small disc-shaped structures at the surface of the centromeres which serve as the sites of attachment of spindle fibres to the chromosomes.

Question 6.
Draw neat and labelled diagram of Anaphase.
Answer:
Anaphase:
a. In this phase, chromatids of each chromosome separate and form two chromosomes called daughter chromosomes.
b. The chromosomes which are formed are pulled away in opposite direction by spindle apparatus.
c. Anaphase ends when each set of chromosomes reach at opposite poles of the cell.

Question 7.
Match the following.

Column I	Column II
1. Prophase	(a) Chromatids moving to opposite poles.
2. Metaphase	(b) Nuclear membrane starts disappearing.
3. Anaphase	(c) Chromosomes at equatorial plane of the cell.
4. Telophase	(d) Nuclear membrane reappears

Answer:

Column I	Column II
1. Prophase	(b) Nuclear membrane starts disappearing.
2. Metaphase	(c) Chromosomes at equatorial plane of the cell.
3. Anaphase	(a) Chromatids moving to opposite poles.
4. Telophase	(d) Nuclear membrane reappears

Question 8.
Observe the given diagram and explain the depicted process in your own words.

Answer:

1. The process depicted in the given diagram is cytokinesis in animal cell.
2. This step takes place at the end of karyokinesis (nuclear division) of mitosis.
3. It depicts the division of the cytoplasmic material in order to form two daughter cells that resemble each other.
4. The division starts with a constriction generally at the equator. This constriction gradually deepens and ultimately joins in the centre dividing into two cells.
5. At the time of cytoplasmic division, organelles like mitochondria and plastids get distributed between the two daughter cells.

Question 9.
Diagrammatically differentiate between cytokinesis in animal cell and plant cell.
Answer:

Question 10.
How cell wall is formed in plant cell?
Answer:
The formation of the new cell wall begins with the formation of a simple precursor, called the ‘cell-plate’ that represents the middle lamella between the walls of two adjacent cells.

Question 11.
What is necrosis?
Answer:
Necrosis is a form of cell injury which leads to the premature death of cells. For example: due to scrape or a harmful chemical.

Question 12.
What is apoptosis? Write its significance.
Answer:

1. Apoptosis also known as programmed cell death or cellular suicide. In apoptosis cells die in controlled way.
2. For example: during embryonic development the cells between the embryonic fingers die in a normal process called apoptosis to give a definite shape to the fingers.
3. Apoptosis also helps in eliminating potential cancer cells.

Question 13.
Which type of cell division is known as reductional division? Why?
Answer:
1. Meiosis is known as reductional division.
2. The number of chromosome is reduced to half, hence, meiosis is known as reductional division.

Question 14.
Describe the various phases of heterotypic division.
Answer:
Heterotypic division is first meiotic division, during which a diploid cell is divided into two haploid cells. The daughter cells resulting from this division are different from the parent cell in chromosome number. Hence the division is called heterotypic division.
It consists of following phases:
1. Prophase -I:
It is the most complicated and longest phase of meiotic division.
It is further divided into five sub-phases viz. leptotene, zygotene, pachytene, diplotene and diakinesis.

a. Leptotene:

1. The volume of the nucleus increases.
2. The chromosomes become long distinct and coiled.
3. They orient themselves in a specific fonn known as bouquet stage. This is characterized with the ends of chromosomes converged towards the side of nucleus where the centrosome lies.
4. The centriole duplicates into two and migrates to opposite poles. [Note: Centrioles divide during Gj phase of interphase.]

b. Zygotene:

1. Pairing of non-sister chromatids of homologous chromosomes takes place by formation of synaptonemal complex. This pairing is called synapsis.
2. Each pair consists of a maternal chromosome and a paternal chromosome. Chromosomal pairs are called bivalents or tetrads.

c. Pachytene:

1. Each individual chromosome begins to split longitudinally into two similar chromatids. Therefore, each bivalent now appears as a tetrad consisting of four chromatids.
2. The homologous chromosomes begin to separate but they do not separate completely and remain attached to one or more points. These points are called chiasmata (Appear like a cross-X).
3. Chromatids break at these points and broken segments are exchanged between non-sister chromatids of homologous chromosomes resulting in recombination.

d. Diplotene:
The chiasma becomes clearly visible in diplotene due to beginning of repulsion between synapsed homologous chromosomes. This is known as desynapsis. Synaptonemal complex also starts to disappear.

e. Diakinesis:

1. The chiasmata begin to move along the length of chromosomes from the centromere towards the ends of chromosomes. The displacement of chiasmata is termed as terminalization.
2. The terminal chiasmata exist till the metaphase.
3. The nucleolus and nuclear membrane completely disappear and spindle fibres begin to appear.

2. Metaphase -1:
a. The spindle fibres are well developed.
b. The tetrads orient themselves on equator in such a way that centromeres of homologous tetrads lie towards the poles and arms towards the equator.
c. They are ready to separate as repulsive force increases.
a. Homologous chromosomes are carried towards the opposite poles by spindle apparatus. This is known as disjunction.
b. The two sister chromatids of each chromosome do not separate in meiosis -I. This is reductional division.
c. The sister chromatids of each chromosome are connected by a common centromere.
d. Both sister chromatids of each chromosome are now different in genetic content as one of them has undergone recombination.

3. Anaphase – I:
1. Homologous chromosomes are carried towards the opposite poles by spindle apparatus. This is known as disjunction.
2. The two sister chromatids of each chromosome do not separate in meiosis -I. This is reductional division.
3. The sister chromatids of each chromosome are connected by a common centromere.
4. Both sister chromatids of each chromosome are now different in genetic content as one of them has undergone recombination.

4. Telophase-I:
a. The haploid number of chromosomes becomes uncoiled and elongated after reaching their respective poles.
b. The nuclear membrane and nucleolus reappear and thus two daughter nuclei are formed.

Cytokinesis -1:
Cytokinesis occurs after karyokinesis and two haploid cells are formed. In many cases, these daughter cells pass through interkinesis.

[Note: The association between the homologous chromosomes i.e. chiasmata remain till metaphase I. During metaphase /, the paired homologous chromosomes move to the metaphase plate. In anaphase [ the spindle fibers begin to shorten. As these spindle fibres shorten, the association between homologous chromosomes (chiasmata) are broken, allowing homologous chromosomes to be pulled to opposite poles.]

Question 15.
What is Homotypic Division? Explain its phases.
Answer:
Two haploid cells formed during first meiotic division divide further into four haploid cells this division is called homotypic division. It consists of five phases: prophase – II, metaphase – II, anaphase – II, telophase – II, and Cytokinesis – II.

1. Prophase-II:
a. The chromosomes are distinct with two chromatids.
b. Each centriole divides into two resulting in formation of two centrioles which migrate to opposite poles and form asters.
c. Spindle fibres are formed between the centrioles.
d. The nuclear membrane and nucleolus disappears in this phase.

2. Metaphase -II:
a. Chromosomes are arranged at the equator.
b. The two chromatids of each chromosome are separated by division of the centromere.
c. Some of the spindle fibres are attached to the centromeres and some are arranged end to end between two opposite centrioles.

3. Anaphase – II:
In this phase, the separated chromatids become daughter chromosomes and move to opposite poles due to the contraction of the spindle fibres attached to centromeres.

4. Telophase – II:
a. In this stage daughter chromosomes starts to uncoil.
b. The nuclear membrane surrounds each group of chromosomes.
c. Nucleolus reappears in this phase.

5. Cytokinesis – II
a. Cytokinesis takes place after the nuclear division.
b. Two haploid cells are formed from each haploid cell.
c. Thus, four haploid daughter cells are formed.
d. These cells then undergo changes to form gametes.

Question 16.
Why meiosis is important?
Answer:

1. Meiotic division produces gametes or spores.
2. If it is absent, the number of chromosomes would double or quadruple resulting in the formation of monstrosities (abnormal gametes).
3. The constant number of chromosomes in a given species across generations is maintained by meiosis and fertilization.
4. Because of crossing over, exchange of genetic material takes place leading to genetic variations, which are the raw materials for evolution.

Question 17.
Observe the diagram and answer the questions given below it.

1. Identify the type of cell division shown in the diagram.
2. Write its significance of meiosis.
Answer:
1. The type of cell division shown in diagram is meiosis II.
2. Meiotic division produces gametes or spores.

1. If it is absent, the number of chromosomes would double or quadruple resulting in the formation of monstrosities (abnormal gametes).
2. The constant number of chromosomes in a given species across generations is maintained by meiosis and fertilization.
3. Because of crossing over, exchange of genetic material takes place leading to genetic variations, which are the raw materials for evolution.

Question 18.
Explain Anaphase-I with a neat labelled diagram.
Answer:

Anaphase:
a. In this phase, chromatids of each chromosome separate and form two chromosomes called daughter chromosomes.
b. The chromosomes which are formed are pulled away in opposite direction by spindle apparatus.
c. Anaphase ends when each set of chromosomes reach at opposite poles of the cell.

Question 19.
What is crossing over? Give its significance.
Answer:
Crossing over:
The process of exchange of genetic material between non-sister chromatids of homologous chromosomes is known as crossing over.
Significance of crossing over:
Crossing over results in genetic recombination of parental characters that leads to variations.

Question 20.
What happens during diakinesis?
Answer:

1. In diakinesis, the chiasmata begin to move along the length of chromosomes from the centromere towards the ends of chromosomes.
2. The displacement of chiasmata is termed as terminalization. The terminal chiasmata exist till the metaphase.
3. The nucleolus disappears and the nuclear membrane also begins to disappear.
4. Spindle fibres starts to appear in the cytoplasm.

Question 21.
Differentiate between anaphase of mitosis and anaphase – I of meiosis.
Answer:

Anaphase of mitosis	Anaphase – I of meiosis
1. Centromere divides into two, resulting in the separation of chromatids.	Centromere does not divide.
2. Homologous chromosomes are not involved.	Homologous chromosomes are involved.
3. Disjunction does not occur.	Disjunction occurs.
4. Same number of chromosomes gather at each pole.	Half the chromosome number gather at respective pole.

Question 22.
Give reasons: Meiosis is known as reductional division.
Answer:
Meiosis is known as reductional division because the parent cell produces four daughter cells each having half the number of chromosomes present in the parent cell.

Question 23.
Fill in the blanks:

1. The process of mitosis maintains the _______.
2. ________ involves the cell death, but it benefits the organism as a whole.
3. Crossing over takes place in _______ phase of Prophase-I.

Answer:

1. The process of mitosis maintains the nucleo-cytoplasmic ratio.
2. Apoptosis involves the cell death, but it benefits the organism as a whole.
3. Crossing over takes place in pachytene phase of Prophase-I.

Question 24.
1. Complete the following flowchart.
2. Explain the type of cell division in which chromosome number remain the same as that of the parent cell.

Answer:
Karyokinesis is the nuclear division which is divided into prophase, metaphase, anaphase and telophase.
1. Prophase:
a. In this phase, condensation of chromatin material, migration of centrioles, appearance of mitotic apparatus and disappearance of nuclear membrane takes place.
b. Due to condensation, each chromosome with its sister chromatids connected by centromere is clearly visible under light microscope.
c. The nucleolus starts to disappear.
d. Centrosome start moving towards the opposite poles of the cell.
e. Mitotic apparatus is almost completely formed.

2. Metaphase:
a. Chromosomes are completely condensed and appear short.
b. Centromere and sister chromatids become very prominent.
c. All the chromosomes are arranged at equatorial plane of cell. This is called metaphase plate.
d. Mitotic spindle is fully formed in this phase.
e. Centromere of each chromosome divides horizontally into two, each being associated with a chromatid. [Note: The centromeres divide at the beginning of anaphase so that the two chromatids of each chromosome become separated from each other.
Source: Cell Division, Donald B. McMillan, Richard J. Harris, in An Atlas of Comparative Vertebrate Histology, 2018.]

3. Anaphase:
a. In this phase, chromatids of each chromosome separate and form two chromosomes called daughter chromosomes.
b. The chromosomes which are formed are pulled away in opposite direction by spindle apparatus.
c. Anaphase ends when each set of chromosomes reach at opposite poles of the cell.

4. Telophase:
a. This is the final stage of karyokinesis.
b. The chromosomes with their centromeres begin to uncoil at the poles.
c. The chromosomes lengthen and lose their individuality.
d. The nucleolus reappears and the nuclear membrane appear around the chromosomes.
e. Spindle fibres breakdown and get absorbed in the cytoplasm. Thus, two daughter nuclei are formed.

These are small disc-shaped structures at the surface of the centromeres which serve as the sites of attachment of spindle fibres to the chromosomes.

Question 25.
While studying mitosis, different teams of students made following observations in the cells focused under microscope.
1. In certain cells chromosomes were arranged at equatorial plane with fibres originating from cylindrical structures at both the poles.
2. Few cells showed chromatids moving towards opposite poles.
a. In first observation which stage of mitosis is seen by students and what is the scientific term used to represent cylindrical structures?
b. Which stage is seen in the second observation?
Answer:
a. The stage observed in the first case is metaphase. The scientific term used to represent the cylindrical structures are centrioles.
b. The other stage seen in second observation is anaphase.

Question 26.
During biology practical students were asked to see the slide mounted under microscope and note down their observations. Few students noted that the stage observed is anaphase of mitosis and others said that it is anaphase I of meiosis. Later while explaining about experiments teacher said that it is anaphase I of meiosis. On what basis teacher confirmed that it is anaphase I of meiosis?
Answer:
Chromosomes moving towards opposite poles during anaphase I do not separate at the centromeres.

Question 27.
Colchicine is an alkaloid extracted from plants. It prevents the formation of spindle fibres. In the presence colchicine, if a cell enters mitosis what would be the outcome?
Answer:
The spindle fibres are necessary for segregating the sister chromatids to opposite poles of the cell during anaphase. In the presence of colchicine, no spindle fibres will form to attach to the kinetochores (small disc¬like structures present on chromosomes). Therefore, the cell will be stuck in mitosis with the condensed pairs of sister chromatids in an unorganized array.

Question 28.
Read the following statements and mention whether they are TRUE or FALSE in respective boxes.
1. Life of all multicellular organisms starts from single cell which is known as zygote.
2. Spindle fibres present between centriole and centromere are known as polar fibres which can contract.
3. Growth of every living organism depends on cell division.
4. Spindle fibres present between opposite centrioles are called as kinetochore fibres which can elongate.

	(i)	(ii)	(iii)	(iv)
(A)	T	T	F	T
(B)	F	F	T	F
(C)	T	F	T	F
(D)	1	T	F	F ‘

Answer:
(C)

Question 29.
Quick Review

Question 30.
Exercise:

Question 1.
Define cell cycle.
Answer:

1. Sequential events occurring in the life of a cell is called cell cycle.
2. Interphase and M – phase are the two phases of cell cycle.
3. Cell undergoes growth or rest during interphase and divides during M – phase.

Question 2.
Observe the following diagram and the questions based on it.

1. If the initial amount of DNA in a cell is 2C then in which phase of cell cycle the amount of this DNA would become 4C? Also name the process.
2. Which sub-phase of the interphase is of short duration?
3. Enlist the phases of karyokinesis in proper order.
Answer:
S – phase (Synthesis phase):
In this phase DNA is synthesized (replicated), so that amount of DNA per cell doubles.
Synthesis of histone proteins takes place in this phase.
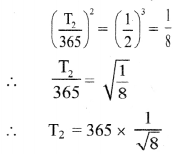
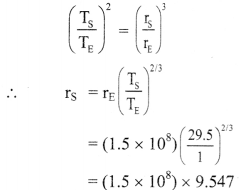
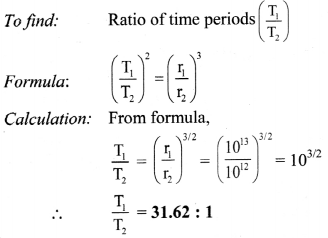
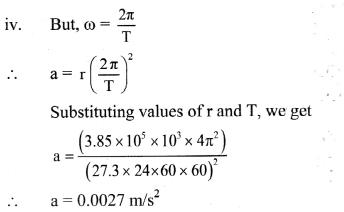
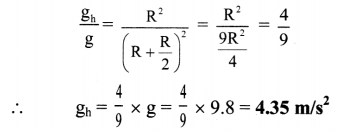
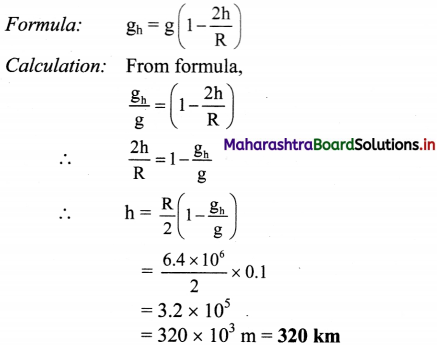
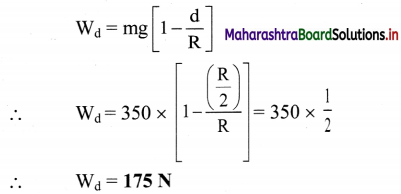
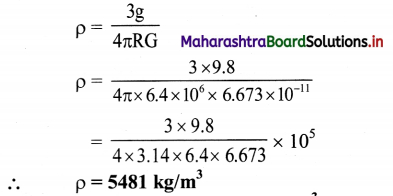
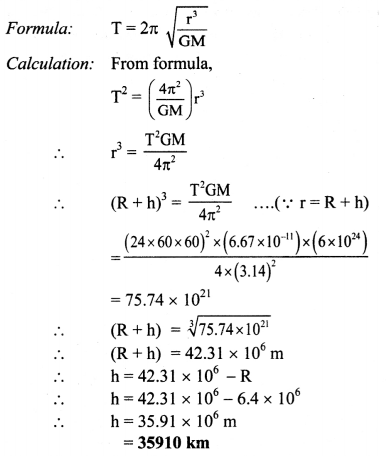
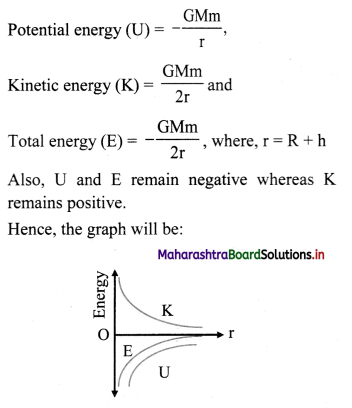
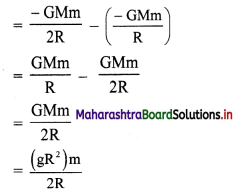
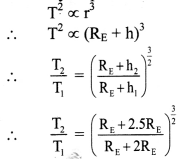
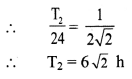
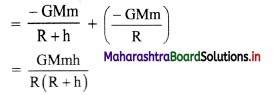
Karyokinesis is the nuclear division which is divided into prophase, metaphase, anaphase and telophase.
1. Prophase:
a. In this phase, condensation of chromatin material, migration of centrioles, appearance of mitotic apparatus and disappearance of nuclear membrane takes place.
b. Due to condensation, each chromosome with its sister chromatids connected by centromere is clearly visible under light microscope.
c. The nucleolus starts to disappear.
d. Centrosome start moving towards the opposite poles of the cell.
e. Mitotic apparatus is almost completely formed.

2. Metaphase:
a. Chromosomes are completely condensed and appear short.
b. Centromere and sister chromatids become very prominent.
c. All the chromosomes are arranged at equatorial plane of cell. This is called metaphase plate.
d. Mitotic spindle is fully formed in this phase.
e. Centromere of each chromosome divides horizontally into two, each being associated with a chromatid. [Note: The centromeres divide at the beginning of anaphase so that the two chromatids of each chromosome become separated from each other.
Source: Cell Division, Donald B. McMillan, Richard J. Harris, in An Atlas of Comparative Vertebrate Histology, 2018.]

3. Anaphase:
a. In this phase, chromatids of each chromosome separate and form two chromosomes called daughter chromosomes.
b. The chromosomes which are formed are pulled away in opposite direction by spindle apparatus.
c. Anaphase ends when each set of chromosomes reach at opposite poles of the cell.

4. Telophase:
a. This is the final stage of karyokinesis.
b. The chromosomes with their centromeres begin to uncoil at the poles.
c. The chromosomes lengthen and lose their individuality.
d. The nucleolus reappears and the nuclear membrane appear around the chromosomes.
e. Spindle fibres breakdown and get absorbed in the cytoplasm. Thus, two daughter nuclei are formed.

These are small disc-shaped structures at the surface of the centromeres which serve as the sites of attachment of spindle fibres to the chromosomes.

Question 3.
During which stage of Prophase-I synapsis occurs?
Answer:
b. Zygotene:
Pairing of non-sister chromatids of homologous chromosomes takes place by formation of synaptonemal complex. This pairing is called synapsis.
Each pair consists of a maternal chromosome and a paternal chromosome. Chromosomal pairs are called bivalents or tetrads.

Question 4.
During which stage disjunction takes place?
Answer:
Telophase-I:
a. The haploid number of chromosomes becomes uncoiled and elongated after reaching their respective poles.
b. The nuclear membrane and nucleolus reappear and thus two daughter nuclei are formed.

Question 5.
What is disjunction?
Answer:
Telophase-I:
a. The haploid number of chromosomes becomes uncoiled and elongated after reaching their respective poles.
b. The nuclear membrane and nucleolus reappear and thus two daughter nuclei are formed.

Question 6.
Why meiosis is known as reductional division?
Answer:
In this type of cell division, the number of chromosomes is reduced to half. Hence, this type of cell division is also called reductional division.

Question 7.
Sketch and label the phase of cell division in which synaptonemal complex is formed?
Answer:
Zygotene:
Pairing of non-sister chromatids of homologous chromosomes takes place by formation of synaptonemal complex. This pairing is called synapsis.
Each pair consists of a maternal chromosome and a paternal chromosome. Chromosomal pairs are called bivalents or tetrads.

Question 8.
Make a schematic representation of a type of cell division in which chromosome number is reduced to half.
Answer:
Karyokinesis is the nuclear division which is divided into prophase, metaphase, anaphase and telophase.
1. Prophase:
a. In this phase, condensation of chromatin material, migration of centrioles, appearance of mitotic apparatus and disappearance of nuclear membrane takes place.
b. Due to condensation, each chromosome with its sister chromatids connected by centromere is clearly visible under light microscope.
c. The nucleolus starts to disappear.
d. Centrosome start moving towards the opposite poles of the cell.
e. Mitotic apparatus is almost completely formed.

2. Metaphase:
a. Chromosomes are completely condensed and appear short.
b. Centromere and sister chromatids become very prominent.
c. All the chromosomes are arranged at equatorial plane of cell. This is called metaphase plate.
d. Mitotic spindle is fully formed in this phase.
e. Centromere of each chromosome divides horizontally into two, each being associated with a chromatid. [Note: The centromeres divide at the beginning of anaphase so that the two chromatids of each chromosome become separated from each other.
Source: Cell Division, Donald B. McMillan, Richard J. Harris, in An Atlas of Comparative Vertebrate Histology, 2018.]

3. Anaphase:
a. In this phase, chromatids of each chromosome separate and form two chromosomes called daughter chromosomes.
b. The chromosomes which are formed are pulled away in opposite direction by spindle apparatus.
c. Anaphase ends when each set of chromosomes reach at opposite poles of the cell.

4. Telophase:
a. This is the final stage of karyokinesis.
b. The chromosomes with their centromeres begin to uncoil at the poles.
c. The chromosomes lengthen and lose their individuality.
d. The nucleolus reappears and the nuclear membrane appear around the chromosomes.
e. Spindle fibres breakdown and get absorbed in the cytoplasm. Thus, two daughter nuclei are formed.

These are small disc-shaped structures at the surface of the centromeres which serve as the sites of attachment of spindle fibres to the chromosomes.

Question 9.
Describe mitosis and its stages in brief.
Answer:
Karyokinesis is the nuclear division which is divided into prophase, metaphase, anaphase and telophase.
1. Prophase:
a. In this phase, condensation of chromatin material, migration of centrioles, appearance of mitotic apparatus and disappearance of nuclear membrane takes place.
b. Due to condensation, each chromosome with its sister chromatids connected by centromere is clearly visible under light microscope.
c. The nucleolus starts to disappear.
d. Centrosome start moving towards the opposite poles of the cell.
e. Mitotic apparatus is almost completely formed.

2. Metaphase:
a. Chromosomes are completely condensed and appear short.
b. Centromere and sister chromatids become very prominent.
c. All the chromosomes are arranged at equatorial plane of cell. This is called metaphase plate.
d. Mitotic spindle is fully formed in this phase.
e. Centromere of each chromosome divides horizontally into two, each being associated with a chromatid. [Note: The centromeres divide at the beginning of anaphase so that the two chromatids of each chromosome become separated from each other.
Source: Cell Division, Donald B. McMillan, Richard J. Harris, in An Atlas of Comparative Vertebrate Histology, 2018.]

3. Anaphase:
a. In this phase, chromatids of each chromosome separate and form two chromosomes called daughter chromosomes.
b. The chromosomes which are formed are pulled away in opposite direction by spindle apparatus.
c. Anaphase ends when each set of chromosomes reach at opposite poles of the cell.

4. Telophase:
a. This is the final stage of karyokinesis.
b. The chromosomes with their centromeres begin to uncoil at the poles.
c. The chromosomes lengthen and lose their individuality.
d. The nucleolus reappears and the nuclear membrane appear around the chromosomes.
e. Spindle fibres breakdown and get absorbed in the cytoplasm. Thus, two daughter nuclei are formed.

These are small disc-shaped structures at the surface of the centromeres which serve as the sites of attachment of spindle fibres to the chromosomes.

Question 10.
Describe chiasmata. Draw diagram to illustrate your answer.
Answer:
Pachytene:
Each individual chromosome begins to split longitudinally into two similar chromatids. Therefore, each bivalent now appears as a tetrad consisting of four chromatids.
The homologous chromosomes begin to separate but they do not separate completely and remain attached to one or more points. These points are called chiasmata (Appear like a cross-X).
Chromatids break at these points and broken segments are exchanged between non-sister chromatids of homologous chromosomes resulting in recombination.

Diplotene:
The chiasma becomes clearly visible in diplotene due to beginning of repulsion between synapsed homologous chromosomes. This is known as desynapsis. Synaptonemal complex also starts to disappear.

Question 11.
Correct the following diagram and write a short note on it.

Answer:
b. Zygotene:
Pairing of non-sister chromatids of homologous chromosomes takes place by formation of synaptonemal complex. This pairing is called synapsis.
Each pair consists of a maternal chromosome and a paternal chromosome. Chromosomal pairs are called bivalents or tetrads.

Question 12.
Explain prophase I in your own words.
Answer:
Prophase -I:
It is the most complicated and longest phase of meiotic division.
It is further divided into five sub-phases viz. leptotene, zygotene, pachytene, diplotene and diakinesis.

Question 13.
Explain homotypic division.
Answer:
Two haploid cells formed during first meiotic division divide further into four haploid cells this division is called homotypic division. It consists of five phases: prophase – II, metaphase – II, anaphase – II, telophase – II, and Cytokinesis – II.

1. Prophase-II:
a. The chromosomes are distinct with two chromatids.
b. Each centriole divides into two resulting in formation of two centrioles which migrate to opposite poles and form asters.
c. Spindle fibres are formed between the centrioles.
d. The nuclear membrane and nucleolus disappears in this phase.

2. Metaphase -II:
a. Chromosomes are arranged at the equator.
b. The two chromatids of each chromosome are separated by division of the centromere.
c. Some of the spindle fibres are attached to the centromeres and some are arranged end to end between two opposite centrioles.

3. Anaphase – II:
In this phase, the separated chromatids become daughter chromosomes and move to opposite poles due to the contraction of the spindle fibres attached to centromeres.

4. Telophase – II:
a. In this stage daughter chromosomes starts to uncoil.
b. The nuclear membrane surrounds each group of chromosomes.
c. Nucleolus reappears in this phase.

5. Cytokinesis – II
a. Cytokinesis takes place after the nuclear division.
b. Two haploid cells are formed from each haploid cell.
c. Thus, four haploid daughter cells are formed.
d. These cells then undergo changes to form gametes.

Question 14.
How does cytokinesis in plant cells differ from animal cells?
Answer:
The formation of the new cell wall begins with the formation of a simple precursor, called the ‘cell-plate’ that represents the middle lamella between the walls of two adjacent cells.

Question 15.
What is the significance of meiosis in sexually reproducing animals?
Answer:

1. Meiotic division produces gametes or spores.
2. If it is absent, the number of chromosomes would double or quadruple resulting in the formation of monstrosities (abnormal gametes).
3. The constant number of chromosomes in a given species across generations is maintained by meiosis and fertilization.
4. Because of crossing over, exchange of genetic material takes place leading to genetic variations, which are the raw materials for evolution.
5. Gametes are produced by the process of meiosis which are essential for sexual reproduction.
6. Diploid organisms have two set of chromosomes (one paternal and one maternal).
7. For a diploid organism to undergo sexual reproduction it needs to create gametes that contain only one set of chromosomes so the number of chromosomes remains same in the next generation.
8. In absence of meiosis, the chromosome number of parents and their offsprings will differ in every generation; hence no species will hold its characters.
9. Also, there will be no crossing over of homologous chromosomes. Thus, there will be no variations with respect to the changing environment in progeny to maintain their existence, which may lead to extinction of species.

Question 16.
Explain the first three stages of Meiosis II.
Answer:
Two haploid cells formed during first meiotic division divide further into four haploid cells this division is called homotypic division. It consists of five phases: prophase – II, metaphase – II, anaphase – II, telophase – II, and Cytokinesis – II.

1. Prophase-II:
a. The chromosomes are distinct with two chromatids.
b. Each centriole divides into two resulting in formation of two centrioles which migrate to opposite poles and form asters.
c. Spindle fibres are formed between the centrioles.
d. The nuclear membrane and nucleolus disappears in this phase.

2. Metaphase -II:
a. Chromosomes are arranged at the equator.
b. The two chromatids of each chromosome are separated by division of the centromere.
c. Some of the spindle fibres are attached to the centromeres and some are arranged end to end between two opposite centrioles.

3. Anaphase – II:
In this phase, the separated chromatids become daughter chromosomes and move to opposite poles due to the contraction of the spindle fibres attached to centromeres.

4. Telophase – II:
a. In this stage daughter chromosomes starts to uncoil.
b. The nuclear membrane surrounds each group of chromosomes.
c. Nucleolus reappears in this phase.

5. Cytokinesis – II
a. Cytokinesis takes place after the nuclear division.
b. Two haploid cells are formed from each haploid cell.
c. Thus, four haploid daughter cells are formed.
d. These cells then undergo changes to form gametes.

Question 17.
Sketch, label and describe telophase in mitosis.
Answer:
Telophase:
a. This is the final stage of karyokinesis.
b. The chromosomes with their centromeres begin to uncoil at the poles.
c. The chromosomes lengthen and lose their individuality.
d. The nucleolus reappears and the nuclear membrane appear around the chromosomes.
e. Spindle fibres breakdown and get absorbed in the cytoplasm. Thus, two daughter nuclei are formed.

These are small disc-shaped structures at the surface of the centromeres which serve as the sites of attachment of spindle fibres to the chromosomes.

Question 18.
Explain the process recombination.
Answer:
a. Recombination is exchange of genetic material between paternal and maternal chromosomes during gamete formation.
b. The points where crossing over takes place is known as chiasmata.
c. Chromatids acquire new combinations of alleles by physically exchanging segments in crossing-over.
d. The exchange of genetic material between homologous chromosomes involves accurate breakage and joining of DNA molecules through a complex mechanism.
e. It is catalyzed by enzymes.

Question 19.
1. What is necrosis?
2. What is apoptosis? Mention its significance.
Answer:
1. Necrosis is a form of cell injury which leads to the premature death of cells. For example: due to scrape or a harmful chemical.
(2) 1. Apoptosis also known as programmed cell death or cellular suicide. In apoptosis cells die in controlled way.
2. For example: during embryonic development the cells between the embryonic fingers die in a normal process called apoptosis to give a definite shape to the fingers.
3. Apoptosis also helps in eliminating potential cancer cells.

Question 20.
Multiple Choice Questions:

Question 1.
Replication of DNA takes place during
(A) prophase
(B) S-phase
(C) G₂ phase
(D) Interkinesis
Answer:
(B) S-phase

Question 2.
During cell division, spindle fibers are attached to
(A) telomere
(B) centromere
(C) chromomeres
(D) chromosome
Answer:
(B) centromere

Question 3.
Which of the following is the shortest phase?
(A) metaphase
(B) anaphase
(C) interphase
(D) S-phase
Answer:
(B) anaphase

Question 4.
Reappearance of nucleolus is during
(A) telophase
(B) prophase
(C) cytokinesis
(D) inter-kinesis
Answer:
(A) telophase

Question 5.
During telophase,
(A) nuclear membrane is formed.
(B) nucleolus appears.
(C) astral rays disappear.
(D) all the above
Answer:
(D) all the above

Question 6.
Cytokinesis in plant cell takes place by
(A) furrowing
(B) cell plate formation
(C) any one of (A) or (B)
(D) none of these
Answer:
(B) cell plate formation

Question 7.
Meiosis is a
(A) homotypic division
(B) equatorial division
(C) reductional division
(D) none of the above
Answer:
(C) reductional division

Question 8.
Formation of Synaptonemal complex during meiosis occurs at
(A) Leptotene
(B) Zygotene
(C) Diplotene
(D) Pachytene
Answer:
(B) Zygotene

Question 9.
Crossing over takes place in the ________ stage.
(A) leptotene
(B) zygotene
(C) pachytene
(D) diplotene
Answer:
(C) pachytene

Question 10.
Crossing over takes place between
(A) sister chromatids
(B) non-homologous chromosomes
(C) non-sister chromatids of homologues
(D) any two chromatids
Answer:
(C) non-sister chromatids of homologues

Question 11.
Crossing over of chromosomes during meiosis leads to
(A) mutation
(B) sex determination
(C) new gene combination
(D) loss of chromosomes
Answer:
(C) new gene combination

Question 12.
Points at which crossing over has taken place between homologous chromosomes are called
(A) chiasmata
(B) synaptonemal complexes
(C) centromeres
(D) telomere
Answer:
(A) chiasmata

Question 13.
Which of the following events take place during diplotene stage of prophase I of meiosis?
(A) Compaction of chromosomes
(B) Formation of synapsis
(C) Process of crossing over
(D) Repulsion of homologues
Answer:
(D) Repulsion of homologues

Question 14.
The correct sequence of stages in prophase I of meiosis is
(A) Leptotene, Pachytene, Zygotene, Diakinesis, Diplotene
(B) Zygotene, Leptotene, Pachytene, Diakinesis, Diplotene
(C) Leptotene, Zygotene, Pachytene, Diplotene, Diakinesis
(D) Diplotene, Diakinesis, Pachytene, Zygotene, Leptotene
Answer:
(C) Leptotene, Zygotene, Pachytene, Diplotene, Diakinesis

Question 15.
In which phase of meiosis are homologous chromosomes separated?
(A) Anaphase I
(B) Prophase II
(C) Anaphase II
(D) Prophase I
Answer:
(A) Anaphase I

Question 16.
Mitosis differs from meiosis in not having
(A) Long prophase
(B) duplication of DNA
(C) Synapsis and crossing over
(D) interphase
Answer:
(C) Synapsis and crossing over

Question 17.
How many divisions are required to produce 128 gametes?
(A) 64
(B) 16
(C) 32
(D) 12
Answer:
(C) 32

Question 18.
Number of cells undergoing meiotic divisions to produce 124 microspores in angiosperm is
(A) 62
(B) 31
(C) 124
(D) 8
Answer:
(B) 31

Question 19.
How many haploid daughter cells are produced at the end of meiosis-II?
(A) 2
(B) 4
(C) 6
(D) 8
Answer:
(B) 4

Question 21.
Competitive Corner:

Question 1.
Crossing over takes place between which chromatids and in which stage of the cell cycle?
(A) Non-sister chromatids of nonhomologous chromosomes at Pachytene stage of prophase I
(B) Non-sister chromatids of nonhomologous chromosomes at Zygotene stage of prophase I
(C) Non-sister chromatids of homologous chromosomes at Pachytene stage of prophase I
(D) Non-sister chromatids of homologous chromosomes at Zygotene stage of prophase I
Answer:
(C) Non-sister chromatids of homologous chromosomes at Pachytene stage of prophase I

Question 2.
After meiosis I, the resultant daughter cells have
(A) four times the amount of DNA in comparison to haploid gamete.
(B) same amount of DNA as in the parent cell in S phase.
(C) twice the amount of DNA in comparison to haploid gamete.
(D) same amount of DNA in comparison to haploid gamete.
Answer:
(C) twice the amount of DNA in comparison to haploid gamete.

Question 3.
Cells in G₀ phase
(A) suspend the cell cycle
(B) terminate the cell cycle
(C) exit the cell cycle
(D) enter the cell cycle
Answer:
(C) exit the cell cycle

Question 4.
The CORRECT sequence of phases of cell cycle is: [NEET (UG) 2019]
(A) S → G₁ → G₂ → M
(B) G₁ → S → G₂ → M
(C) M → G₁ → G₂ → S
(D) G₁ → G₂ → S → M
Answer:
(B) G₁ → S → G₂ → M

Question 5.
The stage during which separation of the paired homologous chromosomes begins is
(A) Diakinesis
(B) Diplotene
(C) Pachytene
(D) Zygotene
Answer:
(B) Diplotene

Question 6.
Which of the following options gives the correct sequence of events during mitosis?
(A) Condensation → nuclear membrane disassembly → crossing over – segregation → telophase
(B) Condensation → nuclear membrane disassembly → arrangement at equator → centromere division → segregation → telophase
(C) Condensation → crossing over → nuclear membrane disassembly → segregation → telophase
(D) Condensation → arrangement at equator → centromere division → segregation → telophase
Answer:
(B) Condensation → nuclear membrane disassembly → arrangement at equator → centromere division → segregation → telophase

Question 7.
Which of the following is not a characteristic feature during mitosis in somatic cells?
(A) Chromosome movement
(B) Synapsis
(C) Spindle fibres
(D) Disappearance of nucleolus
Answer:
(B) Synapsis

Question 8.
Arrange the following events of meiosis in correct sequence. [AIPMT Retest 2015]
(a) Crossing over
(b) Synapsis
(c) Terminalisation of chiasmata
(d) Disappearance of nucleolus
(A) (b), (c), (d), (a)
(B) (b), (a), (d), (c)
(C) (b),(a), (c), (d)
(D) (a), (b), (c), (d)
Answer:
(C) (b),(a), (c), (d)

Balbharti Maharashtra State Board 11th Physics Important Questions Chapter 4 Laws of Motion Important Questions and Answers.

Maharashtra State Board 11th Physics Important Questions Chapter 4 Laws of Motion

Question 1.
‘Rest and motion are relative concepts.’ Explain the statement with an example.
Answer:

1. A body can be described to be at rest or in motion with respect to a system of co¬ordinate axes known as the frame of reference.
2. A body is in motion if it changes its position with respect to a fixed reference point in a frame of reference. On the other hand, a body is at rest if it does not change its position with respect to a fixed reference point in a frame of reference.
3. An object can be said to be at rest with respect to a frame of reference while the same object can be said to be in motion with respect to a different frame of reference.
   Example: In a running train, all the travellers in the train are in a state of rest if the train is taken as the frame of reference. On the other hand, all the travellers in the train are in a state of motion if ground (or platform) is taken as the frame of reference.
4. Thus, motion and rest always need a frame of reference to be described. Hence, rest and motion are relative concepts.

Question 2.
Explain how acceleration and initial velocity decides trajectory of a motion.
Answer:

1. The resultant motion is linear if:
   • initial velocity \(\overrightarrow{\mathrm{u}}\) = 0 (starting from rest) and acceleration \(\overrightarrow{\mathrm{a}}\) is in any direction.
   • initial velocity \(\overrightarrow{\mathrm{u}}\) ≠ 0 and acceleration a is in line with the initial velocity (same or opposite direction).
2. The resultant motion is circular if initial velocity \(\overrightarrow{\mathrm{u}}\) ≠ 0 and acceleration \(\overrightarrow{\mathrm{a}}\) is perpendicular to the velocity throughout.
3. The resultant motion is parabolic if the initial velocity \(\overrightarrow{\mathrm{u}}\) is not in line with the acceleration \(\overrightarrow{\mathrm{a}}\) and \(\overrightarrow{\mathrm{a}}\) = constant.
   e.g., trajectory of a projectile motion.
4. Similarly, various other combinations of initial velocity and acceleration will result into more complicated motions.

Question 3.
State Newton’s first law of motion.
Answer:
Statement: Every inanimate object continues to be in a state of rest or of uniform unaccelerated motion along a straight line, unless it is acted upon by an external, unbalanced force.

Question 4.
An astronaut accidentally gets separated out of his small spaceship accelerating in inter stellar space at a constant rate of 100 m s^-2. What is the acceleration of the astronaut the instant after he is outside the spaceship? (Assume that there are no nearby stars to exert gravitational force on him.) (NCERT)
Answer:

1. Assuming absence of stars in the vicinity, the only gravitational force exerted on astronaut is by the spaceship.
2. But this force is negligible.
3. Hence, once astronaut is out of the spaceship net external force acting on him can be taken as zero.
4. From the first law of motion, the acceleration of astronaut is zero.

Question 5.
Give the magnitude and direction of the net force acting on:

1. a drop of rain falling down with a constant speed.
2. a cork of mass 10 g floating on water.
3. a kite skilfully held stationary in the sky.
4. a car moving with a constant velocity of 30 kmh^-1 on a rough road.
5. a high speed electron in space far from all gravitating objects, and free of electric and magnetic fields. (NCERT)

Answer:

1. The drop of rain falls down with a constant speed, hence according to the first law of motion, the net force on the drop of rain is zero.
2. Since the 10 g cork is floating on water, its weight is balanced by the up thrust due to water. Therefore, net force on the cork is zero.
3. As the kite is skilfully held stationary in the sky, in accordance with first law of motion, the net force on the kite is zero.
4. As the car is moving with a constant velocity of 30 km/h on a road, the net force on the car is zero.
5. As the high-speed electron in space is far from all material objects, and free of electric and magnetic fields, it doesn’t accelerate and moves with constant velocity. Hence, net force acting on the electron is zero.

Question 6.
State Newton’s second law of motion and its importance.
Answer:
Statement: The rate of change of linear momentum of a rigid body is directly proportional to the applied (external unbalanced) force and takes place in the direction of force.
\(\overrightarrow{\mathrm{F}}=\frac{\mathrm{d} \overrightarrow{\mathrm{p}}}{\mathrm{dt}}\)
Where, \(\overrightarrow{\mathrm{F}}\) = Force applied
p = m\(\overrightarrow{\mathrm{v}}\) = linear momentum

Importance of Newton’s second law:

1. It gives mathematical formulation for quantitative measure of force as rate of change of linear momentum.
2. It defines momentum instead of velocity as the fundamental quantity related to motion.
3. It takes into consideration the resultant unbalanced force on a body which is used to overcome Aristotle’s fallacy.

Question 7.
Explain why a cricketer moves his hands backwards while holding a catch. (NCERT)
Answer:

1. In the act of catching the ball, by drawing hands backward, cricketer allows longer time for his hands to stop the ball.
2. By Newton’s second law of motion, force applied depends on the rate of change of momentum.
3. Taking longer time to stop the ball ensures smaller rate of change of momentum.
4. Due to this the cricketer can stop the ball by applying smaller amount of force and thereby not hurting his hands.

Question 8.
Large force always produces large change in momentum on a body than a small force. Is this correct?
Answer:
No. From Newton’s second law, we have.
\(\frac{\mathrm{dP}}{\mathrm{dt}}=\mathrm{F}\) …. (i)
dP = Fdt …. (ii)
From equation (ii), we can infer that a small force acting for a longer time can produce same change in momentum of a body as a large force acting in the same direction for a short time. Hence, the given statement is incorrect.

Question 9.
Newton’s first law is contained in the second law. Prove it.
Answer:

1. From Newton’s second law of motion, we have, \(\overrightarrow{\mathrm{F}}=\frac{\mathrm{d} \overrightarrow{\mathrm{p}}}{\mathrm{dt}}=\frac{\mathrm{d}}{\mathrm{dt}}(\mathrm{m} \overrightarrow{\mathrm{v}})\)
2. For a given body, mass m is constant.
   ∴ \(\overrightarrow{\mathrm{F}}=\mathrm{m} \frac{\mathrm{d} \overrightarrow{\mathrm{v}}}{\mathrm{dt}}=\mathrm{m} \overrightarrow{\mathrm{a}}\)
3. If \(\overrightarrow{\mathrm{F}}\) = zero, \(\overrightarrow{\mathrm{v}}\) is constant. Hence if there is no force, velocity will not change. This is nothing but Newton’s first law of motion.

Question 10.
State Newton’s third law of motion
Answer:
Statement: To every action (force) there is always an equal and opposite reaction force).

Question 11.
State the importance of Newton’s third law of motion.
Answer:

1. Newton’s third law of motion defines action and reaction as a pair of equal and opposite forces acting along the same line.
2. Action and reaction forces always act on different objects.

Question 12.
State the consequences of Newton’s third law of motion.
Answer:

1. Two interacting bodies exert forces which are always equal in magnitude, have the same line of action and are opposite in direction, upon each other. Thus, forces always occur in pairs.
2. If a body A exerts an action force \(\overrightarrow{\mathrm{F}}_{\mathrm{BA}}\) on body B, then body B also exerts an equal and opposite reaction force \(\overrightarrow{\mathrm{F}}_{\mathrm{AB}}\) on body A, simultaneously.
3. Body A experiences the force \(\overrightarrow{\mathrm{F}}_{\mathrm{AB}}\) only and
   body B experiences the force \overrightarrow{\mathrm{F}}_{\mathrm{BA}} only.
4. Both the forces, action and reaction act at the same instant.
5. Both the forces always act on different bodies. Hence, they never cancel each other.
6. Both the forces do not necessarily arise due to contact i.e., they can be non-contact forces. Example: Repulsive forces between two magnets.

Question 13.
If a constant force of 800 N produces an acceleration of 5 m/s² in a body, what is its mass? If the body starts from rest, how much distance will it travel in 10 s?
Solution:
Given: F = 800 N, a = 5 m/s², u = 0, t = 10 s
To find: mass (m), distance travelled (s)
Formulae:

i. F = ma
ii. s = ut + \(\frac{1}{2} \mathrm{at}^{2}\)

Calculation:
From formula (i),
∴ m = \(\frac{\mathrm{F}}{\mathrm{a}}=\frac{800}{5}\) = 160 kg
From formula (ii),
s = \(\frac{1}{2}\) × 5 × (10)² [∵ u = 0]
∴ s = 250 m
Answer:
Mass of the body is 160 kg and the distance travelled by the body is 250 m.

Question 14.
A constant force acting on a body of mass 3 kg changes its speed from 2 m s^-1 to 3.5 m/s in 25 s. The direction of motion of the body remains unchanged. What is the magnitude and direction of the force? (NCERT)
Solution:
Given: u = 2 ms^-1, m = 3 kg,
v = 3.5 m s^-1, t = 25s
To find: Force (F)
Formula: F = ma
Calculation: Since, v = u + at
∴ 3.5 = 2 + a × 25
a = \(\frac{3.5-2}{25}\) = 0.06 m s^-2
From formula,
F = 3 × 0.06 = 0.18 N
Since, the applied force increases the speed of the body, it acts in the direction of the motion.
Answer:
The applied force is 0.18 N along the direction of motion.

Question 15.
A constant retarding force of 50 N is applied to a body of mass 20 kg moving initially with a speed of 15 ms^-1. How long does the body take to stop? (NCERT)
Solution:
Given: m = 20 kg, u = 15ms^-1, v = 0,
F = – 50 N (retarding force)
To find: Time (t)
Formula: v = u + at
Calculation: Since, F = ma
∴ a = \(\frac{\mathrm{F}}{\mathrm{m}}=\frac{-50}{20}\) = -2.5 m s^-2
From formula,
0 = 15 + (-2.5) × t
∴ t = 6s
Answer:
Time taken to stop the body is 6 s.

Question 16.
A hose pipe used for gardening is ejecting water horizontally at the rate of 0.5 m/s. Area of the bore of the pipe is 10 cm2. Calculate the force to be applied by the gardener to hold the pipe horizontally stationary.
Solution:
Let ejecting water horizontally be considered as the action force on the water, then the water exerts a backward force (called recoil force) on the pipe as the reaction force.

Where, V = volume of water ejected
A = area of cross section of bore = 10 cm²
ρ = density of water = 1 g/cc
l = length of the water ejected in time t
\(\frac{\mathrm{d} l}{\mathrm{dt}}\) = v = velocity of water ejected
= 0.5 m/s = 50 cm/s
F = \(\frac{\mathrm{dm}}{\mathrm{dt}} \mathrm{v}\)
= (Aρv) v
= Aρv²
= 10 × 1 × 50²
∴ F = 25000 dyne = 0.25 N
Answer:
The gardener must apply an equal and opposite force of 0.25 N.

Question 17.
What does the term frame of reference mean?
Answer:
A system of co-ordinate axes with reference to which the position or motion of an object is described is called a frame of reference.

Question 18.
Explain the terms inertial and non-inertial frame of reference.
Answer:

1. Inertial frame of reference:
   • A frame of reference in which Newton ‘s first law of motion is applicable is called inertia/frame of reference.
   • A body moves with a constant velocity (which can be zero) in the absence of a net force. The body does not accelerate.
   • Example: A rocket in inter-galactic space (gravity free space between galaxies) with all its engine shut.
2. Non-inertial frame of reference:
   • A frame of reference in which an object suffers acceleration in absence of net force is called non-inertial frame of reference.
   • The body undergoes acceleration.
   • Example: If a car just start its motion from rest, then during the time of acceleration the car will be in a non-inertial frame of reference.

Question 19.
State the limitations of Newton’s laws of motion.
Answer:

1. Newton’s laws of motion are not applicable in a non-inertial (accelerated) frame of reference.
2. Newton’s laws are only applicable to point objects.
3. Newton’s laws are only applicable to rigid bodies.
4. Results obtained by applying Newton’s laws of motion for objects moving with speeds comparable to that of light do not match with the experimental results and Einstein special theory of relativity has to be used.
5. Newton’s laws of motion fail to explain the behaviour and interaction of objects having atomic or molecular sizes, and quantum mechanics has to be used.

Question 20.
Name the different types of fundamental forces in nature.
Answer:
Fundamental forces in nature are classified into four types:

1. Gravitational force
2. Electromagnetic force
3. Strong nuclear force
4. Weak nuclear force

Question 21.
Define gravitational force. Give its examples.
Answer:
Force of attraction between two (point) masses separated by a distance is called as gravitational force.
F = \(\mathrm{G} \frac{\mathrm{m}_{1} \mathrm{~m}_{2}}{\mathrm{r}^{2}}\)
where ‘G’ is constant called the universal gravitational constant = 6.67 × 10^-11 Nm²/kg²

Examples:

1. The motion of moon, artificial satellites around the earth and motion of planets around the sun is due to gravitational force of attraction.
2. The concept of weight of a body in the earth’s gravitational field is due to gravitational force exerted by the earth on a body.

Question 22.
Write down the main characteristics of gravitational force.
Answer:
Characteristics of gravitational force:

1. It is always attractive.
2. It is the weakest of the four basic forces in nature.
3. Its range is infinite.
4. Structure of the universe is governed by this force.

Question 23.
Write a note on electromagnetic (EM) force.
Answer:
Electromagnetic force:

1. The attractive and repulsive force between electrically charged particles is called electromagnetic force.
2. It can be attractive or repulsive.
3. It is stronger than the gravitational force.
4. Example: force of friction, normal reaction, tension in strings, collision forces, elastic forces, fluid friction etc. are electromagnetic in nature.
5. Reaction forces are a result of the action of electromagnetic forces.
6. Since majority of forces are electromagnetic in nature, our life is practically governed by these forces.

Question 24.
Write a note on strong and weak nuclear force.
Answer:

1. Strong nuclear force: The strong force which binds protons and neutrons (nucleons) together in the nucleus of an atom is called strong nuclear force.
   Characteristics of strong nuclear force:
   • It is a very strong attractive force.
   • It is a short range force of the order of 10^-14 m.
   • it is charge independent.
2. Weak nuclear force: The force of interaction between subatomic particles which results in the radioactive decay of atoms is called weak nuclear force.

Characteristics of weak nuclear force:

• It acts between any two elementary particles (pair of subatomic particles).
• It is a stronger force than gravitational force.
• It is much weaker than electromagnetic force or strong nuclear force.
• It is a short range force of the order of 10^-16m.

Question 25.
Three identical point masses are fixed symmetrically on the periphery of a circle. Obtain the resultant gravitational force on any point mass M at the centre of the circle. Extend this idea to more than three identical masses symmetrically located on the periphery. How far can you extend this concept?
Solution:

i. Consider three identical points A, B and C of mass m on the periphery of a circle of radius r. Mass M is at the centre of the circle.
Gravitational forces on M due to these masses are attractive and are given as,
In magnitude, \(\mathrm{F}_{\mathrm{MA}}=\mathrm{F}_{\mathrm{MB}}=\mathrm{F}_{\mathrm{MC}}=\frac{\mathrm{GMm}}{\mathrm{r}^{2}}\)

ii. Forces \(\overrightarrow{\mathrm{F}}_{\mathrm{MB}}\) and \(\overrightarrow{\mathrm{F}}_{\mathrm{MC}}\) are resolved along \(\overrightarrow{\mathrm{F}}_{\mathrm{MA}}\) and perpendicular to \(\overrightarrow{\mathrm{F}}_{\mathrm{MA}}\). Components perpendicular to \(\overrightarrow{\mathrm{F}}_{\mathrm{MA}}\) cancel each other. Components along \(\overrightarrow{\mathrm{F}}_{\mathrm{MA}}\) are,
F_MB cos 60° = F_MC cos 60° = \(\frac{1}{2} F_{M A}\) each.

Magnitude of their resultant is F_MA and its direction is opposite to that of F_MA. Thus, the
resultant force on mass M is zero. For any even number of equal masses, the force due to any mass m is balanced (cancelled) by diametrically opposite mass. For any odd number of masses, the components perpendicular to one of them cancel each other while the components parallel to one of these add up in such a way that the resultant is zero for any number of identical masses m located symmetrically on the periphery.

As the number of masses tends to infinity, their collective shape approaches circumference of the circle, which is nothing but a ring. Thus, the gravitational force exerted by a ring mass on any other mass at its centre is zero.

iii. This concept can be further extended to three-dimensions by imagining a uniform hollow sphere to be made up of infinite number of such rings with a common diameter. Thus, the gravitational force for any mass kept at the centre of a hollow sphere is zero.

Question 26.
A car of mass 1.5 ton is running at 72 kmph on a straight horizontal road. On turning the engine off, it stops in 20 seconds. While running at the same speed, on the same road, the driver observes an accident 50 m in front of him. He immediately applies the brakes and just manages to stop the car at the accident spot. Calculate the braking force.
Solution:
Given: m = 1.5 ton = 1500 kg,
u = 72 kmph = 72 × \(\frac{5}{18} \mathrm{~m} / \mathrm{s}\)m/s = 20 m
s^-1 (on turning engine off),
v = 0, t = 20 s, s = 50 m
To find: Braking force (F)

Formula:

i. v = u + at
ii. v² – u² = 2as
iii. F = ma

Calculation:
On turning the engine off,
From formula (i),
a = \(\frac{0-20}{20}\) = -1 m s^-2
This is frictional retardation (negative acceleration).
After seeing the accident,
From formula (ii),
a₁ = \(\frac{0^{2}-20^{2}}{2(50)}\) = -4 m s^-2
This retardation is the combined effect of braking and friction
∴ braking retardation =4 – 1 = 3 m s^-2
From formula (iii), the braking force, F = 1500 × 3 = 4500 N
Answer:
The braking force is 4500 N.

Question 27.
A body constrained to move along the z-axis of a coordinate system is subject to a constant force F given by \(\overrightarrow{\mathbf{F}}=(-\hat{\mathbf{i}}+2 \hat{\mathbf{j}}+3 \hat{\mathbf{k}})\) N where \(\hat{\mathbf{i}}\), \(\hat{\mathbf{j}}\), \(\hat{\mathbf{k}}\) are unit vectors along the x, y and z axis of the system respectively. What is the work done by this force in moving the body a distance of 4 m along the z-axis? (NCERT)
Solution:
Given: \(\overrightarrow{\mathbf{F}}=(-\hat{\mathbf{i}}+2 \hat{\mathbf{j}}+3 \hat{\mathbf{k}})\) N, \(\overrightarrow{\mathrm{s}}=4 \hat{\mathrm{k}}\)
To find: work done (W)

Formula: W = \(\overrightarrow{\mathrm{F}} \cdot \overrightarrow{\mathrm{s}}\)
Calculation: From formula,
W = \((-\hat{\mathrm{i}}+2 \hat{\mathrm{j}}+3 \hat{\mathrm{k}}) \cdot(4 \hat{\mathrm{k}})\)
= \(12 \hat{\mathrm{k}} \cdot \hat{\mathrm{k}}\) = 12 J
Answer:
The work done by the force is in moving the body 12 J.

Question 28.
Over a given region, a force (in newton) varies as F = 3x² – 2x + 1. In this region, an object is displaced from x₁ = 20 cm to x₂ = 40 cm by the given force. Calculate the amount of work done.
Solution:
Given: F = 3x² – 2x + 1, x₁ = 20 cm = 0.2 m,
x₂ = 40 cm = 0.4 m.
To find: Work done (W)
Formula: W = \(\int_{A}^{B} \vec{F} \cdot \overrightarrow{d s}\)
Calculation:
From formula,
W = \(\int_{x_{1}}^{x_{2}} F \cdot d x=\int_{0.2}^{0.4}\left(3 x^{2}-2 x+1\right) d x\)
= [x³ – x² + x]^0.4
= [0.4³ – 0.4² + 0.4] – [0.2³ – 0.2² + 0.2]
= 0.304 – 0.168 = 0.136 J
The work done is 0.136 J.

Question 29.
A position dependent force f = 7 – 2x + 3x² newton acts on a small body of mass 2 kg and displaces from x = 0 m to x = 5 m, calculate the work done.
Solution:
Given: F = 7 – 2x + 3x², x = 0 at A and x = 5 at B.
To find: Work done (W)
Formula: W = \(\int_{A}^{B} \vec{F} \cdot \overrightarrow{d s}\)
Calculation: From formula,

∴ W = 135 J
Answer:
The work done is 135 J.

Question 30.
State the principle of work-energy theorem in case of a conservative force and explain.
OR
Show that work done on a body by a conservative force is equal to the change in its kinetic energy
Answer:
Principle: Decrease in the potential energy due to work done by a conservative force is entirely converted into kinetic energy. Vice versa, for an object moving against a conservative force, its kinetic energy decreases by an amount equal to the work done against the force.

Work-energy theorem in case of a conservative force:

1. Consider an object of mass m moving with velocity u experiencing a constant opposing force F which slows it down to v during displacement s.
2. The equation of motion can be written as, v² – u² = -2as (negative acceleration for
   opposing force.)
   Multiplying throughout by \(\frac{\mathrm{m}}{2}\), we get,
   \(\frac{\mathrm{1}}{2}\)mu² – \(\frac{\mathrm{1}}{2}\)mv² = (ma)s …. (1)
3. According to Newton’s second law of motion,
   F = ma … (2)
4. From equations (1) and (2), we get,
   \(\frac{\mathrm{1}}{2}\)mu² – \(\frac{\mathrm{1}}{2}\)mv² = F.s
5. But, \(\frac{\mathrm{1}}{2}\)mv²= K_f = final K.E of the body,
   \(\frac{\mathrm{1}}{2}\)mu² = K_i = initial K.E of the body. and, work done by the force = F.s
   ∴ work done by the force = k_f – k_i
   = decrease in K.E of the body.
6. Thus, work done on a body by a conservative force is equal to the change in its kinetic energy.

Question 31.
Explain the work-energy theorem in case of an accelerating conservative force along with a retarding non-conservative force.
Answer:

1. Consider an object dropped from some point at height h.
2. While coming down its potential energy decreases.
   ∴ Work done = decrease in P.E of the body.
3. But, in this case, some part of the energy is used in overcoming the air resistance. This part of energy appears in some other forms such as heat, sound, etc. Thus, the work is not entirely converted into kinetic energy. In this case, the work-energy theorem can mathematically be written as,
   ∴ ∆ PE = ∆ K.E. + W_{air resistance}
   ∴ Decrease in the gravitational P.E. = Increase in the kinetic energy + work done against non-conservative forces.

Question 32.
A liquid drop of 1.00 g falls from height of cliff 1.00 km. It hits the ground with a speed of 50 m s^-1. What is the work done by the unknown force? (Take g = 9.8 m/s²)
Solution:
Given: m = 1.0 g = 1.0 × 10^-3 kg,
h = 1 km = 10³ m, v = 50 ms^-1
To find: Work done (W_f)
Formula: W_f = ∆ K.E – W_g

Calculation:

i. The change in kinetic energy of the drop
∆ K.E = (K.E.)_final (K.E.)_initial
∴ ∆ K.E. = \(\frac{1}{2} \mathrm{mv}^{2}-0\)
= \(\frac{1}{2} \times 1.0 \times 10^{-3} \times(50)^{2}\)
∴ ∆ K.E.= 1.25 J

ii. Work done by the gravitational force is,
W_g = mgh = 1.0 × 10^-3 × 9.8 × 10³ = 9.8 J
∴ W_g = 9.8J
From formula,
W_f = ∆K.E. – W_g = 1.25 – 9.8
W_f = -8.55 J
Answer:
Work done by the unknown force is – 8.55 J.

Question 33.
A body of mass 0.5 kg travels in a straight line with velocity y = ax^3/2, where a = 5 m^1/2s^-1. What is the work done by the net force during its displacement from x = 0 to x = 2m? (NCERT)
Solution:
Given: M = 0.5 kg, y = ax^3/2,
where a = 5 m^-1/2s^-1
Let v₁ and v₂ be the velocities of the body, when x = 0 and x = 2 m respectively. Then,
v₁ = 5 × 0^3/2 = 0, v₂ = 5 × 2^3/2 = \(10 \sqrt{2}\) m
To find: Work done (W)
Formula: Work done = Increase in kinetic energy
W = \(\frac{1}{2} \mathrm{M}\left(\mathrm{v}_{2}^{2}-\mathrm{v}_{1}^{2}\right)\)
Calculation: From formula,
W = \(\frac{1}{2}\) × 0.5 × [latex](10 \sqrt{2})^{2}-0^{2}[/latex]
∴ W = 50J
Answer:
Work done by the net force on the body is 50 J.

Question 34.
A particle of mass 12 kg is acted upon by a force f = (100 – 2x²) where f is in newton and ‘x’ is in metre. Calculate the work done by this force in moving the particle x = 0 to x = -10 m. What will be the speed at x = 10 m if it starts from rest?
Solution:
Given: F = 100 – 2x²
at A, x = 0 and at B, x = -10 m
To find: Work done (W), speed (v)

Formulae:

i. W = \(\int_{A}^{B} \vec{F} \cdot d s\)
ii. W = K.E. = \(\frac{1}{2} \mathrm{mv}^{2}\)

Calculation:
From formula (i),
W = \(\int_{A}^{B} \vec{F} \cdot \overline{d s}=\int_{x=0}^{x=-10} F d x\)

Answer:

1. Work done by the force on the particle is 333.3 J.
2. The speed of the particle at x = 10 will be 7.45 m/s.

Question 35.
Define free body diagram. In the figure given below, draw the free body diagrams for mass of 2 kg, 4 kg and 5 kg and hence state their force equations.

Answer:
i. The diagram showing the forces acting on only one body at a time along-with its acceleration is called a free body diagram.

ii. The free body diagram for the mass of 2 kg is

Free body diagram for 2 kg mass
The force equation is given as,
2a = T₃ – 2g

iii. The free body diagram for the mass of 4 kg is,

The force equation is given as, 4a = T₁ + 4g – T₂

iv. The free body diagram for the mass of 5kg is,

The force equation for the mass of 5 kg is given as,
N + F sin 60° = 5g, along the vertical direction.
T₁ + 10 = F cos 60°, along the horizontal direction (Considering the mass is in equilibrium).

Question 36.
Figure shows a fixed pulley. A massless inextensible string with masses m₁ and m₂ > m₁ attached to its two ends is passing over the pulley. Such an arrangement is called an Atwood machine. Calculate accelerations of the masses and force due to the tension along the string assuming axle of the pulley to be frictionless.

Solution:
Method I: As m₂ > m₁, mass m₂ is moving downwards and mass m₁ is moving upwards.
Net downward force = F = (m₂) g – (m₁) g
= (m₂ – m₁)g
the string being inextensible, both the masses travel the same distance in the same time. Thus, their accelerations are equal in magnitude (one upward, other downward). Let it be a.
Total mass in motion, M = m₂ + m₁
∴ a = \(\frac{F}{M}=\left(\frac{m_{2}-m_{1}}{m_{2}+m_{1}}\right) g\) …. (i)

For mass m₁, the upward force is the force due to tension T and downward force is mg. It has upward acceleration a. Thus, T – m₁g = m₁a
∴ T = m₁(g + a)
Using equation (i), we get,

From the free body equation for the first body,
T – m₁g = m₁a .. (i)

From the free body equation for the second body,
m₂g – T = m₂a … (ii)
Adding (i) and (ii), we get,
a = \(\left(\frac{\mathrm{m}_{2}-\mathbf{m}_{1}}{\mathrm{~m}_{2}+\mathrm{m}_{1}}\right) \mathbf{g}\) ….(iii)
Solving equations. (ii) and (iii) for T, we get,
T = m₂(g – a) = \(\left(\frac{2 m_{1} m_{2}}{m_{1}+m_{2}}\right) g\)

Question 37.
Write a note on elastic collision.
Answer:

1. Collision between two bodies in which kinetic energy of the entire system is conserved along with the linear momentum is called as elastic collision.
2. In an elastic collision,
   \(\mathrm{m}_{1} \overrightarrow{\mathrm{u}_{1}}+\mathrm{m}_{2} \overrightarrow{\mathrm{u}_{2}}=\mathrm{m}_{1} \overrightarrow{\mathrm{v}_{1}}+\mathrm{m}_{2} \overrightarrow{\mathrm{v}}_{2}\)
3. In an elastic collision,
   \(\sum \mathrm{K} \cdot \mathrm{E}_{\text {‘initial }}=\sum \mathrm{K} \cdot \mathrm{E}_{\text {. final }}\)
4. An elastic collision is impossible in daily life.
5. However, in many situations, the interatomic and intermolecular collisions are considered to be elastic.

Question 38.
Write a note on inelastic collision.
Answer:

1. A collision is said to be inelastic if there is a loss in the kinetic energy during collision, but linear momentum is conserved.
2. In an inelastic collision, m₁u₁ + m₂u₂ = m₁v₁ + m₂v₂.
3. In an inelastic collision,
   \(\sum \mathrm{K} \cdot \mathrm{E}_{\text {.initial }} \neq \sum \mathrm{K} \cdot \mathrm{E}_{\text {.final }}\)
4. The loss in kinetic energy is either due to internal friction or vibrational motion of atoms causing heating effect.

Question 39.
Define perfectly inelastic collision. Give an example of it.
Answer:

1. Collision in which the colliding bodies stick together after collision and move with a common velocity is called perfectly inelastic collision.
2. The loss in kinetic energy is maximum in perfectly elastic collision.
3. Example: Lump of mud thrown on a wall sticks to the wall due to the loss of kinetic energy.

Question 40.
In case of an elastic head on collision between two bodies, derive an expression for the final velocities of the bodies in terms of their masses and velocities before collision.
Answer:
Head on elastic collision of two spheres:

i. Consider two rotating smooth bodies A and B of masses m1 and m2 respectively moving
along the same straight line.

ii. Let \(\overrightarrow{\mathrm{u}}_{1}\) = initial velocity of the sphere A before collision.
\(\overrightarrow{\mathrm{u}}_{2}\) = initial velocity of the sphere B before collision.
\(\overrightarrow{\mathrm{v}}_{1}\) = velocity of the sphere A after collision.
\(\overrightarrow{\mathrm{v}}_{2}\) = velocity of the sphere B after collision.

iii. After the elastic collision, the spheres separate and move along the same straight line without rotation.

iv. According to the law of conservation of momentum,
m₁\(\overrightarrow{\mathrm{u}}_{1}\) + m₂\(\overrightarrow{\mathrm{u}}_{2}\) = m₁\(\overrightarrow{\mathrm{v}}_{1}\) + m₂\(\overrightarrow{\mathrm{v}}_{2}\) ….(i)
According to the law of conservation of energy (as kinetic energy is conserved during elastic collision),

v. Since kinetic energy is a scalar quantity, the terms involved in the above equations are scalars.

vi. The equation (1) can be written in scalar form as,

vii. Also the equation (2) can be written as,

viii. Now dividing equation (4) by (3) we get,
(u₁ + v₁) = (u₂ + v₂)
∴ u₁ + v₁ = u₂ + v₂
∴ v₂ = u₁ – u₂ + v₁ … (5)

ix. Comparing equation (3) and (5),

Equations, (6) and (7), represent the final velocities of two spheres after collision.

Question 41.
Are you aware of elasticity of materials? Is there any connection between elasticity of materials and elastic collisions?
Answer:
(Students should answer the question as per their understanding).

Question 42.
Two bodies undergo one-dimensional, inelastic, head-on collision. State an expression for their final velocities in terms
of their masses, initial velocities and coefficient of restitution.
Answer:
If e is the coefficient of restitution, the final velocities after an inelastic, head on collision are given as,

Question 43.
Two bodies undergo one-dimensional, inelastic, head-on collision. State an expression for the loss in the kinetic energy.
Answer:

ii. As e < 1, (1 – e²) is always positive. Thus, there is always a loss of kinetic energy in an inelastic collision.

Question 44.
Two bodies undergo one-dimensional, inelastic, head-on collision. Obtain an expression for the magnitude of impulse.
Answer:
i. When two bodies undergo collision, the linear momentum delivered by the first body to the second body must be equal to the change in momentum or impulse of the second body and vice versa.
∴ Impulse,
|J| = |∆p₁| = |∆p₂|
= |m₁v₁ – m₁u₁| = |m₂v₂ – m₂u₂| ….(1)

In equation (1) and solving, we get,

Question 45.
Two bodies undergo one-dimensional, perfectly inelastic, head-on collision. Derive an expression for the loss in the kinetic energy.
Answer:
i. Let two bodies A and B of masses m₁ and m₂ move with initial velocity \(\overrightarrow{\mathrm{u}}_{1}\) and \(\overrightarrow{\mathrm{u}}_{2}\), respectively such that particle A collides head on with particle B i.e., u₁ > u₂.

ii. If the collision is perfectly inelastic, the particles stick together and move with a common velocity \(\overrightarrow{\mathrm{v}}\) after the collision along the same straight line.
loss in kinetic energy = total initial kinetic energy – total final kinetic energy.

iii. By the law of conservation of momentum,
m₁u₁ + m₂u₂ = (m₁ + m₂)v
∴ v = \(\frac{\mathrm{m}_{1} \mathrm{u}_{1}+\mathrm{m}_{2} \mathrm{u}_{2}}{\mathrm{~m}_{1}+\mathrm{m}_{2}}\)

iv. Loss of Kinetic energy,

iv. Both the masses and the term (u₁ – u₂)² are positive. Hence, there is always a loss in a perfectly inelastic collision. For a perfectly inelastic collision, as e = 0, the loss is maximum.

Question 46.
Distinguish between elastic and inelastic collision.
Answer:

No.	Elastic Collision	Inelastic Collision
i.	In an elastic collision, both momentum and kinetic energy are conserved.	In an inelastic collision, momentum is conserved but kinetic energy is not conserved.
ii.	The total kinetic energy after collision is equal to the total kinetic energy before collision.	The total kinetic energy after the collision is not equal to the total kinetic energy before collision.
iii.	Coefficient of restitution (e) is equal to one.	Coefficient of restitution (e) is less than one. For a perfectly inelastic collision coefficient of restitution is equal to zero.
iv.	Bodies do not stick together in elastic collision.	Bodies stick together in a perfectly inelastic collision.
v.	Sound, heat and light are not produced.	Sound or light or heat or all of these may be produced.

Question 47.
Explain elastic collision in two dimensions.
Answer:
i. Suppose a particle of mass mi moving with initial velocity \(\overrightarrow{\mathrm{u}_{1}}\), undergoes a non head-on collide with another particle of mass m₂ and initial velocity \(\overrightarrow{\mathrm{u}_{2}}\).

ii. Let us consider two mutually perpendicular directions; Common tangent at the point of impact, along which there is no force (or no change of momentum).
Line of impact which is perpendicular to the common tangent through the point of impact, in the two-dimensional plane of initial and final velocities.

iii. Applying the law of conservation of linear momentum along the line of impact, we have, m₁u₁ cos α₁ + m₂u₂ cos α₂ = m₁v₁ cos β₁ + m₂v₂ cos β₂
As there is no force along the common tangent,
m₁u₁ sin α₁ = m₁u₁ sin β₁ and m₂u₂ sin α₂ = m₂v₂ sin β₂
iv. Coefficient of restitution (e) along the line of impact is given as

Question 48.
Two bodies undergo a two-dimensional collision. State an expression for the magnitude of impulse along the line of impact and the loss in kinetic energy.
Answer:
i. For two bodies undergoing a two-dimensional collision, the magnitude of impulse along the line of impact is given as, Magnitude of the impulse, along the line of impact,

ii. The loss in the kinetic energy is given as Loss in the kinetic energy = ∆ (K.E.)

Question 49.
0ne marble collides head-on with another identical marble at rest. If the collision is partially inelastic, determine the ratio of their final velocities in terms of coefficient of restitution e.
Solution:
According to conservation of momentum,
m₁u₁ + m₂u₂ = m₁v₁ + m₂v₂
As m₁ = m₂, we get, u₁ + u₂ = v₁ + v₂
∴ If u₂ = 0, we get, v₁ + v₂ = u₁ ….. (i)
Coefficient of restitution,

Question 50.
A 10 kg mass moving at 5 m/s collides head- on with a 4 kg mass moving at 2 m/s in the same direction. If e = \(\frac{1}{2}\), find their velocity after impact.
Solution:
Given: m₁ = 10 kg, m₂ = 4 kg
u₁ = 5 m/s, u₂ = 2 m/s, e = \(\frac{1}{2}\)
To find: Velocity after impact (v₁ and v₂)

Formulae:

i. m₁u₁ + m₂u₂ = m₁v₁ + m₂v₂
ii. e = \(\left(\frac{v_{2}-v_{1}}{u_{1}-u_{2}}\right)\)

Calculation:

From formula (i),
10 × 5 + 4 × 2 = 10v₁ + 4v₂
∴ 5v₁ + 2v₂ = 29 … (1)

From formula (ii),
v₂ – v₁ = e(u₁ – u₂) = \(\frac{1}{2}\) (5 – 2) = \(\frac{3}{2}\)
∴ 2v₂ – 2v₁ = 3 … (2)
Solving (1) and (2), we have
∴ v₁ = \(\frac{26}{7}\) m/s and v₂ = \(\frac{73}{14}\) m/s
Answer:
The respective velocities of the two masses are \(\frac{26}{7}\) m/s and \(\frac{73}{14}\) m/s.

Question 51.
A metal ball falls from a height 1 m on a steel plate and jumps upto a height of 0.81 m. Find the coefficient of restitution.
Solution:
As the ball falls to the steel plate P.E changes to kinetic energy.

As ground is stationary, both its initial and final velocities are zero.

Question 52.
Two bodies of masses 5 kg and 3 kg moving in the same direction along the same straight line with velocities 5 m s^-1 and 3 m s^-1 respectively suffer one-dimensional elastic collision. Find their velocities after the collision.
Solution:
Given: m₁ = 5kg, u₁ = 5ms^-1, m₂ = 3kg, u₂ = 3 m s^-1
To find: velocities after collision (v₁ and v₂)

Answer:
The velocities of the two bodies after collision are 3.5 m/s and 5.5 m/s.

Question 53.
A 20 g bullet leaves a machine gun with a velocity of 200 m/s. If the mass of the gun is 20 kg, find its recoil velocity. If the gun fires 20 bullets per second, what force is to be applied to the gun to prevent recoil?
Solution:
Given: m₁ = 20g = 0.02 kg, m₂ = 20 kg, v₁ = 200 m/s, t = \(\frac{1}{20}\) s,
To find: Recoil velocity (v₂), applied force (F)

Formulae:

i. v₂ = \(-\frac{\mathrm{m}_{1} \mathrm{v}_{1}}{\mathrm{~m}_{2}}\)
ii. F = ma

Calculation: From formula (i),
∴ v₂ = \(-\frac{0.02}{20} \times 200\)
= -0.2 m/s

Negative sign shows that the machine gun moves in a direction opposite to that of the bullet.

From formula (ii),
∴ F = m₂ × a = 20 × 4 = 80N
Answer:
The recoil velocity of gun is 0.2 m/s and the required force to prevent recoil is 80 N.

Question 54.
A shell of mass 3 kg is dropped from some height. After falling freely for 2 seconds, it explodes into two fragments of masses 2 kg and 1 kg. Kinetic energy provided by the explosion is 300 J. Using g = 10 m/s², calculate velocities of the fragments. Justify your answer if you have more than one options.
Solution:
Total mass = m₁ + m₂ = 3 kg
Initially, when the shell falls freely for 2 seconds, v = u+ at = 0 + 10(2) = 20 ms^-1 = u₁ = u₂
According to conservation of linear momentum,
m₁u₁ + m₂u₂ = m₁v₁ + m₂v₂

There are two possible answers since the positions of two fragments can be different as explained below.
Case 1: v₁ = 30 m s^-1 and v₂ = 0 with the lighter fragment 2 above.
Case 2: v₁ = 10 m s^-1 and v₂ = 40 m s^-1 with the lighter fragment 2 below, both moving downwards.

Question 55.
Bullets of mass 40 g each, are fired from a machine gun at a rate of 5 per second towards a firmly fixed hard surface of area 10 cm². Each bullet hits normal to the surface at 400 m/s and rebounds in such a way that the coefficient of restitution for the collision between bullet and the surface is 0.75. Calculate average force and average pressure experienced by the surface due to this firing.
Solution:
For the collision,
u₁ = 400 m s^-1, e = 0.75
For the firmly fixed hard surface, u₂ = v₂ = 0
e = 0.75 = \(\frac{v_{1}-v_{2}}{u_{2}-u_{1}}=\frac{v_{1}-0}{0-400}\)
∴ v₁ = -300 m/s.
Negative sign indicates that the bullet rebounds in exactly opposite direction.
Change in momentum of each bullet = m(v₁ – u₁)
The same momentum is transferred to the surface per collision in opposite direction.
∴ Momentum transferred to the surface, per collision,
p = m (u₁ – v₁) = 0.04(400 – [-300]) = 28 Ns
The rate of collision is same as rate of firing.
∴ Momentum received by the surface per second, \(\frac{\mathrm{dp}}{\mathrm{dt}}\) = average force experienced by the surface = 28 × 5 = 140 N

This is the average force experienced by the surface of area A = 10 cm² = 10^-3 m²
∴ Average pressure experienced,
P = \(\frac{\mathrm{F}}{\mathrm{A}}=\frac{140}{10^{-3}}\) = 1.4 × 10⁵ N m^-2
∴ P ≈ 1.4 times the atmospheric pressure.
Answer:
The average force and average pressure experienced by the surface due to the firing is 140 N and 1.4 × 10⁵ N m^-2 respectively.

Question 56.
A shell of mass 0.020 kg is fired by a gun of mass 100 kg. If the muzzle speed of the shell is 80 m s^-1, what is the recoil speed of the gun? (NCERT)
Solution:
Given: m₁ = 0.02 kg, m₂ = 100 kg, v₁ = 80 m s^-1
To find: Recoil speed (v₂)
Formula: m₁u₁ + m₂u₂ = m₁v₁ + m₂v₂
Calculation: Initially gun and shell are at rest.
∴ m₁u₁ + m₂u₂ = 0
Final momentum = m₁v₁ – m₂v₂
Using formula,
0 = 0.02 (80) – 100(v₂)
∴ v₂ = \(\frac{0.02 \times 80}{100}\) = 0.016 ms^-1
Answer:
The recoil speed of the gun is 0.016 m s^-1.

Question 57.
Two billiard balls each of mass 0.05 kg moving in opposite directions with speed 6 m s^-1 collide and rebound with the same speed. What is the impulse imparted to each bail due to the other? (NCERT)
Solution:
Given: m = 0.05 kg. u = 6 m/s, v = -6 m/s
To find: Impulse (J)
Formula: J = m (v – u)
calculation: From formula,
J = 0.05 (-6 – 6) = -0.6 kg m s^-1
Answer:
Impulse received by each ball is -0.6 kg m s^-1.

Question 58.
A bullet of mass 0.1 kg moving horizontally with a velocity of 20 m/s strikes a target and brought to rest in 0.1 s. Find the impulse and average force of impact.
Solution:
Given: m = 0.1 kg, u = 20 m/s, t = 0.1 s
To find: Impulse (J), Average force (F)

Formulae:

i. J = mv – mu
ii. F = \(m \frac{(v-u)}{t}\)

Calculation:

From formula (i).
J = m(v – u) = 0.1 (0 – 20) = -2 Ns
From formula (ii),
F = \(\frac{m(v-u)}{t}=\frac{2}{0.1}=20 N\)
Answer:
Magnitude of impulse is 2 Ns, average force of impact is 20 N.

Question 59.
A batsman deflects a ball by an angle of 45° without changing its initial speed which is equal to 54 km/h. What is the impulse imparted to the ball? (Mass of the ball is 0.15 kg) (NCERT)
Answer:
Let the point B represents the position of bat. The ball strikes the bat with velocity v along the path AB and gets deflected with same velocity along BC. such that ∠ABC = 45°


Thus, impulse imparted to the ball is 4.157 kg ms^-1

Question 60.
A cricket ball of mass 150 g moving with a velocity of 12 m/s is turned back with a velocity of 20 m/s on hitting the bat. The force of the ball lasts for 0.01 s. Find the average force exerted on the ball by the bat.
Solution:
m = 0.150 kg, v = 20 m/s,
u = -12 m/s and t = 0.01 s
To find: Average force (F)
Formula: F = \(\frac{m(v-u)}{t}\)
Calculation: From formula,
F = \(\frac{0.150[20-(-12)]}{0.01}\) = 480 N
Answer:
The average force exerted on the ball by the bat is 480 N.

Question 61.
Mass of an Oxygen molecule is 5.35 × 10^-26 kg and that of a Nitrogen molecule is 4.65 × 10^-26 kg. During their Brownian motion (random motion) in air, an Oxygen molecule travelling with a velocity of 400 m/s collides elastically with a nitrogen molecule travelling with a velocity of 500 m/s in the exactly opposite direction. Calculate the impulse received by each of them during collision. Assuming that the collision lasts for
1 ms, how much is the average force experienced by each molecule?
Solution:
Let, m₁ = m_O = 5.35 × 10^-26 kg,
m₂ = m_N = 4.65 × 10^-26 kg,
∴ u₁ = 400 ms^-1 and u₂ = -500 ms_-1 taking direction of motion of oxygen molecule as the positive direction.
For an elastic collision,


Hence, the net impulse or net change in momentum is zero.

Answer:
The average force experienced by the nitrogen molecule and the oxygen molecule are
-4.478 × 10^-20 N and 4.478 × 10^-20 N.

Question 62.
Explain rotational analogue of the force. On what factors does it depend? Represent it in vector form.
Answer:

1. Rotational analogue of the force is called as moment of force or torque.
2. It depends on the mass of the object, the point of application of the force and the angle between direction of force and the line joining the axis of rotation with the point of application.
3. In its mathematical form, torque or moment of a force is given by
   \(\vec{\tau}=\overrightarrow{\mathrm{r}} \times \overrightarrow{\mathrm{F}}\)
   where \(\overrightarrow{\mathrm{F}}\) is the applied force and \(\overrightarrow{\mathrm{r}}\) is the position vector of the point of application of the force from the axis of rotation.

Question 63.
Illustrate with an example how direction of the torque acting on any object is determined.
Answer:
i. Consider a laminar object with axis of rotation perpendicular to it and passing through it as shown in figure (a).

ii. Figure (b) indicates the top view of the object when the rotation is in anticlockwise direction

iii. Figure (c) shows the view from the top, if rotation is in clockwise direction.

iv. The applied force \(\vec{F}\) and position vector \(\vec{r}\) of the point of application of the force are in the plane of these figures.

v. Direction of the torque is always perpendicular to the plane containing the vectors \(\vec{r}\) and \(\vec{F}\) and can be obtained from the rule of cross product or by using the right-hand thumb rule.

vi. In Figure (b), it is perpendicular to the plane of the figure and outwards while in the figure (c), it is inwards.

Question 64.
State the equation for magnitude of torque and explain various cases of angle between the direction of \(\overrightarrow{\mathbf{r}}\) and \(\overrightarrow{\mathbf{F}}\).
Answer:
Magnitude of torque, \(\tau\) = r F sin θ
where θ is the smaller angle between the directions of \(\overrightarrow{\mathbf{r}}\) and \(\overrightarrow{\mathbf{F}}\).

Special cases:

1. If θ = 90°, \(\tau\) = \(\tau\)_max = rF. Thus, the force should be applied along normal direction for easy rotation.
2. If θ = 0° or 180°, \(\tau\) = \(\tau\)_min = 0. Thus, if the force is applied parallel or anti-parallel to \(\overrightarrow{\mathrm{r}}\), there is no rotation.
3. Moment of a force depends not only on the magnitude and direction of the force, but also on the point where the force acts with respect to the axis of rotation. Same force can have different torque as per its point of application.

Question 65.
A force \(\overrightarrow{\mathbf{F}}=\mathbf{3} \hat{\mathbf{i}}+\hat{\mathbf{j}}-\mathbf{4} \hat{\mathbf{k}}\) is applied at a point (3, 4, -2). Find its torque about the point (-1, 2, 4).
Solution:

Question 66.
Define couple. Show that moment of couple is independent of the points of application of forces.
Answer:
A pair of forces consisting of two forces of equal magnitude acting in opposite directions along different lines of action is called a couple.

1. Figure shows a couple consisting of two forces \(\overrightarrow{\mathrm{F}}_{1}\) and \(\overrightarrow{\mathrm{F}}_{2}\) of equal magnitudes and opposite directions acting along different lines of action separated by a distance r.
2. Position vector of any point on the line of action of force \(\overrightarrow{\mathrm{F}}_{1}\) from the line of action of force \(\overrightarrow{\mathrm{F}}_{2}\) is \(\overrightarrow{\mathrm{r}}_{12}\). Similarly, the position vector of any point on the line of action of force \(\overrightarrow{\mathrm{F}}_{2}\) from the line of action of force \(\overrightarrow{\mathrm{F}}_{1}\) is \(\overrightarrow{\mathrm{r}}_{21}\).
3. Torque or moment of the couple is then given mathematically as
   
4. From the figure, it is clear that r₁₂ sinα = r₂₁ sin β = r.
5. If \(\left|\overrightarrow{\mathrm{F}}_{1}\right|=\left|\overrightarrow{\mathrm{F}}_{2}\right|\) = F, the magnitude of torque is given by
   \(\tau=\mathrm{r}_{12} \mathrm{~F}_{1} \sin \alpha\) = \(r_{21} F_{2} \sin \beta=r F\)
6. It clearly shows that the torque corresponding to a given couple, i.e., the moment of a given couple is constant, i.e., it is independent of the points of application of forces.

Question 67.
The figure below shows three situations of a ball at rest under the action of balanced forces. Is the bail in mechanical equilibrium? Explain how the three situations differ.

Answer:

1. In all these cases, as the ball is at rest under the action of balanced forces i.e, there is no net force acting on it. Hence, it is in mechanical equilibrium.
   However, potential energy-wise, the three situations show the different states of mechanical equilibrium.
2. Stable equilibrium: In situation (a), potential energy of the system is at its local minimum. If it is disturbed slightly from its equilibrium position and released, it tends to recover its position. In this situation, the ball is most stable and is said to be in stable equilibrium.
3. Unstable equilibrium: In situation (b), potential energy of the system is at its local maximum. If it is slightly disturbed from its equilibrium position, it moves farther from that position. This happens because initially, if disturbed, it tries to achieve the configuration of minimum potential energy. In this situation, the ball is said to be in unstable equilibrium.
4. Neutral equilibrium: in situation (e), potential energy of the system is constant over a plane and remains same at any position. Thus, even if the ball is disturbed, it still remains in equilibrium, practically at any position. In this situation, the ball is in neutral equilibrium.

Question 68.
Two weights 5 kg and 8 kg are suspended from a uniform rod of length 10 m and weighing 3 kg. The distances of the weights from one end are 2 m and 7 m. Find the point at which the rod balances.
Solution:

Let the rod balance at a point P, x m from the end A of the rod AB. The suspended weight and the centre of gravity of the rod G is shown in the figure.
P = 5 + 3 + 8 = 16kg
Taking moments about A,
16 × x = 5 × 2 + 3 × 5 + 8 × 7
16x = 10 + 15 + 56 = 81
∴ x = \(\frac{81}{16}\) = 5.1 m
Answer:
The rod balances at 5.1 m from end A.

Question69.
A metre stick is balanced on a knife edge at its centre. When two coins, each of mass 5 g are put one on top of the other at the 12.0 cm mark, the stick is found to be balanced at 45.0 cm. What is the mass of the metre stick? (NCERT)

Consider the metre scale AB. Let its mass be concentrated at C at 50 cm. mark. Upon placing coins, balancing point of the scale and the coins system is shifted to C’ at 45 cm mark.
For equilibrium about C’
10(45 – 12) = m (50 – 45)
m = \(\frac{10 \times 33}{5}\) = 66g
Answer:
The mass of the metre scale is 66 g.

Question 70.
The diagram shows a uniform beam of length 10 m, used as a balance. The beam is pivoted at its centre. A 5.0 N weight is attached to one end of the beam and an empty pan weighing 0.25 N Is attached to the other end of the beam.

i. What is the moment of couple at pivot?
ii. If pivot is shifted 2 ni towards left, then what will be moment of couple at new position?
Answer:

Answer:

1. The moment of couple at center is 23.75 N-m.
2. The moment of couple at new position is 13.25 N-m.
   ∴ For equilibrium,
   40 × x = 20 × 0.5
   ∴ x = \(\frac{1}{4}\) = 0.25 m

Hence, the total distance walked by the person is 1.25 m.

Ans
The person can walk 1.25 m before the plank topples.

Question 71.
Define centre of mass.
Answer:
Centre of mass of a body is a point about which the summation of moments of masses in the system is zero.

Question 72.
Derive an expression for the position of centre of mass of a system of n particles and for continuous mass distribution.
Answer:
System of n particles;
i. Consider a system of n particles of masses m₁, m₂, …, m_n having position vectors \(\overrightarrow{\mathrm{r}_{1}}\), \(\overrightarrow{\mathrm{r}_{2}}\),….., \(\overrightarrow{\mathrm{r}_{n}}\) from the origin O.
The total mass of the system is,

Centre of mass for n particles

ii. Position vector \(\vec{r}\) of their centre of mass from the same origin is then given by

iii. If the origin is at the centre of mass, \(\overrightarrow{\mathbf{r}}\) = 0
∴ \(\sum_{1}^{\mathrm{n}} \mathrm{m}_{\mathrm{i}} \overrightarrow{\mathrm{r}}_{\mathrm{i}}\) = 0,

iv. In this case, \(\sum_{1}^{n} m_{i} \vec{r}_{i}\) gives the moment of masses (similar to moment of force) about the centre of mass.

v. If (x₁, x₂, …… x_n), (y₁, y₂, …..y_n), (z₁, z₂, …. z_n) are the respective x, y and z – coordinates of (r₁, r₂,…….. r_n) then x,y and z – coordinates of the centre of mass are given by,

vi. Continuous mass distribution: For a continuous mass distribution with uniform density, the position vector of the centre of mass is given by,
r = \(\frac{\int \vec{r} d m}{\int d m}=\frac{\int \vec{r} d m}{M}\)
Where \(\int \mathrm{dm}=\mathrm{M}\) is the total mass of the object.

vii. The Cartesian coordinates of centre of mass are

Question 73.
State the expression for velocity of the centre of mass of a system of n particles and for continuous mass distribution.
Answer:
Let v₁, v₂,…..v_n be the velocities of a system of point masses m₁, m₂, … m_n. Velocity of the centre of mass of the system is given by

x, y and z components of \(\overrightarrow{\mathbf{v}}\) can be obtained similarly.
For continuous distribution, \(\overrightarrow{\mathrm{v}}_{\mathrm{cm}}\) = \(\frac{\int \vec{v} \mathrm{dm}}{\mathrm{M}}\)

Question 74.
State the expression for acceleration of the centre of mass of a system of n particles and for continuous mass distribution.
Answer:
Let a₁, a₂,…. a_n be the accelerations of a system of point masses m₁, m₂ … m_n.
Acceleration of the centre of mass of the system is given by

x, y and z components of can be obtained similarly.
For continuous distribution, \(\overrightarrow{\mathrm{a}}_{\mathrm{cm}}\) = \(\frac{\int \vec{a} d m}{\mathrm{M}}\)

Question 75.
State the characteristics of centre of mass.
Answer:

1. Centre of mass is a hypothetical point at which entire mass of the body can be assumed to he concentrated.
2. Centre of mass is a location, not a physical quantity.
3. Centre of mass is particle equivalent of a given object for applying laws of motion.
4. Centre of mass is the point at which applied force causes only linear acceleration and not angular acceleration.
5. Centre of mass is located at the centroid, for a rigid body of uniform density.
6. Centre of mass is located at the geometrical centre, for a symmetric rigid body of uniform density.
7. Location of centre of mass can be changed only by an external unbalanced force.
8. Internal forces (like during collision or explosion) never change the location of centre of mass.
9. Position of the centre of mass depends only upon the distribution of mass, however, to describe its location we may use a coordinate system with a suitable origin.
10. For a system of particles, the centre of mass need not coincide with any of the particles.
11. While balancing an object on a pivot, the line of action of weight must pass through the centre of mass and the pivot. Quite often, this is an unstable equilibrium.
12. Centre of mass of a system of only two particles divides the distance between the particles in an inverse ratio of their masses, i.e., it is closer to the heavier mass.
13. Centre of mass is a point about which the summation of moments of masses in the system is zero.
14. If there is an axial symmetry for a given object, the centre of mass lies on the axis of symmetry.
15. If there are multiple axes of symmetry for a given object, the centre of mass is at their point of intersection.
16. Centre of mass need not be within the body.
    Example: jumper doing fosbury flop.

Question 76.
The mass of moon is 0.0 123 times the mass of the earth and separation between them is 3.84 × 10⁸ m. Determine the location of C.M as measured from the centre of the earth.

Solution:

Answer:
The location of centre of mass as measured from the center of the earth is 4.67 × 10⁶ m.

Question 77.
Three particles of masses 3 g, 5 g and 8 g are situated at point (2, 2, 2), (-3, 1, 4) and (-1, 3, -2) respectively. Find the position vector of their centre of mass.
Solution:
m₁ = 3 g, m₂ = 5 g, m₃ = 8 g, x₁ = 2, y₁ = 2, z₁ = 2
x₂ = -3, y₂ = 1, z₂ = 4,
x₃ = -1, y₃ = 3, z₃ = -2
Let (X, Y, Z) be co-ordinates of C.M., then

The co-ordinates of the C.M. are \(\left(-\frac{17}{16}, \frac{35}{16}, \frac{5}{8}\right)\)
Answer:
The position vector of the C.M. is \(-\frac{17}{16} \hat{\mathbf{i}}+\frac{35}{16} \hat{\mathbf{j}}+\frac{5}{8} \hat{\mathbf{k}}\)

Question 78.
In the HCl molecule, the separation between the nuclei of the two atoms is about 1.27 Å (1 Å = 10^-10 m). Find the approximate location of the CM of the molecule, given that a chlorine atom is about 35.5 times as massive as a hydrogen atom and nearly all the mass of an atom is concentrated in its nucleus. (NCERT)
Solution:

Claculation: From formula

Answer:
The location of centre of mass from the nucleus of hydrogen atom is 1.235 A.

Question 79.
Three thin walled uniform hollow spheres of radii 1 cm, 2 cm and 3 cm are so located that their centres are on the three vertices of an equilateral triangle ABC having each side 10 cm. Determine centre of mass of the system.

Solution:
Mass of a thin walled uniform hollow sphere is proportional to its surface area. (as density is constant) hence proportional to r².

Thus, if mass of the sphere at A is m_A = m, then m_B = 4m and m_C = 9m. By symmetry of the spherical surface, their centres of mass are at their respective centres, i.e., at A, B and C. Let us choose the origin to be at C, where the largest mass 9m is located and the point B with mass 4m on the positive x-axis. With this, the co-ordinates of C are (0, 0) and that of B are (10, 0). If A of mass m is taken in the first quadrant, its co-ordinates will be \([5,5 \sqrt{3}]\)

Question 80.
Locate the centre of mass of three particles of mass m₁ = 1 kg, m₂ = 2 kg and m₃ = 3 kg at the corner of an equilateral triangle of each side of 1 m.
Solution:



Answer:
The centre of mass of the system of three particles lies at \(\left(\frac{2}{3} m, \frac{\sqrt{3}}{6} m\right)\) with respect to the particle of mass 1 kg as the origin.

Question 81.
A letter ‘E’ is prepared from a uniform cardboard with shape and dimensions as shown in the figure. Locate its centre of mass.

Solution:
As the sheet is uniform, each square can be taken to be equivalent to mass m concentrated at its respective centre. These masses will then be at the points labelled with numbers 1 to 10, as shown in figure. Let us select the origin to be at the left central mass m₅, as shown and all the co-ordinates to be in cm.

By symmetry, the centre of mass of m₁, m₂ and m₃ will be at m₂ (1, 2) having effective mass 3m. Similarly, effective mass 3m due to m₈, m₉ and m₁₀ will be at m₉ (1, -2). Again, by symmetry, the centre of mass of these two (3m each) will have co-ordinates (1, 0). Mass m₆ is also having co-ordinates (1, 0). Thus, the
effective mass at (1, 0) is 7m.

Using symmetry for m4, m5 and m7, there will be effective mass 3m at the origin (0, 0). Thus, effectively, 3m and 7m are separated by 1 cm along X-direction. Y-coordinate is not required.

Question 82.
A hole of radius r is cut from a uniform disc of radius 2r. Centre of the hole is at a distance r from centre of the disc. Locate centre of mass of the remaining part of the disc.
Solution:
Before cutting the hole, c.în. of the full disc was at its centre. Let this be our origin O. Centre of mass of the cut portion is at its centre D. Thus, it is at a distance x₁ = r form the origin. Let C be the centre of mass of the
remaining disc, which will he on the extension of the line DO at a distance x₂ = x from the origin. As the disc is uniform, mass of any of its part is proportional to the area of that part.

Thus, if m is the mass of the cut disc, mass of the entire disc must be 4m and mass of the remaining disc will be 3m.

Alternate method: (Using negative mass):

Let \(\overrightarrow{\mathrm{R}}\) be the position vector of the centre of mass of the uniform disc of mass M. Mass m is with centre of mass at position vector \(\overrightarrow{\mathrm{r}}\) from the centre of the disc be cut out from the complete disc. Position vector of the centre of mass of the remaining disc is then given by

Question 83.
Define centre of gravity of a body. Under what conditions the centre of gravity and centre of mass coincide?
Answer:

1. centre of gravity of a body is the point around which the resultant torque due to force of gravity on the body is zero.
2. The centre of mass coincides with centre of gravity when the body is in a uniform gravitational field.

Question 84.
Explain how to find the location of centre of mass or centre of gravity of a laminar object.
Answer:

1. A laminar object is suspended from a rigid support at two orientations.
2. Lines are drawn on the object parallel to the plumb line as shown in the figure.
3. Plumb line is always vertical, i.e., parallel to the line of action of gravitational force.
4. Intersection of the lines drawn is then the point through which line of action of the gravitational force passes for any orientation. Thus, it gives the location of the c.g. or c.m.
   

Question 85.
Why do cricketers wear helmet and pads while playing? Is it related with physics?
Answer:
Helmet and pads used by cricketers protects the head, using principles of physics.

1. The framing between the interior of the helmet and pads increases the time over which the impulse acts on the head resulting into reduction of force.
   (Impulse = force × time = constant)
2. The pads spread the force applied by the ball over a wider area reducing pressure at a point.

Question 86.
The diagram shows four objects placed on a flat surface.

The centre of mass of each object is marked M. Which object is likely to fall over?
Answer:
Object C will fall because its centre of mass is not exactly at centre, in turn applying more force on one side of the object resulting in to unbalance force. Whereas, in other objects center of mass is exactly at center resulting into zero rotational or translational motion maintaining their equilibrium.

Question 87.
A boy is about to close a large door by applying force at A and B as shown. State with a reason, which of the two positions, A or B, will enable him to close the door with least force.

Answer:
Boy has to apply more force at point B as compared to point A, because point B is at least distance from the hinge of the door while point A is at maximum distance from hinge of the door. Opening of door applies a moment, which is. given by,
M = F × perpendicular distance
F ∝ \(\frac{1}{\text { distance }}\)
More is the distance from the axis of rotation less will be the force.

Question 88.
How is a seat belt useful for safety?
Answer:
When car hits another car or an object with high speed it applies a high impulse on the driver and due to inertia driver tends to move in forward direction towards the steering. Seat belts spreads the force over large area of the body and holds the driver and protects him from crashing at the steering.

Question 89.
According to Newton’s third law of motion for every action there is equal and opposite reaction, why two equal and opposite forces don’t cancel each other?
Answer:
Forces of action and reaction always act on different bodies, hence they never cancel each other.

Question 90.
Linear momentum depends on frame of reference, but principle of conservation of linear momentum is independent of frame of reference. Why?
Answer:
Observers in different frame find different values of linear momentum of a system, hence linear momentum depends upon frame of reference, but each would observe that the value of linear momentum does not change with time (provided the system is isolated), hence principle of conservation of linear momentum is independent of frame of reference.

Question 91.
Multiple choice Questions

Question 1.
A body of mass 2 kg moving on a horizontal surface with initial velocity of 4 m s^-1 comes to rest after two seconds. If one wants to keep this body moving on the same surface with a velocity of 4 m s^-1, the force required is
(A) 2 N
(B) 4 N
(C) 0
(D) 8 N
Answer:
(B) 4 N

Question 2.
What force will change the velocity of a body of mass 1 kg from 20 m s^-1 to 30 m s^-1 in two seconds?
(A) 1N
(B) 5 N
(C) 10 N
(D) 25 N
Answer:
(B) 5 N

Question 3.
A force of 5 newton acts on a body of weight 9.80 newton. What is the acceleration produced in m/s²?
(A) 0.51
(B) 1.96
(C) 5.00
(D) 49.00
Answer:
(C) 5.00

Question 4.
A body of mass m strikes a wall with velocity v and rebounds with the same speed. Its change in momentum is
(A) 2 mv
(B) mv/2
(C) – mv
(D) Zero
Answer:
(A) 2 mv

Question 5.
A force of 6 N acts on a body of mass 1 kg initially at rest and during this time, the body attains a velocity of 30 m/s. The time for which the force acts on a body is
(A) 10 second
(B) 8 second
(C) 7 second
(D) 5 second
Answer:
(D) 5 second

Question 6.
A bullet of mass 10 g is fired from a gun of mass 1 kg with recoil velocity of gun = 5 m/s. The muzzle velocity will be
(A) 30 km/min
(B) 60 km/min
(C) 30 m/s
(D) 500 m/s
Answer:
(D) 500 m/s

Question 7.
The velocity of rocket with respect to ground is v₁ and velocity of gases ejecting from rocket with respect to ground is v₂ Then velocity of gases with respect to rocket is given by
(A) v₂
(B) v₁ + v₂
(C) v₁ × v₂
(D) v₁
Answer:
(B) v₁ + v₂

Question 8.
Two bodies A and B of masses 1 kg and 2 kg moving towards each other with velocities 4 m/s and 1 m/s suffers a head on collision and stick together. The combined mass will
(A) move in direction of motion of lighter mass.
(B) move in direction of motion of heavier mass.
(C) not move.
(D) move in direction perpendicular to the line of motion of two bodies.
Answer:
(A) move in direction of motion of lighter mass.

Question 9.
Which of the following has maximum momentum?
(A) A 100 kg vehicle moving at 0.02 m s^-1.
(B) A 4 g weight moving at 1000 cm s^-1’.
(C) A 200 g weight moving with kinetic energy of 10^-6 J
(D) A 200 g weight after falling through one kilometre.
Answer:
(D) A 200 g weight after falling through one kilometre.

Question 10.
A bullet hits and gets embedded in a solid block resting on a horizontal frictionless table. What is conserved?
(A) Momentum alone.
(B) K.E. alone.
(C) Momentum and K.E. both.
(D) P.E. alone.
Answer:
(A) Momentum alone.

Question 11.
The force exerted by the floor of an elevator on the foot of a person standing there, is more than his weight, if the elevator is
(A) going down and slowing down.
(B) going up and speeding up.
(C) going up and slowing down.
(D) either (A) and (B).
Answer:
(D) either (A) and (B).

Question 12.
If E, G and N represents the magnitudes of electromagnetic, gravitational and nuclear forces between two electrons at a given separation, then,
(A) N = E = G
(B) E < N < G (C) N > G < E (D) E > G > N
Answer:
(D) E > G > N

Qestion 13.
For an inelastic collision, the value of e is
(A) greater than 1
(B) less than 1
(C) equal to 1
(D) none of these
Answer:
(B) less than 1

Question 14.
A perfect inelastic body collides head on with a wall with velocity y. The change in momentum is
(A) mv
(B) 2mv
(C) zero
(D) none of these.
Answer:
(A) mv

Question 15.
Two masses ma and rnb moving with velocitics v_a and v_b in opposite direction collide
elastically and after the collision m_a and m_b move with velocities v_b and v_a respectively. Then the ratio m_am_b is
(A) \(\frac{v_{a}-v_{b}}{v_{a}+v_{b}}\)
(B) \(\frac{\mathrm{m}_{\mathrm{a}}+\mathrm{m}_{\mathrm{b}}}{\mathrm{m}_{\mathrm{a}}}\)
(C) 1
(D) \(\frac{1}{2}\)
Answer:
(C) 1

Question 16.
The frictional force acts _____.
(A) in direction of motion
(B) against the direction of motion
(C) perpendicular to the direction of motion
(D) at any angle to the direction of motion
Answer:
(B) against the direction of motion

Question 17.
A marble of mass X collides with a block of mass Z, with a velocity Y. and sticks to it. The final velocity of the system is
(A) \(\frac{\mathrm{Y}}{\mathrm{X}+\mathrm{Y}} \mathrm{Y}\)
(B) \(\frac{X}{X+Z} Y\)
(C) \(\frac{X+Y}{Z}\)
(D) \(\frac{X+Z}{X}\)
Answer:
(B) \(\frac{X}{X+Z} Y\)

Question 18.
Two balls lying on the same plane collide. Which of the following will be always conserved?
(A) heat
(B) velocity
(C) kinetic energy
(D) linear momentum.
Answer:
(D) linear momentum.

Question 19.
A body is moving with uniform velocity of 50 km h^-1, the force required to keep the body in motion in SI unit is
(A) zero
(B) 10
(C) 25
(D) 50
Answer:
(A) zero

Question 20.
A coolie holding a suitcase on his head of 20 kg and travels on a platform. then work done in joule by the coolie is
(A) 198
(B) 98
(C) 49
(D) zero
Answer:
(D) zero

Question 21.
Out of the following forces, which force is non-conservative?
(A) gravitational
(B) electrostatic
(C) frictional
(D) magnetic
Answer:
(C) frictional

Question 22.
The work done in conservative force is ____.
(A) negative
(B) zero
(C) positive
(D) infinite
Answer:
(B) zero

Question 23.
The angle between the line of action of force and displacement when no work done (in degree) is
(A) zero
(B) 45
(C) 90
(D) 120
Answer:
(C) 90

Question 24.
If the momentum of a body is doubled, its KE. increases by
(A) 50%
(B) 300%
(C) 100%
(L)) 400%
Answer:
(B) 300%

Question 25.
In perfectly inelastic collision, which is conserved?
(A) P.E. only
(B) K.E. only
(C) momentum only
(D) K.E. and momentum
Answer:
(C) momentum only

Question 26.
In case of elaine collision, which s
(A) Momentum and K.E. is conserved.
(B) Momentum conserved and K.E. not conserved,
(C) Momentum not conserved and K.E. conserved.
(D) Momentum and K.E. both not conserved,
Answer:
(A) Momentum and K.E. is conserved.

Question 27.
Pseudo force is true only in
(A) frame of reference which is at rest
(B) inertial frame of reference.
(C) frame of reference moving with constant velocity.
(D) non-inertial frame of reference
Answer:
(D) non-inertial frame of reference

Question 28.
A men weighing 90kg carries a stone of 20 kg to the top of the building 30m high. The work done by hint is (g = 9.8 m/s²)
(A) 80 J
(B) 100 J
(C) 980 J
(D) 29,400 J
Answer:
(D) 29,400 J

Question 29.
A weight lifter is holding a weight of 100 kg on his shoulders for 45 s, the amount of work done by him in joules is
(A) 4500
(B) 100
(C) 45
(D) zero
Answer:
(D) zero

Question 30.
If m is the mass of a body and E its K.E..then its linear momentum is
(A) \(\mathrm{m} \sqrt{\mathrm{E}}\)
(B) \(2 \sqrt{\mathrm{m}} \mathrm{E}\)
(C) \(\sqrt{m} E\)
(D) \(\sqrt{2 \mathrm{mE}}\)
Answer:
(D) \(\sqrt{2 \mathrm{mE}}\)

Question 31.
Torque applied is masimum when the angle between the directions of \(\overrightarrow{\mathrm{r}}\) and \(\overrightarrow{\mathrm{F}}\) is
(A) 90°
(B) 180°
(C) 0°
(D) 45°
Answer:
(A) 90°

Question 92.
A particle moving with velocity \(\vec{v}\) is acted by three forces shown by the vector triangle PQR. The velocity of the particle will:
(A) remain constant
(B) change according to the smallest force \(\overrightarrow{\mathrm{QR}}\)
(C) increase
(D) decrease

Hint: As the three forces acting on a particle represents a triangle (i.e., a closed loop)
∴ F_net = 0
∴ m \(\vec{a}\) = 0
∴ m\(\frac{\mathrm{d} \overrightarrow{\mathrm{v}}}{\mathrm{dt}}\)
∴ v remains constant
Answer:
(A) remain constant

Question 93.
A force F = 20 + 10y acts on a particle in y-direction where F is in newton and y in meter. Work done by this force to move the particle from y = 0 to y = 1 m is:
(A) 25J
(B) 20J
(C) 30J
(D) SJ
Hint: Work done by variable force, W = \(\int_{y_{\text {initial }}}^{y_{\text {final }}} \mathrm{Fdy}\)


Answer:
(A) 25J

Question 94.
Body A of mass 4m moving with speed u collides with another body B of mass 2m, at rest. The collision is head on and elastic in nature. After the collision the fraction of energy lost by the colliding body A is:
(A) \(\frac{4}{9}\)
(B) \(\frac{5}{9}\)
(C) \(\frac{1}{9}\)
(D) \(\frac{8}{9}\)
Hint:
Fractional loss of K.E. of colliding bodies,

Answer:
(D) \(\frac{8}{9}\)

Question 95.
An object of mass 500 g, initially at rest, is acted upon by a variable force whose X-component varies with X in the manner shown. The velocities of the object at the points X = 8 m and X = 12 m, would have the respective values of

(A) 18m/s and 20.6 m/s
(B) 18 m/s and 24.4 m/s
(C) 23 m/s and 24.4 m/s
(D) 23 m/s and 20.6 m/s
Hint: From work-energy theorem
∆ K.E. = work = area under F-x graph
From x = 0 to x = 8m
\(\frac{1}{2} \mathrm{mv}^{2}\) = (5 × 20) + (3 × 10)
∴ \(\frac{1}{2} \mathrm{mv}^{2}\) = 100 + 30

Answer:
(D) 23 m/s and 20.6 m/s

Question 96.
The centre of mass of two particles system lies
(A) at the midpoint on the line joining the two particles.
(B) at one end of line joining the two particles.
(C) on the line perpendicular in the line joining two particles.
(D) on the line joining the Iwo particles.
Answer:
(D) on the line joining the two particles.

Question 97.
A block of mass m is placed on a smooth inclined wedge ABC of inclination θ as shown in the figure. The wedge is given an acceleration ‘a’ towards the right. The relation between a and θ for the block to remain stationary on the wedge is

Hint:

The mass of block is given to be m. It will remain stationary if forces acting on it are in equilibrium i.e., ma cos θ = mg sin θ
Here, ma = Pseudo force on block.
∴ a = g tan θ
Answer:
a = g tan θ

Question 98.
A moving block having mass m, collides with another stationary block having mass 4m. The lighter block comes to rest after collision, When the initial velocity of the lighter block is v, then the value of coefficient of restitution
(e) will
(A) 0.5
(B) 0.25
(C) 0.8
(D) 0.4
Hint:
Given: m₁ = m, m₂ = 4m, u₁ = v, u₂ = 0, v₁ = 0 According to law of conservation of momentum,
m₁u₁ + m₂u₂ = m₁v₁ + m₂v₂
mv + 4m × 0 = m × 0 + 4mv₂

Answer:
(B) 0.25

Question 99.
In a collinear collision, a particle with an initial speed v₀ strikes a stationary particle of the same mass. If the final total kinetic energy is 50% greater than the original kinetic energy, the magnitude of the relative velocity between the two particles, after collision, is:

Hint:
According to law of conservation of momentum,
mv₀ = mv₁ + mv₂

Answer:
(D) \(\sqrt{2} \mathbf{v}_{0}\)

Question 100.
The mass of a hydrogen molecule is 3.32 × 10^-27 kg. If 10²³ hydrogen molecules strike, per second, a fixed wall of area 2 cm² at an angle of 45° to the normal and rebound elastically with a speed of 10³ m/s, then the pressure on the wall is nearly:
(A) 2.35 × 10² N/m²
(B) 4.70 × 10² N/m²
(C) 2.35 × 10³ N/m²
(D) 4.70 × 10³ N/m²
Hint:

Answer:
(C) 2.35 × 10³ N/m²

Question 101.
A bomb at rest explodes into 3 parts of same mass. The momentum of two parts is -3P\(\hat{\mathrm{i}}\) and 2P\(\hat{\mathrm{j}}\) respectively. The magnitude of momentum of the third part is
(A) P
(B) \(\sqrt{5} \mathrm{P}\)
(C) \(\sqrt{11} \mathrm{P}\)
(D) \(\sqrt{13} \mathrm{P}\)

Answer:
(D) \(\sqrt{13} \mathrm{P}\)

Question 102.
A sphere of mass ‘m’ moving with velocity V collides head-on on another sphere of same mass which is at rest. The ratio of final velocity of second sphere to the initial velocity of the first sphere is (e is coefficient of restitution and collision is inelastic)

Hint:
Initial momentum = mv
Final momentum = mv₁ + mv₂

Answer:
(C) \(\frac{\mathrm{e}+1}{2}\)

Question 103.
Two blocks A and B of masses 3m and m respectively are connected by a massless and inextensible string. The whole system is suspended by a massless spring as shown in figure. The magnitudes of acceleration of A and B immediately after the string is cut, are respectively:

Hint:
Tension in spring before cutting the strip,

Answer:
(B) \(\frac{\mathrm{g}}{3}\), g

Question 104.
A time dependent force F = 6t acts on a particle of mass 1 kg. If the particle starts from rest, the work done by the force during the first 1 sec. will be
(A) 9 J
(B) 18 J
(C) 4.5 J
(D) 22 J
Hint:
F = 6t
m = 1 kg
∴ a = 6t

Answer:
(C) 4.5 J

Question 105.
Consider a drop of rain water having mass Ig falling from a height of 1 km. It hits the ground with a speed of 50 m/s. Take ‘g’ constant with a value 10 m/s². The work done by the
(i) gravitational force and the
(ii) resistive force of air is:
(A) (i) -10 J
(ii) -8.25 J
(B) (i) 1.25 J
(ii) -8.25 J
(C) (i) 100 J
(ii) 8.75 J
(D) (i) 10 J
(ii) -8.75 J
Hint: Work done by gravitation force is given by (W_g)

Answer:
(D) (i) 10 J
(ii) -8.75 J