Class 9th Sanskrit Anand Chapter 1 सुष्ठु गृहीतः चौरः Question Answer Maharashtra Board
Balbharti Maharashtra State Board Class 9 Sanskrit Solutions Anand Chapter 1 सुष्ठु गृहीतः चौरः Notes, Textbook Exercise Important Questions and Answers.
Std 9 Sanskrit Chapter 1 Question Answer
Sanskrit Anand Std 9 Digest Chapter 1 सुष्ठु गृहीतः चौरः Textbook Questions and Answers
भाषाभ्यास:
1. उचितं पर्यायं चिनुत ।
प्रश्न 1.
कस्य धनस्यूत: लुप्त: ?
(अ) देवदत्तस्य
(आ) वीरबलस्य
(इ) भृत्यस्य
(ई) नृपस्य
उत्तरम् :
(अ) देवदत्तस्य
![]()
प्रश्न 2.
गृहे कति भृत्याः आसन् ?
(अ) पञ्च
(आ) सप्त
(इ) षड्
(ई) एक:
उत्तरम् :
(इ) षड्
प्रश्न 3.
वीरबलः कस्य मित्रम् ?
(अ) भृत्यस्य
(आ) धनिकस्य
(इ) यष्टिकायाः
(ई) चौरस्य
उत्तरम् :
(आ) धनिकस्य
![]()
प्रश्न 4.
‘श्व: मम चौर्यं प्रकटितं भवेत्।’ इति चिन्ता कस्य चित्ते जायते?
(अ) दासस्य
(आ) वीरबलस्य
(इ) धनिकस्य
(ई) नृपस्य
उत्तरम् :
(अ) दासस्य
2. कः कं वदति ?
प्रश्न 1.
“पश्यन्तु एता: मायायष्टिकाः।”
उत्तरम् :
वीरबल: सेवकान् वदति।
प्रश्न 2.
“मित्र, अद्य सायङ्काले तव सर्वान् भृत्यान् मम गृहं प्रेषय।”
उत्तरम् :
वीरबल: देवदत्तं वदति।
![]()
3. माध्यमभाषया उत्तरं लिखत । वीरबलेन चौरः कथं गृहीतः ?
प्रश्न 1.
माध्यमभाषया उत्तरं लिखत । वीरबलेन चौरः कथं गृहीतः ?
उत्तरम् :
बिरबल हा सम्राट अकबराचा अतिशय चतुर आणि हजरजबाबी मंत्री होता. त्याच्या चातुर्याच्या अनेक कथा प्रसिद्ध आहेत. त्यांपैकीच एक म्हणजे ‘सुगृहीत: चौर:’. बिरबलाने स्वत:च्या चातुर्यान कसा चोर पकडला, याबाबत ही गोष्ट आहे.
देवदत्त नावाच्या एका श्रीमंत माणसाची पैशाची पिशवी चोरीला गेल्यावर तो आपल्या सेवकांवर संशय घेतो. आपल्या सहा सेवकांपैकीच कोणी एक चोर असणार, याची त्याला खात्री असते. चोराला पकडण्यासाठी तो आपल्या मित्राकडे म्हणजे बिरबलाकडे मदत मागतो. त्यानुसार एके संध्याकाळी बिरबल त्या सहा नोकरांना आपल्या घरी बोलावून समान उंचीची एकेक काठी देतो.
त्या काठ्या जादूच्या असून तुमच्यापैकी जो कोणी चोर असेल, त्याची काठी अंगठ्याएवढी लांब होईल, अशी भीतीही दाखवतो. आपापल्या काठ्या घेऊन दुसऱ्या दिवशी सकाळी यावे असे तो सेवकांना सांगतो. सहापैकी पाच चोर रात्री निश्चिंतपणे झोपतात. मात्र खऱ्या चोराच्या मनात भीती निर्माण होते.
आपली काठी अंगठ्याएवढी लांब होऊन आपली चोरी उघडकीस येण्यापेक्षा अगोदरच काठी कापलेली बरी, असा विचार करून तो काठी कापतो. दुसऱ्या दिवशी बिरबल एकच आखूड काठी बघून चोराला ओळखतो आणि त्याला पकडतो. खजील झालेला चोर क्षमा मागतो आणि पैशाची पिशवी परत देतो.
माणसाची मानसिकता कशी असते हे बिरबल जाणत होता. चोराच्या मनात चांदणे याचा अर्थ जाणून बिरबलाने सर्व साधले. खरा चोर स्वत:ची चोरी उघडकीस येणार नाही यासाठी नक्कीच प्रयत्न करेल. मनुष्याची हीच मानसिकता लक्षात घेऊन बिरबलाने युक्ती केली. अशा प्रकारे केवळ आपल्या युक्तीच्या जोरावर बिरबल चोराला पकडण्यात यशस्वी होतो.
Birbal was a very clever and witty minister in Emperor Akbar’s court. There are several famous accounts of his cleverness. One among them is the story “The thief is well – caught’ – ‘सु गृहीत: चौर:’ This story revolves around Birbal’s cleverness correctly identifying the thief.
When Devdutta realised his money bag was missing he suspected that one of the six servants would be the culprit. To catch the thief, he took Birbal’s help.
Birbal used his understanding of human psychology that the real thief would always do something to conceal his theft. Knowing this Birbal gave the servants a stick each of the same length saying that those sticks were magical and only the thief’s stick would grow longer the next day. The real thief fell for this ploy and cut his stick so no one would know that he was the thief.
However, the next day the thief’s stick was shorter than the others’. Birbal easily identified the thief due to his short stick. Thus, Birbal used his cleverness and understanding of human behavior to catch the thief.
![]()
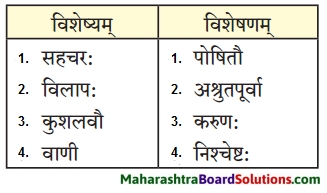
4. स्तम्भमेलनं कुरुत।
प्रश्न 1.
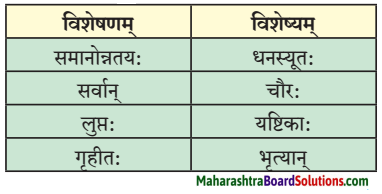
उत्तरम् :
| विशेषणम् | विशेष्यम् |
| समानोन्नतयः | यष्टिकाः |
| सर्वान् | भूत्यान् |
| लुप्तः | धनस्यूतः |
| गृहीत: | चौरः |
5. विरुद्धार्थकशब्दानां युग्मं चिनुत लिखत च।
प्रश्न 1.

उत्तरम् :
- पूर्वम् × पश्चात्
- गताः × आगताः
- दीर्घा × हस्वा
- जागृतः × सुप्तः
- भृत्यः × स्वामी
- धनिकः × दरिद्रः
![]()
6. पाठात् समानार्थक शब्दं चित्वा रेखाचित्रं पूरयत ।
प्रश्न 1.

उत्तरम् :
- धनिकः
- चौरः
- सुप्तः
- भृत्य:
Sanskrit Anand Class 9 Textbook Solutions Chapter 1 सुष्ठु गृहीतः चौरः Additional Important Questions and Answers
एकवाक्यन उत्तरत।
प्रश्न 1.
क: धनिकः आसीत्?
उत्तरम् :
देवदत्त : धनिकः आसीत्।
प्रश्न 2.
सेवका: कदा वीरबलस्य गृहम् अगच्छन् ?
उत्तरम् :
सेवका: सायकाले वीरबलस्य गृहम् अगच्छन्।
![]()
प्रश्न 3.
वीरबलेन सर्वेषां कृते का: आनीता:?
उत्तरम् :
वीरबलेन सर्वेषां कृते समानोन्नतय: मायायष्टिका: आनीताः ।
प्रश्न 4.
चौरस्य यष्टिका कीदृशी भविष्यति ?
उत्तरम् :
चौरस्य यष्टिका अगुलिमात्र दीर्घा भविष्यति।
प्रश्न 5.
रात्रौ सर्वे भृत्याः किम् अकुर्वन् ।
उत्तरम् :
रात्री सर्वे भृत्याः सुखेन निद्राम् अकुर्वन् ।
प्रश्न 6.
किं दृष्ट्वा वीरबालेन चौर: ज्ञात:?
उत्तरम्
लघुष्टिकां दृष्ट्वा वीरबालेन चौर: ज्ञातः।
![]()
प्रश्न 7.
अपराधी भृत्यः किम् अकरोत्?
उत्तरम्
अपराधी भृत्यः अपराधम् अङ्गीकृतवान् धनस्यूतं च प्रत्यर्पितवान्।
प्रश्न 8.
अयं गद्यांश: कस्मात् पाठात् उद्धृतः?
उत्तरम्
अयं गटांशः ‘सुष्ठु गृहीत : चौर: पाठात् उद्धृतः।
सत्यं वा असत्यं लिखत।
प्रश्न 1.
- देवदत्तः निर्धनः आसीत्।
- देवदत्तस्य धनस्यूत: चोरितः।
- देवदत्तस्य गृहे षड्भृत्येषु एक: चौरः ।
- वीरबल: देवदत्तं साहाय्यम् अयाचत।
- यष्टिकाः भिन्नोन्नतयः आसन्।
- सेवकै: यष्टिका: न स्वीकृताः।
- चौरस्य यष्टिका पूर्वमेव दीर्घा।
उत्तरम् :
- असत्यम्
- सत्यम्
- सत्यम्
- असत्यम्
- असत्यम्
- असत्यम्
- असत्यम्
![]()
प्रश्न 2.
- षद् सेवका: सुखेन निद्याम् अकुर्वन्।
- चौरेण यष्किा कीयत्वा हस्वा कृता।
- सर्वेषां राष्टिका: दीर्घा: भूताः।
- लघुष्टिकाया: साहाय्येन चौर: गृहीतः।
- चौर क्षमा प्राथ्यं धनस्यूतं प्रत्यर्पितवान्।
उत्तरम :
- असत्यम्
- सत्यम्
- असत्यम्
- सत्यम्
- सत्यम्
उचितं कारणं चित्वा वाक्यं पुनर्लिखत।
प्रश्न 1.
देवदत्तः वीरबलं साहाय्यम् अयाचत यत: ……..
a. देवदत्त: आरक्षक: आसीत्।
b. चतुर: वीरबल: देवदत्तस्य मित्रम् आसीत्।
उत्तरम् :
देवदत्त: वीरबलं साहाय्यम् अयाचत यत: चतुर: बीरबल: देवदत्तस्य मित्रम् आसीत्।
प्रश्न 2.
सर्वे सेवका: वीरबलस्य गृहम् अगच्छन् यतः …….
a. वीरबलेन भोजनसमारोहः आयोजितः ।
b. वीरबल: तान् परीक्षितुम् ऐच्छत्।
उत्तरम् :
सर्वे सेवका: वीरबलस्य गृहम् अगच्छन् यतः वीरबल: तान् परीक्षितुम् ऐच्छत्।
प्रश्न 3.
वीरबल: सर्वेभ्यः सेवकेभ्य: यष्टिकाम् अयच्छत् यतः ………..
a. सः चौरं ग्रहीतुम् ऐच्छत्।
b. सेवका: यष्टिकाम् अयाचन्त।
उत्तरम् :
वीरबल:सर्वेभ्य:सेवकेभ्य: यष्टिकाम् अयच्छत् यत: स: चौर ग्रहीतुम् ऐच्छत्।
![]()
प्रश्न 4.
एक सेवक: निद्रा न अकरोत् यतः
a. सः अस्वस्थः आसीत्।
b. ‘मम चौर्य प्रकटितं भवेत्’ इति विचारेण स: भीतः।
उत्तरम् :
एक: सेवक: निटांन अकरोत् यतः मम चौर्व प्रकटितं भवेत्’ इति विचारेण स: भीतः।
प्रश्न 5.
चौरेण यतिका लघूकता यतः …………।
a. स: चौयं प्रकटयितुं न ऐच्छत्।
b. तस्य यष्टिका पूर्वमेव दीर्घा आसीत्।
उत्तरम् :
चौरेण यष्टिका लाकृता यत:, स; चौर्य प्रकटयितुं न ऐच्छन्।
प्रश्न 6.
वीरबलेन चौर: सम्यक् ज्ञात: बत्तः …….
a. चौरस्य यष्टिका दोषों अभवत्।
b. चौरस्य यटिका हस्वा अभवत्।
उत्तरम् :
बीरबलेन चौर: सम्यक् ज्ञात: यत: चौरस्य यष्टिका हस्वा अभवत्।
![]()
कः कं वदति।
प्रश्न 1.
प्रात: मम चौर्य प्रकटितं भवेत्।
उत्तरम्
चौर:/सेवक: आत्मानं वदति।
शब्दस्य वर्णविग्रहं कुरुत।
- देवदत्तः – द् + ए + व + अ + अ + त् + त् + अः।
- धनस्यूतः – ध् + अ + न् + अ + स् + य् + ऊ + त् + अः।
- सायङ्काले – स् + आ + य् + अ + ङ् + क् + आ + ल् +ए।
- भृत्यान् – भ + ऋ + त् + य् + आ + न्।
- यष्टिकाः – य् + अ + ष् + ट् + इ + क् + आः।
- एकस्मात् – ए + क् + अ + स् + म् + आ + त्।
- ऋते – ऋ + त् + ए।
- अङ्गुलिमात्रम् – अ+ ङ् + ग् + उ + ल् + इ + म् + आ + त् + र् + अ + म्।
- कर्तयामि – क् + अ + र + त् + अ + य् + आ + म् + इ।
- चिन्तयित्वा – च + इ + न् + त् + अ + य + इ + त् + व् + आ।
- अन्येयुः – अ + न् + य् + ए + द् + य + उः।
- ज्ञात: – ज् + ञ् + आ + त् + अः।
![]()
प्रश्ननिर्माणं कुरुत।
प्रश्न 1.
- देवदत्तः धनिकः आसीत्।
- देवदत्तस्य गृहे षट् भृत्याः आसन्।
- चौर ग्रहीतुं देवदत्त: वीरबल साहाय्यम् अयाचत।
- सर्वे सेवका: सायङ्काले वीरबलस्य गृहम् आगताः।
- वीरबलेन सर्वेषां कृते समानोन्नतय: यष्टिका: आनीताः।
उत्तरम् :
- कः धनिकः आसीत्?
- देवदत्तस्य गृहे कति भृत्या: आसन्?
- चौरं ग्रहीतुं देवदत्तः कं साहाय्यम् अयाचत?
- सर्वे सेवका: सायडकाले कुत्र आगताः?
- वीरबलेन सर्वेषां कृते कीदृश्य : यष्टिका: आनीताः?
![]()
प्रश्न 2.
रात्री सर्वे भृत्या: सुखेन निद्राम् अकुर्वन्।
उत्तरम् :
कदा सर्वे भृत्या: सुखेन निद्राम् अकुर्वन् ।
प्रश्न 3.
लघुष्टिकां दृष्ट्वा वीरबलेन चौर: सम्यक् ज्ञातः ।
उत्तरम् :
लघुष्टिकां दृष्ट्वा केन चौर: सम्यक् ज्ञात:।
प्रश्न 4.
भृत्य अपराधम् अङ्गीकृतवान् ।
उत्तरम् :
मृत्यः किम् अङ्गीकृतवान्?
विशेषण-विशेष्य-सम्बन्धः।
| विशेषणम् | विशेष्यम् |
| धनिकः | देवदत्तः |
| षड् | भृत्याः |
| आगताः | सेवकाः |
| आनीताः | यष्टिका: |
| चोरितः | धनस्यूतः |
| दीर्घा | यष्टिका |
| प्रकटितम् | चौर्यम् |
| ज्ञातः | चौरः |
| अपराधी | भृत्यः |
![]()
त्वान्त/ल्यबन्त/तुमन्त अव्ययानि।
| त्वान्त अव्यय धातु + त्वा / ध्वा ट्वा / ड्वा / अयित्वा | ल्यबन्त अव्यय उपसर्ग + धातु + य / त्य | तुमन्त अव्यय धातु + तुम् / धुम् / दुम् / डुम् / इतुम् / अयितुम् |
| – | स्वीकृत्य | ग्रहीतुम् |
| कृत्वा | – | – |
| चिन्तयित्वा | – | – |
| दृष्ट्वा | – | – |
| पतित्वा | – | – |
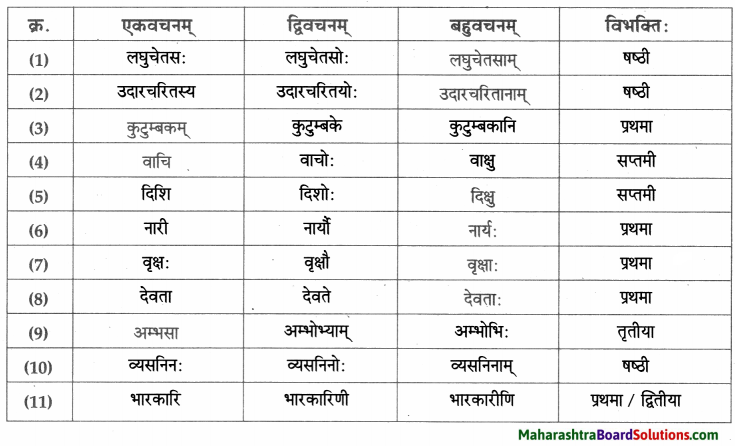
विभक्त्यन्तरूपाणि।
- प्रथमा – देवदत्तः, धनिकः, धनस्यूतः, भृत्याः, षड, चौरः, वीरबलः, सेवकाः, यष्टिकाः, सः, एताः, मायायष्टिकाः, दीर्घा, सर्वे, भृत्याः, अहम, चौरः, भृत्यः, गृहीतः।
- द्वितीया – चौरम्, वीरबलम्, साहाय्यम्, सर्वान, भृत्यान, गृहम, सेवकान्, एकाम, यष्टिकाम्, निद्राम, चौर्यम्, यष्टिकाम्, लघुष्टिकाम, अपराधम्, धनस्यूतम्
- तृतीया – सुखेन, वीरबलेन, सर्वैः, वीरबलेन, येन, केन।
- पञ्चमी – एकस्मात्
- षष्ठी – मम, वीरबलस्य, स्वस्य, तस्य, वीरबलस्य, सर्वेषाम्, मम, देवदत्तस्य।
- सप्तमी – रात्री, पादयोः, तासु, प्रभाते, गृहे।
![]()
पाठात् समानार्थक शब्दं चित्वा रेखाचित्रं पूरयत।
प्रश्न 1.
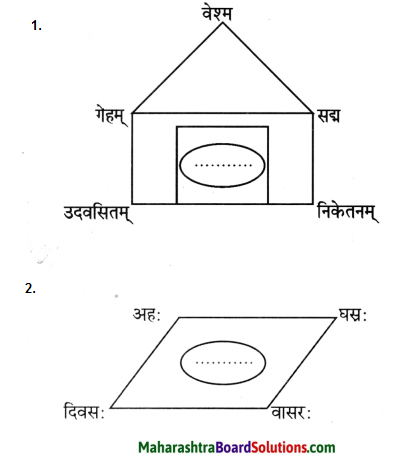
उत्तरम् :
1. गृहम्
2. दिनः
समानार्थकशब्दं योजयित्वा वाक्यं पुनर्लिखत।
निद्रा – निद्रा बालकं पीडयति। स्वापः / सुप्तिः / स्वपा / शयनं बालकं पीडयति।
दिनः – अद्य रम्य: दिनः । अद्य रम्य: दिवस: / अहः।.
![]()
व्याकरणम्
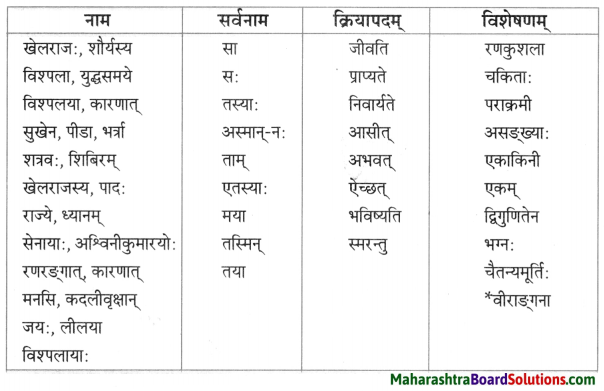
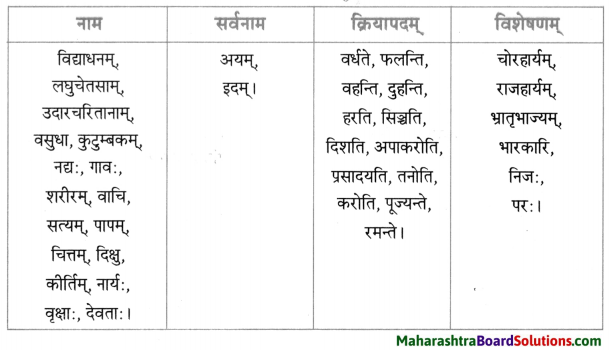
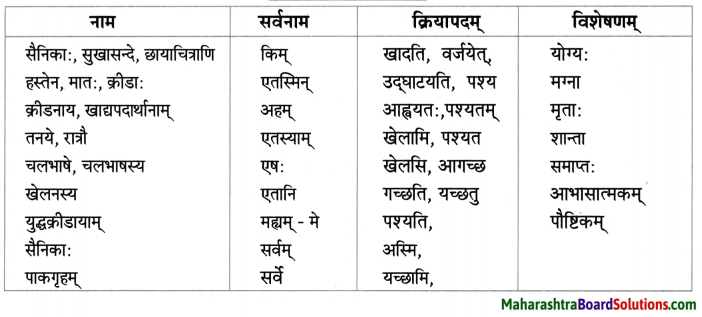
नाम – तालिका।
| नाम | सर्वनाम | क्रियापदम् | विशेषणम् |
| देवदत्तः | कः | कर्तयामि | धनिकः |
| धनिकः | सः | अयाचत | लुप्तः |
| धनस्यूतः | तव | अवदत् | षड् |
| गृहे | येन | अचिन्तयत् | सर्वान् |
| चौरम् | केन | प्रेषय | आगताः |
| भृत्यान् | मम | पश्यतु | समानोन्नतयः |
| मित्र | अहम् | स्यात् | आनीताः |
| वीरबलस्य | तेषु | भविष्यति | चोरितः |
| यष्टिका: | तेषाम् | आसन् | दीर्घा |
| सेवकान् | सर्वान् | अकुर्वन् | गृहीतः |
| रात्रौ | सर्वेषाम् | – | प्रकटितम् |
| यष्टिकाम् | एता: | – | ज्ञात: |
| पादयोः | सर्वैः | – | गृहीत: |
| धनस्यूतम् | सर्वै | – | अपराधी |
समासाः।
| समस्तपदम् | अर्थ: | समासविग्रहः | समासनाम |
| धनस्यूतः | bag of money | धनस्य स्यूतः। | षष्ठी तत्पुरुष समास |
![]()
सुष्ठु गृहीतः चौरः Summary in Marathi and English
प्रस्तावना :
मूल्य रुजविण्यासाठी, कौशल्यविकास तसेच मनोरंजनासाठी कथांचा परिणामकारकपणे वापर फार पूर्वीपासून होत आहे. प्रस्तुत कथेत एका चोराला पकडण्यासाठी बिरबलाने योजलेल्या युक्तीतून फक्त त्याचे चातुर्यच नव्हे तर त्याला असलेले मानवी स्वभावाचे ज्ञानही दिसून येते.
परिस्थिती अनुकूल असेपर्यंत आपल्या चुका लपवण्याचा मानवी स्वभाव असतो. या कथेतील चोराला पकडण्यासाठी बिरबल देवदत्ताच्या सहा सेवकांना काळ्या देतो आणि फक्त चोराचीच काठी बोटभर लांब होईल, अशी भीती घालतो. आपली चोरी लपविण्यासाठी खरा चोर अगोदरच काठी कापून टाकतो आणि दुसऱ्या दिवशी आखूड काठी बघून बिरबल चोराला ओळखतो.
Stories have been the medium of teaching values, skills and providing entertainment since ages. This story revolves around how Birbal used not just his cleverness, but also his knowledge of human behaviour to identify the thief.
A man always tries to hide his mistake or wrongdoing and therefore will not be at exse till he finds a way to take care of it. Birbal gave Deudutta’s six servants & stick each and said that only the thief’s stick would become longer the next day.
The real thief cut his stick so that he would not be caught. But Birbal immediately identified the thief, the one whose stick was shorter than the rest.
![]()
परिच्छेद : 1
देवदत्त: …………….. भविष्यति।
देवदत्त: नाम कोऽपि धनिकः आसीत्। एकदा तस्य धनस्यूतः लुप्तः । तस्य गृहे षड् भृत्याः आसन्। तेषु एव एक: चौरः स्यात्, इति तस्य मतम्। चौर ग्रहीतुं सः वीरबलं साहाय्यम् अयाचत । वीरबल: अवदत, “मित्र ! अद्य सायङ्काले तव सर्वान् भृत्यान् मम गृहं प्रेषय” इति। यथानिश्चितं सर्वे सेवका; वीरबलस्य गृहम् आगताः। वीरबलेन पूर्वमेव सर्वेषां कृते समानोन्नतय: यष्टिका: आनीताः । सः सेवकान् अवदत, “भोः पश्यन्तु, एताः मायायष्टिका: । तासु एका यष्टिकां स्वीकृत्य सर्वे: देवदत्तस्य गृहं गन्तव्यं , श्वः प्रभाते पुनरागन्तव्यम्। येन केनापि धनस्यूत: चोरित: स्यात् तस्य यष्टिका अङ्गुलिमात्रं दीर्घा भविष्यति।”
अनुवाद:
देवदत्त नावाचा कोणी एक श्रीमंत माणूस होता. एकदा त्याची पैशांची पिशवी (थैली) हरवली. त्यांच्या घरी सहा सेवक होते. त्यांच्यापैकीच कोणी एक चोर असेल, असे त्याचे मत होते.
चोराला पकडण्यासाठी त्याने विरबलाकडे मदत मागितली. बिरबल म्हणाला, “मित्रा, आज संध्याकाळी तुझ्या सर्व सेवकांना माझ्या घरी पाठव.” ठरल्याप्रमाणे सगळे सेवक बिरबलाच्या घरी आले. बिरबलाने अगोदरच सगळ्यांसाठी समान उंचीच्या काठ्या आणल्या होत्या.
तो सेवकांना म्हणाला, “हे बघा, या जादुई काठ्या आहेत. त्यातील एक काठी घेऊन सर्वांनी देवदत्ताच्या घरी जावे, उद्या सकाळी परत यावे. ज्या कोणाकडून पिशवी चोरली गेली असेल त्याची काठी बोटभर लांब होईल.”
There was a certain rich man name Devadutta. Once his money bag was missing. There were six servants in his house. It was his opinion that one among them would surely be the thief. He sought Birbal’s help in order to catch the thief. Birbal said, “O friend, send all your servants to my house today, in the evening.
As decided, all the servants came to Birbal’s house. Birbal had brought sticks of the same length for all of them in advance. He said to the servants, “O see, these are magical sticks.
Taking a stick among those, you all go back to Devadutta’s house and come again tomorrow morning. The stick of the one who has stolen the money bag will become longer by a finger.
![]()
परिच्छेद : 2
रात्री ………… प्रत्यर्पितवान्।
रात्री सर्वे भृत्या: सुखेन निद्राम् अकुर्वन् ऋते एकस्मात् स: अचिन्तयत्, “व: प्रात: मम चौर्य प्रकटितं भवेत्। अत: अहम् अधुना एव मम यष्टिकाम् अङ्गलिमात्रं कर्तयामि।” इति चिन्तयित्वा तथा कृत्वा सः सुखेन सुप्तः। अन्येाः लघुष्टिकां दृष्ट्वैव वीरवलेन चौर: सम्यक् ज्ञात: गृहीत: च। अपराधी भृत्य: वीरबलस्य पादयोः पतित्वा स्वस्य अपराधम् अङ्गीकृतवान् धनस्यूतं च प्रत्यर्पितवान्।
अनुवादः
रात्री एकजण वगळता सगळे सेवक शांतपणे झोपले. त्याने विचार केला, “उद्या सकाळी माझी चोरी उघड होईल (प्रकाशात येईल). म्हणून मी आताच माझी काठी बोटभर कापतो.”
असा विचार करून ठरवल्याप्रमाणे करून तो शांतपणे झोपला. दुसऱ्या दिवशी आखूड काठी पाहून बिरबलाने चोराला उत्तमरीत्या (व्यवस्थित) ओळखले आणि पकडले. अपराधी सेवकाने बिरबलाच्या पाया पडून स्वतःचा अपराध मान्य केला आणि पैशांची (थैली) परत दिली.
At night except one, all the servants slept peacefully. He thought, “Tomorrow morning my theft will be revealed. So I shall cut my stick by a finger length now itself.” Thinking that, he did so and slept peacefully.
The next day just by looking at the short stick Birbal correctly recognised and caught the thief. The guilty servant fell at Birbal’s feet and accepted his mistake and returned the money bag.
![]()
सन्धिविग्रहः
- कोऽपि – कः + अपि।
- पूर्वमेव – पूर्वम् + एव।
- पुनरागन्तव्यम् – पुन: + आगन्तव्यम्।
- केनापि – केन + अपि।
- दृष्ट्वैव – दृष्ट्वा + एव।
![]()
समानार्थकशब्दाः
- धनिकः – धनी, धनाक्यः, धनवान, धनसमपत्रः, सधन:,
इभ्यः, श्रीमान्। - भृत्यः – सेवकः, परिचारकः, दासः, दासेयः, किङ्करः,
- चौरः – स्तेनः, तस्करः, मोषकः, पाटच्चरः।
- मित्रम् – सखा, वयस्यः , सुहृद्।
- गृहम् – गेहम्, सदनम्, निकेतनम्, वेश्म, आलयः।
- अवदत् – अभणत्, अकथयत्।
- प्रभाते – प्रात:काले।
- सुष्टु – सम्यक्तया।
- स्वीकृत्य – गृहीत्वा।
- भः – अन्येयुः, अपरेयुः।
- रात्रौ – निशायाम्।
- निद्रा – स्वापः, स्वपा, शयनम् सुप्तिः।
- अधुना – साम्पतम्, इदानीम्।
- दृष्ट्वा – वीक्ष्य, विलोक्य, अवलोक्य।
- ऋते – विना।
- सुप्तः – निद्रितः, निद्राधीनः।
- चौर्यम् – लण्ठनम्।
![]()
विरुद्धार्थकशब्दाः
- धनिकः × दरिद्रः, दीनः।
- दीर्घा × न्हस्वा
- आगताः × गताः, निर्गताः।
- भृत्यः × स्वामी।
- पूर्वम् × पश्चात्।
- स्वीकृत्य × त्यक्त्वा।
- श्वः × अद्य, ह्यः।
- मित्रम् × शत्रुः।
- सायङ्काले × प्रात:काले।
- रात्री × प्रभाते।
- सुखेन × चिन्तया, सचिन्तम्।
- निद्रा × जागृतिः।
- सुप्त: × जागृतः।
![]()
शब्दार्थाः
- धनिक – rich man – श्रीमंत
- धनस्यूतः – money bag – पैशांची पिशवी(थैली)
- भृत्याः – servants – सेवक
- स्यात् – would be – असेल
- ग्रहीतुम् – to catch – पकडण्यासाठी
- अयाचत – sought/asked – याचना केली
- अद्य – today – आज
- प्रेषय – send – तू पाठव
- गन्तव्यम – should – जावे
- यथानिश्चितम् – as decided – ठरवल्याप्रमाणे
- आगताः – came – आले
- पूर्वमेव – in advance – अगोदरच
- समानोन्नतयः – same length – समान उंचीच्या (मापाच्या)
- यष्टिकाः – sticks – काठ्या
- आनीताः – brought – आणल्या
- मायायष्टिकाः – magical sticks – जादुई काठ्या
- पुनरागन्तव्यम् – come again – पुन: यावे
- स्वीकृत्य – taking – स्वीकार करून, घेऊन
- अङ्गुलिमात्रम् – by a finger – बोटभर
- श्चः – tomorrow – उद्या
- चोरितः – stolen – चोरलेला
- दीर्घा – long – लांब
- भविष्यति – will become – होईल
- ऋते – except – शिवाय
- चौर्यम् – theft – चोरी
- प्रकटितं भवेत् – will be revealed – उघड होईल
- कर्तयामि – I shall cut – मी कापतो
- चिन्तयित्वा – thinking – विचार करून
- सुप्त: – slept – झोपला
- अन्येयुः – the next day – दुसऱ्या दिवशी
- दृष्ट्वा – looking – पाहून
- सम्यक् – correctly – उत्तमरीत्या
- ज्ञातः – recognised – ओळखला गेलेला
- गृहीतः – caught – पकडला गेलेला
- पतित्वा – falling – पडून
- अङ्गीकृतवान् – accepted – मान्य केला / कबूल केला
- प्रत्यर्पितवान् – returned – परत दिला
9th Standard Sanskrit Anand Digest Pdf
- सुष्ठु गृहीतः चौरः Class 9 Question Answer
- अव्ययमाला Class 9 Question Answer
- किं मिथ्या ? किं वास्तवम् ? Class 9 Question Answer
- विध्यर्थमाला Class 9 Question Answer
- वीरवनिता विश्पला Class 9 Question Answer
- पिनकोड्प्रवर्तकः महान् संस्कृतज्ञः Class 9 Question Answer
- सूक्तिसुधा Class 9 Question Answer
- मनसः स्वच्छता Class 9 Question Answer
- अमरकोषः Class 9 Question Answer
- काव्यशास्त्रविनोदः Class 9 Question Answer
- मनोराज्यस्य फलम् Class 9 Question Answer