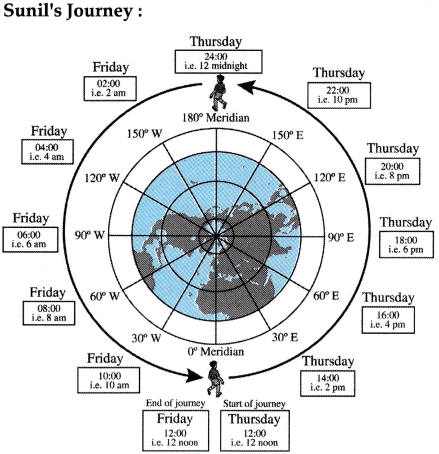
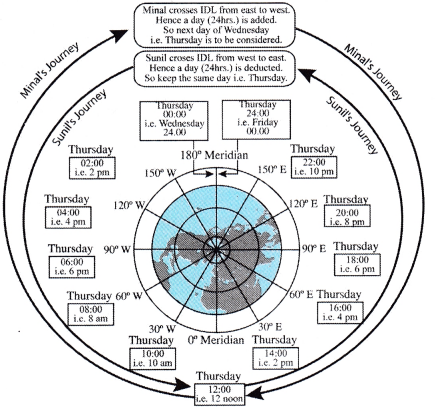
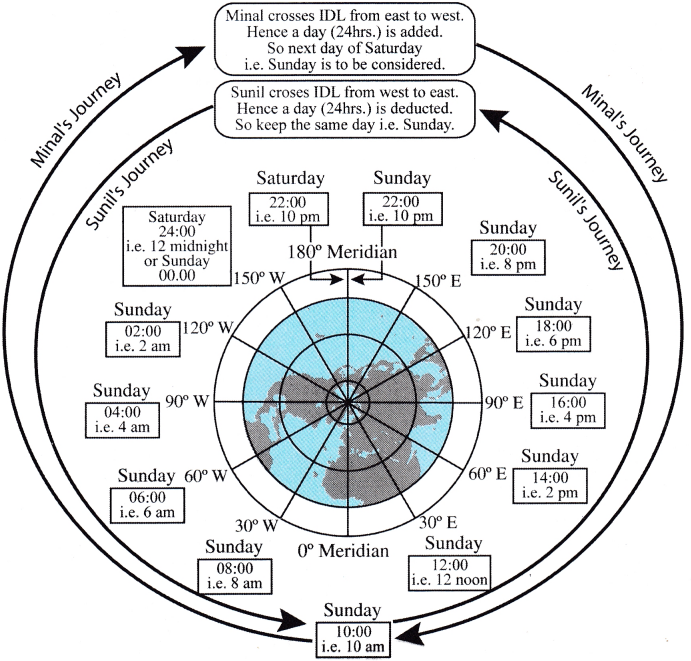
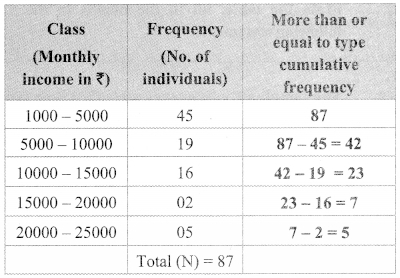
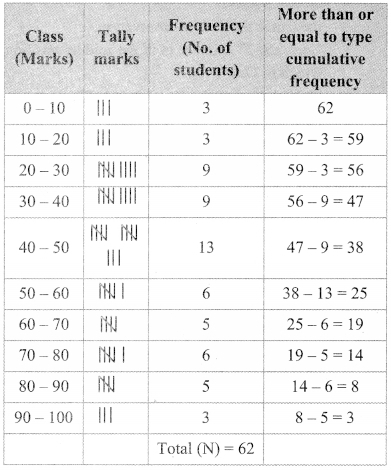
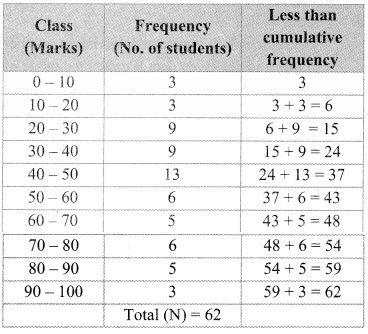
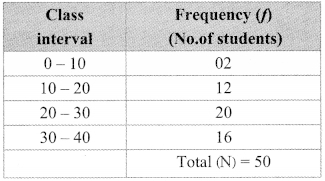
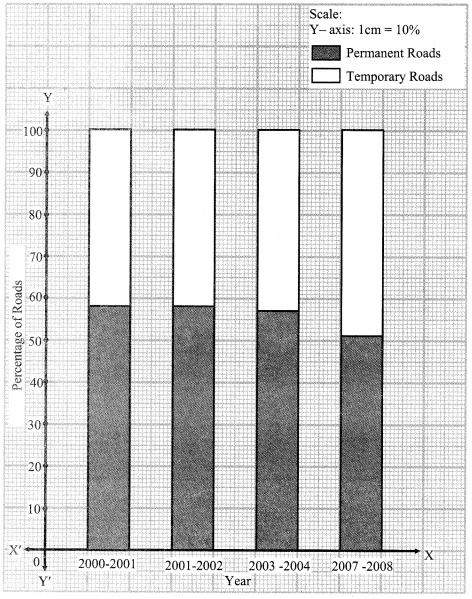
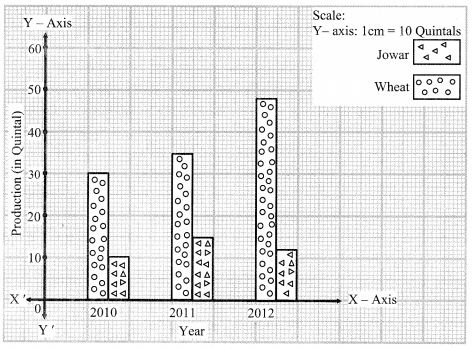
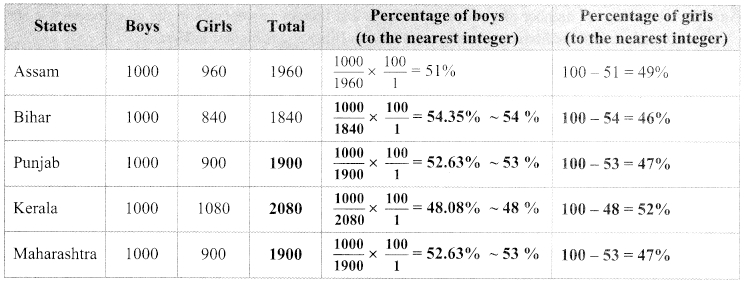
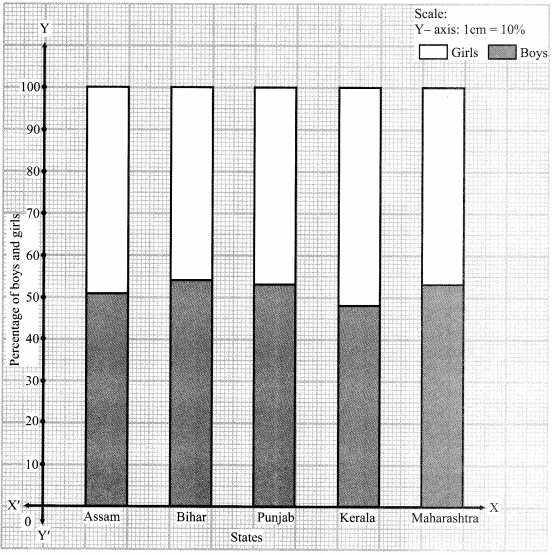
Balbharti Maharashtra State Board Class 9 Geography Solutions Chapter 12 Tourism Notes, Textbook Exercise Important Questions and Answers.
Tourism Class 9 Questions And Answers Maharashtra Board
Class 9 Geography Chapter 12 Tourism Questions And Answers Maharashtra Board
Geography Class 9 Chapter 12 Question Answer Maharashtra Board
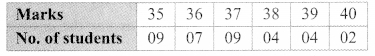
1. Identify the type of tourism from the statement.
(A) Hemant Kumar went to Mexico to learn about the architectural skills of the Mayan culture
Answer:
Cultural Tourism
![]()
(B) Portuguese tourists had come to Goa to participate in the Goa Carnival.
Answer:
Cultural Tourism
(C) To seek treatment in a naturopathy centre, John and Amar went to Kerala.
Answer:
Medical Tourism.
(D) Pundalikrao accomplished the Char Dham Yatra with his family
Answer:
Religious Tourism.
(E) Rameshwari from Pune went to enjoy Hurda ( Roasted Grain) party and learn about modern and traditional methods of agriculture
Answer:
Agro-tourism.
(F) Sayyed family went to Ajmer Durgah.
Answer:
Religious tourism.
2. Match the columns identifying the correlation. Make a chain.
| Group ‘A’ | Group ‘B’ | Group ‘C’ | |
| (1) | Tadoba | Madhya Pradesh | Lake |
| (2) | Bird Sanctuary | Agra | Butterflies |
| (3) | Sanjay Gandhi National Park | Manipur | Kailash caves |
| (4) | Taj Mahal | Nannaj | Film city |
| (5) | Ramoji Film City | Ellora | World-famous wonder |
| (6) | Radhanagari | Mumbai | Ancient cave- paintings |
| (7) | Bimbetka | Hyderabad | Great Indian Bustard (Maaldhok) |
| (8) | Ancient Caves | Kolhapur | Kanheri Caves |
| (9) | Eaglenest Wildlife Sanctuary | Chandrapur | Bison |
| (10) | Loktak | Arunachal Pradesh | Tiger |
Answer:
| Group ‘A’ | Group ‘B’ | Group ‘C’ | |
| (1) | Tadoba | Chandrapur | Tiger |
| (2) | Bird Sanctuary | Nannaj | Great Indian Bustard (Maaldhok) |
| (3) | Sanjay Gandhi National Park | Mumbai | Kanheri Caves |
| (4) | Taj Mahal | Agra | World-famous wonder |
| (5) | Ramoji Film City | Hyderabad | Film city |
| (6) | Radhanagari | Kolhapur | Bison |
| (7) | Bimbetka | Madhya Pradesh | Ancient cave- paintings |
| (8) | Ancient Caves | Ellora | Kailash caves |
| (9) | Eaglenest Wildlife Sanctuary | Arunachal Pradesh | Butterflies |
| (10) | Loktak | Manipur | Lake |
3. Answer in brief.
Question 1.
Differentiate between religious and cultural tourism.
Answer:
| Religious tourism | Cultural tourism |
| (i) religious tourism is a type of tourism in which tourists travel for pilgrimage. | Cultural tourism refers to a type of tourism in which tourists travel to cultural attractions away from residence to gather new information and experience. |
| (ii) In religious tourism various religious places, temples, churches, etc. are visited for performing religious activities and gaining spiritual peace. | In cultural tourism art, architecture, religion, customs, traditions, lifestyle, etc. of a particular region are experienced and studied. |
| (iii) For example, a visit to Shirdi, Haji Ali, Mount Mary Church, etc. | For example, Portuguese tourists participating in Goa carnival. |
Question 2.
What are the objectives of tourism?
Answer:
Following are the objectives of tourism:
- Getting joy
- Getting entertained
- Trading
- Staying
- Availing health facilities
- Getting a glimpse of agricultural life
- Performing religious activities, etc.
![]()
Question 3.
Explain the environmental effects of tourism.
Answer:
Following are the environmental effects of tourism:
- Sometimes, tourists damage tourist place and degrade the environment. Some tourists throw litter and pollute the tourist place.
- Therefore, all tourists must follow the concept of eco-tourism. Eco-tourism includes taking precautions of not degrading and polluting the environment as well as not damaging tourist places.
- Tourism is good for environmental development as the government makes economic investment in developing sanctuaries and national parks for the sake of the tourism industry.
- In eco-friendly tourism, factors like designing houses, resorts, traffic routes are designed in an eco-friendly manner in which electricity and water are used judiciously.
- The concept of recycling and reuse is also applied in eco-friendly tourism.
Question 4.
What opportunities develop with the development of tourism?
Answer:
- Tourism and Economic Development: The development of infrastructural facilities generates employment on an large scale. Eg. transportation, banking, restaurants, shops, resorts, medical services etc.
- Tourism and Environmental development: Due to the Eco-friendly tourism concept, tourist places are developed while caring for the environment. Eg. Eco-tourism.
- Tourism and’ Health: Opportunities to avail health facilities develop with tourism. Eg. Indian Ayurveda, Yoga, Pranayam.
- Tourism and Social Developments: Tourism creates social consciousness among tourists which helps in the development of neglected components of the society. For eg. Visit to Anandwan village.
Question 5.
Suggest measures after explaining the problems arising at the tourist places.
Answer:
(i) The problems that may arise at the tourist places:
- Lack of basic facilities.
- Lack of frequency of means of transportation.
- Lack of medical facilities.
- Lack of communication facilities.
- Petty crimes and security issues.
(ii) Measures to minimize the problems arising at the tourist places:
- Government can provide lodges, eateries, clean drinking water and good sanitation facilities.
- All tourist places can be connected by speedy means of transportation.
- Government can open dispensaries and arrange ambulance facilities.
- Communication facilities can be developed at every tourist place.
- Installation of CCTVs in public places and prompt action by the law and order forces can help tourist immensely.
![]()
Question 6.
Explain with the reason the places of interest that can be developed as tourist centers in your district.
Answer:
- In the district of Mumbai, there are many tourists destinations which fascinate the tourists.
- But there are few places which can be developed as tourist centres, which are currently neglected.
- To name a few, there are some small forts on the beaches of Mumbai and some caves in the suburbs of Mumbai.
- The Sion fort and the fort of Mahim are currently in a bad state and need urgent restoration.
- The Mahakali caves situated in western suburbs of Mumbai need to be developed in order to preserve cultural heritage which can attract many tourists.
(Disclaimer: The students can write answer of this above question in their own words as per the district they belong to.)
Question 7.
Local people get employment due to tourism. Give reasons.
Answer:
- Tourism is an important tertiary occupation through which many tourists visit places of interest.
- Many job opportunities are created to serve the tourists.
- When basic infrastructure improves, it leads to generation of employment.
- Many local people get job opportunities from various restaurants, transportation systems and recreational places.
5. Make a notice board containing instructions for the tourists at a tourist place.
Answer:

6. Explain how relevant is the concept of “Atithi Devo Bha va” (Guest is our God) with respect to tourism.
Answer:
- In Sanskrit “Atithi Devo Bhava” means “Guest is our God”. This is a tag line of India’s, Ministry of Tourism’s campaign to improve the treatment of tourists in India.
- India attracts millions of tourists every year but it still lags far behind compared to other destinations.
- With an attempt to improve the number of tourists travelling to India, the Tourism Department of India started this social awareness campaign which provides training and orientation to taxi drivers, guides, immigration officers, police and other personnel who interact directly with the tourists.
- This campaign educates the citizens of our country about the importance of our cultural heritage, benefits of cleanliness, importance of foreign tourists and maintaining our historical monuments.
- All these efforts will lead to the economic development of our country.
7. The tourism map of Maharashtra is given. Answer the following questions based on the map.
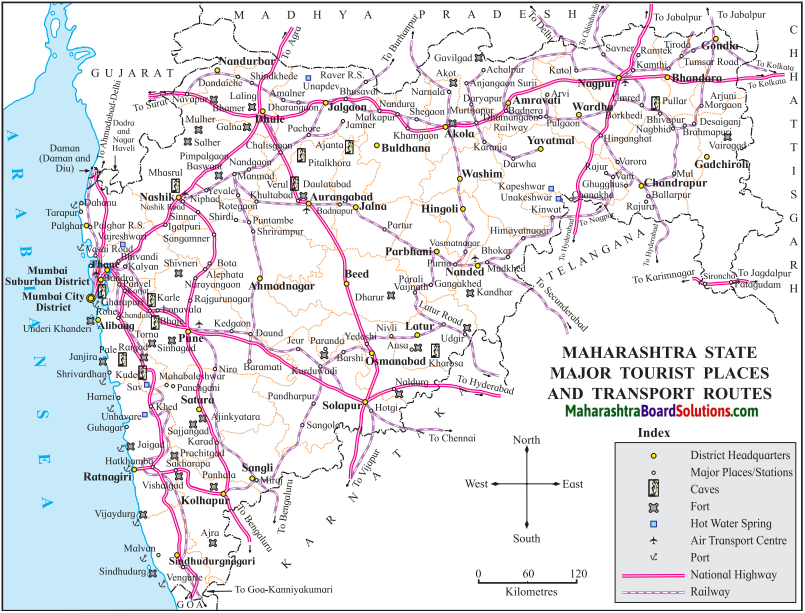
![]()
Question 1.
Make a list of places having hot springs. What are the reasons for these being located here?
Answer:
Say, Vajreshwari, Kapeshwar, Unakeshwar, linhavare, Unapdev are the places that have hot springs.
Reason of hot water springs because geothermally heated ground water rises from the Earth’s crust.
Question 2.
At what places do you find the correlation between transportation routes and the development of tourism?
Answer:
- Aurangabad: The national highway and the airport is located in Aurangabad which facilitates the tourists to visit Ajanta and Ellora caves.
- Shirdi: Since Shirdi has got a railway station, more devotees will visit the temple every year.
Class 9 Geography Chapter 12 Tourism Intext Questions and Answers
Can you tell?
(I) Dear Students, imagine that you have to go on a vacation with your family. Make a list of 15 favourite places in Maharashtra.
After the list is prepared, put your places in the relevant classification.
- Places near the sea
- Historical places
- National parks and sanctuaries
- Hill stations
- Religious places
Select one place from each group which you would like to visit.
Discuss the reasons of your choice in the class.
Answer:
(A) A list of 15 favourite places in Maharashtra
- Mumbai
- Pune
- Nashik
- Alibaug
- Guhagar
- Malvan
- Shirdi
- Shegaon
- Chandoli
- Pench
- Kamala
- Matheran
- Mahabaleshwar
- Jawhar
- Parli Vaijanath.
![]()
(B) A relevant classification of 15 favourite places is as follows:
(1) Places near the sea :
- Alibaug
- Guhagar
- Malvan.
(2) Historical places :
- Pune
- Mumbai
- Nashik.
(3) National parks and sanctuaries :
- Chandoli
- Pench
- Kamala.
(4) Hill stations:
- Matheran
- Mahabaleshwar
- Jawhar.
(5) Religious places :
- Parli Vaijanath
- Shirdi
- Shegaon.
(C) Name of a place from each group which we would like to visit with reason :
(1) Name of a place near the sea which we would like to visit: Guhagar.
Reason: Guhagar is famous for its clean white sand beach. ‘Shri Vyadeshwar’ an ancient shiv temple is also located at Guhagar.
(2) Name of a historical place which we would like to visit: Pune.
Reason : Many historical forts are located in Pune.
(3) Name of a national park and sanctuary which we would like to visit: Karnala.
Reason : Karnala bird sactuary houses many species of resident birds.
(4) Name of a hill station which we would like.to visit: Matheran.
Reason : One can reach Matheran by a Toy Train. Horse riding is another tourist attraction of Matheran. There are gardens, waterfalls, temples, markets and many scenic points at Matheran.
(5) Name of a religious place which we would like to visit: Shegaon.
Reason : A famous ‘Gajanan Maharaj Temple’ is located at Shegaon. Anand Sagar’, a spiritual and entertainment park centre is also located at Shegaon.
![]()
(II) (i) Which new forms of tourism have developed recently? (Textbook Page no. 92)
(ii) Tell the reasons why new forms of tourism emerge. (Textbook Page no. 92)
Answer:
(i) In recent years, following are the new forms of tourism that have developed:
- Eco-Tourism
- Agro-Tourism
- Film Tourism
- Medical Tourism
- Cultural tourism
(ii) Nowadays people are more aware of new tourist destinations and attractions, have the ability to spend and need break from their routine stressful life. They take breaks to refresh and relax or may travel for some psychological and social needs.
Think about it.
We saw the various types of tourism. Just think, can we go into the space for tourism? What will we have to do for that? Where can we go? Present your ideas in the form of picture or paragraphs, etc.
Answer:
Yes, we can go into the space for tourism. We will require a the most powerful rocket built with a spaceship designed to carry atleast 100 people. We can go to the Red Planet-Mars.
Use your brain power!
Some issues related to tourism development are given below. Rewrite the inappropriate ones after correcting them.
Answer:
- The income of a country should be substantially high for development of tourism. Inappropriate: The income of a country is high due to development of tourism.
- Domestic tourism should be given a boost. Inappropriate: Domestic tourism should be given a boost.
- Tourists from other countries should be banned. Inappropriate: Tourists from other countries should be welcomed for economic development of our country.
- Appropriate: Tourists should be assured of a safe journey.
- Appropriate: The cultural heritage of a country should be preserved.
- Appropriate: We should respect other country’s culture.
- Appropriate: Government subsidies1 and incentives should be given for tourism businesses.
- Appropriate: Participation in international sports should be increased.
- Appropriate: Tourism should be encouraged through advertisements.
- Appropriate: It is necessary to preserve the tourist places.
- Appropriate: We should preserve buildings of famous people who have contributed in various sectors for memorials.
- Appropriate: Services and amenities3 should be developed for tourism.
- Tourist agencies should be banned. Inappropriate: Tourists agencies should be encourage for their businesses.
- There is no ample scope in this field. Inappropriate: There is ample scope in the field of tourism.
- Appropriate: Tourism is a type of invisible trade.
- Appropriate: All types of facilities should be developed for tourists.
- The country’s economy does not benefit at all from tourism. Inappropriate: The country’s economy benefits a lot from tourism.
- Appropriate: The hidden potential of other sites should also be developed.
- Appropriate: The forts and castles should be developed.
![]()
Class 9 Geography Chapter 12 Tourism Additional Important Questions and Answers
Complete the following statements by choosing the appropriate alternatives from those given in the brackets:
Question 1.
Visiting various places away from our homes for getting joy, entertainment, trading, staying, etc. is called
(a) boarding
(b) lodging
(c) visiting
(d) tourism1
Answer:
(d) tourism
Question 2.
On the basis of political boundaries, tourism can be of two types and
(a) domestic tourism and local tourism
(b) international tourism and foreign tourism
(c) local tourism and holidays
(d) domestic tourism and international tourism
Answer:
(a) domestic tourism and international tourism
Question 3.
Tourism within the limits of a country is called …………………. .
(a) domestic tourism
(b) international tourism
(c) foreign tourism
(d) abroad tourism
Answer:
(a) domestic tourism
Question 4.
A person from Maharashtra going to Kanyakumari in Tamil Nadu for tourism, a person from Nagpur visiting Ellora Caves in Aurangabad are examples of
(a) international tourism
(b) abroad tourism
(c) domestic tourism
(d) foreign tourism
Answer:
(c) domestic tourism
Question 5.
Crossing country’s boundaries and visiting some other country for tourism is called
(a) local tourism
(b) international/ foreign tourism
(c) city tourism
(d) state tourism
Answer:
(b) international foreign tourism
![]()
Question 6.
Indian going to Switzerland for tourism, an American coming to India for tourism are examples of
(a) city tourism
(b) state tourism
(c) local tourism
(d) intemational/foreign tourism
Answer:
(d) international/ foreign tourism
Question 7.
………………….. tourism should be promoted as it is necessary to preserve the cultural heritage1 of a country.
(a) Mumbai
(b) Local
(c) Andheri
(d) Domestic
Answer:
(d) Domestic tourism
Question 8.
To go to a foreign country, one needs a ………………, ………………, travel insurance and other documents.
(a) passport, train tickets
(b) visa, bus tickets
(c) movie tickets, bus tickets
(d) passport, visa
Answer:
(d) passport and visa
Question 9.
The ……………… system in our smartphones or the GPS instrument is largely used for tourism. For that we need special applications like the
(a) GPRS and atlas
(b) GPS and radio
(c) GPS and google map
(d) google play store and GPRS
Answer:
(c) GPS and google map
Question 10.
Tourism is an important occupation.
(a) primary
(b) secondary
(c) tertiary
(d) quaternary
Answer:
(c) tertiary
![]()
Question 11.
Besides domestic tourists2, foreign tourists also visit various places of interest and therefore, ……………… currency increases in our economy.
(a) domestic
(b) foreign
(c) FOREX
(d) shares
Answer:
(b) foreign
Question 12.
Tourism should be promoted through in various media which will help in its growth.
(a) slogans
(b) word of mouth
(c) references
(d) advertisements
Answer:
(d) advertisements
Question 13.
……………… has implemented many programmes by providing guest houses, water sports, tourist houses near coastal areas, etc.
(a) Maharashtra Tourism Development Corporation (MTDC)
(b) Maharashtra Transportation Development Corporation (MTDC)
(c) Maharashtra Traffic Development Corporation (MTDC)
(d) Maharashtra Training Development Corporation (MTDC)
Answer:
(a) Maharashtra Tourism Development Corporation (MTDC)
Question 14.
……………… is a train started especially for tourism which is operated in coordination with MTDC, Indian Railways and Ministry of Tourism.
(a) Deccan Chronicle
(b) Deccan Express
(c) Mumbai Local
(d) Deccan Odyssey
Answer:
(d) Deccan Odyssey
Question 15.
………………….. train takes the Delhi-Jaipur-Udaipur-Bharatpur-Agra- Delhi route.
(a) Palace of Tiles
(b) Palace on Train
(c) Palace on Wheels
(d) Palace in Rajasthan
Answer:
(c) Palace on Wheels
![]()
Question 16.
The Indian railways have started a new train with coaches having transparent ceiling as a special attraction for tourism.
(a) superdome
(b) astrodome
(c) imax dome
(d) vistadome
Answer:
(d) vistadome
Question 17.
………………….. is an eco-friendly type of tourism.
(a) Medical tourism
(b) Eco-tourism
(c) Social tourism
(d) Religious tourism
Answer:
(b) Eco-tourism
Question 18.
A pollution-free location away from the city holding an agricultural activity where the tourist is shown a glimpse of agricultural life is called
(a) agro-tourism
(b) social tourism
(c) cultural tourism
(d) medical tourism
Answer:
(a) agro-tourism
Question 19.
In and districts of Maharashtra number of agro-tourism centres have been developed.
(a) Pune, Mumbai
(b) Mumbai, Nagpur
(c) Pune, Kolhapur
(d) Kolhapur, Amravati
Answer:
(c) Pune, Kolhapur
![]()
Question 20.
Places, where film shooting takes place, are and
(a) Mumbai film city, Ramoji city
(b) Pune city and Mahableshwar
(c) Ramoji city and Pune city
(d) Lonavala and Khandala
Answer:
(a) Mumbai film city, Ramoji City
Question 21.
………………….. in Konkan is famous for its seabed and the biodiversity found there.
(a) Juhu Beach
(b) Tarkali
(c) Alibaugh
(d) Sindhudurg
Answer:
(b) Tarkali
Question 22.
The tribal life in of Maharashtra and visit to model villages like project of the social activist Baba Amte, Ralegan Siddhi, Hiwre Bazaar, etc. creates social consciousness.
(a) Phonda Ghat, Vrindavan
(b) Melghat, Sunderbans
(c) Melghat, Anandwan
(d) Bor Ghat, Anandwan
Answer:
(b) Melghat, Anandwan
Answer in one sentence
Question 1.
What is meant by tourism?
Answer:
Visiting various places away from our homes for getting joy, entertainment, trading, staying etc. is called tourism.
Question 2.
By which name is the tourism within one’s own country’s boundaries is known?
Answer:
The tourism within one’s own country’s boundaries is known as ‘domestic tourism’.
Question 3.
What do mean by International tourism?
Answer:
Crossing country’s boundaries and visiting some other country for tourism is called international tourism.
![]()
Question 4.
A visit to which places in Maharashtra creates social consciousness?
Answer:
Visit to Melghat to see the tribal life and visit to model villages like Anandwan, Ralegansiddhi, Hiwre Bazaaro of Maharashtra creates social consciousness.
Question 5.
Anandwan’ is a project of which social activist?
Answer:
Anandwan’ is a project of the social activistt Baba Amte.
Question 6.
What is Cultural Tourism?
Answer:
Cultural tourism is a type of tourism in which tourists travel to collect information and to experience traditions, customs, art, lifestyle of people, etc. of a particular region.
Question 7.
What is Health Tourism?
Answer:
Health tourism is a type of tourism in which tourists travel for availing health facilities, achieving mental peace and physical strength.
Question 8.
What do we require when travelling abroad?
Answer:
To go abroad one requires a passport, visa, travel insurance, other documents and currency of the country where we are going.
![]()
Question 9.
Name the agro-tourism centres in Maharashtra.
Answer:
Pune and Kolhapur districts of Maharashtra have agro-tourism centres.
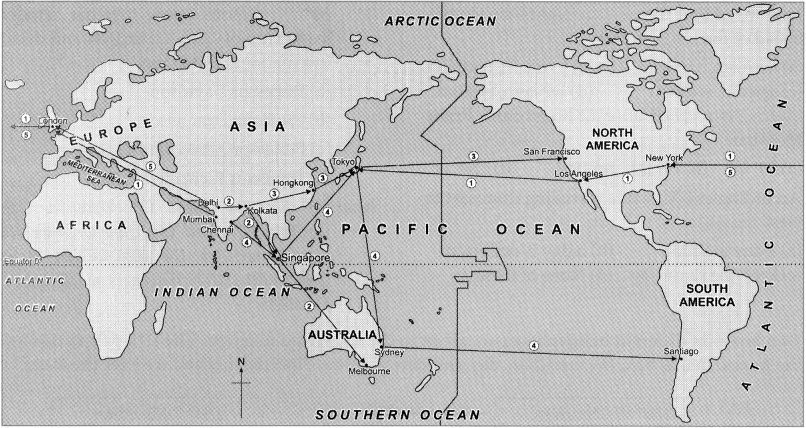
Observe fig. 12.1 and answer the following questions: Use an atlas for reference:
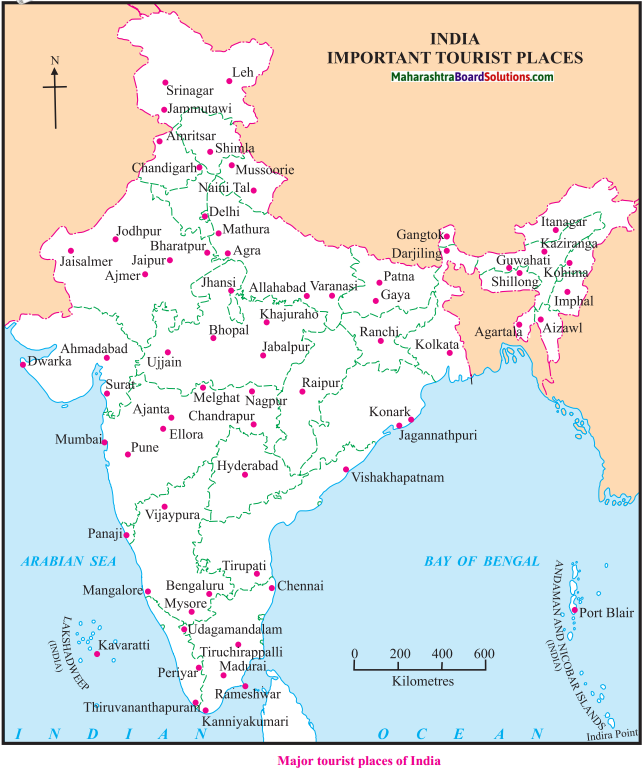
Question 1.
Out of these, which tourist places1 do you know? Make a list.
Answer:
A list of known tourist places :
- Leh
- Shimla
- Mussoorie
- Nainital
- Jaisalmer
- Ajanta
- Ellora
- Tirupati
- Udagamandalam
- Rameshwar
- Jagannath puri
- Konark
- Khajuraho
- Panaji.
![]()
Question 2.
For what are the selected places famous?
Answer:
The selected places are famous for the following:
- Leh, Shimla, Mussoorie, Naini Tal, etc. – famous for their natural beauty.
- Rameshwaram, Tirupati, Jagannathpuri – religious places.
- Ajanta, Ellora, Konark, Khajuraho – cultural sites.
- Mumbai, Pune, Delhi, Kolkata – historical sites
Question 3.
Make a list of religious and historical sites from the map.
Answer:
| Religious sites | Historical sites |
| Tirupati | Dwarka |
| Mathura | Agra |
| Varanasi | Jhansi |
| Konark | Jaipur |
Question 4.
Make a list of hill stations, places having sanctuaries and places along the coasts.
Answer:
(A) Hill stations –
- Shimla
- Massoorie
- Nainital
- Darjiling
(B) Sanctuaries –
- Maharashtra – Koyna, Wild life Sanctuary
- Goa – Bird sanctuary
- Gujarat – Indina wild Ass. sanctuary
- Karnataka – Bhadra wild life sanctuary
(C) Places along coast –
- Mumbai
- Panaji
- Mangalore
- Chennai
![]()
Question 5.
Correlate the physiography and tourist places in India.
Answer:
- There is a close relationship between physiography and tourist places in India.
- Many tourist places are developed at various locations having different physiography.
- The hill stations like Jammu, Srinagar, Nainital, Darjiling, Udagamandalam are developed in mountainous region of India.
- The tourist places like Ujjain, Pune, Jabalpur, etc. are located at plateau region of India.
- The tourist places Jodhpur, etc. are developed in desert area of India.
- The tourist places like Mumbai, Panaji, Mangalore, Kanniyakumari, Chennai, etc. are located in the coastal area of India.
The tourism map of Maharashtra is given below. Answer the following questions based on the map:
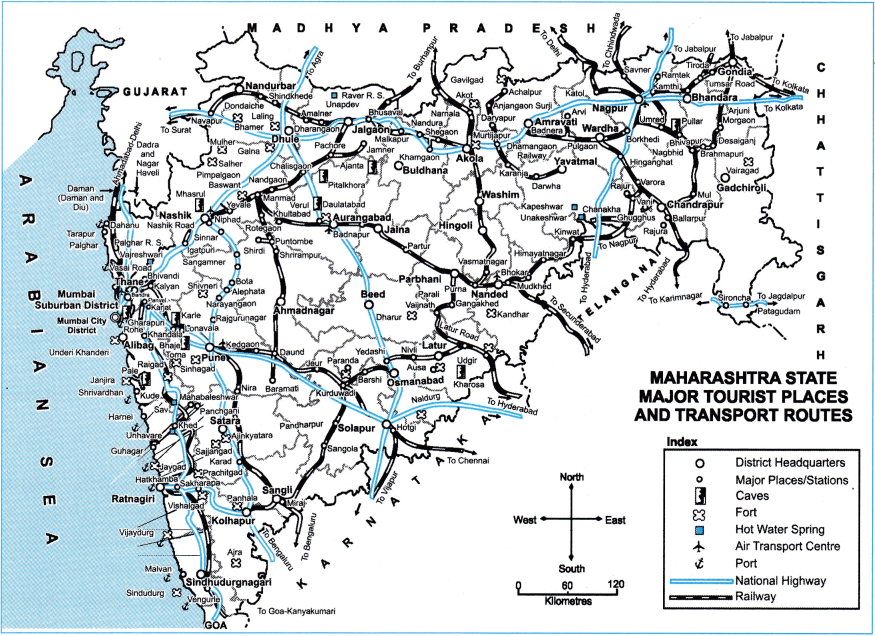
Question 3.
Name the ports of Konkan coast and is there any connectivity of ports with tourism?
Answer:
Mumbai, Vijaydurg, Sindhudurg, Ratnagiri, Jaygad, Harne, Shrivardhan.
Yes, there is a close connectivity between ports and tourism as tourists can reach such places by sea without wasting much time in travelling.
![]()
Question 4.
Name some of the caves in Maharashtra.
Answer:
Karle, Bhaje, Mhasrul, Ajanta, Verul, Kharosa, Pullar are some of the caves in Maharashtra.
Give reason
Question 1.
Social development be accomplished through tourism.
Answer:
- When facets1 like rural lifestyle, tribal life and culture are included in tourism then tourism gets a social dimension1 and the neglected components can be included in development.
- The tribal life of Mel ghat of Maharashtra and visit to model villages like ‘Anandwan’, a project of the social activist Baba Amte, Ralegansidhhi, Hiwre Bazaar, etc. creates social consciousness and gives a fillip to development there.
- There is a great potential2 for development of such a type of tourism in India through which social development can be accomplished.
Question 3.
Tourism is an invisible Trade’.
Answer:
- Invisible Trade is a trade in which exchange of services occurs. Example of invisible trade. Tourism is a type of invisible trade.
- Various restaurants, shops, transportation systems and recreational places are developed because of tourism and contribute directly to the economy.
- Also, basic infrastructure improves and employment is generated which is an advantage to the economy indirectly.
- Thus, tourism plays an important role in economic development and hence it is said that tourism is an invisible trade.
Question 4.
Tourism is good for environmental development.
Answer:
- Tourism is good for environmental development.
- Because of eco-friendly tourism concept, tourist places can be developed while caring for the environment.
- Factors like housing, resorts, traffic routes, etc. are also designed in an eco-friendly manner.
- In this type of development process, electricity, water are used very judiciously. The concept of recycle and reuse is also applied.
- Natural environment is maintained and tourism is developed too.
![]()
Answer in 2-3 Sentences.
Question 1.
What is the speciality of Deccan Odyssey?
Answer:
- Deccan Odyssey is a luxury train operated in co-ordination with MIDC, Indian Railways and Ministry of Tourism.
- Deccan Odyssey is like a moving palace.
- It originates at Mumbai and takes tourists to Nasik, Ellora, Ajanta, Koihapur, Goa and Ratnagiri.
Question 2.
Write about the luxury train for tourists visiting Northern india.
Answer:
- Palace on wheels” is the luxury train that covers the Northern India.
- This train takes the Deihi-Jaipur, Udaypur, Bharatpur, Agra Delhi route.
- Domestic arid foreign tourists enjoy travelling in this train.
Question 3.
Why is Vistadome a special attraction for tourists?
Answer:
- The coaches of Vistadome have a transparent ceiling which is a special attraction for tourists.
- It runs on the Vishakapatnam Kirandul route.
- As the train has a glass roof, the tourists can enjoy the panaromic view of the beautiful Arakuvalley, Anantgiri Ghat and Borra Coves.
Question 4.
What is Tarkarli in Konkan renowned for?
Answer:
- Tarkarli in Konkan is renowned for its seabed and biodiversity.
- Tourists can do snorkelling and scuba diving here.
- MTDC has set up a ‘scuba diving’ training centre of International standards at Tarkarli.
Question 5.
How is the GPS useful for tourists?
Answer:
- The GPS instrument found in applications like Google App location.
- Information regarding all possible routes of travel, distances, time require according to the type of vehicle, amenities on the way like petrol pump, restaurants etc. becomes available through GPS.
![]()
Write short note on:
Question 1.
GPS for tourism
Answer:
- The GPS system in our smartphones or the GPS instrument is largely used for tourism.
- For that we need special applications like the Google Map.
- We can know our exact location with the help of the map.
- Once it is decided where we want to head, then information regarding all the possible routes of travel, distances, time required according to type of vehicle, amenities on the way like petrol pump, restaurants, lodges, etc. becomes available in the app.
- We can use this for tourism.
Question 2.
MTDC
Answer:
- Maharashtra Tourism Development Corporation (MTDC) has implemented many programmes.
- It has provided guest houses, water sports, tourist houses near coastal areas, etc.
- “Deccan Odyssey” is a train started especially for tourism which is operated in coordination with MTDC, Indian Railways and Ministry of Tourism.
- The train originates at Mumbai and takes tourists to places like Nashik, Ellora, Ajanta, Kolhapur, Goa and Ratnagiri and back to Mumbai.
Question 3.
Domestic Tourism.
Answer:
- Tourism within the limits of the country is called Domestic Tourism.
- For example, a person from Maharashtra going to Kanyakumari in Tamil Nadu for a vacation, a person from Nagpur visiting Ellora Caves in Aurangabad and so on.
Question 4.
International Tourism.
Answer:
- Crossing the country’s boundaries and visiting some other country for tourism is called International Tourism.
Glossary - For example, an Indian going to Switzerland 1 for tourism, an American coming to India for tourism and so on.
Question 5.
Things required to travel to a foreign country.
Answer:
Following are the things required to travel to a foreign country:
- A Passport, visa, travel insurance and other documents.
- Currency of the country you are going to visit.
![]()
Question 6.
Eco-friendly tourism (Eco tourism).
Answer:
- The concept of eco-friendly tourism came into light when it was realised that due to increasing population, pollution and urbanization, environment is degrading.
- The nature of ecotourism is eco-friendly.
- In ecotourism, the tourists the place are visited by taking precautions of not damaging or degrading the environment.
- Ecotourism includes avoiding sound pollution, and air pollution, not hurting trees, birds, animals, etc. in the forests, etc.
Question 7.
Agro tourism.
Answer:
- Recently the concept of ‘agro-tourism’ has arisen which is visiting a pollution-free location away from the city holding an agricultural activity.
- In agro-tourism, the tourists are shown a glimpse of agricultural life.
- For a change from the urban life, tourists live on the farm and accept the hospitality of the farmers on payment in agro-tourism.
- In Pune and Kolhapur districts of Maharashtra number of agro-tourism centres have been developed.
Question 8.
Film tourism.
Answer:
- In film tourism, the locations where films are shot are shown to the tourists.
- A number of amenities and facilities are provided at such places to attract tourists.
- Mumbai film city, Ramoji film city are the famous film tourist places in India.
Question 9.
Coastal (sea) tourism
Answer:
- Coastal (sea) tourism is a new type of tourism.
- Tarkarli in Konkan is famous for snorkelling and scuba diving. It is also famous for its seabed and the biodiversity.
- MTDC has started a ‘scuba diving training centre’ of international standards at Tarkarli of Malwan taluka in Sindhudurg district.
Answer in brief:
Question 1.
Which new forms of tourism have developed recently?
Answer:
In recent years, the following are the new forms of tourism that have developed:
- Eco-tourism: While touring a place the environment should not be degraded or damaged by tourists, then it is called Ecotourism.

- Agro-tourism: Concept of ‘agro-tourism’ has arisen where pollution-free location away from the city holding an agricultural activity is visited. There the tourists are shown a glimpse of agricultural life.
- Film Tourism: Film tourism is a new type of tourism. The locations where films are shot are shown to tourists.
- Medical Tourism: The medicinal and surgical treatment in Indian hospitals are cost-effective and therefore, many patients from various developing countries come here for treatment.
- Medical Tourism develops from such facilities required by these tourists.
Question 2.
Explain the importance of tourist development in India.
Answer:
- India is diverse in terms of culture and nature, due to which India has a lot of potential for tourism.
- The richness of natural beauty in India, attractive landscapes and high mountains like Flimalayas exquisite coastal areas attract tourists.
- Also, the diversity of Indian culture, festivals, traditions and customs have resulted in opportunities for tourism in India.
Question 8.
What are the reasons of following medical tourists in India?
Answer:
- Many tourists visit India to avail health facilities, to achieve mental peace and physical strength through Indian Ayurveda, Yoga and Pranayam.
- The medicinal and surgical treatment in Indian hospitals are cost-effective and therefore many patients from various developing countries come here for treatment.
- Hence, medical Tourism develops from the facilities required by these tourists.
9th Std Geography Questions And Answers:
- Distributional Maps Class 9 Geography Questions And Answers
- Endogenetic Movements Class 9 Geography Questions And Answers
- Exogenetic Movements Part 1 Class 9 Geography Questions And Answers
- Exogenetic Movements Part 2 Class 9 Geography Questions And Answers
- Precipitation Class 9 Geography Questions And Answers
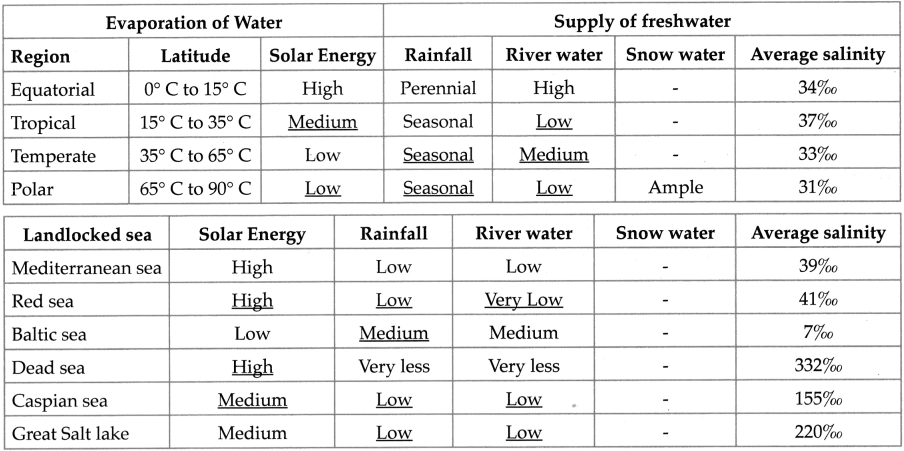
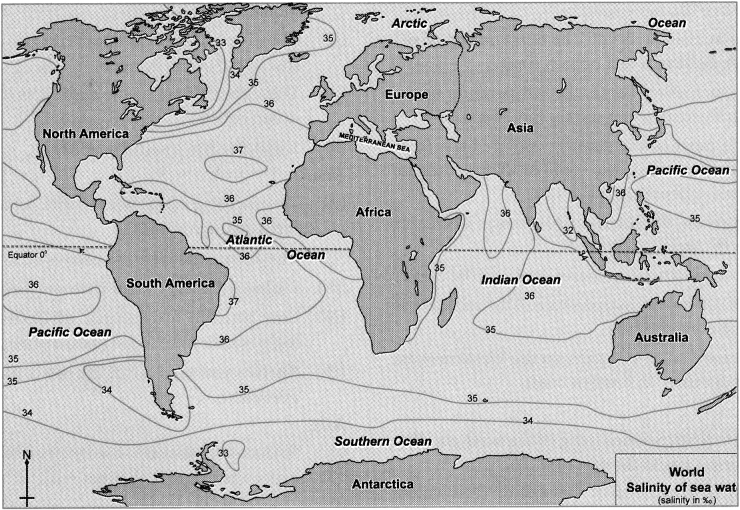
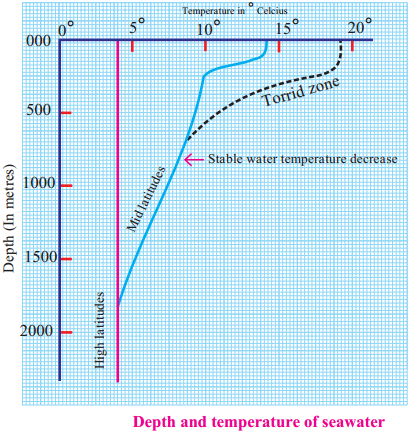
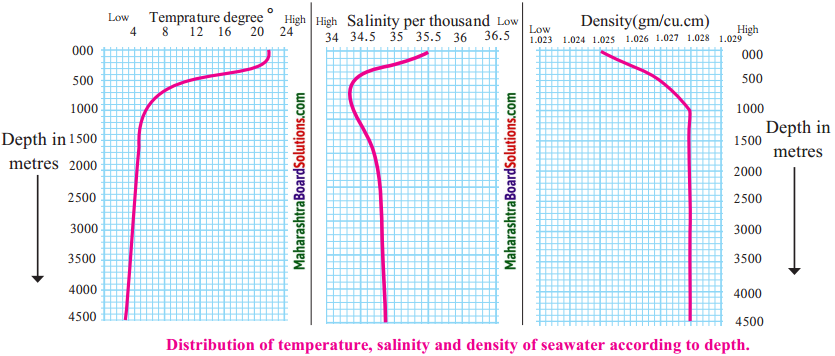
- Properties of Sea Water Class 9 Geography Questions And Answers
- International Date Line Class 9 Geography Questions And Answers
- Introduction to Economics Class 9 Geography Questions And Answers
- Trade Class 9 Geography Questions And Answers
- Urbanisation Human Class 9 Geography Questions And Answers
- Transport and Communication Class 9 Geography Questions And Answers
- Tourism Class 9 Geography Questions And Answers